Effect of Defective Graphene on Mechanical Properties of Reinforced Resin Matrix Composites
-
摘要: 基于分子结构力学和多尺度方法,采用有限元商业软件ABAQUS,针对石墨烯存在的几种缺陷,构建了含有不同数量的双原子空缺缺陷和Stone-Wales(S-W)缺陷的石墨烯增强环氧树脂复合材料的有限元模型,研究了石墨烯的缺陷在不同石墨烯体积分数下对复合材料弹性模量以及界面层应力传递的影响。数值模拟结果表明,随着石墨烯体积分数的增加,完美石墨烯和缺陷石墨烯增强环氧树脂复合材料弹性模量均呈现线性上升。其次,缺陷种类和数量之间的结果对比表明,空缺缺陷会明显降低复合材料的弹性模量,且随着缺陷数量的增加,变化更明显。而S-W缺陷在一定程度上增加了复合材料的杨氏模量,对于剪切模量,则出现下降趋势。另外,界面层的应力传递一定程度上反映了缺陷对复合材料的影响。Abstract: Based on the molecular structural mechanics and multi-scale method, the finite element model of graphene reinforced epoxy resin composite with different number of vacancy defects and Stone-Wales (S-W) defects was constructed by using the finite element commercial software ABAQUS.The effects of graphene defects on the elastic modulus and interfacial stress transfer of composites with different volume fractions of graphene were investigated.The numerical simulation results show that the elastic modulus of the perfect graphene and the defective graphene reinforced epoxy resin composite increases linearly with the increase of the volume fraction of graphene.Secondly, the comparison between the number of defects and the type of defects shows that the vacancy defect can obviously reduce the modulus of elasticity of the composite, and the change is more obvious with the increase of the number of defects.However, the S-W defect increases the Young's modulus of the composites to a certain extent, but the shear modulus decreases.In addition, the stress transfer from the interface layer to some extent reflects the effect of defects on the composites.
-
Key words:
- graphene /
- defect /
- composites /
- mechanical properties /
- multi-scale method
-
晶粒尺寸对多晶材料的力学性能具有很大的影响。霍尔-佩奇效应[1–2]指出,纳米/亚微米多晶材料具有更高的硬度。该理论已在超高压制备的纳米立方氮化硼[3]、亚微米立方氮化硼[4]、纳米孪晶立方氮化硼[5–6]、纳米多晶金刚石[7–8]、纳米孪晶金刚石[9]等多晶材料中得到应用。现有的亚微米/纳米多晶陶瓷块体材料多以纳米粉末为初始材料,在常压和低压(低于1 GPa)环境下[10–13]烧结制备。纳米粉末的表面能高,易团聚,在常压下由高温主导的烧结过程中通常会长大而失去纳米材料的特性[14–15],难以获得细晶结构的多晶陶瓷块体材料。此外,单分散纳米粉末的制备工艺复杂,在技术上存在一定的困难。因此,以纳米粉末为初始材料制备细晶结构的多晶陶瓷块体材料仍然是一个挑战。
压力(强)可以缩小原子间距、改变物质的电子轨道和键合方式,是决定物质存在状态及改变物质性质的基本热力学要素[16]。高压和相变相结合逐渐发展成为一种制备纳米/亚微米多晶陶瓷材料的有效方法[17–23]。高压可以抑制原子的长程扩散进而抑制晶粒长大,利用高压相变合成多晶材料的过程中新相具有更高的成核率[17, 20],并且在高压环境下晶体具有更慢的生长速率;陶瓷材料在特定的热力学条件下一般会发生相变(晶体结构变化),相变是一个“推陈出新”的过程,形成新相的晶粒尺寸与初始材料的晶粒尺寸无必然联系:因此,采用高压相变法可以获得小于初始粉末晶粒尺寸的多晶块体材料。Irifune等[7]利用高压相变方法,将多晶石墨直接转化成晶粒尺寸为10~20 nm的纳米多晶金刚石(Nano Polycrystalline Diamond, NPD),努普硬度达120~140 GPa。王海阔等[8]以晶粒尺寸 10
μ m的石墨为初始材料,合成出平均晶粒尺寸为20 nm、耐磨性和热稳定性优异的NPD。目前,以大颗粒粉晶(大单晶或微米晶)为初始材料、通过高压相变制备的细晶粒多晶陶瓷块体以超强共价键的超硬材料为主,本研究探索以微米晶单斜相ZrO2为初始材料,利用高压相变法制备细晶粒四方相多晶ZrO2块体材料,试图将微米晶初始材料结合高压相变制备细晶粒多晶块体的方法引入陶瓷领域。ZrO2具有高强度、高热稳定性、耐磨和生物相容性好等优点,广泛应用于人造牙齿[24]、特种刀具、人工关节、氧传感器等领域。ZrO2在常温常压下为稳定的单斜相(空间群P21/c),在常温至其熔点(2715 ℃)之间会发生两种相变:1170 ℃时发生单斜相向四方相(空间群P42/nmc)的相变,2370 ℃时出现向立方相(Fm
¯3 m)的相变[25],这两种相变都是可逆的。通过加入稳定剂(Y2O3、CeO、CaO等)形成固溶体的方式,可获得常温常压稳定的四方相和立方相[26],其中Y2O3稳定的四方相ZrO2多晶陶瓷材料因存在相变增韧现象而受到广泛关注[24, 27–28]。高压条件下ZrO2单斜相向四方相的转变也得到了证实。Whitney[29–30]通过理论计算发现,在3.6 GPa、室温条件下出现ZrO2单斜相向四方相的转变,并通过实验验证了该结果。Vahldiek等[31]在1.5~2 GPa、1200~1700 ℃条件下合成了单斜相和四方相混合的样品。Kulcinski[32]在超过3.7 GPa、室温条件下直接将单斜相转变成四方相。Alzyab等[33]研究了纯ZrO2以及质量分数为3%、4%和5% Y2O3掺杂的ZrO2的高压相变行为,3%和4% Y2O3掺杂的ZrO2实现了单斜相向四方相的不可逆转变。ZrO2的高压相变研究多在金刚石对顶砧中进行,主要研究不同压力下ZrO2的物相结构,但是通过高压相变方式制备小于初始原料晶粒尺寸的ZrO2多晶陶瓷材料并未引起广泛关注。本研究将晶粒尺寸为2
μ m的单斜相ZrO2与摩尔分数为3%的Y2O3混合,在5.5 GPa、800~1700 ℃温压条件下通过高压相变合成部分相变的亚微米四方相多晶ZrO2块体陶瓷材料,进一步发展以微米晶为初始材料通过高压相变制备细晶粒多晶块体材料的方法。此方法有望解决以纳米粉末为初始材料时因纳米粉末的团聚、吸附、易长大的特性导致烧结所得多晶材料力学性能不佳的问题[34–35]。1. 实 验
1.1 实验组装以及压力和温度标定
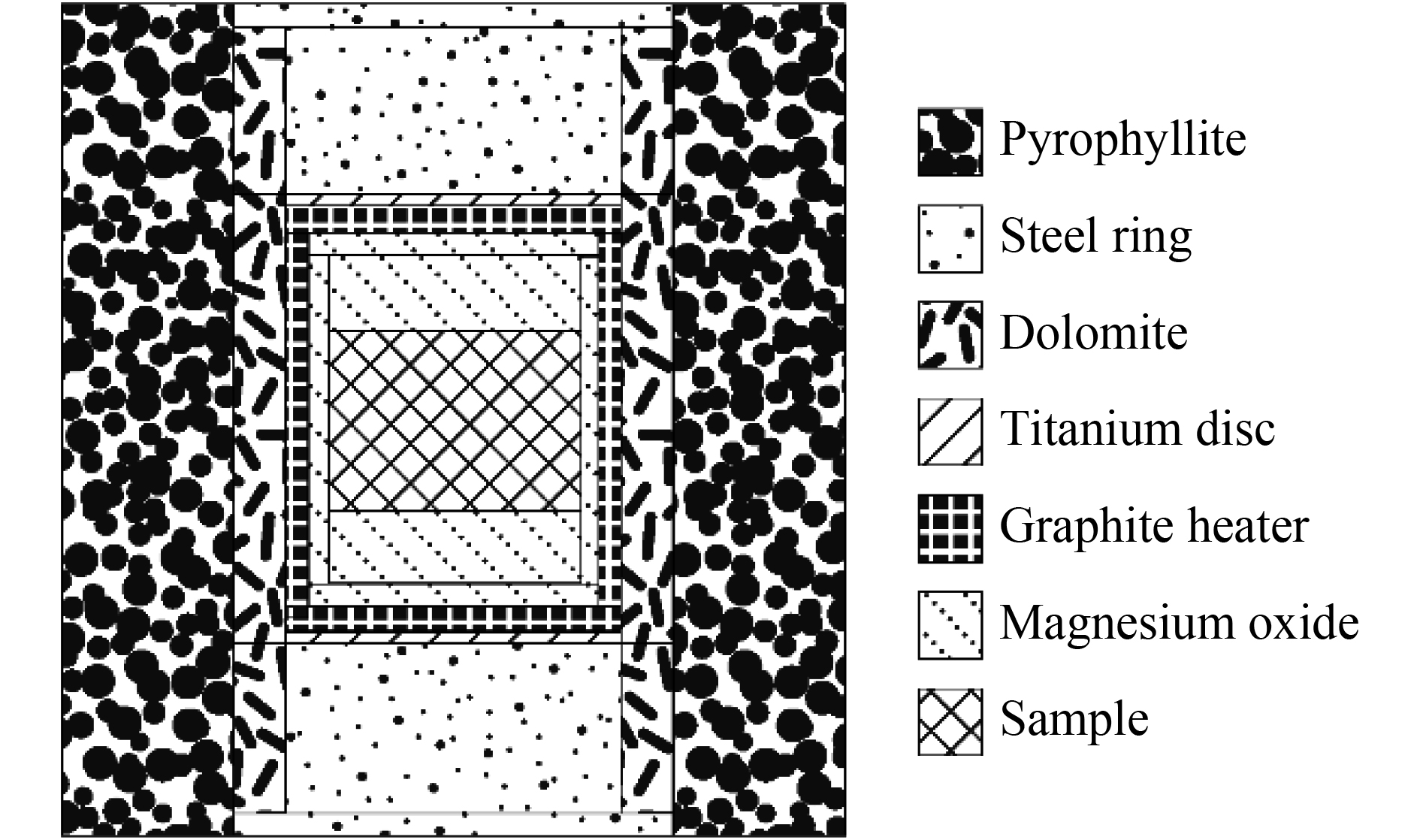
实验使用的设备为国产铰链式6×800 t六面顶压机。加热组装中,以MgO陶瓷管为高压内衬,石墨管为加热管,叶蜡石为传压介质,加压前在300 ℃下烘烤 12 h[36],组装示意图见图1。
利用金属Bi在2.55 GPa、Tl在3.67 GPa、Ba在5.5 GPa下相变导致电阻突变的特点,采用高压原位电阻测量方式,对腔体压力进行标定[36–37],采用B型热电偶(Pt6%Rh-Pt30%Rh)对加热组装进行了温度标定[38–39]。
1.2 实验原料及过程
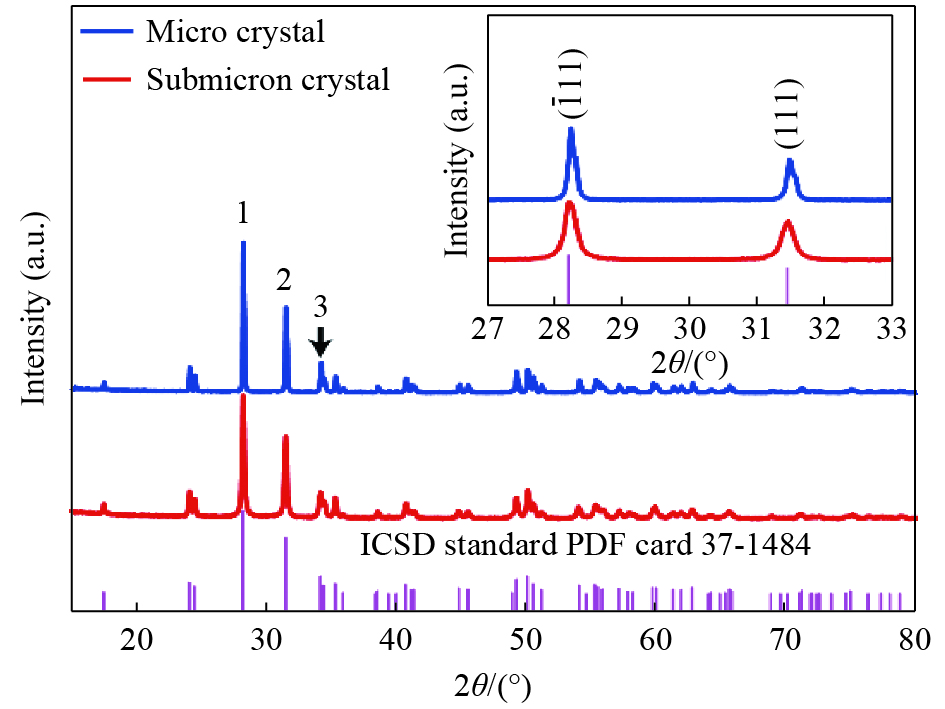
微米晶单斜相ZrO2由亚微米单斜相ZrO2在马弗炉中于1500 ℃下保温12 h制得。图2为亚微米晶原料和微米晶原料的X射线衍射(XRD)图谱。经分析,与单斜相ZrO2的ICSD(无机晶体结构数据库)标准PDF卡37-1484的峰位和强度吻合。1号、2号和3号峰是ZrO2单斜相的3个最强峰,其中:1号峰(2
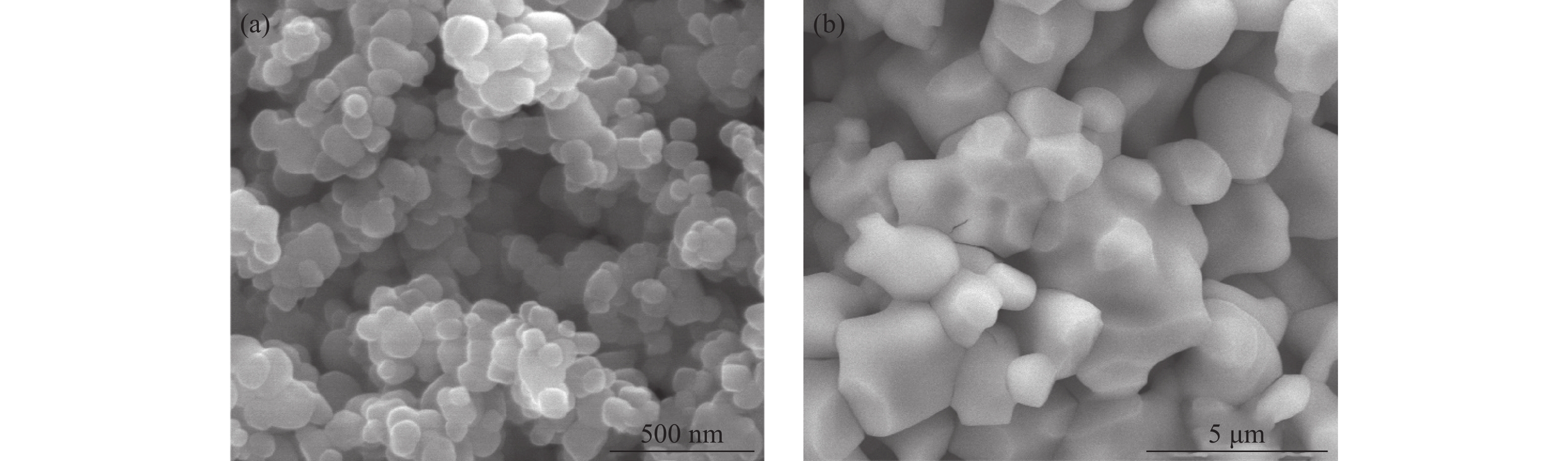
θ =28.2°)和2号峰(2θ =31.5°)为特征峰,分别对应ZrO2单斜相(¯1 11)和(111)晶面[40];3号峰位于34.2°,对应于单斜相(002)晶面。从图2插图可以看出,微米晶原料由于晶粒尺寸较大,导致衍射峰较窄。图3(a)和图3(b)分别为亚微米晶和微米晶单斜相ZrO2原料的扫描电镜(SEM)照片。从图3(b)中可以看出,晶粒尺寸均在微米级,平均晶粒尺寸为2μ m,表明高温热处理亚微米原料获得了微米晶原料。微米晶单斜相ZrO2与3%(摩尔分数)Y2O3原料(购于上海麦克林生化科技有限公司,平均晶粒尺寸为50 nm,纯度为99.99%)加入一定量的酒精混合,超声处理3 h后在真空干燥箱中充分干燥,将混合原料在10 MPa预压压力下压制成直径为11 mm的圆柱后放入MgO陶瓷管。样品在5.5 GPa、800~1700 ℃温压条件下合成,其中:在800~1400 ℃温度范围内,保温时间设置为1 h;1600和1700 ℃的保温时间分别设置为30和20 min。
1.3 物性分析手段
样品相成分通过对破碎后的粉状ZrO2样品进行XRD表征的方式确定,样品中四方相的晶胞参数通过GSAS软件精修XRD图谱的方式确定,实验仪器为荷兰PANalytical锐影系列X射线衍射仪,阳极靶材为Cu靶,工作电压40 kV,电流为45 mA。样品中四方相的含量通过XRD传统物相定量方法(绝热法定量)估算。样品中的Y元素通过对亚微米晶粒进行能谱(EDS)表征定性确定。样品形貌特征通过德国Zeiss/Auriga FIB扫描电子显微镜(SEM)观察,通过统计SEM照片中100个四方相晶粒尺寸得到样品中四方相的晶粒分布,SEM的工作电压为30 kV,工作电流为50
μ A。采用日本JEM-2010型透射电镜(TEM)观察亚微米晶粒的微观结构,TEM的加速电压为200 kV。2. 结果分析与讨论
2.1 XRD分析
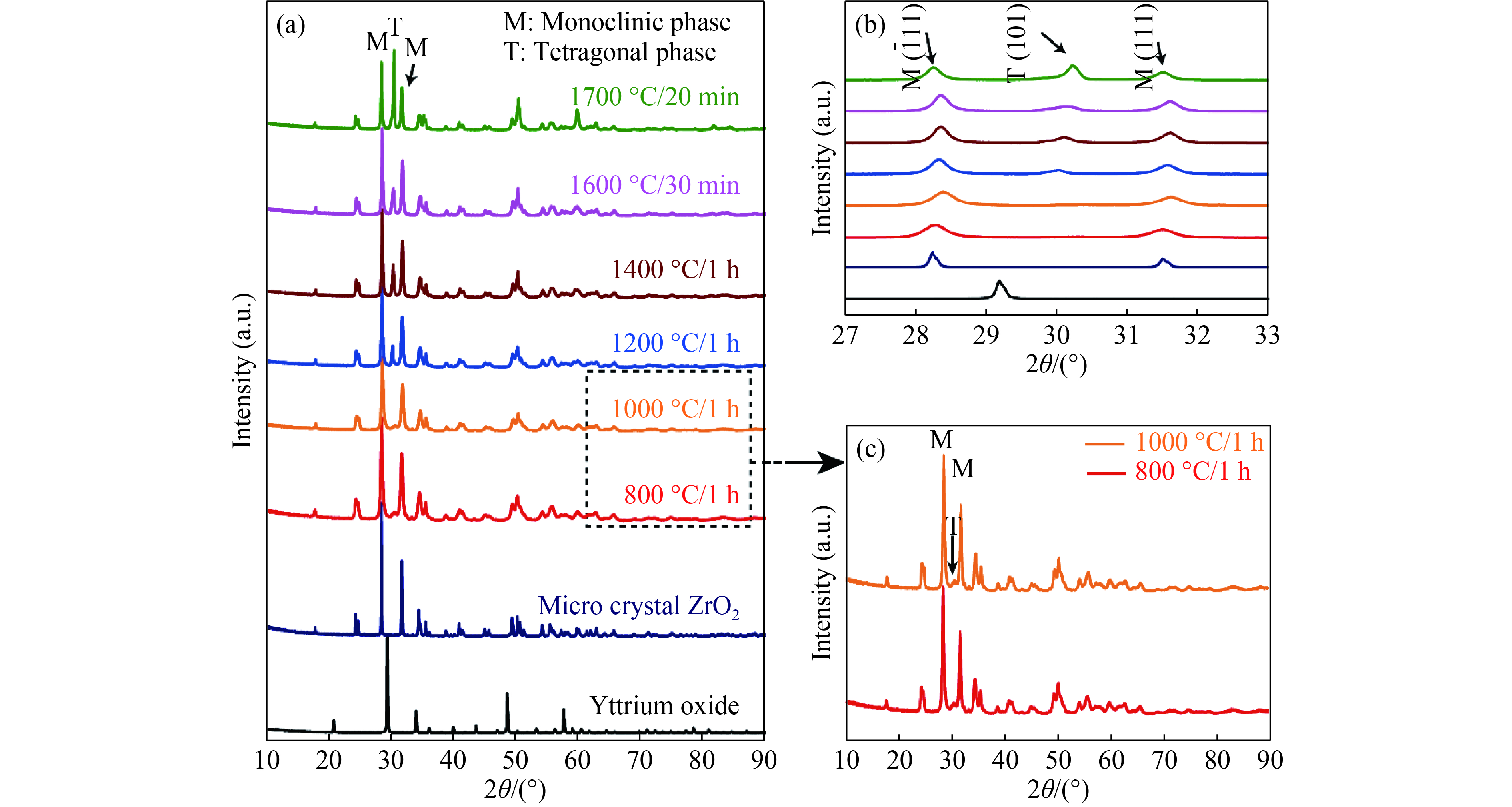
图4(a)和图4(b)为5.5 GPa、不同温度及保温时间条件下合成样品的XRD图谱,其中M代表单斜相的特征峰,T代表四方相的特征峰。可以看出,在800~1700 ℃范围内随着温度的升高,四方相的衍射峰强度逐渐增强,单斜相的衍射峰强度逐渐减弱,表明部分单斜相转化为四方相,采用XRD物相定量分析方法估算5.5 GPa、1700 ℃烧结后四方相ZrO2的质量分数约为50%。如图4(c)所示,在800~1000 ℃范围内可以观察到微弱的四方相特征峰(2
θ =30.3°)[40],对应于四方相的(101)晶面,表明只有少量四方相ZrO2生成。固态相变的驱动力来源于过冷度,过冷温度对于形核、生长以及相变速率均有重要的影响,升高反应温度有利于相变过程的进行,与本实验结果相一致。 图 4 5.5 GPa不同温度条件下样品的XRD图谱(a)、单斜相和四方相的特征峰放大图谱(b)以及(a)中方框标记的800和1000 ℃条件下合成样品的XRD图谱(c)Figure 4. (a) XRD pattern of samples sintered at 5.5 GPa and different temperatures; (b) magnified range of the characteristic diffraction peaks of monoclinic and tetragonal phases from (a); (c) XRD patterns of samples sintered at 800 and 1000 ℃ marked with dotted line in (a)
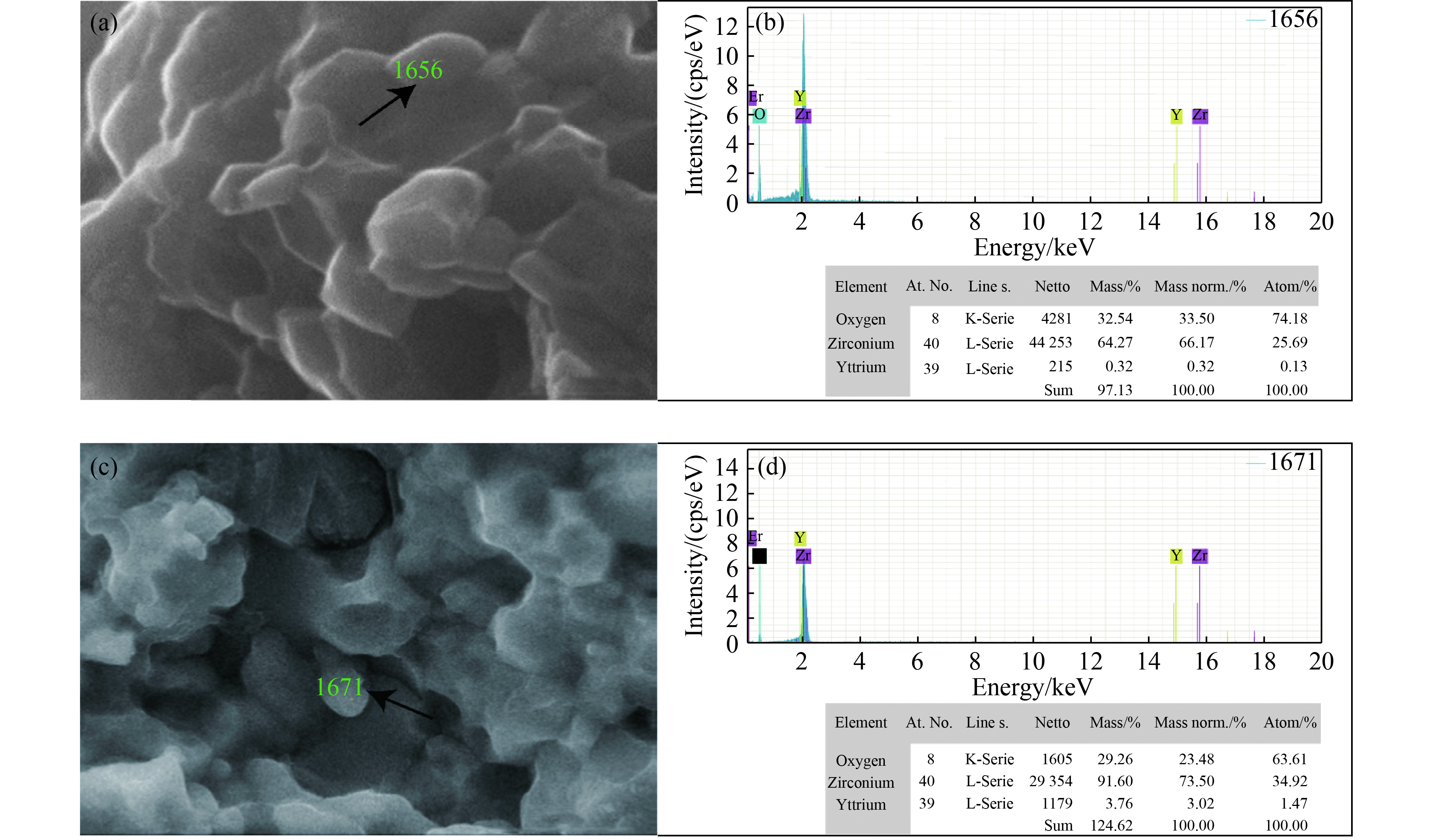
图 4 5.5 GPa不同温度条件下样品的XRD图谱(a)、单斜相和四方相的特征峰放大图谱(b)以及(a)中方框标记的800和1000 ℃条件下合成样品的XRD图谱(c)Figure 4. (a) XRD pattern of samples sintered at 5.5 GPa and different temperatures; (b) magnified range of the characteristic diffraction peaks of monoclinic and tetragonal phases from (a); (c) XRD patterns of samples sintered at 800 and 1000 ℃ marked with dotted line in (a)氧化锆的四方相在常温常压下是不稳定的,通过掺杂离子(如Y3+)可以截获稳定的四方相[26, 41]。如表1所示,1400 ℃下制备的样品中四方相的晶胞参数a和c分别为0.3607(6) nm和0.5219(8) nm,1700 ℃下制备的样品中四方相的晶胞参数a和c分别为0.3615(5) nm和0.5168(3) nm,比未掺杂的ZrO2的晶胞参数[42]稍大,但与掺杂的四方相Zr0.94Y0.06O1.97的晶胞参数[43]相似。Y3+的离子半径大于Zr4+的离子半径,Y3+替代Zr4+位置时,由于离子半径的差异会导致晶格畸变,使晶胞参数变大。由于本研究掺杂的Y2O3量较少,四方相的晶胞参数a和c的变化不明显。图5所示的EDS结果表明,1400和1700 ℃合成样品中的亚微米晶粒均发现了Y元素。EDS结果和精修得出的晶胞参数结果证实了Y3+替代Zr4+形成置换式固溶体。
表 1 室温下稳定四方相氧化锆的晶胞参数Table 1. Unit-cell parameters of tetragonal zirconia stabilized at room temperature 图 5 (a) 1400 ℃温度条件下样品中亚微米晶粒的SEM照片,(b) (a)中箭头所指亚微米晶粒的EDS能谱,(c) 1700 ℃温度条件下样品中亚微米晶粒的SEM照片,(d) (c)中箭头所指亚微米晶粒的EDS能谱Figure 5. (a) SEM images of submicron grains in the sample sintered at 1400 ℃; (b) EDS spectra of the submicron grain directed by the arrow; (c) SEM images of submicron grains in the sample sintered at 1700 ℃; (d) EDS spectra of the submicron grain directed by the arrow
图 5 (a) 1400 ℃温度条件下样品中亚微米晶粒的SEM照片,(b) (a)中箭头所指亚微米晶粒的EDS能谱,(c) 1700 ℃温度条件下样品中亚微米晶粒的SEM照片,(d) (c)中箭头所指亚微米晶粒的EDS能谱Figure 5. (a) SEM images of submicron grains in the sample sintered at 1400 ℃; (b) EDS spectra of the submicron grain directed by the arrow; (c) SEM images of submicron grains in the sample sintered at 1700 ℃; (d) EDS spectra of the submicron grain directed by the arrow2.2 SEM和TEM分析
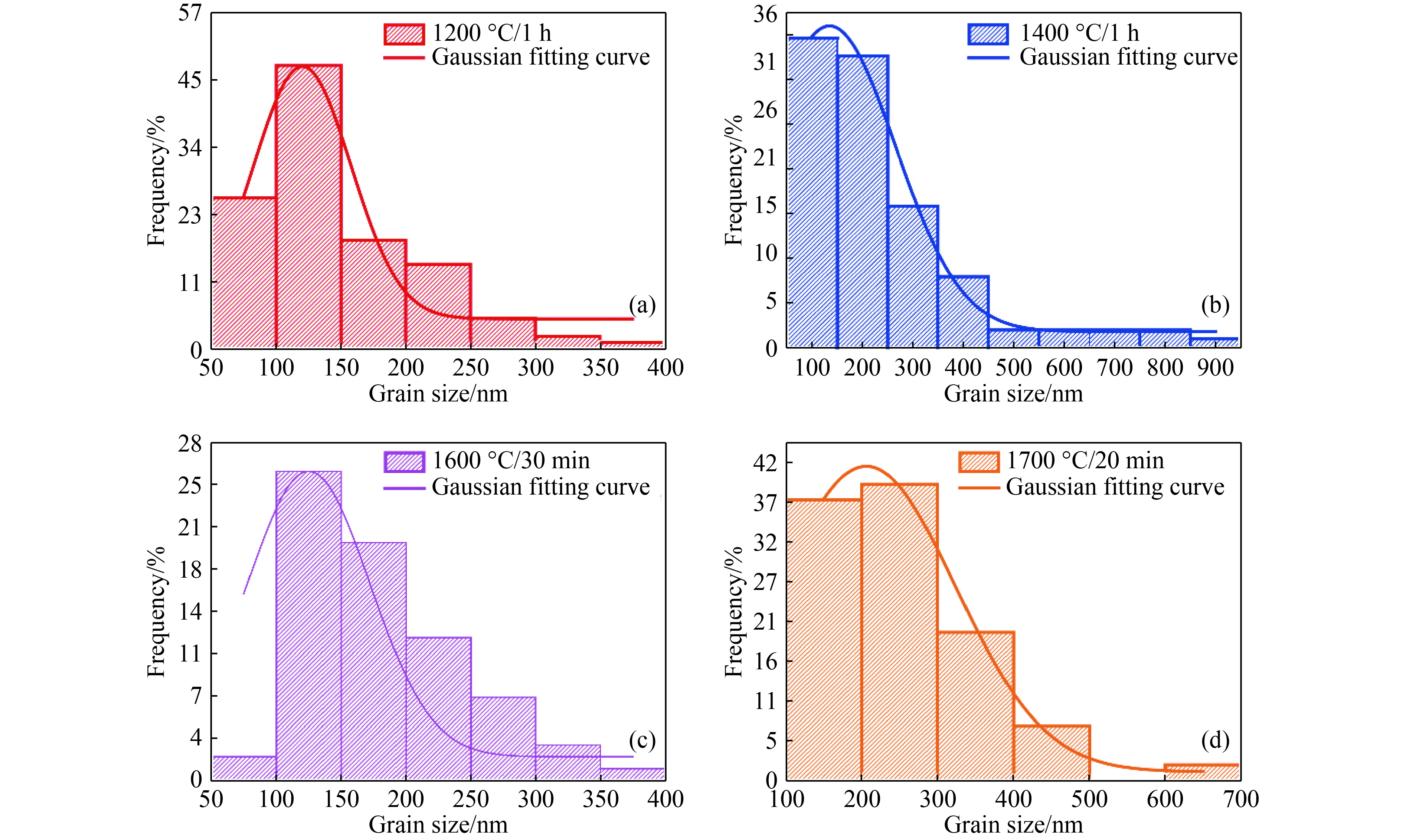
图6为5.5 GPa压力下不同温度合成样品的SEM照片,图7为5.5 GPa、不同温度下合成样品中亚微米晶粒的晶粒尺寸分布图。从图6中可以看到,高压烧结后得到的单斜相与四方相ZrO2烧结体的结构致密,无裂纹。从图6(a)中可以观察到,在1200 ℃保温 1 h条件下合成的样品中有一定量的微米级晶粒,可能是未相变单斜相晶粒长大的结果;从图6(b)也可以观察到在微米晶粒周围存在大量纳米和亚微米晶粒,推测为微米晶单斜相ZrO2相变后再结晶的四方相细晶粒(TEM表征也证明了该推测)。经统计,亚微米晶粒的平均晶粒尺寸为(145±62) nm,远小于初始单斜相微米晶ZrO2的晶粒尺寸,如图7(a)所示。
如图6(h)所示,1700 ℃保温 20 min条件下合成的样品中同样可以观察到亚微米细晶粒,这些细晶粒可能是由母相(单斜相)“孕育”而出的四方相晶粒。从图7(d)可以看出,该样品中亚微米晶粒的平均晶粒尺寸为(245±107) nm,相较于低温(1200 ℃)合成样品,晶粒有所长大。图6显示了1400 ℃保温 1 h和 1600 ℃保温 30 min条件下合成样品的SEM照片。从图6(d)和图6(f)可以看出,样品中均出现亚微米晶粒。如图7(b)和图7(c)所示,1400和1600 ℃条件下合成样品中亚微米晶粒的平均晶粒尺寸分别为(246±165) nm和(183±62) nm。
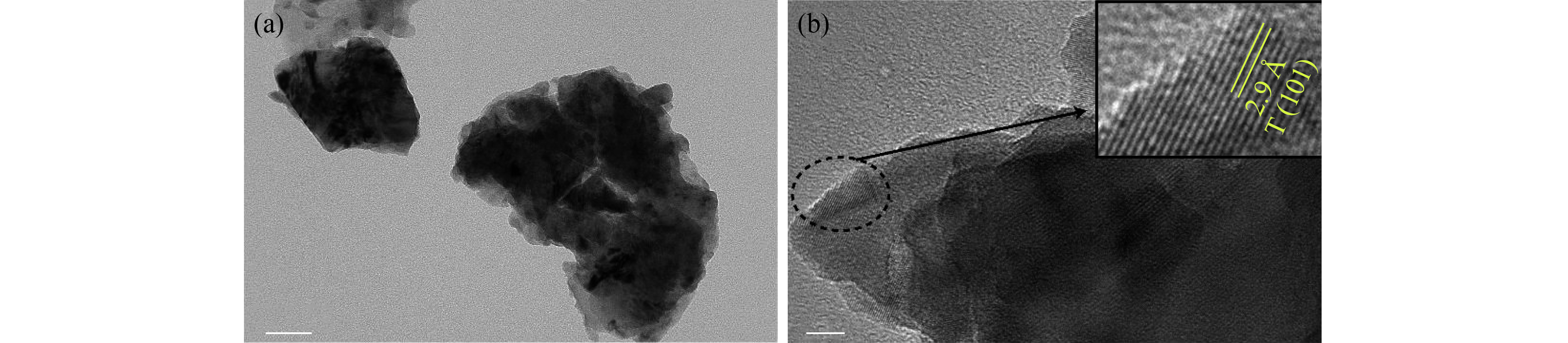
为了证明上述亚微米晶粒为微米晶单斜相ZrO2相变后再结晶的四方相晶粒的猜测,对5.5 GPa、1700 ℃条件下制备的样品中的亚微米晶粒进行TEM表征。图8(a)和图8(b)分别为样品中亚微米晶粒的低倍TEM照片和HRTEM照片。如图8(b)插图所示,亚微米晶粒的晶面间距为2.9 Å,与ICSD标准PDF卡70-4430中四方相(101)晶面间距(2.9624 Å)相符合,与Y2O3原料的(222)晶面间距(3.0610 Å)、(400)晶面间距(2.6509 Å)、(440)晶面间距(1.8745 Å)、(622)晶面间距(1.5986 Å)不符合,证实了亚微米晶粒中四方相结构的存在,支持了上述关于单斜相微米晶ZrO2相变后再结晶形成的亚微米颗粒为四方相ZrO2晶粒的猜测。
 图 8 5.5 GPa、1700 ℃合成样品的TEM照片((a)样品中亚微米晶粒的低倍TEM照片;(b)样品中亚微米晶粒的HRTEM照片,插图为虚线椭圆部位的放大图)Figure 8. TEM images of the sample sintered at 5.5 GPa and 1700 ℃ ((a) Low-magnification TEM image of submicron grains in the sample; (b) HRTEM image of submicron grains in the sample. Inset: Magnified HRTEM image marked with the dotted ellipse in (b))
图 8 5.5 GPa、1700 ℃合成样品的TEM照片((a)样品中亚微米晶粒的低倍TEM照片;(b)样品中亚微米晶粒的HRTEM照片,插图为虚线椭圆部位的放大图)Figure 8. TEM images of the sample sintered at 5.5 GPa and 1700 ℃ ((a) Low-magnification TEM image of submicron grains in the sample; (b) HRTEM image of submicron grains in the sample. Inset: Magnified HRTEM image marked with the dotted ellipse in (b))新相的形成要经历形核、生长的过程。高压的引入可以提高成核率,同时抑制晶粒的生长速率,新相的晶粒尺寸与初始材料的晶粒尺寸无必然联系。本研究中XRD、SEM和TEM表征结果表明,以单斜相微米晶ZrO2为初始材料通过高压相变合成的样品中含有再结晶的纳米和亚微米四方相ZrO2晶粒。高压下以微米晶为初始材料相变合成晶粒尺寸更细的多晶块体陶瓷材料,可以避免以纳米粉末为初始材料在常压和低压(低于1 GPa)烧结过程中纳米晶粒长大的问题,同时克服了以纳米初始粉末为初始材料时存在的表面吸附、易团聚的难题。
3. 结论与展望
以微米晶单斜相ZrO2为初始材料,在5.5 GPa、800~1700 ℃温压条件下通过高压相变法实现了单斜相向四方相的部分转变,获得了小于初始材料晶粒尺寸的亚微米四方相ZrO2晶体的块体陶瓷。
微米晶原料由于表面能低以及表面成核位点较少,在本实验的温压条件下未能实现纯相的亚微米四方相ZrO2多晶块体陶瓷的制备。需进一步探索合适的压强和温度匹配关系,从而实现高致密度、优异力学性能、纯相、亚微米四方相ZrO2多晶块体陶瓷的制备,期望将在超硬材料领域实现应用的以大颗粒粉晶(大单晶或微米晶)为初始材料通过高压相变制备高性能细晶粒多晶块体的方法推广到陶瓷领域。
-
表 1 等效梁单元的材料属性
Table 1. Material properties of the equivalent beam element
d/nm L/nm kr/(μN·rad-1) kθ/(aN·m·rad-2) kτ/(aN·m·rad-2) E/GPa G/GPa 0.146 0.142 0.652 0.875 0.278 5 780 878 表 2 石墨烯的材料属性参数
Table 2. Material property parameters of graphene
Ex/GPa Ey/GPa Ez/GPa ν12 ν13 ν23 Gxy/GPa Gxz/GPa Gyz/GPa 730 709 58.5 0.46 0.2 0.2 434 7.5 7.5 -
[1] NOVOSELOV K S, GEIM A K, MOROZOV S V, et al.Electric field effect in atomically thin carbon films[J].Science, 2004, 306(5696):666-669. doi: 10.1126/science.1102896 [2] BALANDIN A A, GHOSH S, BAO W Z, et al.Superior thermal conductivity of single-layer graphene[J].Nano Letters, 2008, 8(3):902-907. doi: 10.1021/nl0731872 [3] 刘晓毅, 王奉超, 吴恒安.石墨烯及其复合材料纳米力学研究进展[J].固体力学学报, 2016, 37(5):398-420. http://www.cnki.com.cn/Article/CJFDTOTAL-GTLX201605002.htmLIU X Y, WANG F C, WU H A.Progress in nanomechanical research of graphene and its composites[J].Acta Mechanica Solida Sinica, 2016, 37(5):398-420. http://www.cnki.com.cn/Article/CJFDTOTAL-GTLX201605002.htm [4] LEE C G, WEI X D, KYSAR J W, et al.Measurement of the elastic properties and intrinsic strength of monolayer grapheme[J].Science, 2008, 321(5887):385-388. doi: 10.1126/science.1157996 [5] RESKETI N A, AMIRI H A, DEHESTANI M.Effects of size and shape on elastic constants of graphene sheet[J].Structures, 2018, 13:131-138. doi: 10.1016/j.istruc.2017.12.005 [6] VUL A Y, SOKOLOV V I.Nanocarbon studies in Russia:from fullerene to nanotubes and nanodiamonds[J].Nanotechnologies in Russia, 2009, 4(7/8):397-414. doi: 10.1134/S1995078009070027 [7] RAO C N R, BISWAS K, SUBRAHMANYAM K S, et al.Graphene, the new nanocarbon[J].Journal of Materials Chemistry, 2009, 19(17):2457-2469. doi: 10.1039/b815239j [8] SPANOS K N, GEORGANTZINOS S K, ANIFANTIS N K.Mechanical properties of graphene nanocomposites:a multiscale finite element prediction[J].Composite Structures, 2015, 132:536-544. doi: 10.1016/j.compstruct.2015.05.078 [9] SPANOS K N, ANIFANTIS N K.Finite element prediction of stress transfer in graphene nanocomposites:the interface effect[J].Composite Structures, 2016, 154:269-276. doi: 10.1016/j.compstruct.2016.07.058 [10] GIANNOPOULOS G I, KALLIVOKAS I G.Mechanical properties of graphene based nanocomposites incorporating a hybrid interphase[J].Finite Element in Analysis and Design, 2014, 90:31-40. doi: 10.1016/j.finel.2014.06.008 [11] PAPAGEORGIOU D G, KINIOCH I A, YOUNG R J.Mechanical properties of graphene and graphene-based nanocomposites[J].Progress in Materials Science, 2017, 90:75-127. doi: 10.1016/j.pmatsci.2017.07.004 [12] 孙帅.石墨烯缺陷的特点与应用研究[D].天津: 天津大学, 2015: 10-30.SUN S.Study on the characteristics and application of graphene defects[D].Tianjin: Tianjin University, 2015: 10-30. [13] 韩同伟, 贺鹏飞, 王健, 等.单层石墨烯薄膜拉伸变形的分子动力学模拟[J].新型炭材料, 2010, 25(4):261-266. http://d.old.wanfangdata.com.cn/Periodical/xxtcl201004004HAN T W, HE P F, WANG J, et al.Molecular dynamics simulation of tensile deformation of monolayer graphene films[J].New Carbon Materials, 2010, 25(4):261-266. http://d.old.wanfangdata.com.cn/Periodical/xxtcl201004004 [14] 韩同伟, 施元君, 贺鹏飞, 等.Stone-Wales拓扑缺陷对石墨烯拉伸力学性能的影响[J].固体力学学报, 2011, 32(6):619-624. http://d.old.wanfangdata.com.cn/Periodical/gtlxxb201106011HAN T W, SHI Y J, HE P F, et al.Effect of Stone-Wales topological defects on tensile mechanical properties of graphene[J].Acta Mechanica Solida Sinica, 2011, 32(6):619-624. http://d.old.wanfangdata.com.cn/Periodical/gtlxxb201106011 [15] 韩同伟, 贺鹏飞, 王健, 等.空位缺陷对单层石墨烯薄膜拉伸力学性能的影响[J].同济大学学报, 2010, 38(8):1210-1214. doi: 10.3969/j.issn.0253-374x.2010.08.020HAN T W, HE P F, WANG J, et al.Effect of vacancy defects on tensile mechanical properties of single layer graphene films[J].Journal of Tongji University, 2010, 38(8):1210-1214. doi: 10.3969/j.issn.0253-374x.2010.08.020 [16] LI C Y, CHOU T W.A structural mechanics approach for the analysis of carbon nanotubes[J].International Journal of Solids and Structures, 2003, 40(10):2487-2499. doi: 10.1016/S0020-7683(03)00056-8 [17] LI C Y, CHOU T W.Elastic moduli of multi-walled carbon nanotubes and the effect of van der Waals forces[J].Composites Science and Technology, 2003, 63(11):1517-1524. doi: 10.1016/S0266-3538(03)00072-1 -







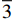
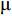

 下载:
下载:
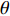
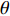

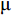


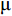
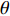



 下载:
下载: