含缺陷的石墨烯对增强树脂基复合材料力学性能的影响

宋鲁彬; 郭章新; 李忠贵; 栾云博; 赵聃; 张祺

doi:10.11858/gywlxb.20180586

含缺陷的石墨烯对增强树脂基复合材料力学性能的影响

doi: 10.11858/gywlxb.20180586

宋鲁彬^{1, 2,},
郭章新^{1, 2,*, ,},
李忠贵^{1, 2},
栾云博¹,
赵聃¹,
张祺¹

1.
太原理工大学力学学院材料强度与结构冲击山西省重点实验室, 山西太原 030024
2.
西安交通大学机械结构强度与振动国家重点实验室, 陕西西安 710049

基金项目:

国家自然科学基金 11602160

国家自然科学基金 21501129

国家自然科学基金 11502155

山西省高等学校创新项目 2017117

西安交通大学机械结构强度与振动国家重点实验室开放课题 SV2017-KF-01

山西省自然科学青年基金 201601D021026

山西省"1331工程"重点创新团队项目

详细信息

作者简介:
宋鲁彬(1993-), 男, 硕士研究生, 主要从事复合材料及其结构的力学性能研究. E-mail:1978193041@qq.com

通讯作者:
郭章新(1983-), 男, 博士, 讲师, 主要从事复合材料及其结构的力学性能研究. E-mail:woxintanran215@163.com

中图分类号: O341
计量
- 文章访问数: 7170
- HTML全文浏览量: 2948
- PDF下载量: 21
出版历程
- 收稿日期: 2018-06-20
- 修回日期: 2018-07-06

Effect of Defective Graphene on Mechanical Properties of Reinforced Resin Matrix Composites

SONG Lubin^{1, 2
,},
GUO Zhangxin^{1, 2,*
, ,},
LI Zhonggui^{1, 2},
LUAN Yunbo¹,
ZHAO Dan¹,
ZHANG Qi¹

1.
College of Mechanics, Shanxi Key Laboratory of Material Strength & Structure Impact, Taiyuan University of Technology, Taiyuan 030024, China
2.
State Key Laboratory for Strength and Vibration of Mechanical Structures, Xi'an Jiaotong University, Xi'an 710049, China

摘要

摘要: 基于分子结构力学和多尺度方法，采用有限元商业软件ABAQUS，针对石墨烯存在的几种缺陷，构建了含有不同数量的双原子空缺缺陷和Stone-Wales（S-W）缺陷的石墨烯增强环氧树脂复合材料的有限元模型，研究了石墨烯的缺陷在不同石墨烯体积分数下对复合材料弹性模量以及界面层应力传递的影响。数值模拟结果表明，随着石墨烯体积分数的增加，完美石墨烯和缺陷石墨烯增强环氧树脂复合材料弹性模量均呈现线性上升。其次，缺陷种类和数量之间的结果对比表明，空缺缺陷会明显降低复合材料的弹性模量，且随着缺陷数量的增加，变化更明显。而S-W缺陷在一定程度上增加了复合材料的杨氏模量，对于剪切模量，则出现下降趋势。另外，界面层的应力传递一定程度上反映了缺陷对复合材料的影响。
- 石墨烯 /
- 缺陷 /
- 复合材料 /
- 力学性能 /
- 多尺度方法
Abstract: Based on the molecular structural mechanics and multi-scale method, the finite element model of graphene reinforced epoxy resin composite with different number of vacancy defects and Stone-Wales (S-W) defects was constructed by using the finite element commercial software ABAQUS.The effects of graphene defects on the elastic modulus and interfacial stress transfer of composites with different volume fractions of graphene were investigated.The numerical simulation results show that the elastic modulus of the perfect graphene and the defective graphene reinforced epoxy resin composite increases linearly with the increase of the volume fraction of graphene.Secondly, the comparison between the number of defects and the type of defects shows that the vacancy defect can obviously reduce the modulus of elasticity of the composite, and the change is more obvious with the increase of the number of defects.However, the S-W defect increases the Young's modulus of the composites to a certain extent, but the shear modulus decreases.In addition, the stress transfer from the interface layer to some extent reflects the effect of defects on the composites.
- graphene /
- defect /
- composites /
- mechanical properties /
- multi-scale method

HTML全文

碳纳米材料常作为聚合物纳米复合材料的增强相。由于纳米材料可以在不削弱基体材料加工性、初始机械性能和轻质性的前提下，增强材料的机械性能以及电学、热学、光学和其他的功能型性能^[1-3]，可以在航空航天和汽车行业作为优秀的结构材料，并在电子器械中作为传感器和其他电子器件，在新能源等其他领域也具有广阔的应用前景。石墨烯(Graphene)是一种二维晶体，是由C—C共价键结合组成六角形呈蜂窝状规则排列的碳纳米材料，具有优异的力学特性，在材料、微纳米加工、药物传递等领域是一种革命性的材料。各项实验测试证明，石墨烯的杨氏模量大约为1 TPa，拉伸强度约为130 GPa^[4]。与富勒烯的零维和碳纳米管的一维结构相比，石墨烯的二维平面结构使其比表面积更大，在复合材料中可以与基体有更多的接触面，石墨烯的这些性质使其可以作为与碳纳米管相似甚至更好的增强材料^[5-7]。

国内外已经有许多研究者针对完美石墨烯增强树脂基复合材料的力学性能进行了有限元模拟^[8-11]。然而，实验观察发现，与石墨和碳纳米管相同，缺陷同样普遍地存在于石墨烯中。从严格意义上讲，因为人工制备时各种设备及制备方法的限制，石墨烯并非绝对平整的由六元环组成的大分子，结构中往往存在各种各样的缺陷，比如由一个C—C键旋转90°形成的Stone-Wales缺陷、由六元环中C原子丢失而产生的单空穴缺陷和多重孔穴缺陷等^[12-15]。研究表明，这些缺陷会影响石墨烯的物理化学性质和结构变形机制，降低石墨烯的力学性能，比如杨氏模量和泊松比。纵向和横向刚度以及拉伸、扭转和弯曲刚度也会产生一定的改变。如果将石墨烯作为复合材料的增强相，缺陷就可能会通过与基体的复合作用对石墨烯增强复合材料的力学性能产生一定影响。因此，有必要研究石墨烯的缺陷对聚合物复合材料力学性能的影响。

结合Li等^[16-17]提出的分子结构力学的相关研究，基于Giannopoulos等^[10]提出的多尺度代表性体积单元，通过ABAQUS有限元软件对含有缺陷的石墨烯增强复合材料的代表性体积单元进行建模，研究了不同数量空缺缺陷和Stone-Wales(S-W)缺陷对石墨烯增强复合材料的弹性模量和界面应力传递的影响。

1. 数值模拟

1.1 等效梁单元参数

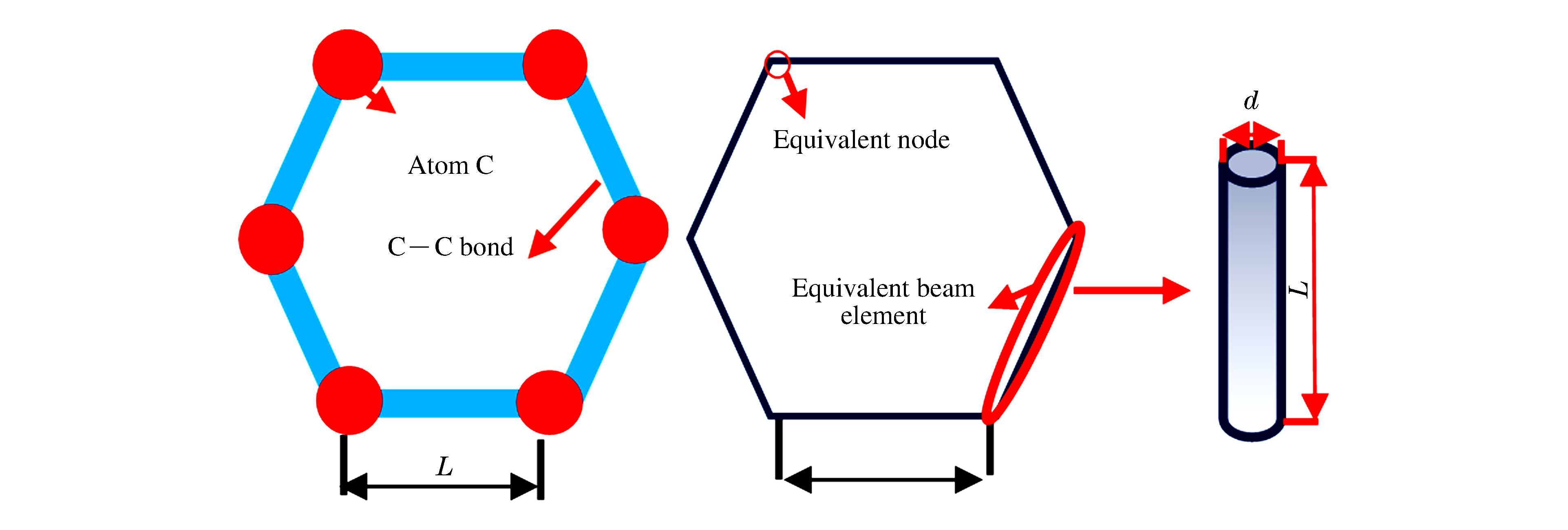
Li等^[16-17]提出一种分子结构力学方法，其基本思想是把石墨烯结构当做一种网状结构，石墨烯基本组成单元C—C共价键和C原子分别等效为有限元中的梁单元和单元节点，如图 1所示，其中d代表梁单元直径，L代表梁单元长度。基于C—C共价键和梁单元在拉伸、弯曲以及扭转条件下的相似性，利用分子力学和结构力学的关系，来确定等效梁的截面属性和各项材料参数。

图 1 石墨烯六边形晶格与有限元模型的等效替代

Figure 1. Equivalent substitution of graphene hexagon lattice with finite element model

下载: 全尺寸图片幻灯片

在原子级别，原子核可以视为质点，势能主要取决于原子核的相对位置和对应的力场。忽略静电力的作用，小应变下，石墨烯的总势能取决于键的化学能、键结和非键结作用的势能，表达式为

${U_{{\text{tot}}}} = \sum {{U_r}} + \sum {{U_\theta }} + \sum {{U_\varphi }} + \sum {{U_{\text{w}}}} + \sum {{U_{{\text{vdw}}}}}$

(1)

式中:U_r为共价键的拉伸势能，U_θ为共价键的键角弯曲势能，U_φ为二面角的扭曲势能，U_w为平面扭转势能，U_vdw为非键结作用产生的范德华势能。

对于共价键，总势能主要来源于前4项的作用。为了描述碳原子在小变形下的共价相互作用，利用简谐形式将二面角的扭转势能和平面外扭转势能结合，由共价键连接的碳原子间相互作用引起的振动势能可以表达为

${U_r} = \frac{1}{2}{k_r}{\left( {r-{r_0}} \right)^2} = \frac{1}{2}{k_r}{\left( {\Delta r} \right)^2}$

(2)

${U_\theta } = \frac{1}{2}{k_\theta }{\left( {\theta-{\theta _0}} \right)^2} = \frac{1}{2}{k_\theta }{\left( {\Delta \theta } \right)^2}$

(3)

${U_\tau } = \frac{1}{2}{k_\tau }{\left( {\tau-{\tau _0}} \right)^2} = \frac{1}{2}{k_\tau }{\left( {\Delta \tau } \right)^2}$

(4)

式中:k_r、k_θ和k_τ分别表示与共价键的拉伸、弯曲和扭转相关的力常数，Δr、Δθ和Δτ分别代表相对平衡位置的键长、键角和二面角的变化量。

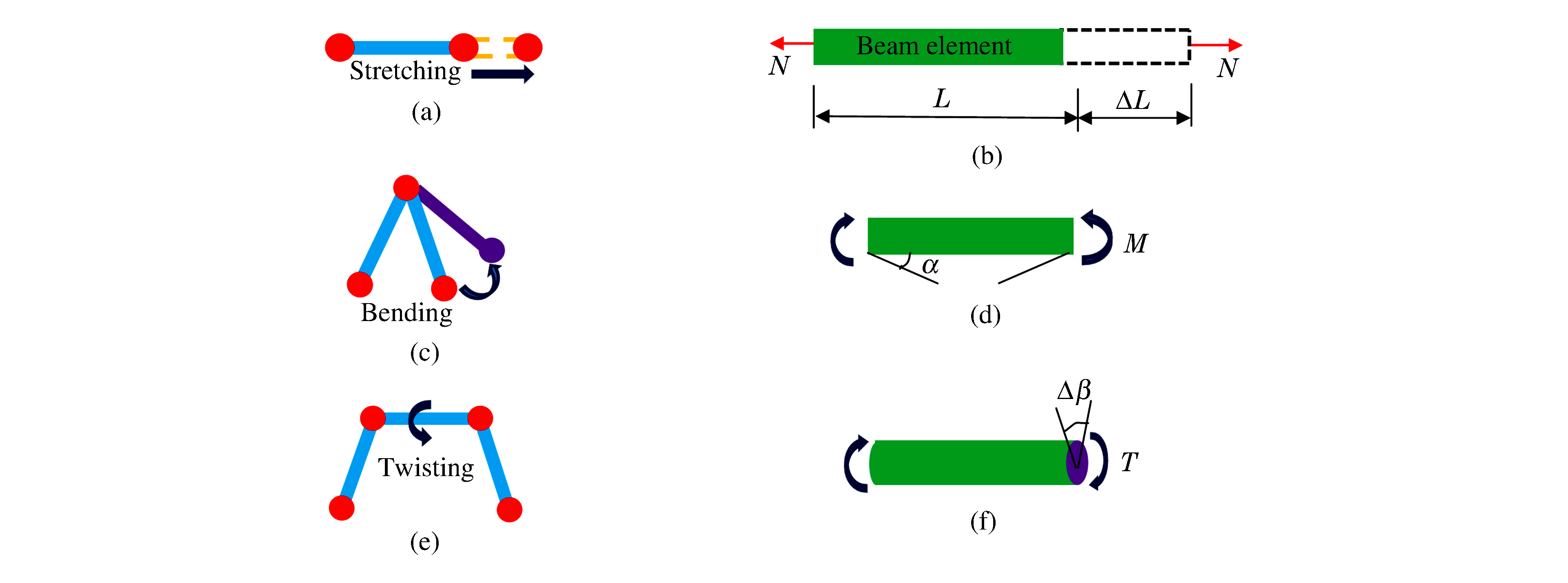
根据结构力学可知，梁的总势能由拉伸、弯曲、旋转组成。图 2为等截面梁产生不同势能的不同加载情况。

图 2 石墨烯C-C键与梁单元之间的变形等效

Figure 2. Deformation equivalence between the C-C bond of graphene and the beam element

下载: 全尺寸图片幻灯片

假设代表碳-碳共价键的梁单元弹性模量为E，长度为L，横截面面积为A，转动惯量为I，则梁单元只受轴向拉伸载荷N时，应变能为

${U_N} = \frac{1}{2}\int_0^L {\frac{{{N^2}}}{{EA}}{\text{d}}L} = \frac{1}{2}\frac{{{N^2}L}}{{EA}} = \frac{1}{2}\frac{{EA}}{L}{\left( {\Delta L} \right)^2}$

(5)

式中:ΔL为梁的伸长量。在纯弯矩M的作用下，梁单元的应变能可以表达为

${U_M} = \frac{1}{2}\int_0^L {\frac{{{M^2}}}{{EI}}{\text{d}}L} = \frac{1}{2}\frac{{EI}}{L}{\left( {2\alpha } \right)^2}$

(6)

式中:α为梁的弯曲变形。同理，在纯扭矩T的作用下，梁单元的应变能为

${U_T} = \frac{1}{2}\int_0^L {\frac{{{T^2}}}{{GJ}}{\text{d}}L} = \frac{1}{2}\frac{{{T^2}L}}{{GJ}} = \frac{1}{2}\frac{{GJ}}{L}{\left( {\Delta \beta } \right)^2}$

(7)

式中:J为极惯性矩，G为剪切模量，Δβ为扭转角。

(2) 式~(4)式和(5)式~(7)式分别表示了分子力学和结构力学两个不同体系中的势能表达式，由于两个体系间的等效性，可以直接将结构力学理论中的参数EA、EI、GJ分别与分子力学理论中的参数k_r、k_θ、k_τ相对应, 并假设梁单元的横截面为圆形，并令A=πd²/4，I=πd⁴/32，J=πd⁴/64，那么根据力场常数，可以导出梁单元的各项参数计算公式。其中，参数d、E、G由(8)式、(9)式、(10)式得出，参数L、k_r、k_θ、k_τ来自文献[10]，具体参数见表 1。

$d = 4\sqrt {\frac{{{k_\theta }}}{{{k_r}}}}$

(8)

$E = \frac{{k_r^2L}}{{4\pi {k_\theta }}}$

(9)

$G = \frac{{k_r^2{k_\tau }L}}{{8\pi k_\theta ^2}}$

(10)

表 1 等效梁单元的材料属性

Table 1. Material properties of the equivalent beam element

d/nm	L/nm	k_r/(μN·rad^-1)	k_θ/(aN·m·rad^-2)	k_τ/(aN·m·rad^-2)	E/GPa	G/GPa
0.146	0.142	0.652	0.875	0.278	5 780	878

下载: 导出CSV

| 显示表格

1.2 有限元模型

采用ABAQUS软件，构建了含有不同数量的双原子空缺缺陷和S-W缺陷石墨烯的有限元模型。本研究中缺陷的数量分别是1、5和9个，因此对于含有空缺缺陷和S-W缺陷的石墨烯，文中用K1、K5、K9和S-W1、S-W5、S-W9来简化表示。图 3为K9和S-W9缺陷石墨烯的有限元模型。

图 3 含有9个双原子空缺缺陷(a)和9个S-W缺陷(b)的有限元模型

Figure 3. Finite element model containing nine diatomic vacancy defects and nine S-W defects

下载: 全尺寸图片幻灯片

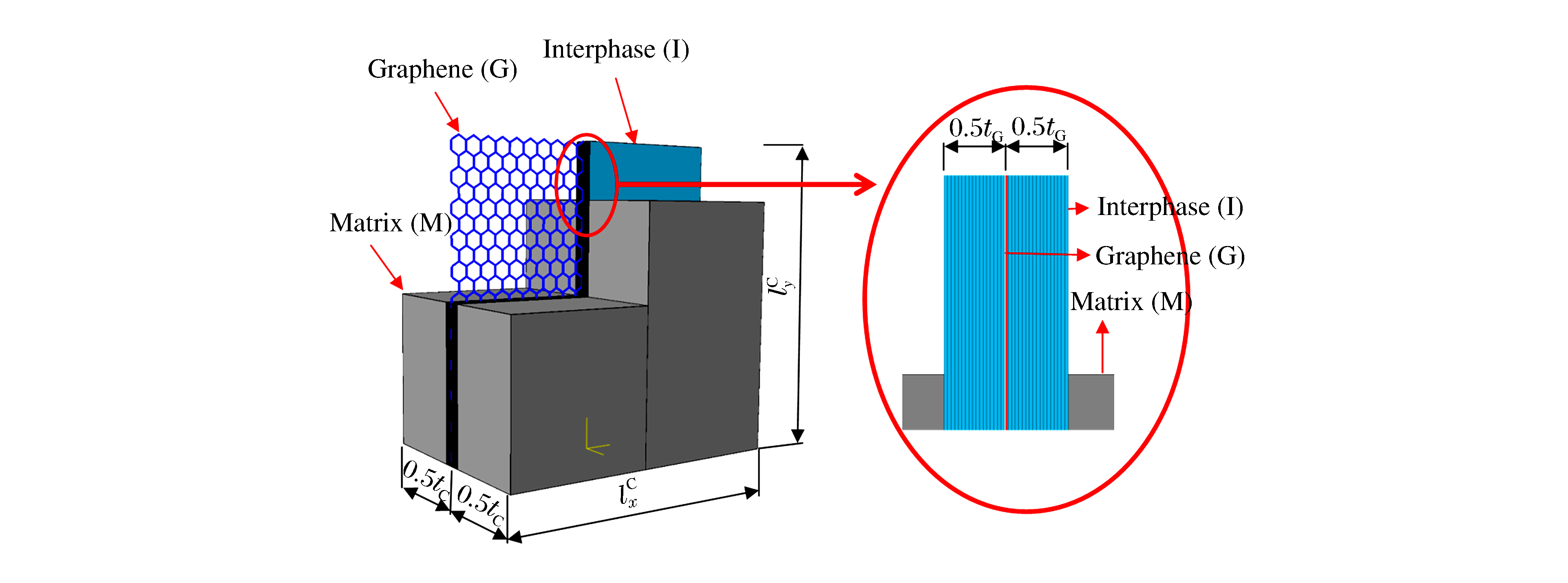
石墨烯与基体材料之间的界面区域非常复杂，由于原子间的相互作用，使各种化学键和范德华力等共存于此。本次数值模拟旨在探究石墨烯的缺陷对复合材料弹性模量的影响，于是简化其间复杂的力学状态，转而采用变参数的板层来近似模拟界面的力学行为。对于界面层，仍使用三维实体单元进行建模，并划分为34层。同时，赋予该界面层各向异性的材料属性，可以更形象地模拟石墨烯与基体之间的应力传递过程。界面层各层材料属性为

$P_{ij}^{\text{I}}\left( z \right) = Az{{\text{e}}^{-Cz}} + B$

(11)

$P_{ij}^{\text{I}}\left( { + 0.5{t_{\text{G}}}} \right) = P_{ij}^{\text{I}}\left( {-0.5{t_{\text{G}}}} \right) = P_{ij}^{\text{M}}$

(12)

$P_{ij}^{\text{I}}\left( {{0^ + }} \right) = P_{ij}^{\text{I}}\left( {{0^-}} \right) = kP_{ij}^{\text{G}}$

(13)

$\frac{{{\text{d}}P_{ij}^{\text{I}}\left( z \right)}}{{{\text{d}}z}}\left| {_{z = + 0.5{t_{\text{G}}}}} \right. = \frac{{{\text{d}}P_{ij}^{\text{I}}\left( z \right)}}{{{\text{d}}z}}\left| {_{z =-0.5{t_{\text{G}}}}} \right. = 0$

(14)

式中：P表示力学参数，可以代表杨氏模量或者剪切模量，即P=E, G, ν；P_ij^I表示界面层模量和泊松比(ν), P_ij^G表示石墨烯的模量和泊松比(ν)；A、B、C为常数，由(12)式、(13)式、(14)式计算得出；k表示刚度系数，这里取k=1。石墨烯的材料属性参数见表 2。

表 2 石墨烯的材料属性参数

Table 2. Material property parameters of graphene

E_x/GPa	E_y/GPa	E_z/GPa	ν₁₂	ν₁₃	ν₂₃	G_xy/GPa	G_xz/GPa	G_yz/GPa
730	709	58.5	0.46	0.2	0.2	434	7.5	7.5

下载: 导出CSV

| 显示表格

环氧树脂利用三节点八面体单元构建，由文献[10]赋予其各向同性的材料属性：E_m=3 GPa，ν_m=0.34。图 4为整体的复合材料的有限元模型示意图。通过改变t_C，可以得到不同体积分数石墨烯的复合材料模型。另外，单层石墨烯的等效厚度为t_G=0.34 nm。

图 4 石墨烯增强环氧树脂基复合材料的代表性体积单元

Figure 4. Representative volume element of graphene reinforced epoxy matrix composites

下载: 全尺寸图片幻灯片

最后，在ABAQUS中施加适当的边界条件。例如，求解x方向弹性模量的边界条件为：在X=0的面上，约束所有节点的自由度，在y=0、y=W以及z=0、z=H施加对称的约束。最后在X=L的面上所有节点，施加非零位移ΔL。获取X=L面上相应的所有节点的反力，即ⁿf_i，便可以求得复合材料的相关弹性模量

$E_{ii}^{\text{C}} = \frac{{\left( {\sum\limits_{n = 1}^N {^n{f_i}} } \right)/\left( {{l_j}{t_{\text{G}}}} \right)}}{{\Delta {l_i}/{l_j}}}\;\;\;\;\;\;\;\;i = x, y$

(15)

$G_{ii}^{\text{C}} = \frac{{\left( {\sum\limits_{n = 1}^N {^n{f_j}} } \right)/\left( {{l_j}{t_{\text{G}}}} \right)}}{{\Delta {l_j}/{l_i}}}\;\;\;\;\;\;\;\;i = x, y\;\;\;j = x, y, z$

(16)

2. 数值模拟结果及讨论

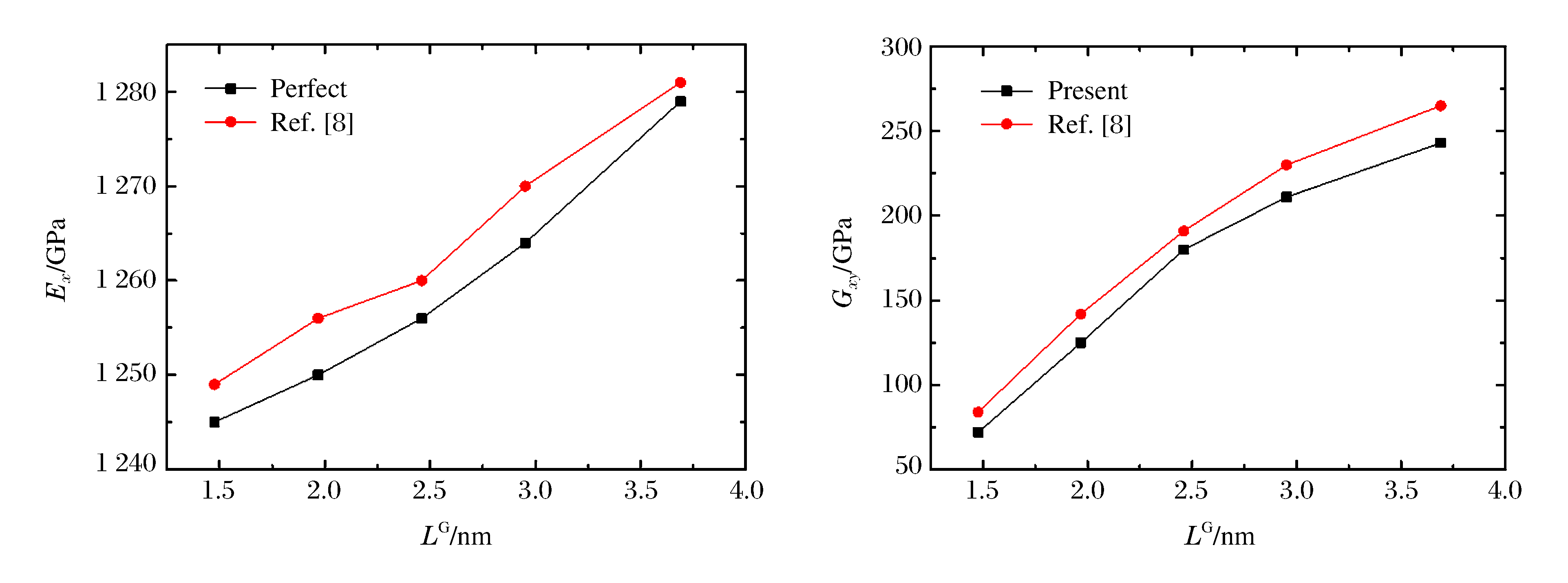
为了验证模拟计算的正确性和合理性，首先进行基于梁单元的纯石墨烯的仿真结果对比，构建了不同尺寸的石墨烯，计算其弹性模量，并与文献[8-9]作对比，如图 5所示。结果表明，石墨烯的弹性模量随尺寸的增大逐渐增大，仿真模拟结果与其他研究人员的结果基本吻合。同时说明，基于分子结构力学方法，利用有限元模拟的正确性和合理性。

图 5 不同尺寸石墨烯的杨氏模量和剪切模量

Figure 5. Young's modulus and shear modulus of graphene with different sizes

下载: 全尺寸图片幻灯片

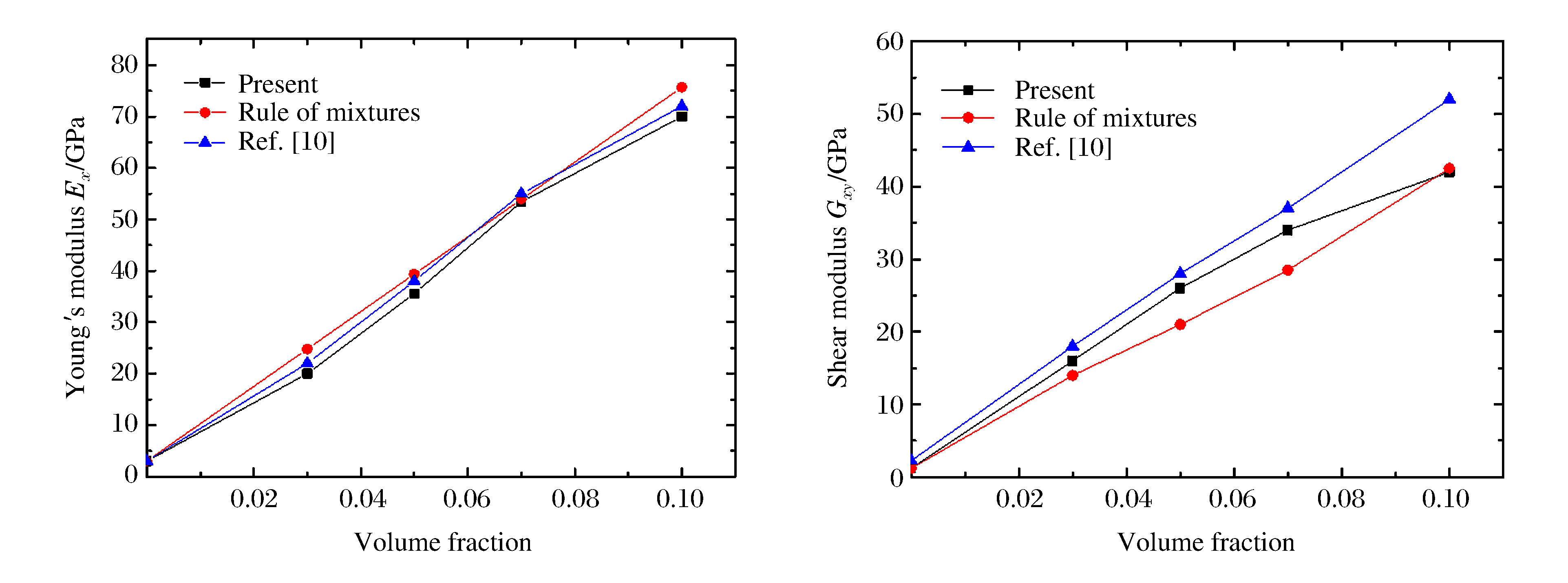
另外，为了表明界面层划分结果的正确性和合理性，同样进行了基于多尺度方法的石墨烯增强环氧树脂复合材料的有限元模拟，构建了不同体积分数下无缺陷石墨烯增强环氧树脂复合材料的代表性体积单元，并计算了其弹性模量。图 6结果对比可见，本研究的模拟结果与其他研究结果^[10]是基本一致的，说明界面层复杂性简化合理以及本研究模拟正确。另外，本研究带有缺陷的石墨烯增强环氧树脂复合材料的建模方法与无缺陷的是一致的，因此可以保证结果的正确性。

图 6 无缺陷石墨烯增强环氧树脂复合材料的杨氏模量和剪切模量

Figure 6. Young's modulus and shear modulus of perfect graphene reinforced epoxy composites

下载: 全尺寸图片幻灯片

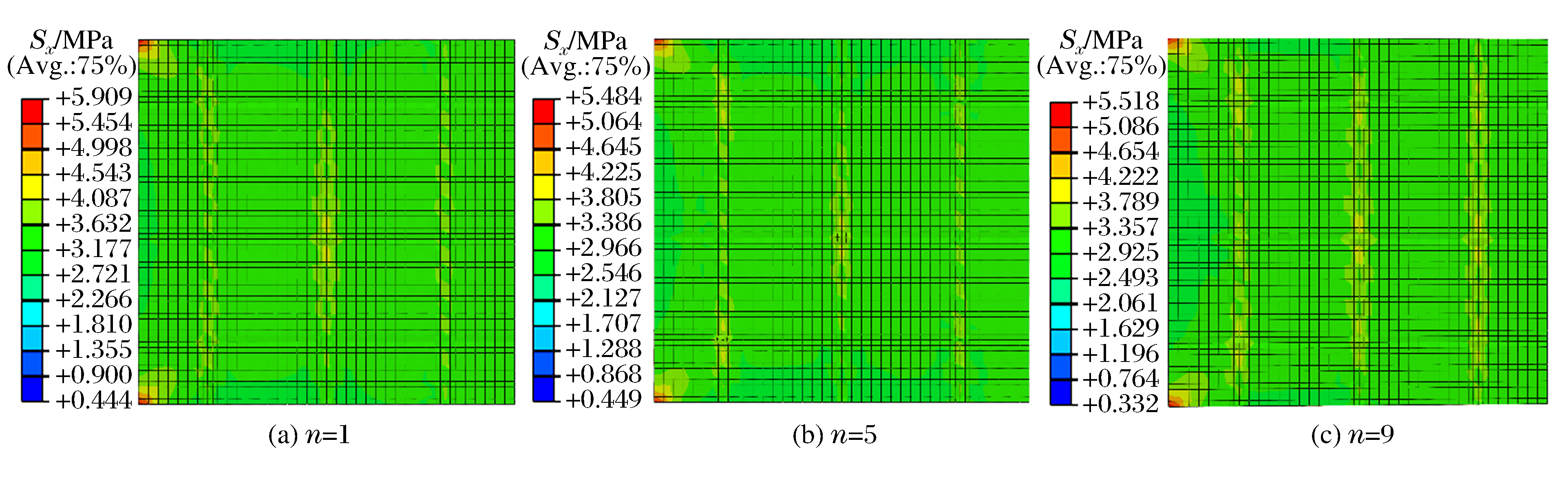
图 7分别为双原子空缺缺陷数量为1、5和9时界面层的应力云图。可以看出，应力集中出现在缺陷存在处，说明缺陷的存在的确对石墨烯增强复合材料的弹性模量造成一定的影响。另外对比图 7(a)、图 7(b)和图 7(c), 可以看出，随着缺陷数量的增加，应力集中现象会更明显，说明缺陷的数量越多，对复合材料弹性性能的影响会越大。另外，图 7(c)表明，随着缺陷的增加，出现了明显应力不均。这是由于石墨烯尺寸固定下，缺陷数量的增多使石墨烯的整体力学性能降低，各个缺陷之间的应力传递相互影响增大。

图 7 含有不同数量空缺缺陷石墨烯复合材料的界面层应力云图

Figure 7. Stress cloud map of interface layer containing different vacancy defects graphene composites

下载: 全尺寸图片幻灯片

图 8为S-W缺陷数量n分别为1、5和9时界面层的应力云图，可以发现，应力集中仍然出现在S-W缺陷处。然而对比图 8会发现，S-W缺陷造成的应力集中现象不如空缺缺陷明显。在缺陷数量方面，同样可以发现，随着缺陷数量的增加，应力集中现象也是逐渐增强的，但是增强幅度明显低于空缺缺陷的情况，说明缺陷的种类对石墨烯与界面层之间的应力传递有很大影响。

图 8 含有不同数量S-W缺陷石墨烯复合材料的界面层应力云图

Figure 8. Stress cloud map of interface layer containing different S-W defects graphene composites

下载: 全尺寸图片幻灯片

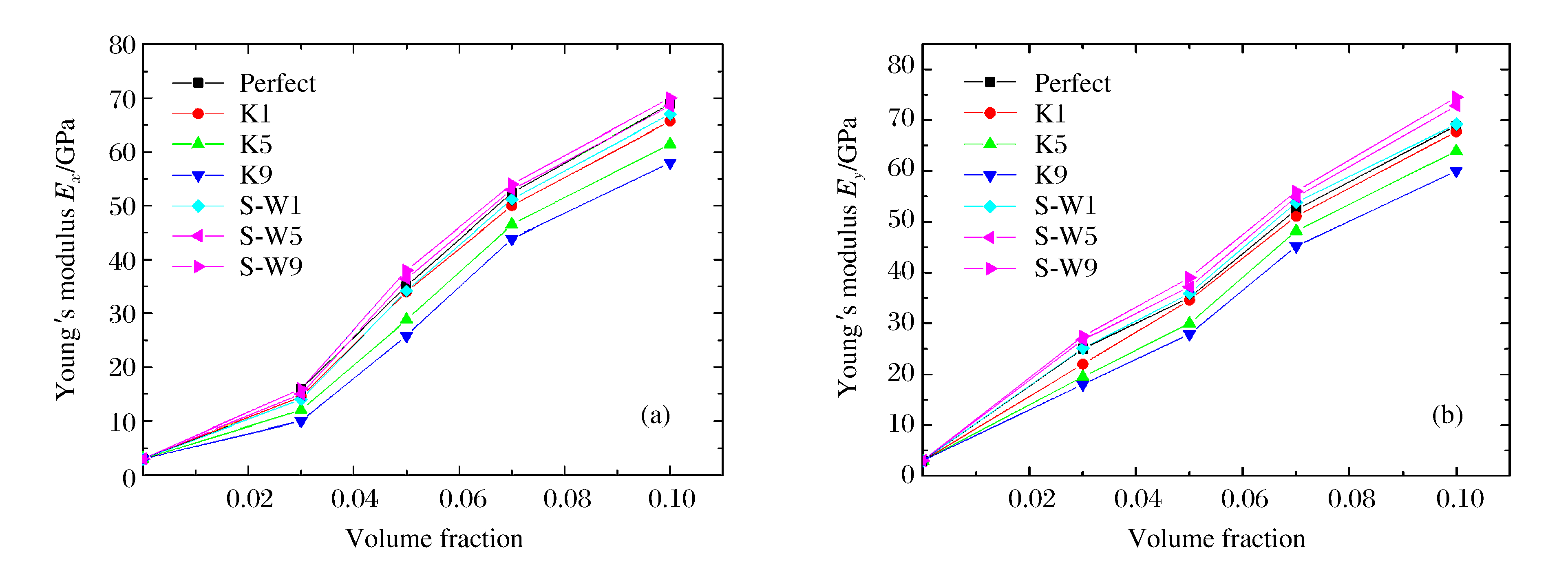
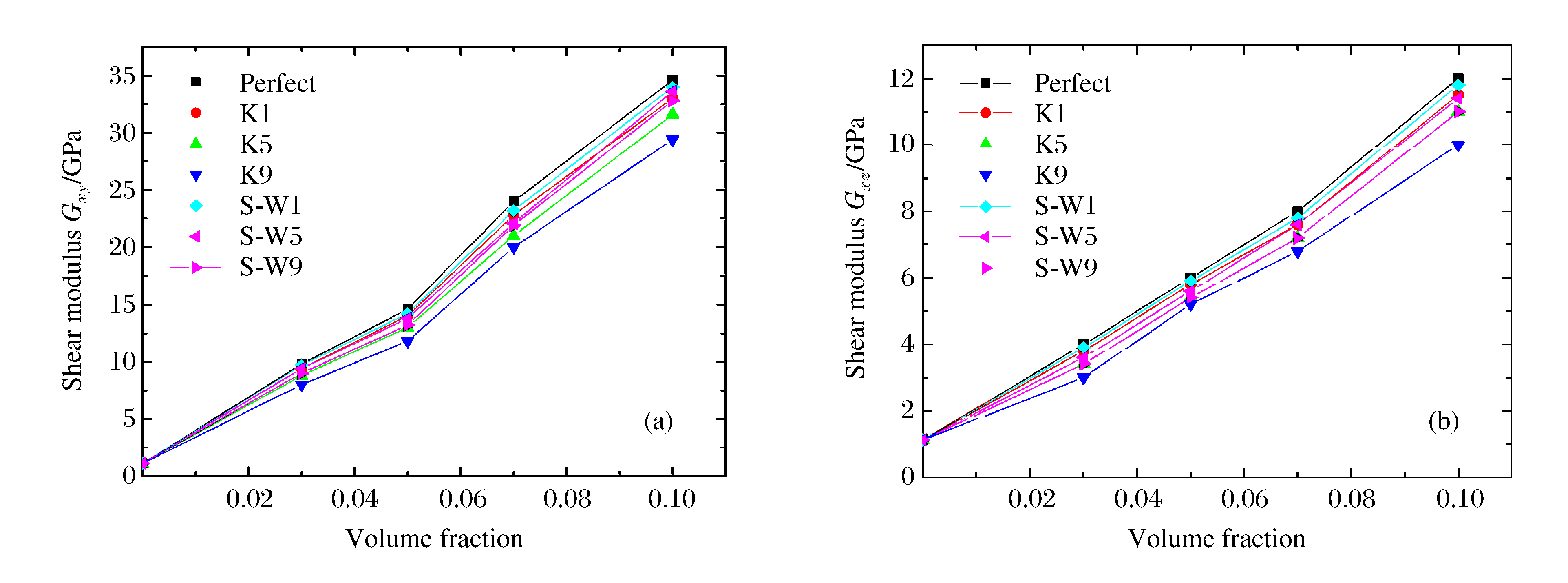
图 9和图 10表示了缺陷数量和种类对复合材料杨氏模量和剪切模量的影响，没有缺陷的石墨烯命名为Perfect，并与其他有缺陷的石墨烯增强树脂基复合材料作对比。

图 9 不同缺陷情况杨氏模量E_x和E_y随体积分数的变化

Figure 9. Variation of Young's modulus E_x and E_y with volume fraction under different defects

下载: 全尺寸图片幻灯片

图 10 不同缺陷情况剪切模量G_xy和G_xz随体积分数的变化

Figure 10. Variation of Young's modulus G_xy and G_xz with volume fraction under different defects

下载: 全尺寸图片幻灯片

图 9(a)表示了含有不同缺陷种类、数量的石墨烯对复合材料杨氏模量E_x产生的影响。可以发现，空缺缺陷明显降低了复合材料整体的杨氏模量E_x，并且随着缺陷数量的增加，影响越明显。然而，S-W缺陷在一定程度上却增加了复合材料的杨氏模量E_x。不过，随着S-W缺陷数量的增加，S-W缺陷对复合材料杨氏模量E_x的影响逐渐削弱。图 9(b)则表达了缺陷数量和种类对杨氏模量E_y的影响。可以发现，与杨氏模量E_x相比，E_y呈现出几乎同样的变化，只不过E_y的降低幅度要略大于E_x。并且，因为缺陷方向朝向y方向，杨氏模量E_y要高于E_x。另外，可以发现，随着石墨烯体积含量的增加，所有类型复合材料杨氏模量都呈现近似线性增长。综上所述，并不是所有缺陷都会降低复合材料的杨氏模量。空缺缺陷会明显降低复合材料的杨氏模量。S-W缺陷能在一定程度上增加其杨氏模量，这可能是因为在S-W缺陷处，中间一根键旋转90°，此时键的方向与拉伸方向相同，更容易进行应力的传递。另外，不论是空缺缺陷还是S-W缺陷，缺陷数量越多，增强或削弱能力都会降低。

图 10为缺陷数量和种类对环氧树脂基复合材料剪切模量G_xy和G_xz的影响。由图 10(a)可以发现，不论是空缺缺陷还是S-W缺陷都会降低复合材料的剪切模量G_xy。S-W缺陷的削弱能力要明显低于空缺缺陷。就缺陷数量来说，石墨烯缺陷数量越多，对剪切模量G_xy的影响越大，这与之前所提到的应力集中是有一定关系的。缺陷数量越多，应力集中越明显，这在一定程度上削弱了界面层之间应力传递，于是降低了剪切模量G_xz。并且空缺缺陷应力集中现象要明显强于S-W缺陷，说明空缺缺陷更容易降低复合材料的模量。从图 10(b)可以观察到，与杨氏模量的变化规律类似，剪切模量也出现一致的相似性，只不过剪切模量G_xz的大小要明显小于剪切模量G_xy，这与石墨烯面外弹性性能要远远弱于面内的弹性性能有关。另外，随着石墨烯体积含量的增加，剪切模量也出现线性增长。

最后，模拟了石墨烯增强环氧树脂复合材料在拉伸和剪切载荷下，界面层的正应力σ_x和切应力τ_xy随界面层位置的变化，即从X=0到X=L，进一步对比其与弹性模量之间的关系。

图 11为界面层的正应力σ_x和切应力τ_xy随界面层位置的变化曲线。由图 11 (a)可以看出，正应力σ_x从X=0到X=L/2，出现非线性的降低，且在X=0位置处的变化最明显。另外，可以看出不同缺陷之间有明显的差异，空缺缺陷的应力要明显低于S-W缺陷情况的应力，缺陷数量越多，应力值越小。与杨氏模量对比发现，同样的空缺缺陷的应力值要小于没有缺陷(Perfect)的应力值, 而S-W缺陷的应力值却略大于没有缺陷的复合材料。由图 11(b)可见，缺陷数量为零时切应力值最大，而S-W缺陷虽然比缺陷为零时要小，却还是略大于含有空缺缺陷情况的应力值，这与剪切模量的变化规律一致。另外，随着界面层位置的远离，发现切应力τ_xy的变化也出现了非线性降低，并且在靠近X=0处的变化同样更明显，在远离X=0处，每个模型的切应力都在零值附近起伏，数值基本相同。综上所述，一方面，在靠近位移约束为零的位置处，应力的变化要更明显；另一方面，界面层的应力传递一定程度上对应了缺陷对复合材料的影响。

图 11 界面层的正应力σ_x和切应力τ_xy随界面层位置的变化

Figure 11. Variation of normal stress σ_x and shear stress τ_xy at the interface layer with the position of the interface layer

下载: 全尺寸图片幻灯片

3. 结论

(1) 复合材料界面层的应力集中多发生在缺陷处，空缺缺陷的应力集中比S-W缺陷情况下更显著，随着缺陷数量的增加，应力集中现象越来越明显。

(2) 空缺缺陷会明显降低复合材料的杨氏模量，而S-W缺陷却在一定程度上增加了复合材料的杨氏模量。

(3) 无论是空缺缺陷还是S-W缺陷，均在一定程度上降低了复合材料的剪切模量。随着石墨烯体积含量的增加，杨氏模量和剪切模量均呈现线性增长。

(4) 界面层的应力传递一定程度上反映了缺陷对复合材料的影响，并且随着位置远离X=0处，界面层的正应力σ_x和切应力τ_xy出现非线性降低，且在X=0位置处，变化最明显。

图 1 石墨烯六边形晶格与有限元模型的等效替代

Figure 1. Equivalent substitution of graphene hexagon lattice with finite element model

下载: 全尺寸图片幻灯片

图 2 石墨烯C-C键与梁单元之间的变形等效

Figure 2. Deformation equivalence between the C-C bond of graphene and the beam element

下载: 全尺寸图片幻灯片

图 3 含有9个双原子空缺缺陷(a)和9个S-W缺陷(b)的有限元模型

Figure 3. Finite element model containing nine diatomic vacancy defects and nine S-W defects

下载: 全尺寸图片幻灯片

图 4 石墨烯增强环氧树脂基复合材料的代表性体积单元

Figure 4. Representative volume element of graphene reinforced epoxy matrix composites

下载: 全尺寸图片幻灯片

图 5 不同尺寸石墨烯的杨氏模量和剪切模量

Figure 5. Young's modulus and shear modulus of graphene with different sizes

下载: 全尺寸图片幻灯片

图 6 无缺陷石墨烯增强环氧树脂复合材料的杨氏模量和剪切模量

Figure 6. Young's modulus and shear modulus of perfect graphene reinforced epoxy composites

下载: 全尺寸图片幻灯片

图 7 含有不同数量空缺缺陷石墨烯复合材料的界面层应力云图

Figure 7. Stress cloud map of interface layer containing different vacancy defects graphene composites

下载: 全尺寸图片幻灯片

图 8 含有不同数量S-W缺陷石墨烯复合材料的界面层应力云图

Figure 8. Stress cloud map of interface layer containing different S-W defects graphene composites

下载: 全尺寸图片幻灯片

图 9 不同缺陷情况杨氏模量E_x和E_y随体积分数的变化

Figure 9. Variation of Young's modulus E_x and E_y with volume fraction under different defects

下载: 全尺寸图片幻灯片

图 10 不同缺陷情况剪切模量G_xy和G_xz随体积分数的变化

Figure 10. Variation of Young's modulus G_xy and G_xz with volume fraction under different defects

下载: 全尺寸图片幻灯片

图 11 界面层的正应力σ_x和切应力τ_xy随界面层位置的变化

Figure 11. Variation of normal stress σ_x and shear stress τ_xy at the interface layer with the position of the interface layer

下载: 全尺寸图片幻灯片

表 1 等效梁单元的材料属性

Table 1. Material properties of the equivalent beam element

d/nm	L/nm	k_r/(μN·rad^-1)	k_θ/(aN·m·rad^-2)	k_τ/(aN·m·rad^-2)	E/GPa	G/GPa
0.146	0.142	0.652	0.875	0.278	5 780	878

下载: 导出CSV

表 2 石墨烯的材料属性参数

Table 2. Material property parameters of graphene

E_x/GPa	E_y/GPa	E_z/GPa	ν₁₂	ν₁₃	ν₂₃	G_xy/GPa	G_xz/GPa	G_yz/GPa
730	709	58.5	0.46	0.2	0.2	434	7.5	7.5

下载: 导出CSV

参考文献(17)

[1]	NOVOSELOV K S, GEIM A K, MOROZOV S V, et al.Electric field effect in atomically thin carbon films[J].Science, 2004, 306(5696):666-669. doi: 10.1126/science.1102896
[2]	BALANDIN A A, GHOSH S, BAO W Z, et al.Superior thermal conductivity of single-layer graphene[J].Nano Letters, 2008, 8(3):902-907. doi: 10.1021/nl0731872
[3]	刘晓毅, 王奉超, 吴恒安.石墨烯及其复合材料纳米力学研究进展[J].固体力学学报, 2016, 37(5):398-420. http://www.cnki.com.cn/Article/CJFDTOTAL-GTLX201605002.htm LIU X Y, WANG F C, WU H A.Progress in nanomechanical research of graphene and its composites[J].Acta Mechanica Solida Sinica, 2016, 37(5):398-420. http://www.cnki.com.cn/Article/CJFDTOTAL-GTLX201605002.htm
[4]	LEE C G, WEI X D, KYSAR J W, et al.Measurement of the elastic properties and intrinsic strength of monolayer grapheme[J].Science, 2008, 321(5887):385-388. doi: 10.1126/science.1157996
[5]	RESKETI N A, AMIRI H A, DEHESTANI M.Effects of size and shape on elastic constants of graphene sheet[J].Structures, 2018, 13:131-138. doi: 10.1016/j.istruc.2017.12.005
[6]	VUL A Y, SOKOLOV V I.Nanocarbon studies in Russia:from fullerene to nanotubes and nanodiamonds[J].Nanotechnologies in Russia, 2009, 4(7/8):397-414. doi: 10.1134/S1995078009070027
[7]	RAO C N R, BISWAS K, SUBRAHMANYAM K S, et al.Graphene, the new nanocarbon[J].Journal of Materials Chemistry, 2009, 19(17):2457-2469. doi: 10.1039/b815239j
[8]	SPANOS K N, GEORGANTZINOS S K, ANIFANTIS N K.Mechanical properties of graphene nanocomposites:a multiscale finite element prediction[J].Composite Structures, 2015, 132:536-544. doi: 10.1016/j.compstruct.2015.05.078
[9]	SPANOS K N, ANIFANTIS N K.Finite element prediction of stress transfer in graphene nanocomposites:the interface effect[J].Composite Structures, 2016, 154:269-276. doi: 10.1016/j.compstruct.2016.07.058
[10]	GIANNOPOULOS G I, KALLIVOKAS I G.Mechanical properties of graphene based nanocomposites incorporating a hybrid interphase[J].Finite Element in Analysis and Design, 2014, 90:31-40. doi: 10.1016/j.finel.2014.06.008
[11]	PAPAGEORGIOU D G, KINIOCH I A, YOUNG R J.Mechanical properties of graphene and graphene-based nanocomposites[J].Progress in Materials Science, 2017, 90:75-127. doi: 10.1016/j.pmatsci.2017.07.004
[12]	孙帅.石墨烯缺陷的特点与应用研究[D].天津: 天津大学, 2015: 10-30. SUN S.Study on the characteristics and application of graphene defects[D].Tianjin: Tianjin University, 2015: 10-30.
[13]	韩同伟, 贺鹏飞, 王健, 等.单层石墨烯薄膜拉伸变形的分子动力学模拟[J].新型炭材料, 2010, 25(4):261-266. http://d.old.wanfangdata.com.cn/Periodical/xxtcl201004004 HAN T W, HE P F, WANG J, et al.Molecular dynamics simulation of tensile deformation of monolayer graphene films[J].New Carbon Materials, 2010, 25(4):261-266. http://d.old.wanfangdata.com.cn/Periodical/xxtcl201004004
[14]	韩同伟, 施元君, 贺鹏飞, 等.Stone-Wales拓扑缺陷对石墨烯拉伸力学性能的影响[J].固体力学学报, 2011, 32(6):619-624. http://d.old.wanfangdata.com.cn/Periodical/gtlxxb201106011 HAN T W, SHI Y J, HE P F, et al.Effect of Stone-Wales topological defects on tensile mechanical properties of graphene[J].Acta Mechanica Solida Sinica, 2011, 32(6):619-624. http://d.old.wanfangdata.com.cn/Periodical/gtlxxb201106011
[15]	韩同伟, 贺鹏飞, 王健, 等.空位缺陷对单层石墨烯薄膜拉伸力学性能的影响[J].同济大学学报, 2010, 38(8):1210-1214. doi: 10.3969/j.issn.0253-374x.2010.08.020 HAN T W, HE P F, WANG J, et al.Effect of vacancy defects on tensile mechanical properties of single layer graphene films[J].Journal of Tongji University, 2010, 38(8):1210-1214. doi: 10.3969/j.issn.0253-374x.2010.08.020
[16]	LI C Y, CHOU T W.A structural mechanics approach for the analysis of carbon nanotubes[J].International Journal of Solids and Structures, 2003, 40(10):2487-2499. doi: 10.1016/S0020-7683(03)00056-8
[17]	LI C Y, CHOU T W.Elastic moduli of multi-walled carbon nanotubes and the effect of van der Waals forces[J].Composites Science and Technology, 2003, 63(11):1517-1524. doi: 10.1016/S0266-3538(03)00072-1