Crystal Plasticity Finite Element Simulation of High-Rate Shock Deformation Process of <100> LiF
-
摘要: 结合状态方程建立晶体塑性有限元模型，模拟高速冲击加载条件下<100> LiF的动态弹塑性大变形行为，得到应力波剖面特征、动态力学演化规律及其连续介质力学根源。结果表明：毫米级样品经约15 GPa以内的低压冲击，波剖面具有弹塑性双波响应、弹性前驱衰减和应力松弛现象，其决定性因素包括样品厚度、外加压力和材料本构；从连续介质力学角度分析得到,应力松弛本质上是由于黏性塑性流动，导致总应变增速小于塑性应变增速，从而使弹性应变减小、压力降低；提出用压力关于时间的三阶导数大于零作为判断条件，对应力波剖面上双波和单波响应的临界压力进行估测，发现随着样品掺杂浓度的增加，临界压力增大；高速冲击变形的温升效应不可忽略，且温升绝大部分来自弹性体积变形的贡献。Abstract: A crystal plasticity finite element model combined with equation of state was built to simulate the dynamic elastic-plastic large deformation behavior of <100> LiF under high-rate shock loading. The characterization of the stress wave profile, the patterns of the dynamic mechanical evolution and their essential causes in view of the continuum mechanics were obtained through simulations, with the following results achieved: (1) the wave profiles of millimeter-sized specimens exhibit elastic-plastic two-wave response, elastic precursor decay and stress relaxation below 15 GPa; (2) in view of continuum mechanics, the stress relaxation is essentially due to the viscous plastic flow which accounts for the increase rate of the total strain being less than that of the plastic strain, and which further reduces the elastic strain and pressure; (3) the third derivative of pressure to time being greater than zero was proposed as a criterion for estimating the critical pressure of the two-wave and the one-wave response of the stress wave profile, and the estimation result indicated that the critical pressure increased with the increase of the doping concentration in specimen; (4) the effect of temperature rise during the high-rate shock deformation is non-negligible, and the elastic volumetric deformation contributes to most of the temperature rise.
-
Key words:
- LiF /
- crystal plasticity /
- equation of state /
- stress relaxation /
- two-wave response
-
俯冲带是地球系统水循环的关键场所，板片俯冲过程中含水矿物的结构稳定性决定了水输入地球深部的方式、形式和数量，同时也影响了板片的俯冲深度和俯冲速度，以及地幔物质的黏度和熔融情况，并可诱发岛弧火山和深源地震[1-3]。早期的洋-洋俯冲、洋-陆俯冲和陆-陆俯冲，俯冲板片的温度普遍偏低，板片携带物的结构稳定性主要取决于其所处深度（正压力）和移动速度（剪切力）[4]。旋转式金刚石对顶砧压机(RDAC)可通过垂直加压和水平旋转模拟冷俯冲板片的高压剪切作用，是研究含水矿物结构稳定性的有效工具。三水铝石（
$\gamma $ -Al(OH)3）是金属羟基化合物（如铝土矿、褐铁矿和硬锰矿）的重要代表，与非金属羟基化合物（含水硅酸盐矿物、含水磷酸盐矿物和含水硫酸盐矿物等）同时广泛存在于洋壳和陆壳的沉积岩和变质岩中。研究高压剪切作用下三水铝石的结构稳定性对于研究金属羟基类矿物随着板块俯冲进入地球深部的结构稳定性起到了一定的指导作用。三水铝石是铝土矿的主要成分，是含铝硅酸盐矿物分解和水解的产物。三水铝石含羟基，呈弱碱性，医学上常作为抗酸药物用以缓解胃酸过多造成的胃灼热和胃不适；三水铝石为层状多孔铝质矿物，工业上常用作玻璃、纸张、油墨、陶瓷、润滑油、化妆品等的色谱，制造业中的吸收剂，同时也是冶炼铝最重要的矿物原料[5-6]。常温常压下，
$\gamma $ -Al(OH)3属于单斜晶系，空间群为P21/n （No.14，晶胞中分子个数Z=8），由夹心饼干式的(OH)-Al-(OH)配位八面体层沿c轴方向平行叠置而成，Al3+仅占据夹层中2/3的八面体空隙，羟基在层内和层间各占一半，如图1所示。$\gamma $ -Al(OH)3与α-Al(OH)3 （空间群P21/n）、$\,\beta $ -Al(OH)3 （空间群P-1）和$\zeta $ -Al(OH)3 （空间群P-1）3种Al(OH)3化合物互为同质多象。
图 1$\gamma$ -Al(OH)3 的晶体结构（绿色键为层内羟基，黄色键为层间羟基）Figure 1. Crystal structure of$\gamma $ -Al(OH)3 (The green binding is intralayer OH, and the yellow binding is interlayer OH.)常温准静水压条件下，
$\gamma $ -Al(OH)3在压力约为3 GPa时发生结构相变。Huang等[7-8]根据拉曼光谱和X射线衍射测量结果，认为$\gamma $ -Al(OH)3的高压相为$\,\beta $ -Al(OH)3 （空间群P-1）。Liu等[6, 9]根据高压X射线衍射谱和红外吸收谱，将高压相定为正交结构。Komatsu等[10]根据单晶衍射和分子动力学计算结果，将高压相定为单斜结构$\eta $ -Al(OH)3（空间群P21/c）。比较前人对$\gamma $ -Al(OH)3高压相的解析结果，可以发现，无论将其定为正交相还是单斜，其晶胞参数的主要差异在于$\,\beta $ 角不同。研究结果表明，在准静水压环境下，$\gamma $ -Al(OH)3结构的相变为微结构调整，$\,\beta $ 角从初始的94.61°减小到高压相的90°或90.36°，晶面没有滑移，晶轴和晶胞体积没有出现不连续相变。静水压环境是一种理想的实验条件，自然界中绝大多数物质都处于非静水压环境，尤其是运动的俯冲板片。不仅俯冲板片的上下层之间和前进方向存在极大的差应力，并伴随火山爆发或地震，而且其内部也会出现明显挤压。应用RDAC创造的高压剪切环境能够更真实地反映俯冲板片携带物进入地球深部的受力过程。为此，本研究将应用RDAC装置，通过探测高压剪切作用下含水矿物的高压原位拉曼谱和卸压衍射谱，研究$\gamma $ -Al(OH)3的结构稳定性。1. RDAC和实验方法
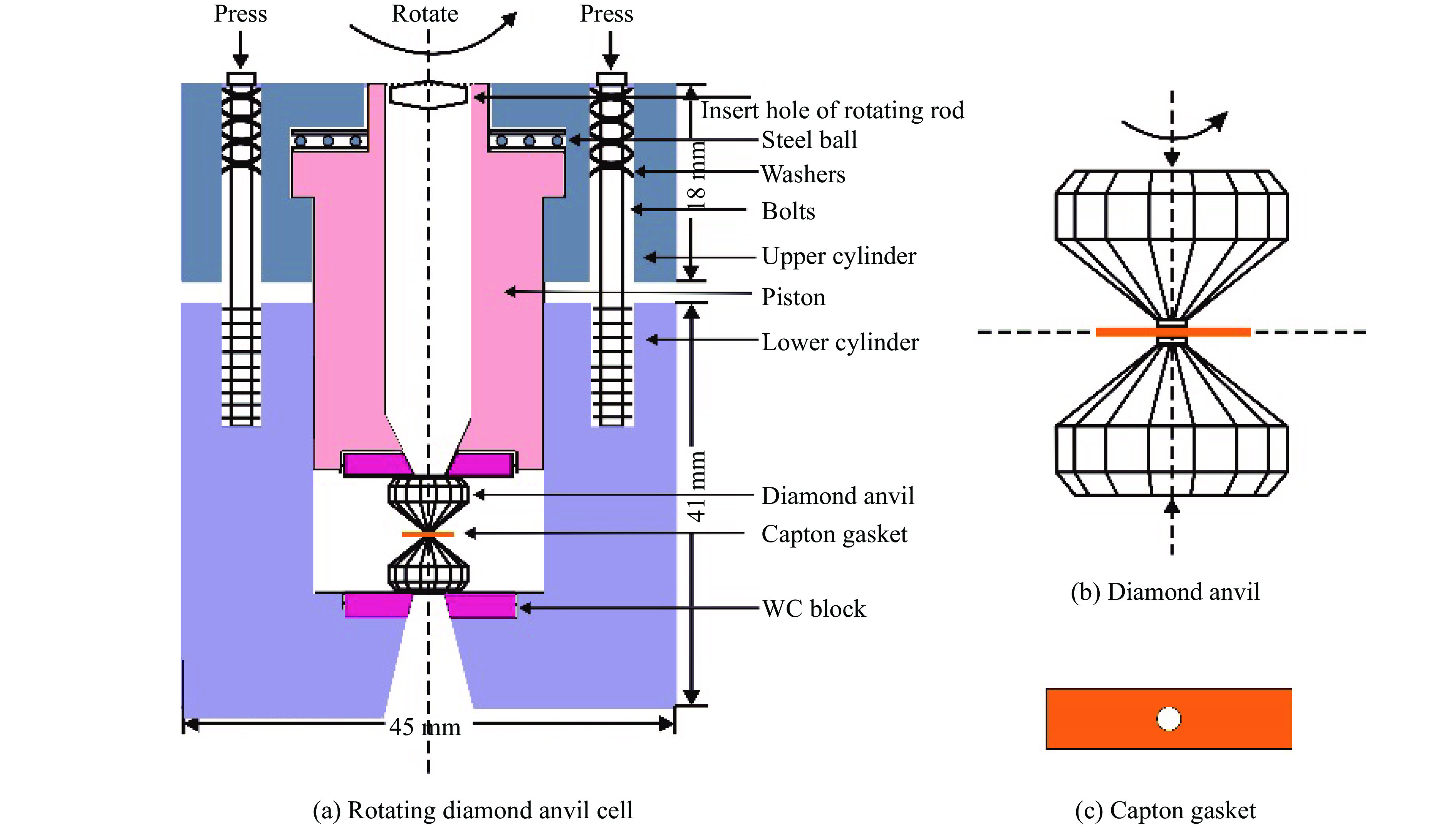
如图2(a)所示，RDAC简称旋转压机，由上下外圆筒、内活塞和金刚石压砧3个主要部分构成。高压剪切实验通过转动加压螺丝使压机的上下圆筒不断靠近，推动活塞和下圆筒上的金刚石压砧面挤压封垫，样品受到竖直方向的压缩。加压后，用大号六角旋转杆转动内活塞，压实的样品在水平方向被剪切。为了使样品达到最强剪切并确保金刚石压砧在高压剪切实验中的安全性，对DAC进行特殊加工设计，优化了封垫材料。金刚石压砧的腰面从普通的16面改为32面，在砧面磨出一个20 μm高的圆柱形，如图2(b)所示。这样设计加工的金刚石压砧能确保较大压力下的灵活旋转，其棱角和整体无破损。比较铼片、T301不锈钢片和聚酰亚胺塑料片（capton）等封垫的硬度，发现在加压过程中，capton封垫更容易被压缩，可保证样品为主要受力体，高压剪切效果明显，因此在进行高压剪切实验时选用了capton封垫片，如图2(c)所示。
三水铝石购于阿尔法公司，常温常压下拉曼谱和衍射谱测试结果表明三水铝石为单一
$\gamma $ -Al(OH)3相（见结果部分）。首先，将样品装入直径400 μm的capton封垫样品孔，压实后清除金刚石砧面和封垫周围的残余样品并合上压机。对样品分步加压至1.5 GPa（中心压力）并旋转一周（转动角为45°）；继续加压至3.5 GPa后，再次旋转一周。加压和旋转过程中测试样品腔内21个点位的压力和拉曼光谱。高压剪切实验的压力标定采用金刚石拉曼主峰波数随压力增加而频移的方法，计算公式为p=AΔωω0[1+12(B−1)Δωω0] (1) 式中：p为压力，
$\omega $ 0=1334 cm−1，A=547；B=3.75，Δ$\omega / \omega $ 0为相对拉曼波数变化[11]。同时，为与本研究进行对比，开展了静水压实验，选用普通对称型金刚石对顶砧，砧面直径为500 μm，T301不锈钢片封垫，预压封垫厚度约50 μm，样品孔直径为150 μm。先将三水铝石样品压成厚约20 μm的薄片，取直径约为80 μm的一片装入样品腔，并在样品周围放置2颗红宝石颗粒作为压标，利用红宝石荧光漂移法测定实验压力[12]。将液氮冷冻的Ar装入样品腔中作为传压介质，以使样品的实验压力保持在准静水压力。采用Renishaw 2000型显微共聚焦拉曼光谱仪测试拉曼光谱。激光器光源波长为532 nm，样品处光斑直径约为2 μm。通过20倍长焦距物镜背散射收集样品的拉曼信号。光栅刻度为1 800 l/mm，光谱分辨率为1 cm−1。采谱时间为120 s，测量范围包括两个部分：低波数段100～1 100 cm−1和高波数段2 800～4 800 cm−1。衍射谱测试采用Rigaku D-max Rapid-V型微区衍射仪（μ-XRD），该微区衍射仪配备了微聚焦旋转阳极源（microMax-007HFM X-ray generator）和弯曲成像板探测器。Cu Kα边辐射激发时的工作电压和电流分别为40 kV和30 mA。准直管直径为0.1 mm，收集时间为300 s。内置CCD照相机收集的二维图像由Rigaku 2DP软件转换为衍射角和强度数据。
2. 结果与讨论
2.1 高压剪切实验中
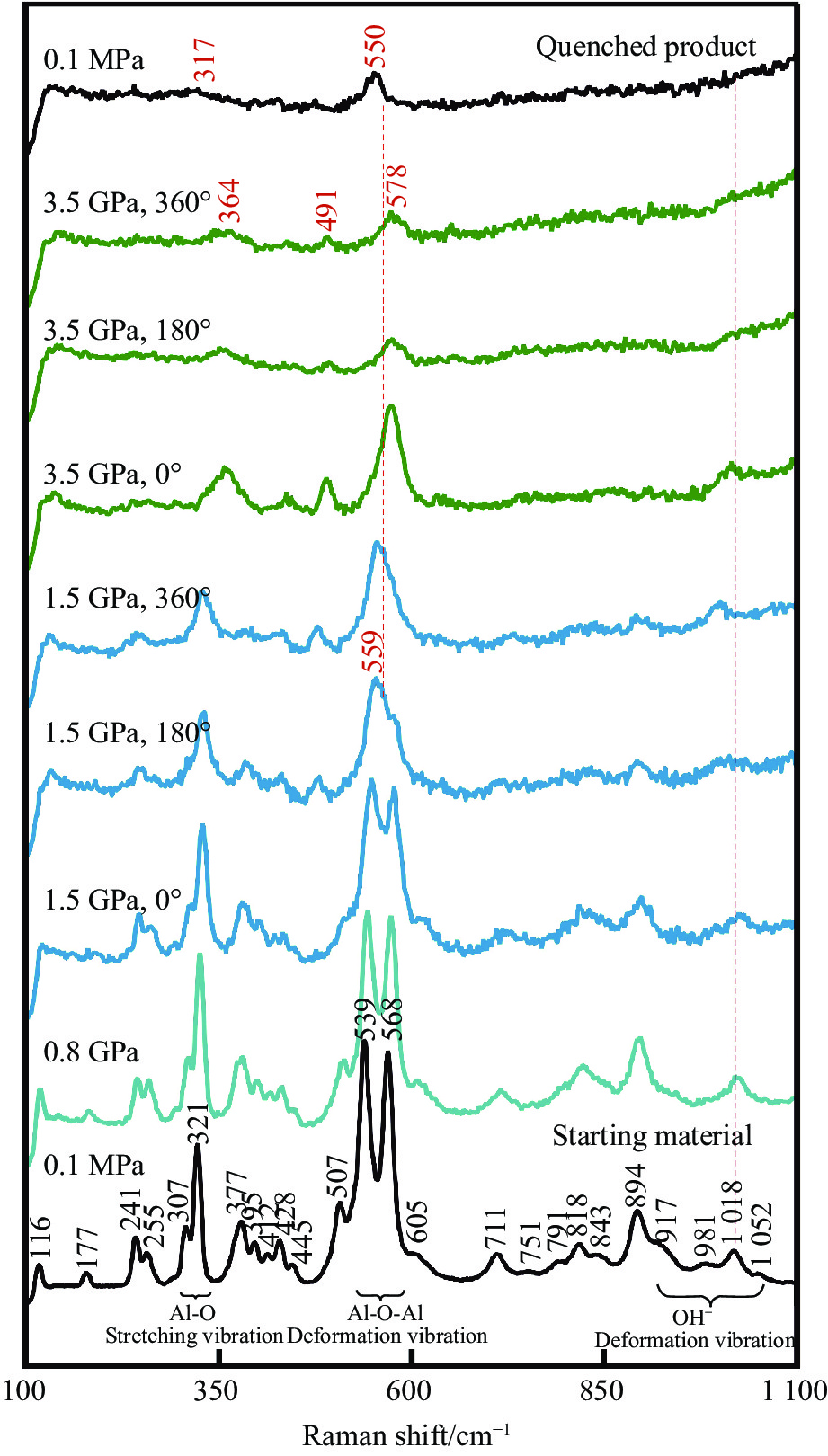
$\gamma $ -Al(OH)3的拉曼光谱$\gamma $ -Al(OH)3的拉曼振动光谱主要分布在3300～3700 cm−1高波数段（图3中常压谱）和100～1100 cm−1低波数段（图4中常压谱）。高波数段包含3618、3524、3434和3363 cm−1 4个超强拉曼峰，属于羟基伸缩振动。低波数段的较强拉曼峰有1018、894、818、711、568、539、507、428、395、377、321、307、255、241、177和116 cm−1，较弱拉曼峰有1052、981、922、843、791、751、605、445和412 cm−1。其中，1052、1018、981和922 cm−1峰属于羟基变形振动，对应高波数段的羟基伸缩振动；568、539和321 cm−1为低波数段的3个最强峰，568和539 cm−1峰归属于Al-O-Al变形振动，321 cm−1与其肩峰307 cm−1归属于Al-O伸缩振动[13]。
图 3 高压剪切作用下$\gamma $ -Al(OH)3在2 800～4 800 cm−1高波数段的代表性拉曼光谱Figure 3. Representative Raman spectra of$\gamma $ -Al(OH)3 in the range of 2 800−4 800 cm−1 under high pressure and shear stress
图 4 高压剪切作用下$\gamma $ -Al(OH)3在100～1 100 cm−1低波数段的代表性拉曼光谱Figure 4. Representative Raman spectra of$\gamma $ -Al(OH)3 in the range of 100−1 100 cm−1 under high pressure and shear stress图3、图4也显示了高压剪切实验中
$\gamma $ -Al(OH)3的拉曼测试光谱。图3中高波数段代表性拉曼光谱中，加压至中心压力0.8 GPa时，4个羟基伸缩振动峰的相对强度发生了明显变化，3524 cm−1峰成为最强拉曼峰。常压下3363和3434 cm−1拉曼峰分别向低波数方向移动了4个波数，即3359和3430 cm−1拉曼峰；常压下的3524和3618 cm−1峰没有随着压力的增大向低波数方向移动。继续加压至中心压力1.5 GPa，仍然是3524 cm−1峰最强；3363和3434 cm−1两个拉曼峰继续向低波数方向移动，峰位分别为3348和3422 cm−1；3524和3618 cm−1峰仍然未发生位移；同时3560 cm−1处出现一个新的拉曼峰（红色箭头所示）。加压至中心压力1.5 GPa时，进行第1次旋转剪切。转角为180°时的代表性拉曼光谱显示，常压下的4个强拉曼峰完全消失，3560 cm−1处形成新的最强峰，峰宽明显大于常压拉曼谱。同时，3303 cm−1处也出现了一个新的拉曼宽峰（蓝色箭头所示），强度较弱。继续转动压机，1.5 GPa、转角360°时的代表性拉曼谱显示，4362和4392 cm−1处出现两个波数差为30 cm−1的红宝石荧光峰（图3中“#”处）。继续加压至3.5 GPa并旋转压机，第2次高压剪切实验的拉曼谱显示，样品新出现的2个拉曼峰稳定，相对强度不变，峰位移动小（两个新峰的压力行为与初始结构的3363、3434 cm−1峰随着压力增加向低波数方向快速移动完全不同）。新出现的两个拉曼峰和红宝石荧光峰在腔体内5～6个测试点都能够观察到。3.5 GPa、转角360°时，样品拉曼峰的读数为3304和3561 cm−1。缓慢卸压至常压，高压剪切作用观察到的样品拉曼峰保存下来，其峰位为3306和3563 cm−1。高波数段的拉曼谱显示，高压剪切作用下，三水铝石的(OH)-Al-(OH)配位八面体层内和层间羟基环境发生改变，羟基伸缩振动由4个强峰变为2个弱峰。根据Walrafen等[14]报道的含水氢氧化物特征拉曼谱，加压至1.5 GPa时出现了3560 cm−1新峰，可认为含水羟基
${\rm{H}}_3{\rm{O}}_2^- $ （H(2) –${\rm{O}}_{(1)}^{\text{(−)}}$ ······H(1)–O(2)–H(3)）中没有氢键作用的羟基OH（O(2)–H(3)）伸缩振动[15]。加压至1.5 GPa、旋转180°时出现的3303 cm−1新峰为${\rm{H}}_3{\rm{O}}_2^- $ 和H2O中有氢键作用的羟基OH（O(2) –H(1)）伸缩振动峰[14-15]。因此，在高压剪切作用下三水铝石发生了脱羟基，生成水（H2O）和水合离子${\rm{H}}_3{\rm{O}}_2^- $ 。图4为低波数段代表性拉曼谱。随着压力增加，非静水压环境下三水铝石的拉曼峰强度开始减弱。中心压力为1.5 GPa时，三水铝石的拉曼模式基本不变。在1.5 GPa下旋转180°时，常压539和568 cm−1峰合二为一，在559 cm−1处产生拉曼峰，但拉曼峰强度明显减弱；原来以1018 cm−1为代表的4个羟基变形振动峰仍然可见。继续加压至3.5 GPa时，拉曼模式不变。转动360°时，拉曼峰强度明显降低，拉曼峰读数分别为364、491和578 cm−1。缓慢卸至常压，可见峰波数为317和550 cm−1。低波数段拉曼谱显示，在高压剪切作用下，三水铝石中(OH)-Al-(OH)配位八面体层内的Al-O-Al变形振动和Al-O伸缩振动受到压制，(OH)-Al-(OH)配位八面体层内和层间的羟基变形振动遭到破坏。
2.2 准静水压下的拉曼光谱
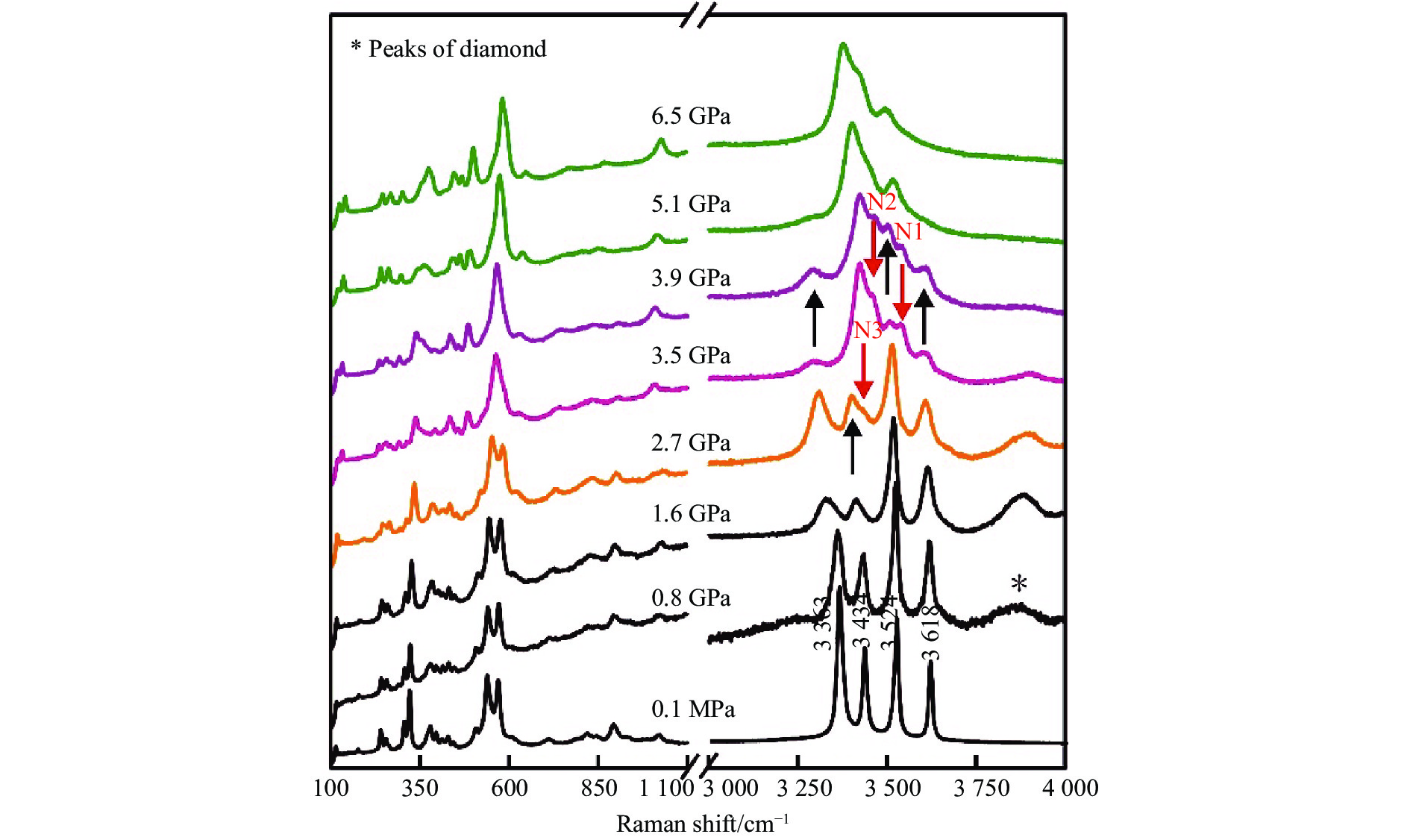
为查明高压剪切作用下三水铝石结构的失稳机制，区分静水压和非静水压环境对其结构稳定性的影响，同时开展了准静水压环境下三水铝石的原位高压拉曼光谱实验，如图5所示。实验结果显示：(1) 在2.7～3.9 GPa压力区间内，三水铝石发生了结构相变；(2) 高波数段的4个常压拉曼峰相继在相变区间消失（黑色箭头），并出现N1、N2和N3（红色箭头）3个新峰；(3) 在低波数段，随着压力增大，除最强峰快速向高波数方向移动而与邻位峰合并之外，谱图形状基本保持不变，峰的相对强度不变。上述实验结果与前人在准静水压环境下测试的原位拉曼光谱结果[7-8]一致，但与高压剪切环境下的拉曼光谱结果完全不同。对比前人在准静水压条件下测试的三水铝石单晶和粉晶同步辐射X射线衍射数据，本研究在准静水压环境下观察到的三水铝石结构相变就是
$\gamma $ -Al(OH)3单斜相（空间群P21/n，Z=8)到正交相（空间群Pbca，Z=8）[6]或单斜结构$\eta $ -Al(OH)3（空间群P21/b, Z=8）的转变[10]。$\,\beta $ 角由$\gamma $ -Al(OH)3相的94.61°转变为高压相的90°或90.34°，晶轴变化较小。因此，三水铝石 (OH)-Al-(OH)配位八面体层平行叠置而成的夹心饼干式框架没有改变，(OH)-Al-(OH)配位八面体层的羟基环境得到调整。
图 5 准静水压作用下$\gamma$ -Al(OH)3的代表性拉曼光谱Figure 5. Representative Raman spectra of$\gamma $ -Al(OH)3 under quasi-hydrostatic pressure2.3 高压剪切下的X射线衍射谱
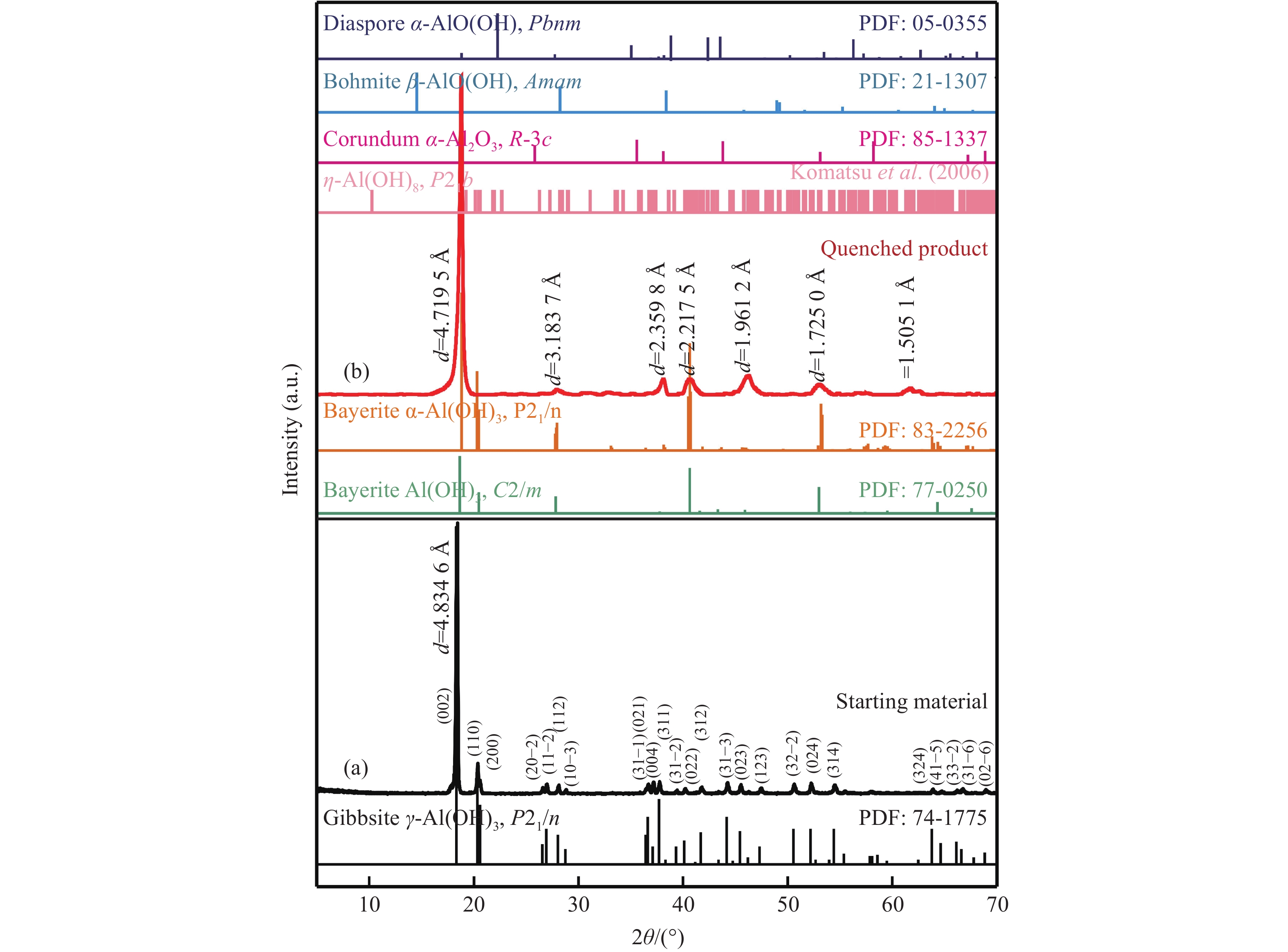
图6为三水铝石高压剪切实验样品的微区X射线衍射谱。其中，图6(a)为常温常压下三水铝石的衍射谱。可以看到，所有衍射峰都较尖锐，且均可指标化为三水铝石
$\gamma $ -Al(OH)3的单斜结构P21/n （No.14，Z=8），计算晶格常数（a=8.6552 Å，b=5.0722 Å，c=9.7161 Å，$\,\beta $ =94.61°，V = 425.17 Å3）与文献[7]中的数据基本一致，反映了样品的结晶度较高，且没有杂质。图6(b)为高压剪切作用后三水铝石卸至常压的衍射谱。卸压衍射谱具有7个较强衍射峰，其晶面间距（d）分别为4.7195、3.1837、2.3598、2.2175、1.9612、1.7250和1.5051 Å，其中最强衍射峰的晶面间距为4.7195 Å。对比三水铝石的卸压衍射谱与常压相衍射谱发现，三水铝石卸压后的最强峰d值略小于$\gamma $ -Al(OH)3常压相的最强峰d值（d(002)=4.8346 Å），同时缺失$\gamma $ -Al(OH)3相在2$\theta $ ≈20°的(110)和(200)峰。
图 6 高压剪切作用前后样品的X射线衍射谱及其晶体结构指认Figure 6. X-ray diffraction spectra of starting material and quenched product and the index of crystal structure为了解读高压剪切作用后三水铝石卸压样品的晶体结构，将卸压样品的衍射谱与三水铝石的高压相
$\eta $ -Al(OH)3（P21/b）、脱水产物AlO(OH)和Al2O3以及其他三水铝石结构（多形三羟铝石（$\alpha $ -Al(OH)3，P21/n）、三斜水铝石（$\,\beta $ -Al(OH)3，P-1）和辉绿岩（$\zeta $ -Al(OH)3，P-1））的标准数据进行对比[16-22]。对比结果显示，卸压衍射谱与三水铝石高压相以及三水铝石脱水产物的衍射谱最强峰位置和衍射谱模式都存在较大差异。然而，卸压衍射谱却与三羟铝石结构的衍射谱相似，其最强峰均在d=4.72 Å附近，6个弱峰的d值在三羟铝石相中都有衍射线条对应。较大区别在于卸压谱缺失2$\theta $ ≈20°的衍射线条。尽管实验室里的微区衍射数据不能用于晶体结构精修和解析卸压相的结构，但是通过与其相关物相衍射谱图对比分析，发现初始$\gamma $ -Al(OH)3的单斜结构经高压剪切作用后在c轴方向发生较大压缩，晶胞间距变小；a、b轴水平方向的(OH)-Al-(OH)配位八面体层框架中Al-O原子间距不变，其在层间的对称性发生了明显变化。需要说明的是，由于氢原子极弱的X射线衍射效应，无法根据现有的常规X射线衍射结果分析氢原子是否从(OH)-Al-(OH)八面体层框架中分离出来形成小分子。2.4 高压剪切实验中腔体压力分布及结构转变机制
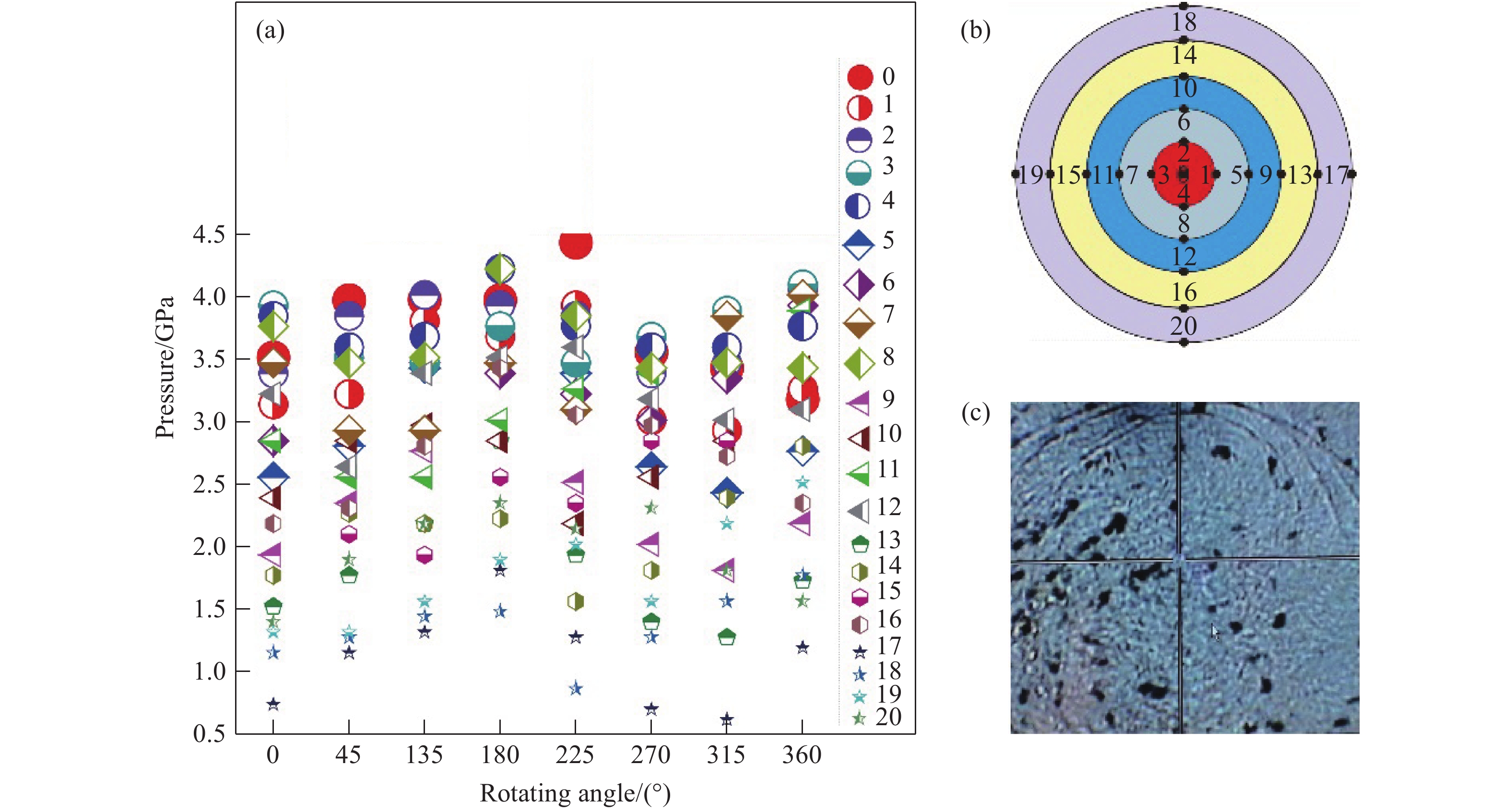
为了查明高压剪切作用下三水铝石结构转变的力学机制，对腔体内不同位置的压力进行了测试和统计。图7(a)为加压至3.5 GPa旋转剪切实验中腔体内的压力分布及测试点的数字和图形标识，图7(b)和图7(c)分别为测试点分布和腔体内样品的显微图。将直径为400 μm的腔体样品分为5个环形测试区（不同颜色圆环），在十字叉中心和每个环形区的对称方位各选取2个测试点（圆环中的数字），每次加压或旋转后测试21个不同位置金刚石和样品的拉曼光谱。图7中腔体内样品图片和压力分布显示：(1) 3.5 GPa下旋转剪切实验过程中，腔体内的压力分布极不均衡，中心区压力普遍高于边缘区；(2) 压机旋转过程中，直径为400 μm的腔体边缘出现压力最小值（0.5 GPa），腔体中心出现压力最大值（4.5 GPa）；(3) 在压机旋转前期（0～225°），腔体内压力整体上升，在旋转后期（225°～360°），压力有所回落，其中，后期压力回落原因可能与样品旋转带出、腔体内样品减少相关；(4) 压机旋转过程中，腔体样品在中间区域的空隙（图7(c)黑点）和边缘的褶皱（图7(c)右上和左上）表明腔体内样品发生了相对滑移。
图 7 高压剪切实验腔体内的压力分布和测试点的数字、图形标识(a)，测试点分布(b)，腔体内显微图片(c)Figure 7. (a) Pressure distribution of sample chamber under high pressure and shear stress, and marks of measurement point; (b) distribution of measurement point; (c) microscopic image of sample高压剪切作用下腔体内压力的不均匀分布表明，样品处于一个具有较大差应力的非静水压环境。在这样的环境下，三水铝石单斜结构发生了与准水压环境下完全不同的变化。此时，
$\gamma $ -Al(OH)3夹心饼干式(OH)-Al-(OH)配位八面体层骨架发生脱羟基，脱羟基后残余的O-Al-O多面体仍保持层间叠置。此时，层间距离、层与层间的相对位置以及羟基的周围环境都发生了明显改变。三水铝石高压新物相与初始$\gamma $ -Al(OH)3相比，具有完全不同的弹塑性和介电性，对板片俯冲的速度和深度存在较大影响。3. 结 论
区别于传统对称式金刚石对顶砧，旋转压机在轴向压缩的同时还可以实施垂直于轴的水平旋转，实现高压剪切作用。这种压机是模拟材料剪切受力环境、研究板块俯冲过程含水矿物结构稳定性的有效工具。本研究应用旋转压机对三水铝石
$\gamma $ -Al(OH)3开展了原位高压拉曼光谱和常压微区X射线衍射谱测试。实验发现：在高压剪切作用下三水铝石发生脱羟基作用生成H2O和${\rm{H}}_3{\rm{O}}_2^- $ ，残余的O-Al-OH配位多面体骨架保持c轴方向的层向叠置。这种结构改变与准静水压条件下发生的晶体结构微调相变完全不同，这是由于腔体内样品在高压下受到水平方向的强烈剪切作用。高压剪切作用下，三水铝石的结构稳定性研究为查明板块冷俯冲带中含水矿物的稳定性、推演俯冲板片的物理化学性质及其俯冲速率都具有重要意义。 -
图 3 CPFEM模拟的<100> LiF波剖面与实验的比对结果
Figure 3. Comparison of wave profiles of <100> LiF from CPFEM simulations and experiments
图 4 加载条件和样品厚度相同、样品掺杂浓度不同（LiF11～LiF13，掺杂浓度依次减小）时模拟的应力波剖面
Figure 4. Stress wave profiles from models with same loading condition, specimen thickness and different doping concentrations (LiF11–LiF13, with doping concentration decreasing in order)
图 5 采用Johnson-Cook本构方程对LiF01、LiF02模型的模拟结果
Figure 5. Simulation results of LiF01 and LiF02 models using Johnson-Cook constitutive equation
图 6 LiF03、LiF04、LiF05和LiF12双波和 单波响应的临界压力
Figure 6. Critical pressure of two-wave and one-wave response of LiF03–LiF05 and LiF12 models
图 7 模拟LiF03、LiF04、LiF05和LiF12在13.4 GPa压力下的双波响应
Figure 7. Two-wave response of LiF03–LiF05 and LiF12 models under 13.4 GPa pressure by simulation
图 8 模拟LiF03、LiF04、LiF05、LiF12在0～40 GPa 冲击压力范围内温度随压力的响应
Figure 8. Temperature responding to pressure ranging from 0 to 40 GPa of LiF03–LiF05 and LiF12 models by simulation
表 1 模拟中采用的模型信息
Table 1. Model information in simulations
Sample No. Flyer material Window material v/(m·s–1) Sample’s thickness/mm Ref. LiF01 Al Quartz 340.0 1.35 [6] LiF02 Al Quartz 340.0 1.98 [6] LiF03 LiF LiF 423.8 3.0 [17] LiF04 LiF LiF 1 321.6 3.0 [17] LiF05 LiF LiF 1 641.5 3.0 [17] LiF06 Fused sillica Fused sillica 340.9 1.143 [18] LiF11, LiF12, LiF13 LiF LiF 340.0 3.0 Note: LiF01–LiF06 models were built based on the parameters of specimens and experiments in references, while LiF11–
LiF13 models were designed for comparison of profile characteristic differences with successively increased specimen
doping concentration.
下载: 导出CSV
表 2 <100> LiF的超弹性本构参数
Table 2. Hyperelastic constitutive parameters of <100> LiF
Subscript Cij/GPa $\dfrac{{{\rm{d}}{{C}_{ij}}}}{{{\rm{d}}{p}}}$ $\dfrac{{{\rm{d}}{{C}_{ij}}}}{{{\rm{d}}{T}}}/(\rm MPa \cdot K^{-1})$ K0/GPa $ K_0^{\prime}$ ρ0/(g·cm–3) cV/(J·kg–1·K–1) Γm 11 113.97 9.97 –75.56 69.97 4.43 2.64 1 612.02 1.68 12 47.67 2.73 –28.39 44 63.64 1.38 –13.94
下载: 导出CSV
表 3 <100> LiF的晶体塑性本构参数
Table 3. Crystal plasticity constitutive parameters of <100> LiF
Sample No. τ0/MPa B n ${\dot \gamma _{{\rm{off}}}}$/μs–1 ${\dot \gamma _{\rm{0}}}$/μs–1 m λ $\dfrac{{{\tau _{\rm T}}}}{\theta}/{\rm μs}^{-1}$ LiF01, LiF02, LiF11 121.0 4.0 0.08 3.5×10–9 0.12 0.09 4.7×10–2 0.15 LiF03, LiF04, LiF05, LiF12 113.9 0.30 LiF06, LiF13 86.4 0.40
下载: 导出CSV
-
[1] 王礼立, 胡时胜, 杨黎明, 等. 材料动力学 [M]. 合肥:中国科学技术大学出版社, 2017: 7-9, 111-113. [2] 经福谦. 实验物态方程导引 [M]. 2版. 北京: 科学出版社, 1999: 7-26. [3] BECKER R. Effects of crystal plasticity on materials loaded at high pressures and strain rates [J]. International Journal of Plasticity, 2004, 20(11): 1983–2006. doi: 10.1016/j.ijplas.2003.09.002 [4] DE S, ZAMIRI A R. A fully anisotropic single crystal model for high strain rate loading conditions with an application to α-RDX [J]. Journal of the Mechanics and Physics of Solids, 2014, 64: 287–301. doi: 10.1016/j.jmps.2013.10.012 [5] GILMAN J J. Plastic anisotropy of LiF and other rocksalt-type crystals [J]. Acta Metallurgica, 1959, 7(9): 608–613. doi: 10.1016/0001-6160(59)90130-0 [6] ASAY J R, FOWLES G R, DURALL G E, et al. Effects of point defects on elastic precursor decay in LiF [J]. Journal of Applied Physics, 1972, 43(5): 2132–2145. doi: 10.1063/1.1661464 [7] ROSENBERG G, DUVALL G E. Precursor amplitudes in LiF from shocks propagating in <111> directions [J]. Journal of Applied Physics, 1980, 51(1): 319–330. doi: 10.1063/1.327375 [8] WINEY J M, GUPTA Y M. Nonlinear anisotropic description for the thermomechanical response of shocked single crystals: inelastic deformation [J]. Journal of Applied Physics, 2006, 99(2): 023510. doi: 10.1063/1.2161414 [9] ASARO R J. Micromechanics of crystals and polycrystals [J]. Advances in Applied Mechanics, 1983, 23(8): 1–115. [10] HOGER A. The stress conjugate to logarithmic strain [J]. International Journal of Solids and Structures, 1987, 23(12): 1645–1656. doi: 10.1016/0020-7683(87)90115-6 [11] 黄克智. 固体本构关系 [M]. 北京: 清华大学出版社, 1999: 205-208. [12] LEMAITRE J, CHABOCHE J L. Mechanics of solids materials [M]. Cambrige: Cambridge University Press, 1990: 311-313. [13] ASARO R J, RICE J R. Strain localization in ductile single crystals [J]. Journal of the Mechanics and Physics of Solids, 1977, 25(5): 309–338. doi: 10.1016/0022-5096(77)90001-1 [14] KUMAR A, HAUSER F E, DORN J E. Viscous drag on dislocations in aluminum at high strain rates [J]. Acta Metallurgica, 1968, 16(9): 1189–1197. doi: 10.1016/0001-6160(68)90054-0 [15] STEINBERG D J, COCHRAN S G, GUINAN M W. A constitutive model for metals applicable at high-strain rate [J]. Journal of Applied Physics, 1980, 51(3): 1498–1504. doi: 10.1063/1.327799 [16] NEMAT-NASSER S, GUO W G, KIHL D P. Thermomechanical response of AL-6XN stainless steel over a wide range of strain rates and temperatures [J]. Journal of the Mechanics and Physics of Solids, 2001, 49(8): 1823–1846. doi: 10.1016/S0022-5096(00)00069-7 [17] 李雪梅, 俞宇颖, 张林, 等. <100>LiF的低压冲击响应和1 550 nm波长下的窗口速度修正 [J]. 物理学报, 2012, 61(15): 156202 doi: 10.7498/aps.61.156202LI X M, YU Y Y, ZHANG L, et al. Elastic-plastic response of shocked (100) LiF and its window correction at 1 550 nm wavelength [J]. Acta Physica Sinica, 2012, 61(15): 156202 doi: 10.7498/aps.61.156202 [18] ASAY J R, HICKS D L, HOLDRIDGE D B. Comparison of experimental and calculated elastic-plastic wave profiles in LiF [J]. Journal of Applied Physics, 1975, 46(10): 4316–4322. doi: 10.1063/1.321454 [19] MILLER R A, SMITH C S. Pressure derivatives of the elastic constants of LiF and NaF [J]. Journal of Physics and Chemistry of Solids, 1964, 25(12): 1279–1292. doi: 10.1016/0022-3697(64)90043-5 [20] 于锦泉, 肖亚斌, 周显明, 等. 冲击压缩下单晶 LiF 高压声速及卸载路径研究 [J]. 高压物理学报, 2005, 19(3): 206–212 doi: 10.3969/j.issn.1000-5773.2005.03.003YU J Q, XIAO Y B, ZHOU X M, et al. Study on sound velocity and unloading path for single crystal LiF under shock compression [J]. Chinese Journal of High Pressure Physics, 2005, 19(3): 206–212 doi: 10.3969/j.issn.1000-5773.2005.03.003 [21] ANDERSSON S, BACKSTROM G. Thermal conductivity and heat capacity of single-crystal LiF and CaF2 under hydrostatic pressure [J]. Journal of Physics C: Solid State Physics, 1987, 20(35): 5951. doi: 10.1088/0022-3719/20/35/011 [22] NELDER J A, MEAD R. A simplex method for function minimization [J]. The Computer Journal, 1965, 7(4): 308–313. doi: 10.1093/comjnl/7.4.308 [23] YAO S L, PEI X Y, YU J D, et al. A dislocation-based explanation of quasi-elastic release in shock-loaded aluminum [J]. Journal of Applied Physics, 2017, 121(3): 035101. doi: 10.1063/1.4974055 [24] NEWTON M D, O'KEEFFE M, GIBBS G V. Ab initio calculation of interatomic force constants in H6Si2O7 and the bulk modulus of α quartz and α cristobalite [J]. Physics and Chemistry of Minerals, 1980, 6(4): 305–312. doi: 10.1007/BF00307620 [25] GRAHAM R A. Shock-wave compression of x-cut quartz as determined by electrical response measurements [J]. Journal of Physics and Chemistry of Solids, 1974, 35(3): 355–372. doi: 10.1016/S0022-3697(74)80029-6 [26] RAMMO N N, FARID S B. Thermal expansion coefficients and Grüneisen parameters of quartz at high temperature by X-ray method [J]. Powder Diffraction, 1994, 9(2): 148–150. doi: 10.1017/S0885715600014147 [27] MEADE C, JEANLOZ R. Frequency-dependent equation of state of fused silica to 10 GPa [J]. Physical Review B, 1987, 35(1): 236. doi: 10.1103/PhysRevB.35.236 [28] BOGARDUS E H. Third-order elastic constants of Ge, MgO, and fused SiO2 [J]. Journal of Applied Physics, 1965, 36(8): 2504–2513. doi: 10.1063/1.1714520 [29] JOHNSON J N, JONES O E, MICHAELS T E. Dislocation dynamics and single-crystal constitutive relations: shock-wave propagation and precursor decay [J]. Journal of Applied Physics, 1970, 41(6): 2330–2339. doi: 10.1063/1.1659227 [30] DING J L, ASAY J R, AO T. Modeling of the elastic precursor behavior and dynamic inelasticity of tantalum under ramp wave loading to 17 GPa [J]. Journal of Applied Physics, 2010, 107(8): 083508. doi: 10.1063/1.3373388 -
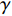
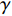
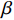
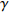
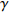
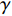
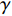
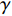
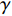
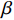
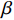
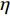
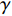
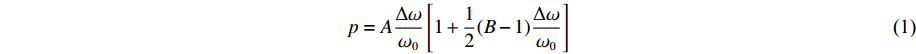
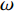
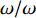
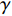
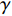
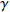
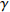
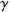
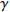
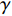
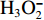
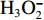
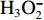
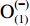
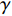
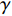
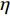
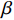
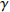
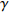
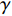
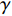
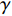
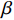
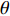
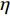
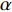
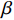
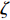
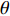
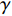
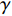
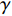
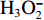
下载:
下载:
