钢筋混凝土结构在建筑、桥梁工程中有广泛的应用。混凝土作为非弹性材料，在动态和静态加载条件下会表现出不同的力学特性，且存在明显的加载率效应。国内外许多研究表明，钢筋混凝土结构在高应变率加载条件下具有较高的变形抗力，结构的失效模式也会发生相应的变化。例如，Ožbolt等^[1]将率相关微平面模型引入3D有限元模型中，分析了加载速率对锚杆拔出时混凝土失效模式的影响。Travaš等^[2]模拟了素混凝土梁在准静态和动态加载下的结构响应，发现准静态和低速冲击(v=2 m/s)下梁发生弯曲破坏；当冲击速度大于2 m/s时，梁的破坏模式由弯曲破坏转为剪切破坏。付应乾等^[3]对钢筋混凝土梁进行三点弯曲试验，探讨了不同冲击高度下钢筋混凝土结构的破坏机理。Saatci等^[4]对钢筋混凝土梁进行不同质量锤头的落锤冲击试验，发现梁可承载的冲击力随抗剪强度的升高而增大。展婷变等^[5]对钢筋混凝土梁进行落锤试验，分析了冲击速率对最大承载力和梁变形的影响。Zineddin等^[6]对钢筋混凝土板进行不同高度的冲击试验，发现高速冲击下配筋率对于改善混凝土结构的层裂破坏有较大作用，但对最大承载力影响较小。范向前等^[7]开展了非标准钢筋混凝土三点弯曲梁试件断裂试验，认为梁能够承载的最大载荷由配筋情况决定。沈培峰^[8]研究了配筋率对钢筋混凝土梁断裂性能的影响以及钢筋在裂缝扩展过程中的限裂作用。落锤试验^[9-12]是目前研究钢筋混凝土梁在低速冲击下破坏的有效手段。由于试件制备过程中浇筑技术和温度等外在条件影响，混凝土的力学性能具有一定的离散性。另一方面，实验过程中数据采集和加载条件的控制难免存在误差，因此最终实验结果的可靠性降低。

随着计算机软件的开发和有限元技术的不断成熟，数值仿真计算为实验结果的分析提供了有效的参考依据。李敏等^[13]模拟了地震作用范围内加载速率对钢筋混凝土梁影响，姜华等^[14]使用弹塑性断裂带模型(EPDC)模拟了钢筋混凝土梁在动态冲击下的结构响应。有限元建模存在本构模型不同、网格划分精细程度不一等差别，与实验结果进行对比后可验证其有效性。本研究基于ABAQUS有限元软件，模拟并分析了钢筋混凝土梁在不同高度冲击下的动态响应。通过模拟与实验结果对比，验证了模型的有效性，并讨论了不同冲击速度下，配筋率对钢筋混凝土梁承载力、抗变形能力和破坏模式的影响。

1.   有限元模型

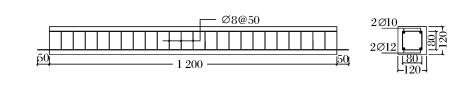
付应乾等^[3]利用落锤对钢筋混凝土梁进行三点弯曲试验，探讨了不同冲击高度下混凝土结构的破坏机理。本研究对该实验进行有限元建模，所用模型尺寸与参考文献[3]一致，如图 1所示。混凝土设计强度为C30，实验用梁长1.2 m，截面尺寸为120 mm×120 mm，纵向钢筋采用HRB335，箍筋采用HPB235，保护层厚度为20 mm。

图  1  梁尺寸及配筋截面图(单位：mm)

Figure  1.  Beam size and reinforcement section (Unit: mm)

下载: 全尺寸图片 幻灯片

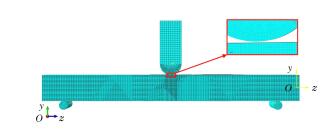
模型中通过减小锤头的几何尺寸和增大密度来保持冲击能量不变。支座密度为7 800 kg/m³，弹性模量为200 GPa，泊松比为0.3。为减少计算时间，根据$v = \sqrt {2gh}$ (1g=9.8 m/s²)，落锤下落高度1.2、2.4和4.8 m，在预定义场中将初始速度分别设为4.85、6.86和9.60 m/s。对锤头约束在只有y方向存在位移，锤头与混凝土梁上表面接触为通用接触。支座设置为在x方向上(即混凝土梁纵向)存在位移。锤头与混凝土梁上表面初始距离为1 mm。有限元模型如图 2所示。模型中混凝土采用CDP(Crack Damage Plastic)模型，参数^[15]如表 1所示。混凝土采用三维8节点缩减积分单元(C3D8R)，网格尺寸为1.2 mm×1.2 mm×1.2 mm。

图  2  有限元模型

Figure  2.  Finite element model

下载: 全尺寸图片 幻灯片

表  1  混凝土模型参数^[15]

Table  1.  Parameters of concrete^[15]

Dilation angle/(°)	Eccentricity	fb0/fc0	K	Viscosityparameter	Density/(kg·m-3)	E/GPa	ν
30	0.1	1.16	0.666 7	0.000 5	2 400	26.48	0.167

下载: 导出CSV 

| 显示表格

钢筋采用三折线模型，有限元模型中网格单元为三维8节点缩减积分单元(C3D8R)，网格尺寸为1.2 mm×1.2 mm×1.2 mm，以Embed形式嵌入混凝土基体中。模型中不考虑钢筋与混凝土之间的握裹力和拉拔效应。试验表明^[16]，高应变率作用下建筑钢筋的弹性模量E保持不变，钢筋HPB235的弹性模量为210 GPa，HRB335和HRB400的弹性模量为200 GPa。钢筋的屈服强度和极限强度随应变率的增大而提高，其变化规律可表示为

式中: ${\dot \varepsilon }$为钢筋的应变率，${{{\dot \varepsilon }_0}}$=0.000 3 s^－1，f_{y, d}和f_{y, st}分别为钢筋动态和静态加载下屈服强度，f_{u, d}和f_{u, st}分别为钢筋动态和静态加载下极限强度。

林峰等^[16]通过实验，得到HPB235和HRB335的D₁和D₂分别为10.05、8.73和6.38、6.54。以三折线建筑钢筋模型为例，其应力-应变关系可表示为

式中: ε_{b, d}和ε_{e, d}分别为屈服平台开始和结束时相应的应变，ε_{b, d}=f_{y, d}/E，ε_{e, d}=ε_{b, d}+(ε_{e, st}－ε_{b, st})；ε_{u, d}为极限强度对应的应变，ε_{u, d}=ε_{u, st}。

钢筋HRB335选用${\dot \varepsilon }$=2.9 s^－1时的屈服强度和极限强度，HPB235选用${\dot \varepsilon }$=6.5 s^－1时的屈服强度和极限强度，见表 2。

表  2  钢筋参数^[16]

Table  2.  Parameter of steel model^[16]

Reinforced grades	Diameter/mm	ρ/(kg·m-3)	E/GPa	ν	Yield strength/MPa	Ultimate strength/MPa
HPB235	8	7 862	210	0.3	400	540
HRB335	10	7 860	200	0.3	438	687
HRB335	12	7 850	200	0.3	438	687

下载: 导出CSV 

| 显示表格

2.   有限元模型验证

将模拟得到的冲击力、跨中挠度的最大值与实验结果^[3]进行对比，如表 3所示。不同冲击速度下得到的冲击力和跨中挠度最大值与实验结果吻合较好，且略大于实验值。该现象的原因是实验中梁两端会架设钢板来保持其在冲击过程中不会产生太大位移，而有限元模型中出于简化模型没有建立这些约束条件。另一方面，下落过程中锤头与下滑轨道存在摩擦，导致锤头与混凝土梁上表面接触时的瞬时速度相比计算值较小。这两个方面最终导致实验结果比模拟结果偏小。

表  3  模拟与实验结果对比

Table  3.  Comparison of results between FEM and experiment

Method	Drop height (m)/Mass (kg)	Impact velocity/(m·s-1)	Maximum impact force/kN	Maximum deflection of mid-point/mm
Experiment	1.2/124		104.0	28.3
FEM	-/124	4.85	149.1	30.7
Experiment	2.4/124		94.7	51.2
FEM	-/124	6.86	134.7	56.5
Experiment	4.8/124		173.9	90.4
FEM	-/124	9.60	181.2	102.9

下载: 导出CSV 

| 显示表格

随着冲击速度的提高，钢筋在实验中的屈服强度和极限强度与有限元模型中的值接近，冲击力峰值误差逐渐减小。实验中通过激光位移传感器测量锤头位移，默认锤头位移与梁跨中挠度值相同，模拟中提取梁上表面中心点的位移时程曲线。实际工况中锤头位移达到最大值后锤头开始反弹，梁具有少量动能继续向下运动，跨中挠度继续增大，实验测得的锤头位移最大值比梁跨中挠度最大值偏小。随着冲击高度的增加，锤头反弹时梁具有的动能相对增大，跨中挠度增大值相对增大，导致模拟与实验的误差增大。

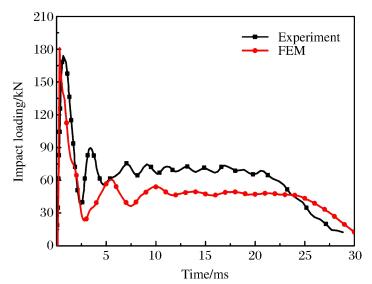
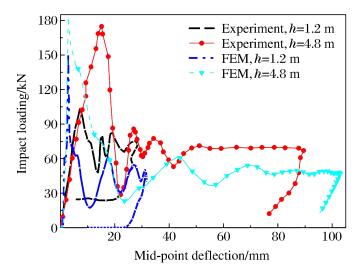
冲击高度为4.8 m(即v=9.60 m/s)时的冲击力模拟与实验结果对比如图 3所示。由图 3可知，模拟得到的冲击力时程曲线与实验结果变化趋势基本一致，峰值分别为181.2和173.9 kN，模拟值比实验值大4.2%，该误差在允许范围内。

图  3  冲击速度为9.60 m/s时的冲击力时程曲线

Figure  3.  Time history curves of the impact forces at v=9.60 m/s

下载: 全尺寸图片 幻灯片

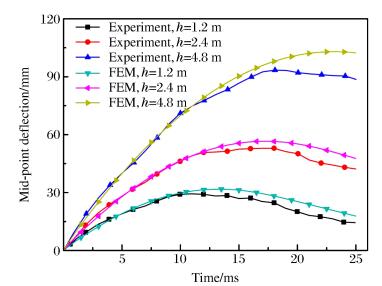
图 4为不同冲击速度下跨中挠度时程曲线的模拟与实验结果对比。由图 4可知，随着冲击速度的提高，跨中挠度不断增大。当第一次冲击结束时，锤头与混凝土梁上表面分离，梁出现轻微回弹效应，跨中挠度达到极值后开始减小。由表 3可知，模拟得到的最大值比实验值偏大，分别增大8.5%、10.3%和13.8%，该结果是模拟中冲击速度比实际值偏大导致的。

图  4  不同冲击速度下的跨中挠度曲线

Figure  4.  Time history curves of the mid-point deflection at different impact velocities

下载: 全尺寸图片 幻灯片

由图 3和4可知，本研究模型可以很好地模拟出不同冲击速度下钢筋混凝土梁的结构响应。选取v=4.85 m/s和v=9.60 m/s时的冲击力-跨中挠度曲线与实验结果进行对比，如图 5所示。模拟与实验得到的曲线变化趋势相同，冲击力在锤头和垫块接触后迅速增大，随后冲击能量减小，一部分能量用于梁的破坏，另一部分被梁的惯性消耗。当冲击速度为4.85 m/s时，冲击力随跨中挠度的增大呈振荡发展，梁的破坏模式是弯曲失效；当冲击速度为9.60 m/s时，在跨中点形成塑性铰后，冲击力由振荡变化逐渐趋向稳定，梁的破坏模式由弯曲失效转变为局部剪切失效。

图  5  不同冲击速度下冲击力-跨中挠度曲线

Figure  5.  Time history curves of impact relative to mid-point deflection at different velocities

下载: 全尺寸图片 幻灯片

图 5中h=4.8 m时冲击力峰值的模拟值和实验值分别为173.9和181.2 kN，误差为4.20%。h=1.2 m时冲击力峰值的模拟值与实验值分别为149.1和104.0 kN，误差为43.36%。钢筋应变率随冲击高度的增大而增大，其屈服强度和极限强度均有所增大，且差别较小。有限元模型参考林峰等^[16]的实验，钢筋HRB335选用${\dot \varepsilon }$=2.9 s^-1时的屈服强度和极限强度，HPB235选用${\dot \varepsilon }$=6.5 s^-1时的屈服强度和极限强度，见表 2。h=1.2 m时实验中钢筋的屈服强度和极限强度小于模型中的值，导致模型中梁的承载力较高，冲击力峰值误差增大。

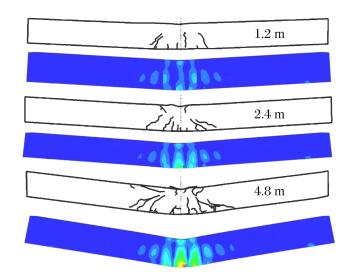
实验中通过高速摄影记录了钢筋混凝土梁在不同冲击速度下的变形、损伤及破坏状况。图 6表示不同冲击高度下跨中挠度达到最大值时梁表面的损伤破坏情况，简图为对高速摄像记录的照片处理后的裂纹分布图，其下方分别是相对应冲击速度下梁的塑性应变云图。由图 6可知，随着冲击速度的提高，梁的挠度不断增大。冲击速度较低时，裂纹从梁下表面中心处产生且数量较多，此时梁发生弯曲破坏；冲击速度增大时，在跨中发生局部剪切破坏，梁的破坏模式发生改变。

图  6  梁的损伤破坏状况

Figure  6.  Damage and failure of beams at different falling heights

下载: 全尺寸图片 幻灯片

3.   配筋率对梁承载力和破坏模式的影响

配筋率是钢筋混凝土构件中纵向受力钢筋面积与构件的有效面积之比，在桥梁工程中，一般指面积配筋率，即受拉钢筋面积与主梁面积之比。计算公式为P=A_s/A，其中A_s为纵向受力钢筋截面面积，A为主梁面积。上述实验中配筋率为2.66%，根据建筑工程钢筋配筋率规范^[17]可知，混凝土强度为30 MPa，纵向受力钢筋为HRB335时，最大配筋率为2.62%。

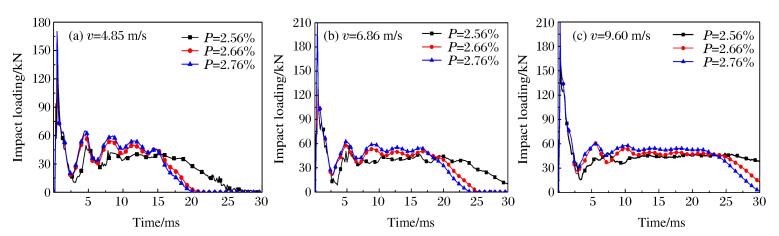
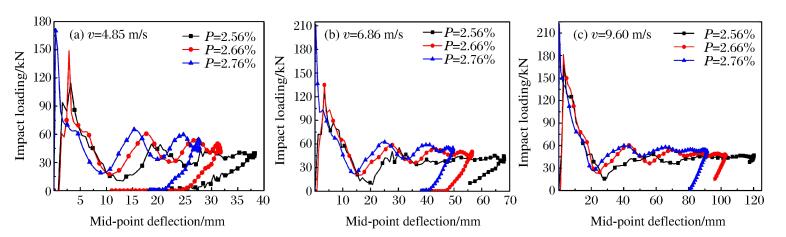
基于本研究模型，通过改变单根钢筋直径得到配筋率为2.56%、2.66%和2.76%的梁在不同冲击速度下的冲击力时程曲线，如图 7所示，表 4为冲击力峰值结果。由图 7可知，不同配筋率下冲击力变化趋势相同，最大冲击力随着配筋率的增大而增大，且冲击力达到峰值的时间随配筋率的增大而减小。配筋率的增大，显著地提高了梁的承载能力。表 4中百分比代表配筋率为2.66%和2.76%的梁的冲击力相比配筋率为2.56%的增大百分数。当冲击速度为4.85 m/s时，以配筋率2.56%作为基准，其增大3.9%(P=2.66%)时，梁的承载力最大值增大30%；增大7.8%(P=2.76%)时，梁的承载力最大值增大49%。冲击速度为6.86 m/s时，配筋率增大3.9%和7.8%时梁的承载力最大值分别增大7.2%和60.8%。冲击速度为9.60 m/s时，配筋率增大3.9%和7.8%时梁的承载力最大值分别增大7.1%和31.3%。低速冲击下，梁的失效模式主要为弯曲破坏，承载能力随配筋率的增大显著提高。随着冲击速度的增大，梁的失效模式由弯曲破坏转向剪切破坏，钢筋对于提高混凝土梁承载力的效果逐渐减小。

图  7  不同配筋率梁在不同冲击速度下的冲击力时程曲线

Figure  7.  Time history curves of impact force for reinforced beams with different reinforcement ratios at different impact velocities

下载: 全尺寸图片 幻灯片

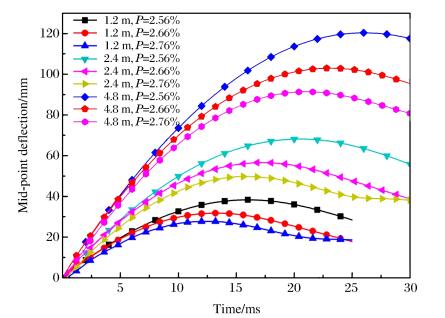
表  4  冲击力峰值结果对比

Table  4.  Comparison of the results of maximum impact force

Impact velocity/(m·s-1)	Reinforcement ratio:2.56%			Reinforcement ratio:2.66%			Reinforcement ratio:2.76%
	Max. impact force/kN	Time/ms		Max. impact force/kN	Time/ms		Max. impact force/kN	Time/ms
4.85	114	0.66		149(+30.0%)	0.6		170(+49.0%)	0.5
6.86	125	0.50		134(+7.2%)	0.5		201(+60.8%)	0.4
9.60	169	0.33		181(+7.1%)	0.3		222(+31.3%)	0.3

下载: 导出CSV 

| 显示表格

不同配筋率梁在不同冲击速度下的跨中挠度时程曲线和具体数值如图 8和表 5所示。同一冲击速度下，梁的抗变形能力随配筋率的增大而提高，变形相对较小，跨中挠度达到最大值的时间随配筋率的增大而减小。表 5中百分数表示配筋率为2.66%和2.76%的梁跨中挠度相对配筋率为2.56%的减小百分比。冲击速度大于6.86 m/s时，配筋率对梁抗变形能力的增强效果随冲击速度的增大而逐渐减弱。

图  8  不同配筋率梁在不同冲击速度下的跨中挠度时程曲线

Figure  8.  Deflection time curves of beams with different reinforcement ratios at different impact velocities

下载: 全尺寸图片 幻灯片

表  5  跨中位移峰值结果对比

Table  5.  Comparison of results of maximum mid-point deflection

Impact velocity/(m·s-1)	Reinforcement ratio:2.56%			Reinforcement ratio:2.66%			Reinforcement ratio:2.76%
	Max. deflection/mm	Time/ms		Max. deflection/mm	Time/ms		Max. deflection/mm	Time/ms
4.85	38.26	16.0		31.72(-17.10%)	13.32		27.70(-27.60%)	12.3
6.86	68.15	25.5		56.56(-17.00%)	17.30		49.73(-27.03%)	15.6
9.60	120.33	26.0		102.94(-14.43%)	25.00		91.37(-24.07%)	21.0

下载: 导出CSV 

| 显示表格

图 9为不同冲击速度下冲击力-跨中挠度曲线。由图 9可知，改变配筋率后，冲击力变化趋势一致。冲击速度为4.85 m/s时，不同配筋率梁的冲击力随跨中挠度增大呈振荡变化，梁的破坏模式为弯曲失效。冲击速度为6.86 m/s时，配筋率较小的梁冲击力在跨中挠度达到40 mm后逐渐稳定，梁的破坏模式由弯曲失效转为剪切失效，其他配筋率梁的冲击力随跨中挠度增大保持振荡变化，其发生弯曲破坏。冲击速度为9.60 m/s时，配筋率为2.56%和2.66%的梁发生剪切失效，配筋率为2.76%的梁发生弯曲失效。因此，随着配筋率的减小，梁在冲击过程中发生剪切破坏的可能性增大。

图  9  不同配筋率梁在不同冲击速度下的冲击力-跨中挠度曲线

Figure  9.  Impact loading-mid-point displacement curves for beams with different reinforcement ratios at different impact velocities

下载: 全尺寸图片 幻灯片

4.   结论

在钢筋的本构模型中引入应变率效应，对钢筋混凝土梁进行有限元建模。利用该模型模拟了在不同冲击高度下梁的结构响应，通过对比实验和模拟的冲击力、跨中挠度时程曲线和峰值，验证了模型的有效性。基于该模型模拟了不同配筋率钢筋混凝土梁在不同冲击速度下的结构响应，得到以下结论：

(1) 不同冲击速度下，配筋率的增大提高了梁的承载力，当冲击速度为4.85 m/s时，增大配筋率对梁承载力的增强效果更为明显；

(2) 冲击速度一定时，梁的抗变形能力随配筋率的增大而提高，当冲击速度较大时，随着冲击速度的增大，配筋率对梁抗变形能力的增强效果逐渐减小；

(3) 低速冲击下，配筋率对梁的破坏模式影响微弱，当冲击速度较大时，随着配筋率的增大，梁的破坏模式由剪切破坏转变为弯曲破坏。