Thermodynamic Properties of Mg2X (X=Si, Ge) Phases under Pressure by First-Principles Calculations
-
摘要: 采用基于密度泛函理论的第一性原理方法,研究了压力作用下Mg2Si和Mg2Ge的结构、弹性和热力学性质。计算结果表明:0 GPa压力作用下两者的晶格参数与实验值以及其他理论值吻合较好,且相对晶格常数a/a0和晶胞体积V/V0均随压力的增大而减小;在0~25 GPa压力作用下,Mg2Si和Mg2Ge相体模量B、剪切模量G、杨氏模量E均随压力的增大而增大,材料的刚度和塑性均增强,当压力达到15 GPa时,材料由脆性转变为延性。最后借助准谐德拜模型和Gibbs软件,研究了温度与压力对Mg2Si和Mg2Ge的德拜温度、体模量、热容和热膨胀系数的影响。Abstract: The structural, elastic and thermodynamic properties of Mg2Si and Mg2Ge phases under pressure were calculated using the first-principles based on the density functional.The calculated results indicated that the lattice parameters under 0 GPa are fairly consistent with the experimental value and other theoretical data.The ratio of a/a0 and V/V0 decreased as the external pressure increases.An appropriate pressure (0-25 GPa) can improve their stiffness and plasticity because the bulk modulus B, shear modulus G, and Young's modulus E almost linearly increase with pressure.The brittleness of the material turns to ductility at 15 GPa.Finally, the effect of temperature and pressure on the Debye temperature, bulk modulus, heat capacity and linear thermal expansion coefficient was studied using the quasi-harmonic Debye model and Gibbs software.
-
Key words:
- first-principles /
- Mg2Si /
- Mg2Ge /
- structural property /
- elastic property /
- thermodynamic property
-
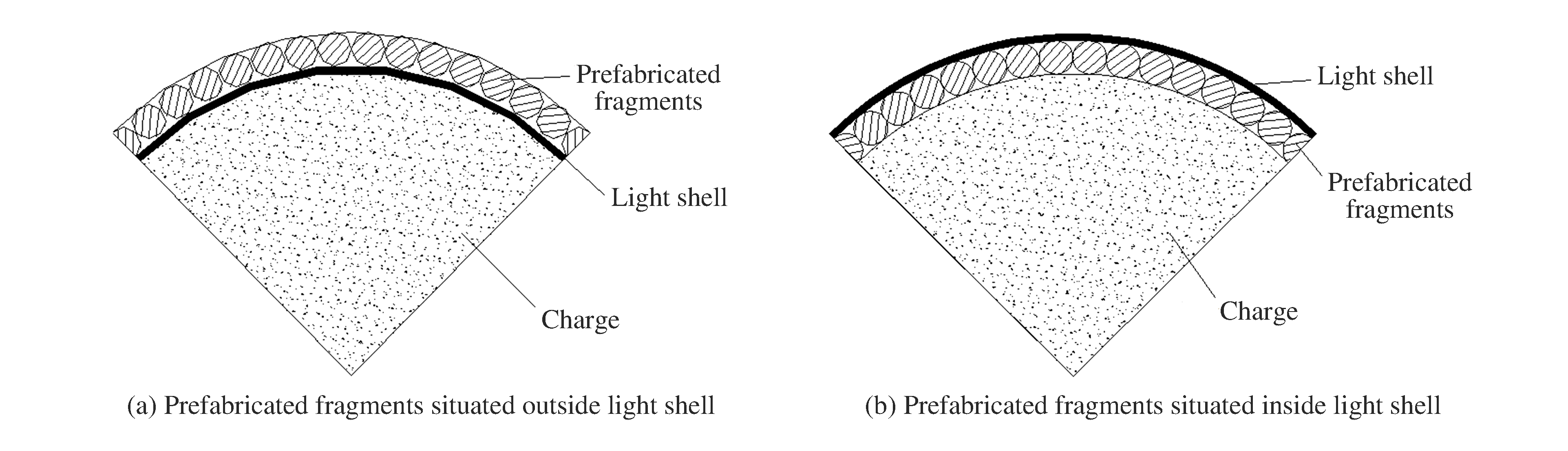
全预制破片类杀伤战斗部的轻质壳体常采用高强度铝合金加工而成,主要用于提高战斗部结构强度、控制战斗部外形等,同时也便于装药过程的实施,而预制破片可布置于壳体内侧或外侧[1]。由于壳体材料与破片材料(如低碳钢、钨合金等)的阻抗存在较大差异,在爆炸加载过程中,不同的阻抗顺序将造成应力波的传播过程出现较大差别[2-3],可能导致破片的初速及完整性发生改变,进而影响战斗部的威力。因此,合理设计预制破片与轻质壳体的阻抗匹配顺序对于该类杀伤战斗部的优化设计具有重要意义。
采用数值模拟方法研究该问题时,轻质壳体在加载初期由于变形过大而被失效删除,常难以对比不同阻抗顺序造成的差异[4]。因此,最直接的方式是对两种工况下的破片速度进行实际测量,并回收破片进行完整性分析。由于常用的断通靶等电测方式难以直接获得破片初速,一些科研人员基于圆筒结构实验模型,采用更高精度的转镜式高速扫描法及激光干涉测速法获取了爆炸加载过程中不同金属破片的初速,并分析了金属材料与炸药的匹配性[5-6];但该方法不适用于干扰因素较多的预制破片工况,且无法观测破片加速过程中的形态。而脉冲X射线测量方法虽然可观测破片加速过程中的形态,但常由于破片飞散方向杂乱难以准确获得破片的初速。正是由于上述观测及实验方法的限制,导致目前很少见到预制破片与轻质壳体的阻抗匹配方面的报道。
本研究拟基于预制破片与轻质壳体的典型阻抗排布顺序,依据炸药装药爆轰加载释能特点,设计与杀伤战斗部爆轰加载过程较为相似的滑移爆轰单元结构实验模型(即采用滑移爆轰方式对“单条”破片进行加载),从而获得不同阻抗排布顺序下的破片初速及形态,并结合冲击波在不同介质间的传播规律,分析破片及壳体中的冲击波压力及粒子速度变化过程,以期能为相关杀伤战斗部的设计提供参考。
1. 爆轰驱动过程影响因素分析
图 1显示了杀伤战斗部全预制破片和轻质壳体的两种典型放置工况,即破片置于轻质壳体外侧和内侧。在爆轰加载过程中,破片外置时,爆轰产物首先冲击轻质壳体,使壳体中形成较强的冲击波,到达预制破片界面时,其冲击波主要由低阻抗介质向高阻抗介质传播;而破片内置时,冲击波则由高阻抗介质向低阻抗介质传播。因此,虽然这两种工况的装填比基本相同,但冲击波传播过程存在明显差异,进而可能影响破片的初速及变形。
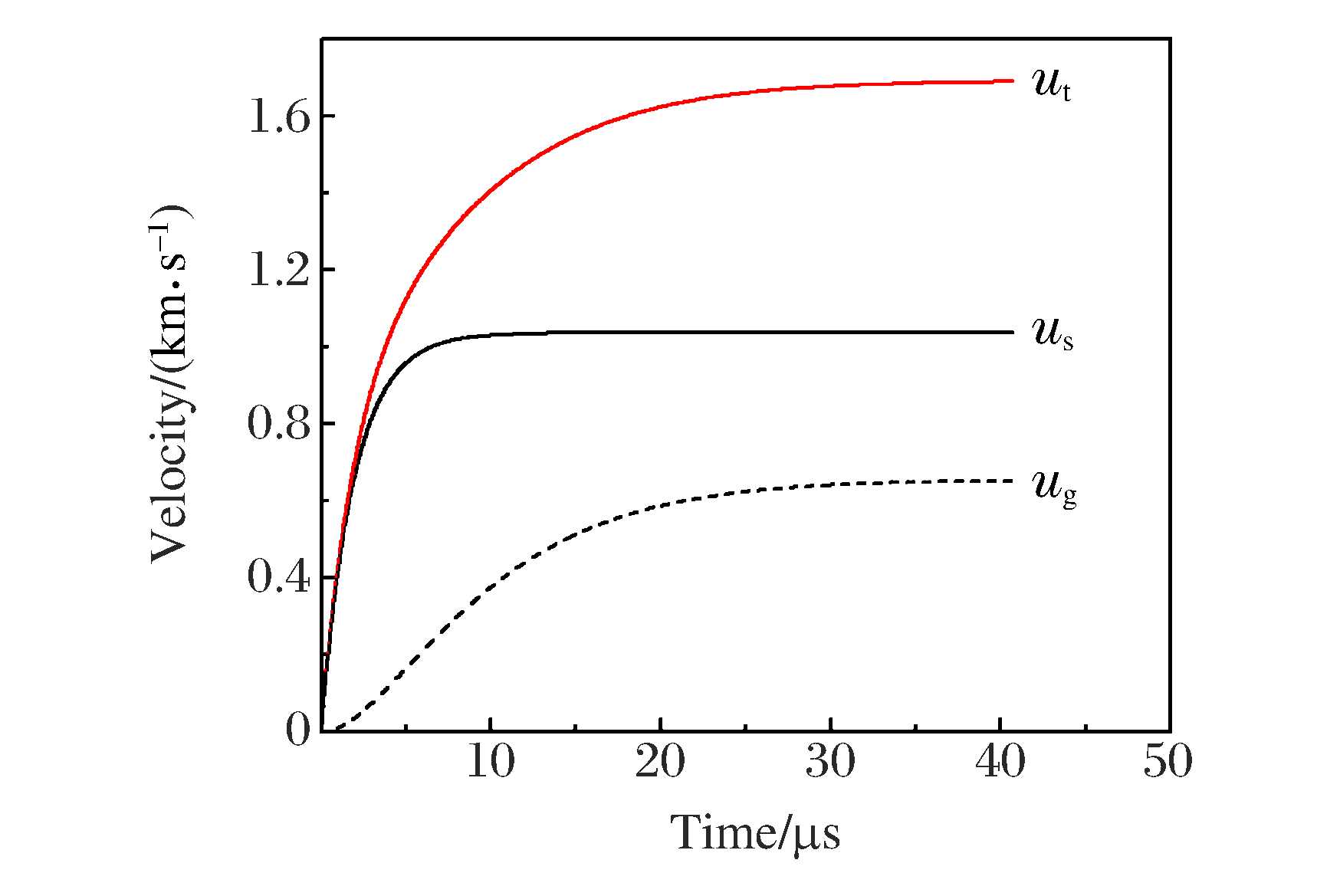
然而,炸药爆轰加载过程并不是持续的冲击波驱动作用,由于爆轰产物膨胀过程分为高、中、低压3个阶段,且对战斗部破片及壳体的有效加载阶段主要位于高压及中压阶段,因此,一些研究认为炸药装药对破片及壳体的爆轰加载主要依靠两种作用力的共同作用,即冲击波的驱动力和气体爆轰产物的膨胀力[7]。冲击波驱动力可以使破片及壳体在加载初期速度迅速提高,但持续时间较短;而气体爆轰产物膨胀推动力虽然峰值相对偏低,但持续时间较长。以B炸药滑移爆轰驱动∅50 mm圆筒(即标准圆筒试验)为例。图 2显示了圆筒壁的加速历程。为了将两种作用力的作用效果区分开,采用us表示冲击波驱动力单独加载下圆筒壁的速度,ug表示气体产物膨胀力单独加载下圆筒壁的速度,ut(ut=us+ug)为两种作用力共同加载下圆筒壁的速度。从图 2中可以看出,整个加载过程可以明显地划分为两个阶段,即冲击碰撞加速阶段和气体产物膨胀力驱动加速阶段[7-8]。由于气体产物的膨胀力相对偏低,可假定在破片外置和内置两种工况下该阶段的加速效果差异较小,其差异主要在冲击碰撞加速阶段,因此可通过相应的单元实验对该阶段的加载规律进行分析。
2. 爆轰加载实验
2.1 实验原理
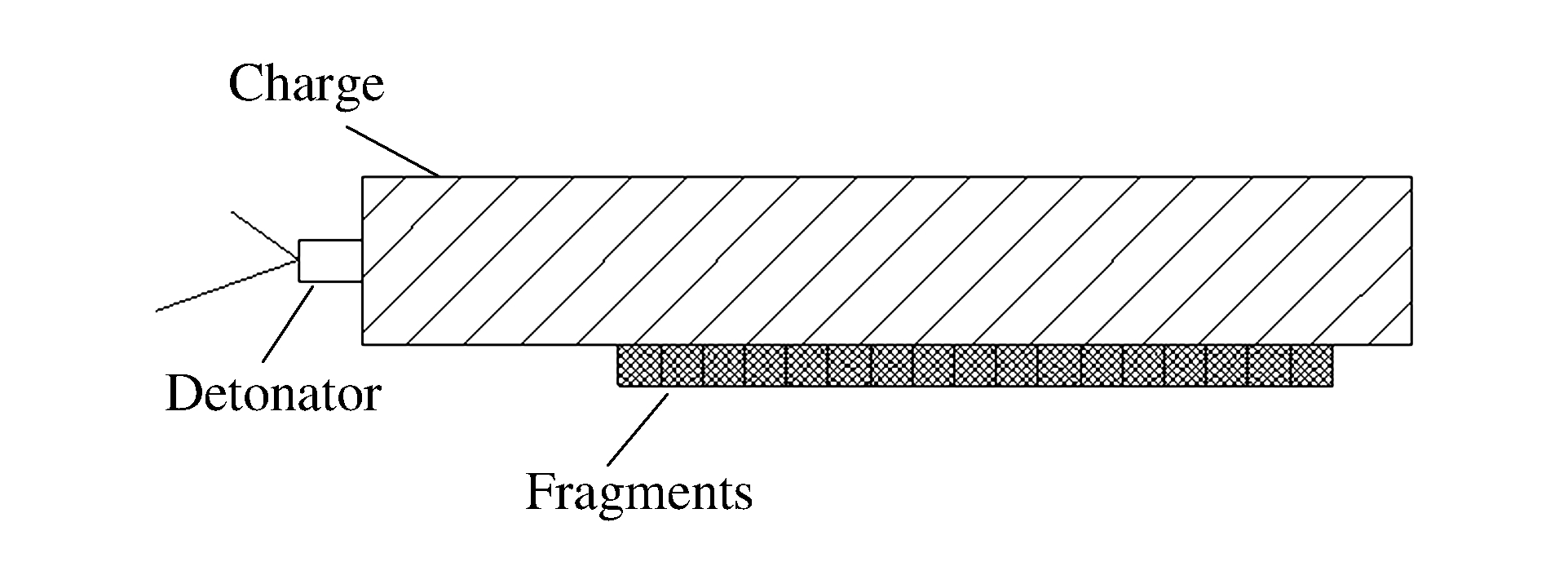
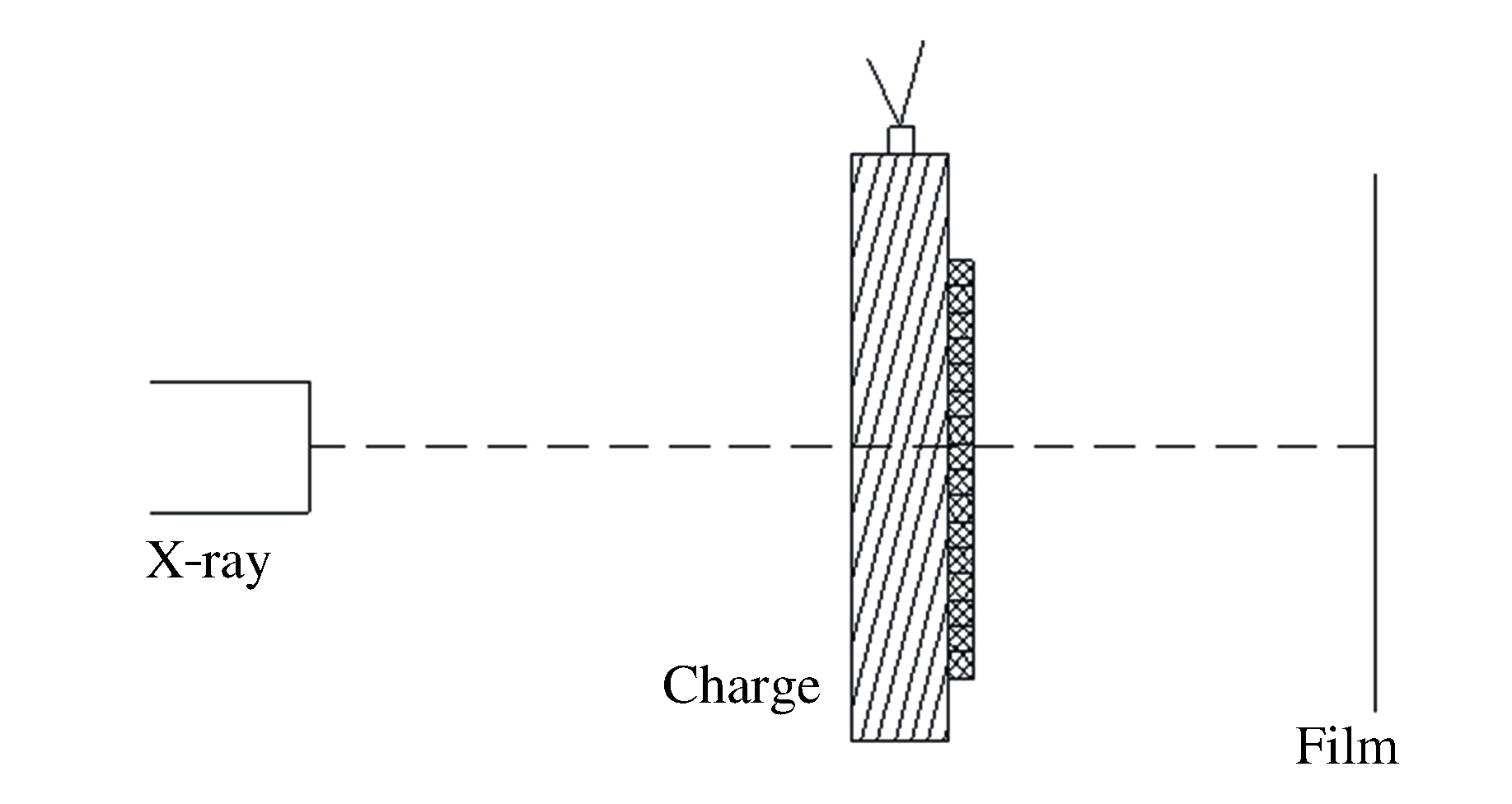
由于常用的断通靶测量方法在爆炸近场所受干扰因素较多,因此拟采用脉冲X射线系统对破片的分布及加速过程进行观测。从光学成像原理方面分析,脉冲X射线测量过程实际是将三维的实验模型投影到二维平面,因此,为了避免底片中的图像过于杂乱,只能对局部单位模块进行研究。为了尽可能地模拟实际战斗部的爆轰加载方式,采用滑移爆轰方式对“单条”破片进行加载,其中,起爆点为圆柱形装药一端面的中心处(如图 3所示),在柱形装药的中段,其径向冲量接近常量,则沿传爆方向的破片具有相同的加速度,且能够规则排列。此外,考虑到稀疏波的影响,破片距装药首、尾位置均空余一段距离,以保证破片所受爆炸冲量一致。这种加载方式对爆轰产物没有有效的约束,对破片的加载主要依靠冲击波驱动力作用。
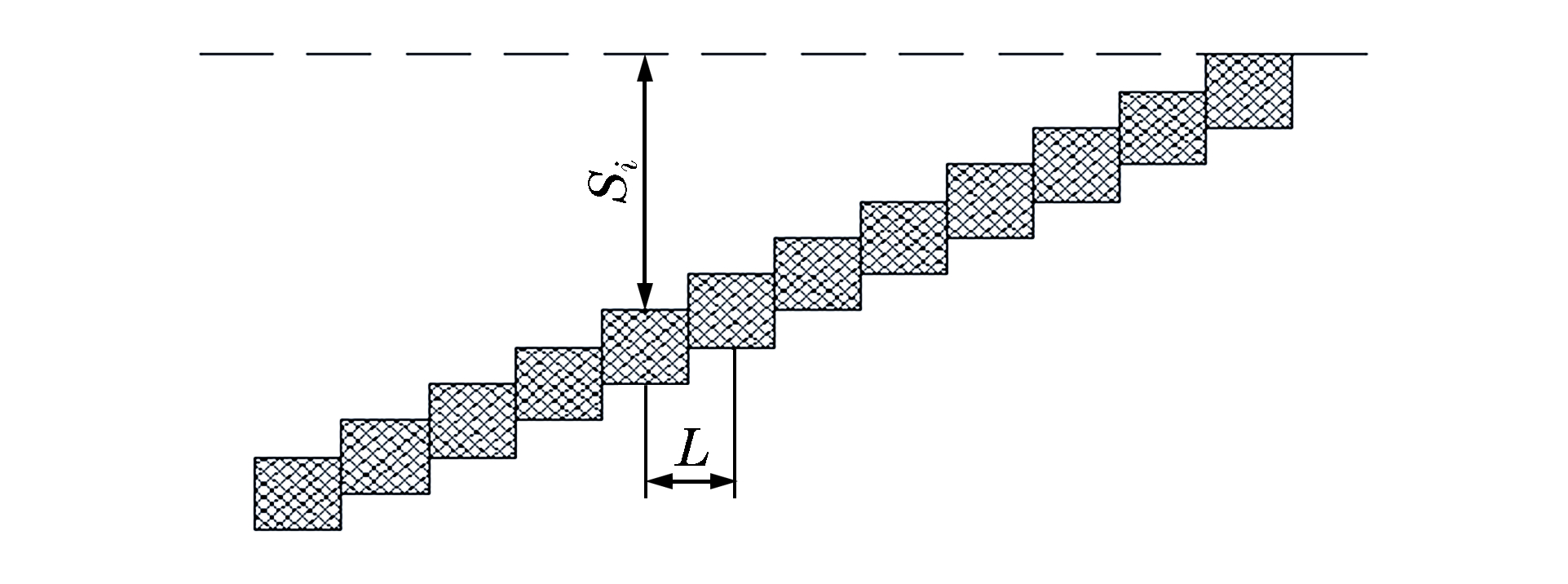
在滑移爆轰加载过程中,相邻破片运动的初始时间间隔固定,破片的飞散轨迹如图 4所示。根据X射线照片的判读结果可计算出各个破片的速度,其计算公式为
vi=ζ(Si−Si−1)ζL/D=Si−Si−1L/D (1) 式中:vi为第i个破片的速度,ζ为实物尺寸与图像尺寸的比例,L为底片中相邻破片质心间的距离,Si为底片中第i个破片距装药下表面的距离,D为炸药的爆速。由于(1)式中不显含ζ,所以在获取破片的飞行速度时,不需要考虑底片中ζ的具体值。但有时在实验底片中无法显现装药的下表面,故难以判读破片运动的真实距离,只能获得其相对距离,同时考虑到不同破片的速度有时会出现波动,因此,实际分析时可根据(2)式计算破片的初速,即
v=n∑i=2(Si−Si−1)(n−1)L/D=D⋅Sn−S1(n−1)L=Dk (2) 式中:n表示破片的总数,Sn-S1和(n-1)L分别表示底片中第n个破片与第1个破片之间的纵向和横向的距离。因此,(2)式也可以表示为:破片速度等于底片中破片曲线的斜率k与炸药爆速的乘积。
2.2 实验过程及结果
实验时,破片及轻质壳体的材料、尺寸均根据实际战斗部进行选择。破片采用93钨合金方形破片,尺寸为6.5 mm×6.5 mm×3.8 mm,质量约为2.81 g;轻质壳体材料选用LY12铝合金,厚度为1.8 mm,宽度略大于破片。将破片排布并粘接在条状铝合金板上,然后再与炸药侧面粘接,以模拟破片内置及外置两种工况。实验所用主装药均为B炸药,密度为1.65 g/cm3,爆速约为7.8 km/s。由于装药的长度大于底片盒的宽度,故实验时将装药竖立放置,破片向侧向运动(实验布局如图 5所示);同时,由于采用的数字成像板不能二次曝光,图像中难以同时出现装药的基准线,因此起爆前先拍一发静止相片以判断装药的轴线是否与竖直悬挂的底片平行,然后再将成像板重新装入底片盒进行实验。
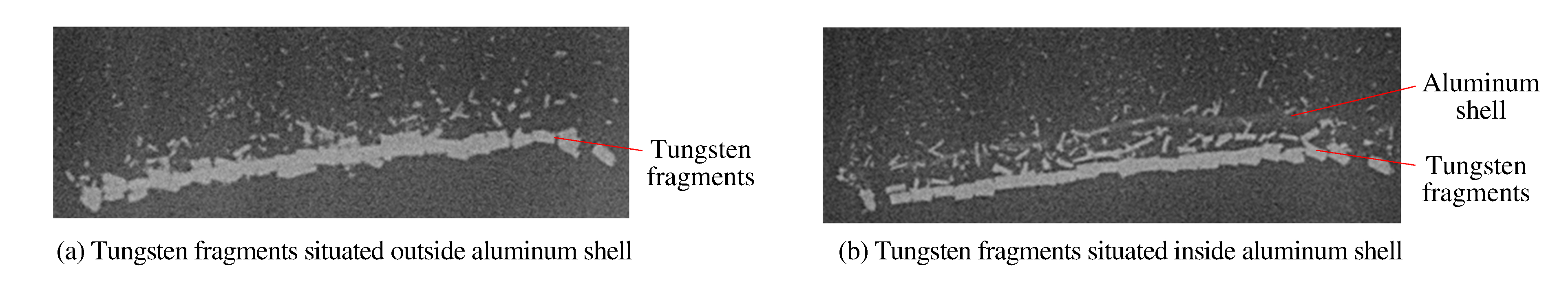
图 6为爆轰加载实验所获得的X射线摄影底片。图 6(a)显示了破片外置时钨破片和铝壳加速过程中的状态,其中并没有独立显示出铝壳体的迹线,且钨破片的下边界不太整齐,表明铝壳体与钨破片粘接在一起,两者速度基本一致;钨破片上方有部分细小的碎渣,其图像灰度与钨破片近似,表明爆轰加载过程中钨破片发生了轻微的破损。图 6(b)显示了破片内置时钨破片与铝壳加速过程中的状态,可以看出,钨破片下表面较为完好,但上表面处既存在一些细小的碎渣,同时还有较完整的条状碎裂体,其图像灰度与钨破片近似,可能是由于钨破片直接与炸药接触,其压力较高致使破片发生了较明显的层裂;钨破片图像上方出现了一条灰度相对较浅的线条,这是铝壳体的迹线(X射线摄影中,图像灰度与物质的密度相关),表明加载过程中铝壳体的速度明显高于钨破片。
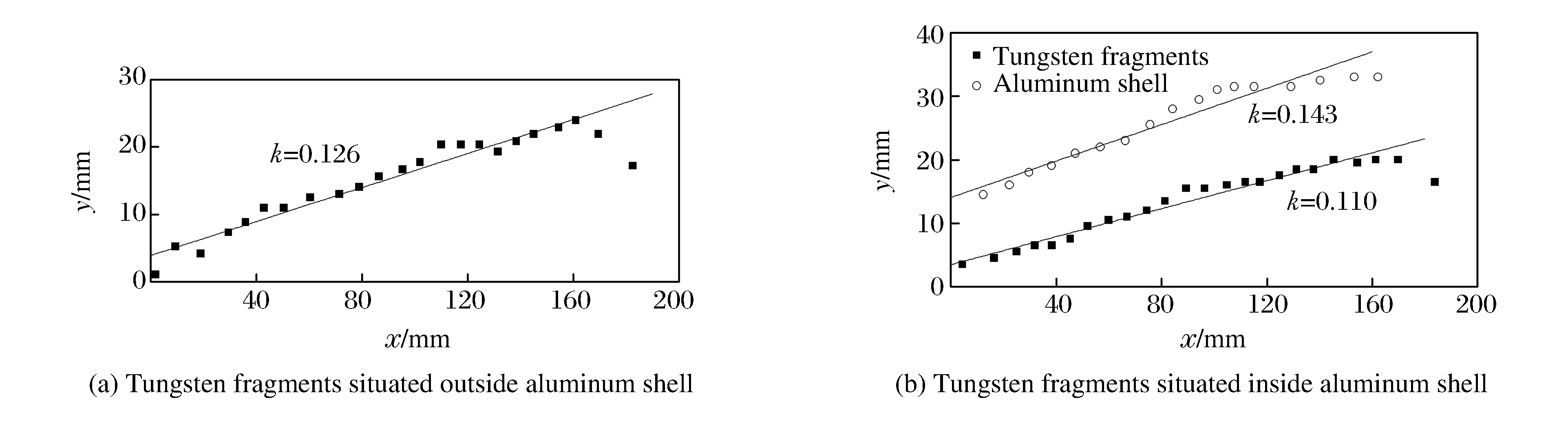
底片图像的横向距离(设坐标为x)可用于计算时间,纵向距离(设坐标为y)可用于计算破片的位移。由于每张底片的放大比不同,所以这些数据仅表示在底片中所处的位置,其数值并没有实际的物理意义,仅用于计算曲线的斜率。采用最小二乘法获得曲线的斜率k后,再按照(2)式计算破片的初速v。
图 7为底片的判读结果。对数据进行拟合计算时,考虑到边侧少量破片在加载时受到稀疏波等因素的影响,其速度明显偏低,故将其删去后再拟合,所获结果列于表 1。
表 1 不同工况下的破片及壳体速度Table 1. Velocity of fragments and shell under different conditionsWorking condition Shell Fragments k v/(m·s-1) k v/(m·s-1) Tungsten fragments situated outside aluminum shell 0.126 983 0.126 983 Tungsten fragments situated inside aluminum shell 0.143 1 115 0.110 858 2.3 分析与讨论
从表 1中可以看出,破片内置时,钨破片的速度明显偏低,铝壳体的速度相对较高,且破片与壳体的动能之和也低于破片外置时的状态。这可能是因为此时钨破片直接与炸药接触,其上表面发生了相对严重的层裂(如图 6(b)所示),其碎裂部分的质量较大,且速度相对偏高,因此从整体上看,破片外置时,钨破片的完整性及速度均具有明显优势。轻质壳体和破片的完整性及初速均涉及其受力过程,故对应力波在这两种介质中的传播过程进行分析。
为了简化分析过程,不考虑应力波在同一介质中的衰减,主要分析在不同介质间传播时的应力变化。分析过程中,首先需要描述爆轰产物作用过程的p(u)曲线以及铝合金壳体、钨合金破片的p(u)曲线,即波阵面压力与波阵面后粒子速度的关系曲线。若炸药以滑移爆轰方式驱动壳体及破片,则爆轰产物的p(u)曲线可根据(3)式计算[9]
u=2Dγγ2−1⋅[1−(ppCJ)γ−12γ] (3) 式中:pCJ=ρ0D2/(γ+1),ρ0和D分别为炸药密度和爆速;B炸药的多方指数γ=2.706[9]。铝合金和钨合金的p(u)曲线根据(4)式计算
P=ρu(c0+λu) (4) 式中:ρ和c0分别为金属材料的密度及声速,λ为本构方程参数。其具体数值列于表 2。
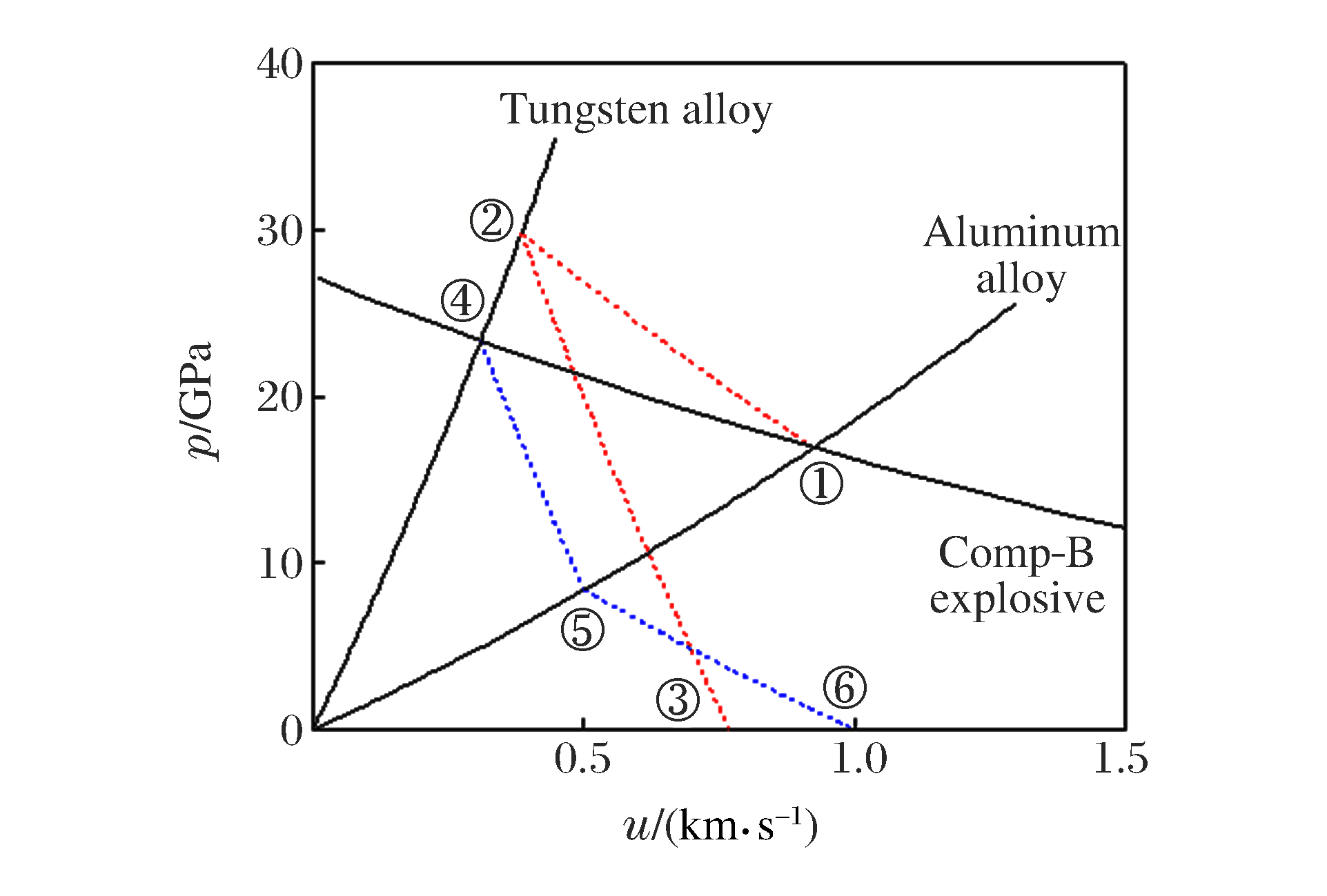
图 8为应力波在不同介质中传播过程示意图,其中:3条实线分别表示B炸药爆轰产物的等熵线以及铝合金壳体、钨合金破片的p(u)曲线,爆轰产物的等熵线与金属材料p(u)曲线的交点坐标即为金属材料中的入射冲击波压力和波阵面后的粒子速度;金属介质间应力波的传播路径主要沿冲击材料在该状态点的反演线(如图 8中虚线所示),其计算表达式为[10]
p=ρ(2u0−u)[c0+λ(2u0−u)] (5) 式中:u0表示碰撞前的粒子速度。反演曲线与被冲击材料p(u)曲线的交点即表示碰撞后的状态。此外,由于空气的密度及声速均远低于金属材料,因此图 8中采用横坐标轴近似其p(u)曲线。
由图 8和表 3可知, 破片置于轻质壳体外侧时:钨破片一端受到从壳体传入的冲击波作用,另一端受到空气反射的拉伸波作用,使其速度容易快速提升;而轻质壳体一端受到爆轰产物传入的冲击波作用,另一端受到钨破片反射的冲击波作用,两个作用方向相反,限制其速度过快提升,不会过多地占用炸药所转换的动能,同时没有拉伸波作用,轻质壳体也不容易快速破裂,在实际战斗部结构中可以增加对爆轰产物的约束,有利于对后期爆轰产物膨胀驱动能量的利用。
表 3 材料界面处的状态参数Table 3. State parameters of material interfacesInterface No. u/(km·s-1) p/GPa ① 0.931 16.82 ② 0.385 29.70 ③ 0.770 ④ 0.310 23.30 ⑤ 0.502 8.26 ⑥ 1.004 破片置于轻质壳体内侧时:虽然钨破片受到爆轰产物的冲击波及铝合金壳体反射的拉伸波作用,但从整体上看,其界面的平均粒子速度低于破片外置时的状态;而铝合金壳体由于外侧没有高阻抗介质阻挡,所受到的拉伸波作用可使其速度快速提升,但也易造成过早破裂。该工况在一些具有特定要求的战斗部中具有某些方面的优势,设计时可考虑在破片与装药之间增添一些性能较好的缓冲体,以提升破片的完整性;在轻质壳体与破片之间,也可适当增设缓冲材料,以减小轻质壳体与破片之间的速度差,不仅可以避免壳体过多地占用能量,也可减小壳体所受到的冲击力,延缓其破裂时间,从而增加对爆轰产物的约束时间,提升爆轰产物驱动能量的利用率。当然,本研究只针对冲击碰撞阶段的加速过程,未进行两个加速阶段的系统研究,也未涉及轻质壳体的动态破裂机理问题,还需要进一步深入研究。
3. 结论
(1) 预制破片置于轻质壳体外侧时,初始应力波由低阻抗金属材料向高阻抗金属材料传播,使破片受到壳体传入的冲击波及空气传入的拉伸波作用,其速度易迅速提升,且破损轻微;而轻质壳体没有受到拉伸波作用,不易快速破裂,对后期爆轰产物膨胀驱动能量的利用较为有利。
(2) 预制破片置于轻质壳体内侧时,初始应力波主要由高阻抗金属材料向低阻抗金属材料传播,虽然破片受到爆轰产物传入的冲击波及壳体反射的拉伸波作用,但其初速低于破片外置时的状态,且易发生破损,甚至有明显层裂现象;而铝合金壳体由于受到空气传入的拉伸波作用,其速度可快速提升,但也易造成其过早破裂,可能减少对爆轰产物的约束时间,降低炸药驱动能量的利用率。
-
表 1 Mg2Si和Mg2Ge的晶格常数
Table 1. Equilibrium crystal parameters (a, c) of Mg2Si and Mg2Ge
表 2 Mg2Si和Mg2Ge的弹性常数
Table 2. Moduli of Mg2Si and Mg2Ge
Phase p/GPa C11 C12 C44 B/GPa G/GPa E/GPa G/B υ 0 110.48 22.04 44.72 51.52 44.52 103.69 0.86 0.160 Calc.[18] 115.21 22.14 43.11 53.163 44.48 104.34 0.84 0.173 5 143.03 42.46 52.29 75.99 51.49 126.00 0.68 0.22 Mg2Si 10 158.67 52.89 61.20 88.15 57.87 142.45 0.66 0.23 15 180.72 68.97 61.32 106.22 59.14 149.65 0.56 0.27 20 200.49 85.12 64.56 123.58 61.81 158.93 0.50 0.27 25 218.17 102.33 66.19 140.94 62.88 164.22 0.45 0.31 0 105.80 21.18 41.90 49.39 42.07 98.29 0.85 0.17 Calc.[19] 113.56 20.56 45.70 51.56 46.02 106.40 0.85 0.16 5 140.74 45.52 55.27 77.26 52.21 127.83 0.68 0.22 Mg2Ge 10 154.03 50.75 54.12 85.17 53.13 131.95 0.62 0.24 15 175.03 68.13 59.79 103.76 57.25 145.07 0.55 0.27 20 191.53 83.03 61.82 119.19 58.79 151.48 0.49 0.29 25 209.82 101.41 63.24 137.54 59.62 156.29 0.43 0.31 -
[1] 陈茜, 谢泉, 杨创华, 等.掺杂Mg2Si电子结构及光学性质的第一性原理计算[J].光学学报, 2009, 29(1):229-235. http://cqvip.com/QK/95626X/200901/29234382.htmlCHEN Q, XIE Q, YANG C H, et al.First-principles calculation of electronic structure and optical properties of Mg2Si with doping[J].Acta Optica Sinica, 2009, 29(1):229-235. http://cqvip.com/QK/95626X/200901/29234382.html [2] 傅利, 赵宇宏, 杨晓敏, 等.Mg-Al-Si-Ca合金系金属间化合物的电子结构和力学性能的第一性原理计算[J].稀有金属材料与工程, 2014, 43(11):2733-2738. http://www.cnki.com.cn/Article/CJFDTotal-COSE201411035.htmFU L, ZHAO Y H, YANG X M, et al.First-principle calculation for electronic structure and mechanical properties of intermetallics in Mg-Al-Si-Ca Alloy[J].Rare Metal Materials and Engineering, 2014, 43(11):2733-2738. http://www.cnki.com.cn/Article/CJFDTotal-COSE201411035.htm [3] ZHANG J, FAN Z, WANG Y Q, et al.Microstructural development of Al-15 wt.% Mg2Si in situ composite with mischmetal addition[J].Materials Science and Engineering A, 2000, 281(1/2):104-112. https://www.researchgate.net/publication/271916998_Application_Prospects_and_Microstructural_Features_in_Laser-Induced_Rapidly_Solidified_High-Entropy_Alloys [4] 余本海, 刘墨林, 陈东.第一性原理研究Mg2Si同质异相体的结构、电子结构和弹性性质[J].物理学报, 2011, 60(8):584-594. http://wuxizazhi.cnki.net/Sub/jcwl/a/WLXB201107076.htmlYU B H, LIU M L, CHEN D.First principles study of structural, electronic and elastic properties of Mg2Si polymorphs[J].Acta Physica Sinica, 2011, 60(8):584-594. http://wuxizazhi.cnki.net/Sub/jcwl/a/WLXB201107076.html [5] TANI J, KIDO H.First-principles and experimental studies of impurity doping into Mg2Si[J].Intermetallics, 2008, 16(3):418-423. doi: 10.1016/j.intermet.2007.12.001 [6] JUND P, VIENNOIS R, COLINET C, et al.Lattice stability and formation energies of intrinsic defects in Mg2Si and Mg2Ge via first principles simulations[J].Journal of Physics:Condensed Matter, 2013, 25(3):4469-4487. [7] GANESHAN S, SHANG S L, WANG Y, et al.Temperature dependent elastic coefficients of Mg2X (X=Si, Ge, Sn, Pb) compounds from first-principles calculations[J].Journal of Alloys and Compounds, 2010, 498(2):191-198. doi: 10.1016/j.jallcom.2010.03.153 [8] WANG H, JIN H, CHU W, et al.Thermodynamic properties of Mg2Si and Mg2Ge investigated by first principles method[J].Journal of Alloys and Compounds, 2010, 499(1):68-74. doi: 10.1016/j.jallcom.2010.01.134 [9] 张建新, 高爱华, 郭学锋, 等.Mg-Sn-Si合金中Mg2(Si, Sn)复合相的结构与性能研究[J].物理学报, 2013, 62(17):483-488.ZHANG J X, GAO A H, GUO X F, et al.Study on microstructure and poperties of Mg2(Si, Sn) compound phase in Mg-Sn-Si magnesium alloy[J].Acta Physica Sinica, 2013, 62(17):483-488. [10] SHI D M, WEN B, MELNIK R.First-principles studies of Al-Ni intermetallic compounds[J].Journal of Solid State Chemistry, 2009, 182(10):2664-2669. doi: 10.1016/j.jssc.2009.07.026 [11] WANG F, SUN S J, YU B, et al.First principles investigation of binary intermetallics in Mg-Al-Ca-Sn alloy:stability, electronic structures, elastic properties and thermodynamic properties[J].Transactions of Nonferrous Metals Society of China, 2016, 26(1):203-212. doi: 10.1016/S1003-6326(16)64107-9 [12] FAGAN S B, MOTA R, BAIERLE R J.Stability investigation and thermal behavior of a hypothetical silicon nanotube[J].Journal of Molecular Structure, 2001, 539(1/2/3):101-106. https://www.sciencedirect.com/science/article/pii/S0166128000007776 [13] CHADI D J.Special points for Brillouin-zone integrations[J].Physical Review B, 1977, 16(4):5188-5192. [14] FISCHER T H, ALMLOF J.General methods for geometry and wave function optimization[J].Journal of Physical Chemistry, 1992, 96(24):9768-9774. doi: 10.1021/j100203a036 [15] TANI J, KIDO H.Lattice dynamics of Mg2Si and Mg2Ge compounds from first-principles calculations[J].Computational Materials Science, 2008, 42(3):531-536. doi: 10.1016/j.commatsci.2007.08.018 [16] CAO Y, ZHU J C, NONG Z S, et al.First-principles studies of the structural, elastic, electronic and thermal properties of Ni3Nb[J].Computational Materials Science, 2013, 77(3):208-213. [17] PUGH S F.Relations between the elastic moduli and the plastic properties of polycrystalline pure metals[J].Philosophical Magazine, 1954, 45(367):823-843. [18] 任玉艳, 刘桐宇, 李英民.Mg2Si金属间化合物的结构稳定性, 热力学和力学性能的第一性原理计算[J].中国科学:物理学力学天文学, 2016, 46(8):26-33. http://wuxizazhi.cnki.net/Sub/jcwl/a/WLXB201107076.htmlREN Y Y, LIU T Y, LI Y M.First-principles calculation on structure stability, thermodynamic and mechanical properties of Mg2Si intermetallics[J].Scientia Sinica physica, Mechanica and Astronomica, 2016, 46(8):26-33. http://wuxizazhi.cnki.net/Sub/jcwl/a/WLXB201107076.html [19] 常少梅.压力作用下Mg2X(X=Sn, Ge, Si)晶体结构与弹性性质[J].兰州理工大学学报, 2012, 38(5):168-171.CHANG S M.Structural and elastic properties of Mg2X (X=Sn, Gn, Si) under pressure[J].Journal of Lanzhou University of Technology, 2012, 38(5):168-171. [20] GUO S D.First-principles calculations of Mg2X (X=Si, Ge, Sn) semiconductors with the calcium fluorite structure[J].Journal of Semiconductors, 2015, 36(5):8-13. -








 下载:
下载:








 下载:
下载: