在压力的作用下，物质的原子间距缩短，体积收缩，内部能量状态随之发生改变；当压力达到一定阈值后，物质出现晶体结构的“突变”，即压致相变，从而引起物质热学、力学和光学等性质的改变。自Bridgman^[1–2]的开创性工作以来，人们结合实验和理论研究获取了大量物质体系在数百吉帕甚至更高压力范围的温度-压力相图，发现了极其丰富的高压新相。然而，越来越多的实验和理论研究表明，在不同加载应变率下得到的相变结果可能与平衡态相图不一致，这是因为高压下热力学稳定相的形成需要经历成核和生长等与时间密切相关的过程。因此，加载应变率是物质高压相变行为的关键影响因素之一^[3–12]。高压相变行为的应变率效应是目前高压科学的前沿科学问题和研究热点，相关实验和理论工作仍存在巨大挑战。在实验方面，发展覆盖宽应变率范围的系列动态加载平台和高时间分辨的原位相结构诊断手段是当前的重点工作之一。

根据加载应变率的不同，高压实验通常被分为静高压和动高压两类。在以金刚石对顶砧（diamond anvil cell，DAC）和大腔体压机为代表的静高压实验中，压力加载一般持续几分钟甚至更长的时间。由于加压很慢，样品始终趋于热力学平衡态，因此，静高压实验通常获取物质的平衡态相图。然而，在以轻气炮和激光为代表的动高压实验中，压力加载时间在纳秒至微秒量级，物质在短时间内偏离热力学平衡态并有可能形成亚稳相，导致实验得到不同于平衡态的相图。在介于动高压与静高压之间的压力加载过程（微秒至分钟的时间尺度）中，由于加载技术发展滞后，相应材料的高压相变行为研究仍处于初步阶段，根据有限的报道^[6–12]，物质的高压相变行为同样受加压速率的调控，如相界移动和亚稳相形成等。值得注意的是，应变率表征材料变形的速度，单位为s⁻¹，而加压速率则表征压力的变化速度，单位为GPa/s，二者之间可相互转化。在相同的加压速率下，试样的应变率会因体模量的不同而不同。

由于缺乏以X射线衍射（X-ray diffraction，XRD）为代表的原位相结构诊断技术对宽广应变率加载下物质相变动力学过程的系统观测，人们难以开展基于原子尺度物理机制的相变动力学过程建模和数值模拟研究。目前，还没有建立统一的物理模型来描述相变动力学过程是如何从静态压缩演化到高应变率冲击加载，更无法解释静高压和冲击压缩实验中相变机制不一致的问题。跨越从静高压到动高压的宽广应变率，统一认知高压相变动力学过程是未来压缩科学发展的重要挑战之一，相关研究具有重要的科学和工程意义。

单晶硅是电子工业的基础，广泛应用于大规模集成电路和太阳能电池。在高压作用下，半导体硅在约11 GPa转变成金属相Si-Ⅱ^[13–16]，这种电子转变直接破坏了集成电路的应用基础。另外，硅是一种典型的硬脆材料，在对硅基片进行机械加工的过程中，硅的力学性能直接影响产品的良品率。因此，硅的高压相变研究具有重要的物理和工程意义。另外，硅的高压相极其丰富，拥有大量亚稳相，并且动力学因素在硅的高压相变中扮演重要角色，因此，硅是研究高压相变动力学的理想材料，对于普适相变动力学过程的理论建模具有重要意义。本研究以硅为例，依次介绍其在准静态（静高压）、中等应变率（快速加/卸压）和高应变率（轻气炮和激光）加载下的高压相变行为，探讨加载应变率对其相变行为的影响。

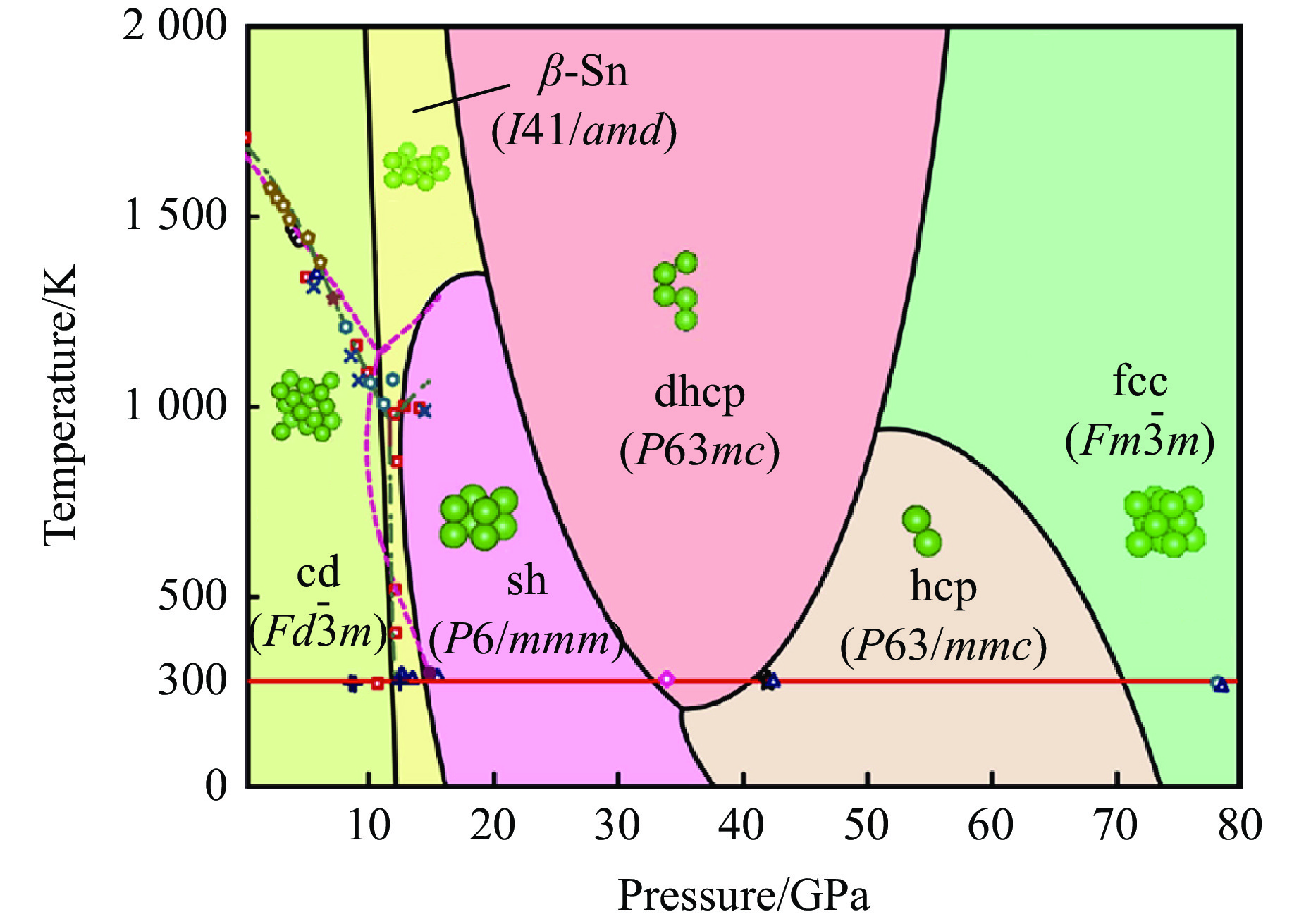
1.   静高压加载下硅的相变行为

在高压科学和实验技术领域，DAC发挥着至关重要的作用。研究高压相变最直接、最理想的方法是XRD，其他测试手段（如声速和电阻率）也能给出结构相变伴随的物性突变，但是不能提供相变前后样品的微观晶体结构信息。结合DAC高压XRD实验以及第一性原理计算，人们发现了硅的丰富的高压相，获取了其宽广温度-压力范围的相图，如图1^[13–16]所示。常温常压下，硅的稳定相是立方金刚石（cubic diamond，cd）相，常记为Si-Ⅰ相。当压力升高至约11 GPa时，Si-Ⅰ相转变为β-Sn结构的金属相，也记为Si-Ⅱ相。Si-Ⅱ相的密度比Si-Ⅰ相高约22%。由于存在动力学壁垒，从Si-Ⅰ相到Si-Ⅱ相的相变是不可逆的。进一步对硅加压，Si-Ⅱ相在约14 GPa转变成简单六角（simple hexagonal，sh）结构，即Si-Ⅴ相。继续升压至33 GPa时，Si-Ⅴ相转变成双层密排六方（double hexagonal close-packed，dhcp）结构，即Si-Ⅵ相。随着压力的进一步升高，Si-Ⅵ相在约41 GPa时转变成密排六方（hexagonal close-packed，hcp）相，即Si-Ⅶ相。当压力升高到约72 GPa时，Si-Ⅶ相又转变为面心立方（face-centered cubic，fcc）结构，即Si-Ⅹ相，这一结构在248 GPa以内保持稳定。在高压的作用下，硅中较弱的Si―Si键不断地发生变形、断裂和重组，进而形成如此复杂的高压相图。静高压实验得到的平衡态相图常作为理论计算校核的基准。

图  1  硅的高温-高压相图^[13–16]

Figure  1.  High temperature and high pressure phase diagram of silicon^[13–16]

下载: 全尺寸图片 幻灯片

硅从半导体到金属相的转变主要是由于高压导致硅原子的配位数改变，致使其价电子不能完全局域在共价键上，因而出现了自由电子。随着外界压力的升高，硅原子的配位数不断增加，共价键也随之减弱，即从4配位数Si-Ⅰ相中的三维共价键网络变为12配位数Si-Ⅹ相中的完全金属键。除上述热力学稳定相外，硅还存在丰富的亚稳相。如果从热力学角度考虑，这些亚稳相硅都不可能稳定存在于任何压力条件下。然而，在不同的实验条件下，这些能量相对较高的亚稳相硅（如bc8和r8）已经被成功制备出来，因此，动力学因素在硅的相变过程中扮演着至关重要的角色。

2.   快速加/卸压下硅的相变行为

2.1   快速卸压下硅的相变行为

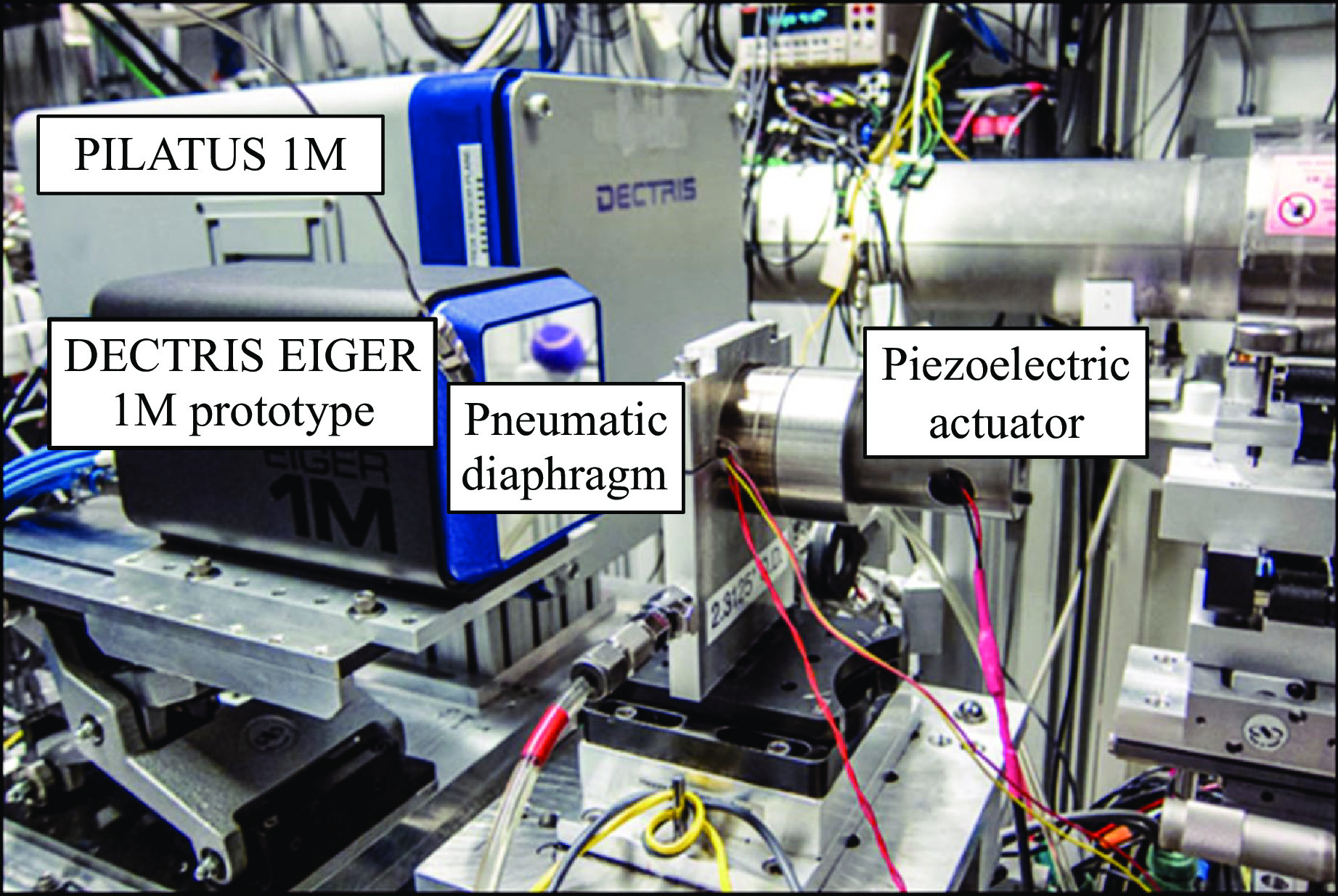
在DAC静高压实验中，人们通常采用手动拧紧螺丝来减小金刚石压砧的间距，进而压缩样品腔的体积，在样品中产生高压。基于压电陶瓷和DAC的快速加压装置^[17–20]（dynamic diamond anvil cell，dDAC）是利用压电陶瓷在外加正电压下长度增大的原理，通过设计金属套筒将压电陶瓷与DAC紧密固定到一起，如图2所示，只需对压电陶瓷施加快速增大的电压，就可以对DAC压腔中的样品实现快速压缩，加压速率可达10⁻³～10⁵ GPa/s （对应的应变率为10⁻³～10² s⁻¹），相应的时间尺度介于静高压与动高压之间。dDAC装置既可以实现快速加压，也可以实现快速卸压，且加/卸压速率可精密控制。

图  2  同步辐射时间分辨XRD探测系统^[18]

Figure  2.  Synchrotron radiation-based time-resolved XRD diagnostic system^[18]

下载: 全尺寸图片 幻灯片

结合基于第三代同步辐射光源的时间分辨X射线技术和加压速率精密可控的dDAC装置，可直接获取样品在加压过程中晶体结构的实时演化信息，通过分析实验数据，可明确相变的阈值压力、相变路径以及可能的亚稳相^{[7–12, 18–19]}。通过连续调节样品的加压速率并获取不同加压速率下样品的结构演化信息，可获取高压相变过程与加压速率（或应变率）的依赖关系。

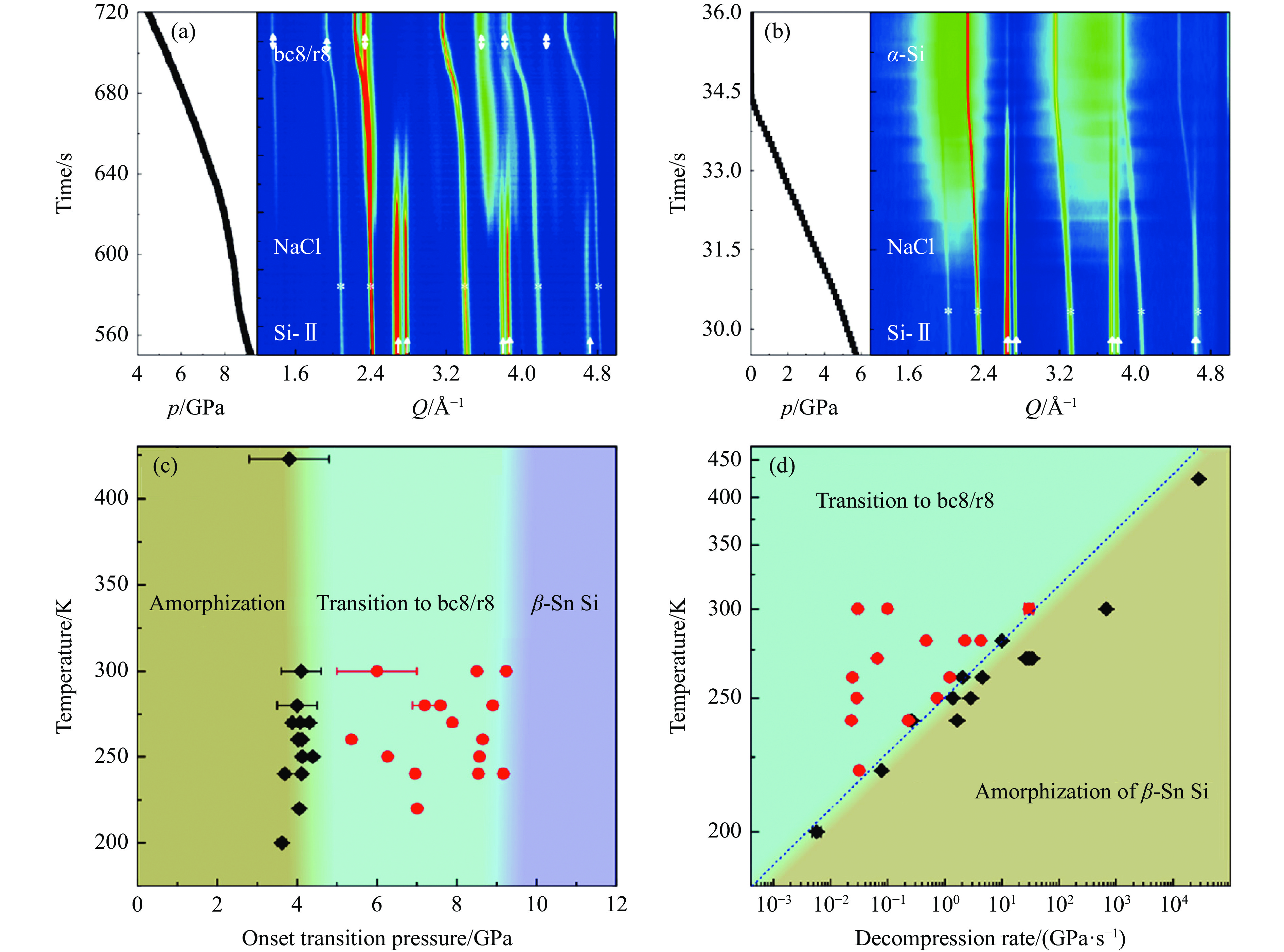
硅在卸压条件下的相变行为备受关注，主要是因为高压相硅（Si-Ⅱ）在不同卸压过程中可以转化为不同结构的亚稳相硅，这些亚稳相可截留至常压，且具有优异的光电性质。Lin等^[7]联合dDAC快速加/卸载装置、低温技术和同步辐射时间分辨XRD原位诊断技术，系统研究了Si-Ⅱ在不同温度和卸压速率下的相变过程，发现Si-Ⅱ在卸压过程中存在2种不同的相变路径，如图3所示，其中：p为压力，Q为散射矢量。缓慢卸压下，Si-Ⅱ在4.3～9.2 GPa压力范围内经历热活化的晶体-晶体转变，并转化为bc8/r8结构的晶体硅，其相变压力和初始应变依赖于卸压速率和温度。与之不同的是，在快速卸压下，约4.3 GPa时，Si-Ⅱ的四面体结构因力学不稳定性而扩展到临界体积应变，并塌陷为非晶硅。研究表明，卸压速率和温度共同影响Si-Ⅱ的相变动力学过程，这对于亚稳相硅的合成具有重要的指导意义。

图  3  Si-Ⅱ在不同温度和卸压速率下的相变过程^[7]

Figure  3.  Phase transition process for Si-Ⅱ at different decompression rates and temperatures^[7]

下载: 全尺寸图片 幻灯片

2.2   快速加压下硅的相变行为

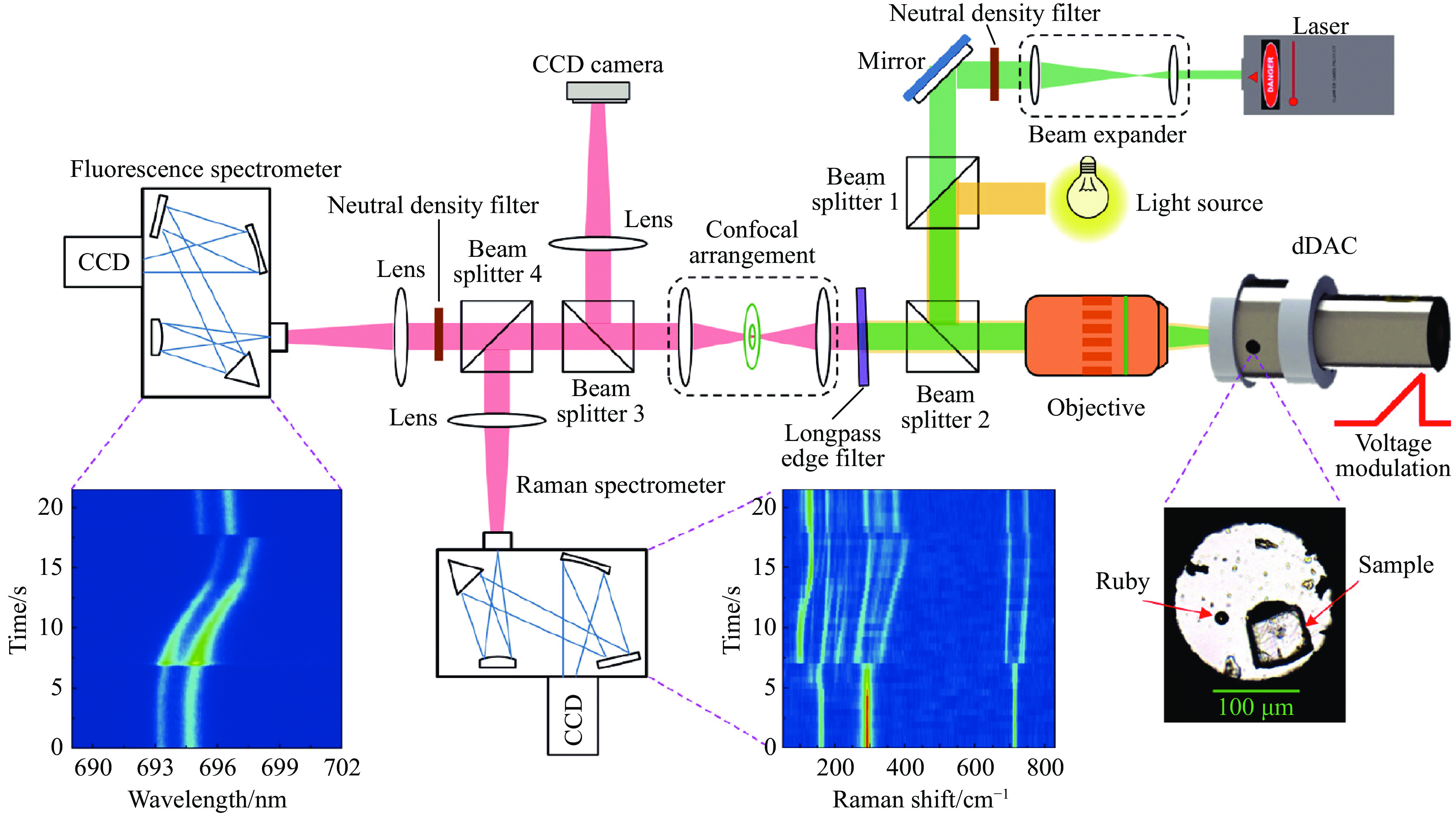
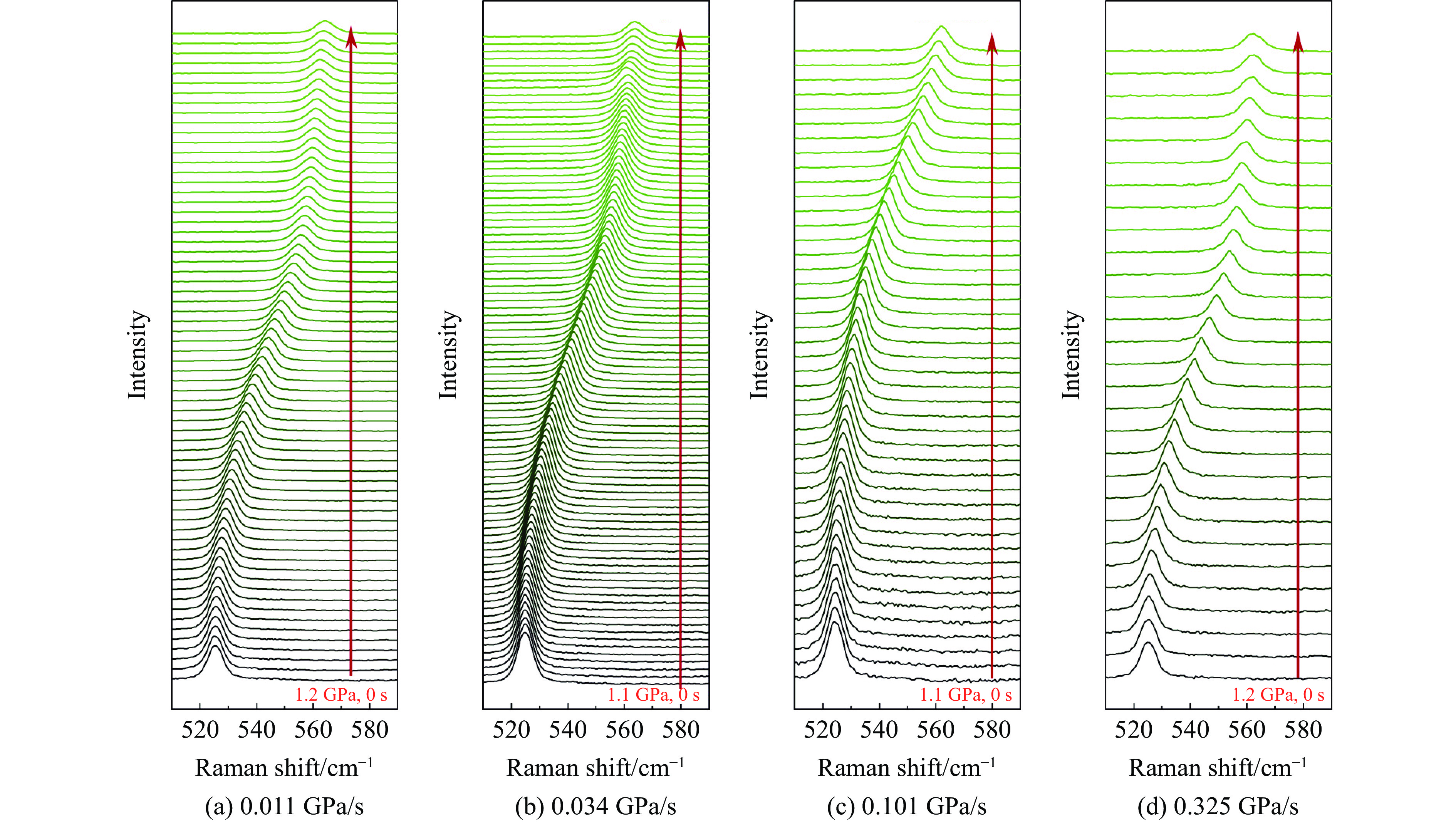
拉曼光谱是一种振动、转动光谱。在物质的相变过程中，随着结构的改变，拉曼光谱也发生变化。因此，在加压过程中，通过实时、连续监测样品的拉曼光谱，可以得到物质结构相变的阈值压力，进而获取相变速率和相变路径等动力学信息。Chen等^[21]利用显微共聚焦系统、分光系统和2台光谱仪，实现了红宝石荧光和样品拉曼光谱的同时测量，通过函数发生器控制和同步快速加压装置dDAC、2台光谱仪的快门以及时序记录系统，成功实现了可控加压与相结构演化的连续测量，如图4所示，为快速压缩下物质相变动力学研究提供了一种有效的手段。

图  4  时间分辨拉曼光谱探测系统^[21]

Figure  4.  Time-resolved Raman spectroscopy system^[21]

下载: 全尺寸图片 幻灯片

在静高压加载下，当压力升高至约11 GPa时，Si-Ⅰ相转变为Si-Ⅱ相，如图1所示。但是，从高压相Si-Ⅱ卸压时，硅并没有返回Si-Ⅰ相，而是转变成亚稳相，且最终形成哪一种亚稳相取决于卸压速率^[7]。硅在卸压过程中呈现的复杂相变行为尤其是亚稳相的形成不禁让人质疑其在快速加压下能否出现相同的亚稳相。另外，高应变率激光冲击压缩下，Si-Ⅰ到Si-Ⅱ的相界降到约5 GPa；而在纳米压痕实验中，Si-Ⅱ相的出现发生在约8 GPa。这些相界均下移至Si-Ⅰ的热力学稳定场中，然而关于快速压缩下加压速率对Si-Ⅰ→Si-Ⅱ相界的调控问题至今尚无报道。

本研究利用自主研制的时间分辨拉曼光谱系统（图4），通过在DAC样品腔中添加传压介质甲醇-乙醇来消除剪应力的影响，结合快速加压装置具备的加压速率连续调控的能力，获得了加压速率对硅在Si-Ⅰ稳定场中结构响应的影响。通过实验，本研究获取了0.011～0.325 GPa/s加压速率下硅的时间分辨拉曼数据，如图5所示。在每一种加压速率下，随着压力的升高，Si-Ⅰ相对应的拉曼峰都平滑地向高波数方向移动，反映了压力对晶体结构的压缩效应，即键长缩短，振动频率加快。另外，随着压力的升高，静水压特性降低，晶体缺陷的积累导致拉曼峰展宽增大，但拉曼峰的展宽与加压速率无明显依赖关系。在3和9 GPa压力下，硅均未出现突变，如拉曼峰的新生、消失或者劈裂等现象，表明快速压缩下硅在Si-Ⅰ稳定场中没有出现快速卸压过程中形成的亚稳相。另外，当压力升高至11 GPa时，硅尚未发生Si-Ⅰ到Si-Ⅱ的相变，即相界在快速压缩条件下没有出现显著下移。因此，在加压速率介于0.011～0.325 GPa/s的快速压缩下，硅在Si-Ⅰ稳定场中呈现出高结构稳定性，不仅Si-Ⅰ→Si-Ⅱ相界没有显著下移，而且也未发现亚稳相形成。

图  5  单晶硅在不同加压速率下的时间分辨拉曼光谱

Figure  5.  Time-resolved Raman spectra for single crystal silicon at different compression rates

下载: 全尺寸图片 幻灯片

3.   冲击压缩下硅的相变行为

3.1   轻气炮冲击压缩下硅的相变行为

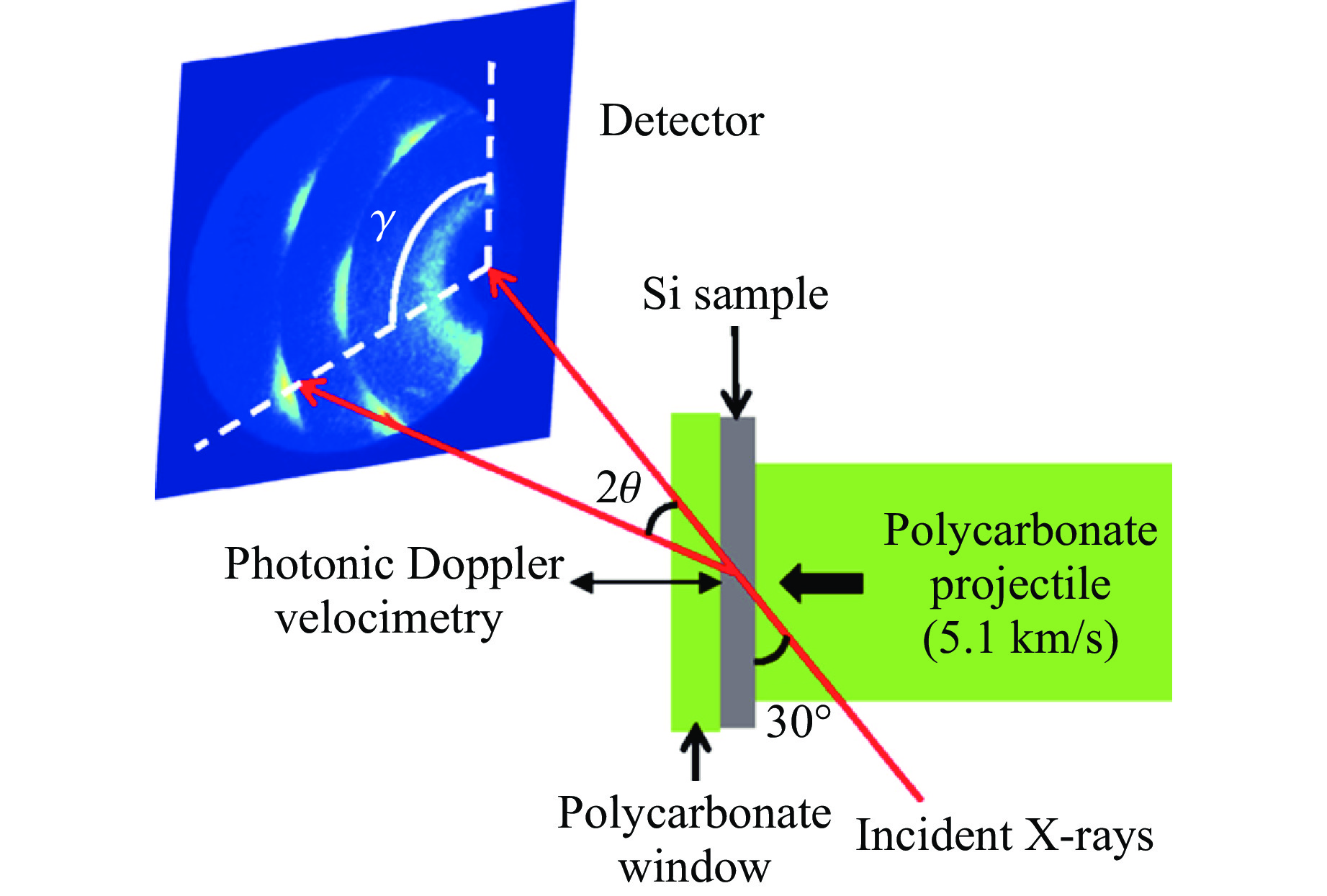
华盛顿州立大学的Gupta团队^[14]基于同步辐射光源先进光子源（Advance Photon Source， APS），在动态压缩线站（Dynamic Compression Sector，DCS）发展了轻气炮冲击压缩下材料结构响应的原位XRD表征技术，如图6所示。利用宽谱粉光代替单色光来提高单发X射线通量，以满足单发XRD应用于物质晶体结构分析的要求。另外，结合X射线闪烁体、分光系统和4台可见光ICCD相机，实现了4幅XRD图像的连续采集，时间间隔为153.4 ns，单个脉冲的时间宽度约为80 ps。

图  6  平板冲击压缩下单晶硅结构响应的时间分辨XRD技术^[14]

Figure  6.  Configuration for the plate impact time-resolved XRD experiments performed on single crystal silicon^[14]

下载: 全尺寸图片 幻灯片

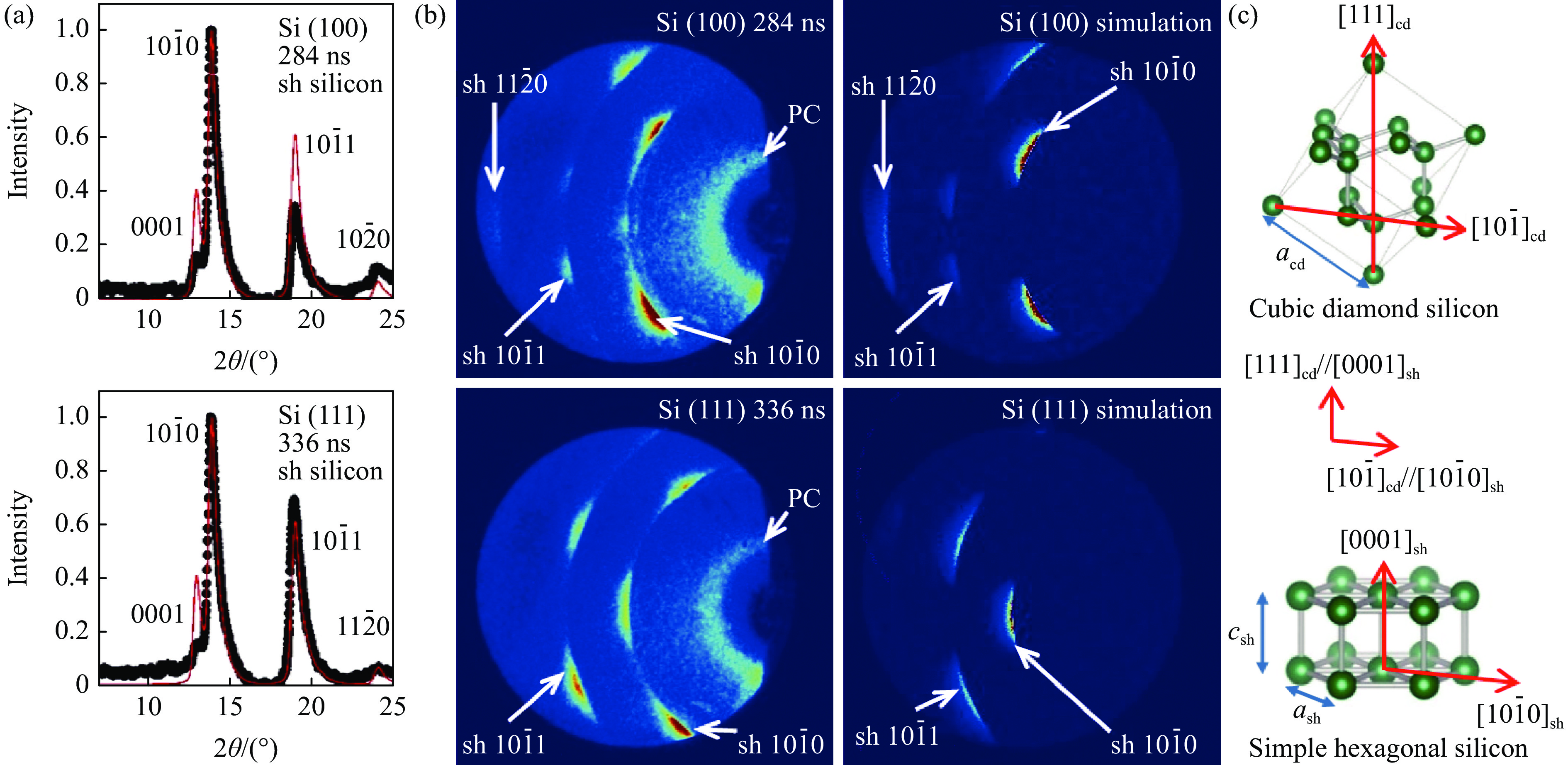
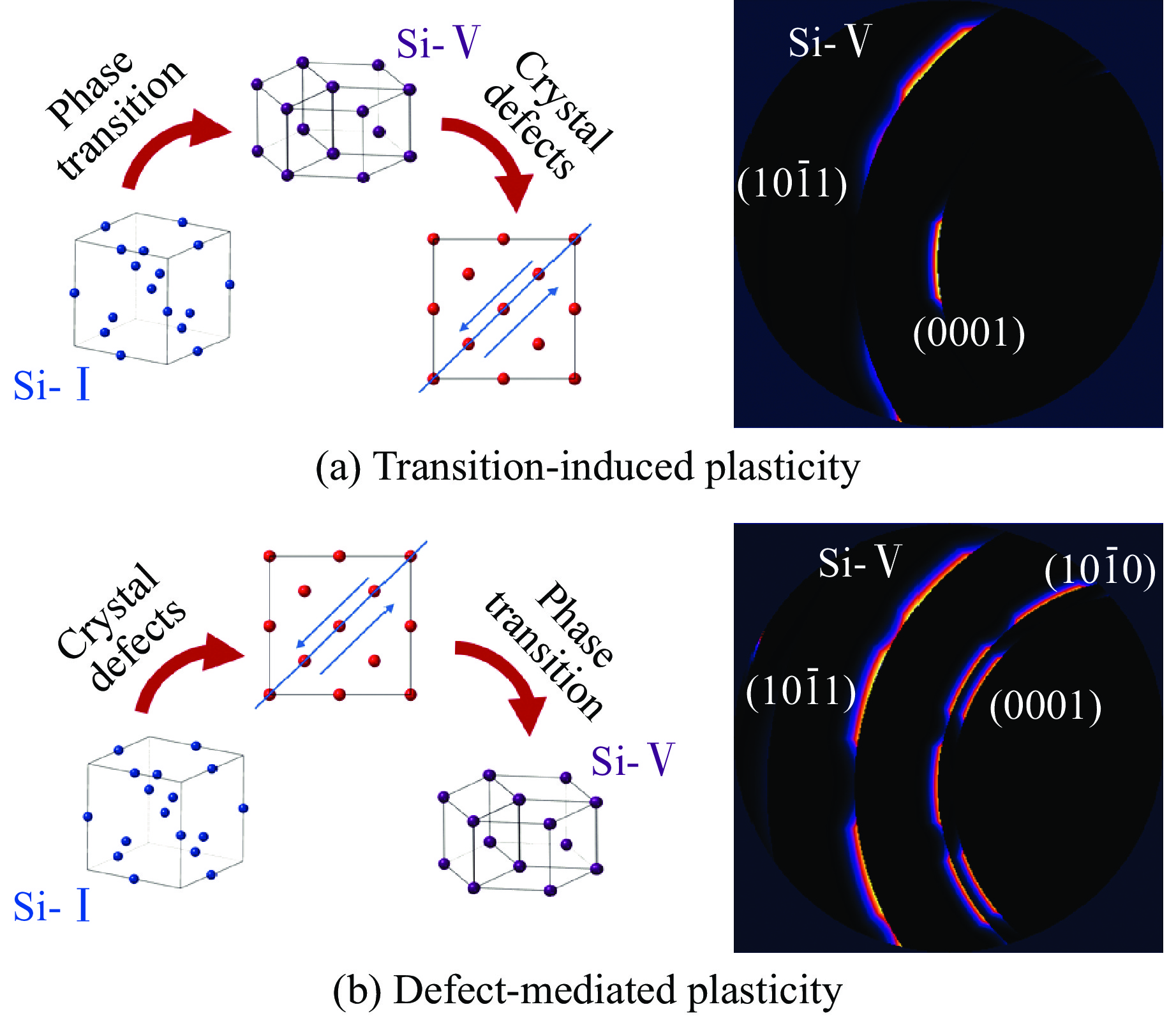
轻气炮平板撞击是目前实验室应用最广泛的冲击波加载技术，加载应变率范围为10⁵～10⁶ s⁻¹。在轻气炮冲击压缩下，当冲击压力为19 GPa时，无论是Si-Ⅰ单晶，还是多晶硅，都转变成Si-Ⅴ相，与硅高压相图（见图1）一致。对于单晶硅样品，冲击压缩下Si-Ⅴ相的XRD图像呈现出明显的择优取向，结合初始相的XRD图像，可以获取高压相变机制研究中极其关心的相变前、后晶体的取向关系。结合(100)和(111)晶向单晶硅在相变前、后的XRD图像演化信息和系统的正向模拟结果，Gupta团队^[14]首次直接获取了冲击压缩下单晶硅由Si-Ⅰ相转变为Si-Ⅴ相的晶体取向关系，即[111]_cd//[0001]_sh和[$10\overline 1$]_cd//[$10\overline{1}0$]_sh，如图7所示，其中：a_cd为Si-Ⅰ相的晶格常数，a_sh和c_sh为Si-Ⅴ相的晶格常数。

图  7  轻气炮冲击压缩（19 GPa）下单晶硅的结构响应^[14]

Figure  7.  Structural response of single crystal silicon under shock compression (19 GPa) by light gas gun^[14]

下载: 全尺寸图片 幻灯片

3.2   激光冲击压缩下硅的相变行为

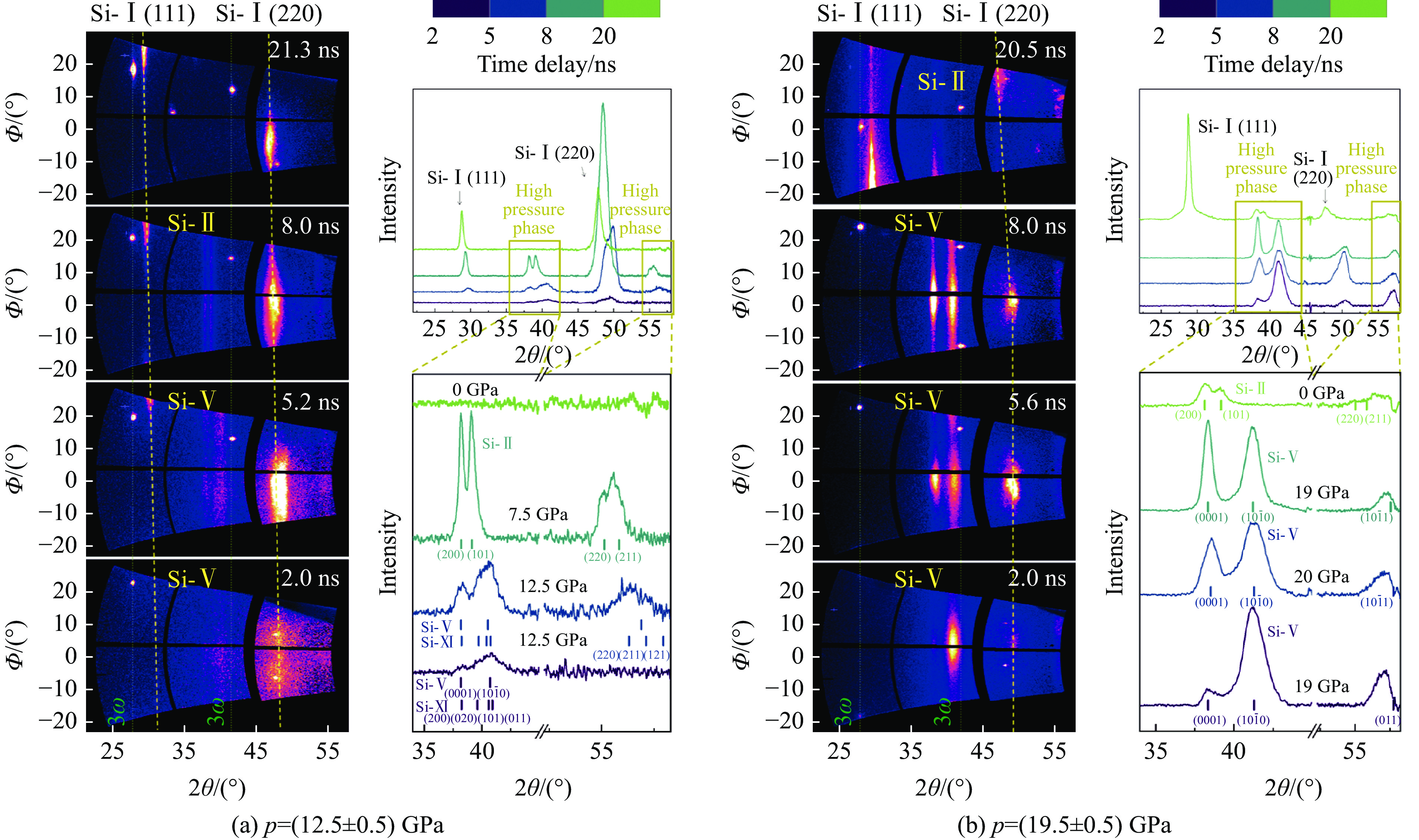
随着高功率激光技术的进步，激光加载技术越来越广泛地用于更高应变率下的冲击实验，应变率范围达10⁹～10¹² s⁻¹。Pandolfi等^[15]基于泵浦-探测模式，利用纳秒激光加载，结合飞秒时间分辨自由电子激光XRD技术，获取了高应变率冲击加载下单晶硅的结构响应特征，如图8所示，其中：Φ为衍射空间的径向角度，3ω表示三背频X射线引起的衍射峰。实验结果表明，当冲击压力为12.5 GPa时，Si-Ⅰ相直接转变为Si-Ⅴ相和Si-Ⅺ相；当卸压至7.5 GPa时，Si-Ⅱ相在样品中形成，并在卸压至常压时完全转变成Si-Ⅰ相。当冲击压力为19.5 GPa时，Si-Ⅰ相直接转变为Si-Ⅴ相，在卸压过程中，Si-Ⅴ首先转变为Si-Ⅱ相，卸压至常压时，硅为Si-Ⅰ与Si-Ⅱ的混合相。在高应变率冲击加载后的等熵卸压过程中，未观察到静高压和快速卸压实验中形成的亚稳相，而是得到了Si-Ⅱ相和Si-Ⅰ相，从而为Si-Ⅱ高压相的合成并截获至常压提供了新的思路。无论是准静态加载还是中等应变率加载，Si-Ⅰ相到Si-Ⅱ相的相变均是不可逆的，但是，在高应变率激光加载下，当压力卸至常压时，高压相硅全部转变成Si-Ⅰ相，可能是由于在冲击诱导的高温高压条件下等熵卸载时相变更倾向于晶体-晶体相转变^[15]，而不是低应变率加载实验中经常观察到的非晶化转变。

图  8  激光冲击压缩下单晶硅的结构响应^[15]

Figure  8.  Structural evolution of single crystal silicon under laser shock compression^[15]

下载: 全尺寸图片 幻灯片

结合单晶硅冲击相变前后衍射峰的择优取向关系以及XRD正向模拟，结果表明，冲击压缩下单晶硅剪应力的释放方式取决于加载应变率，如图9^[15]所示：在轻气炮加载下，剪应力是通过位错主导的塑性变形来释放，随后再发生结构相变，即弹性变形→塑性变形→相变；相反，在高应变率激光加载下，剪应力是通过结构相变来释放，随后再发生塑性变形，即弹性变形→相变→塑性变形。

图  9  缺陷主导和相变诱发塑性变形示意图及对应的XRD图像^[15]

Figure  9.  Schematic diagram of the defect-mediated and transition-induced plastic deformation and their XRD signature^[15]

下载: 全尺寸图片 幻灯片

4.   讨　论

在高压作用下，硅的相图非常丰富，且存在大量热力学亚稳相，同时这些亚稳相的形成与加载应变率密切相关，因此，研究硅的高压相变动力学对普适相变动力学过程的理论建模具有重要的科学意义。在静水压条件下，硅的高压相变压力和路径都已经得到了很好的解释，但是，在冲击加载下，硅的冲击相变结果与静高压相图不一致。研究目标和实验平台的差异导致不同研究者获得的硅的冲击相变压力和路径存在很大的差异，对实验结果的解释也各不相同。高应变率激光加载结合原位XRD表征实验结果表明，随着冲击压力的升高，硅的相变路径是Si-Ⅰ→Si-Ⅱ→Si-Ⅺ→Si-Ⅴ与液相的混合相→液相^[16]，该结果得到了最新的分子动力学数值模拟的印证^[22]，然而，轻气炮与动态XRD结合的实验仅给出了Si-Ⅰ→Si-Ⅴ的相变路径^[14]。

另外，相对于静高压实验，冲击加载实验伴随着显著的剪应力。在激光冲击实验中发现硅的固-固相变压力远低于静高压实验给出的结果^[16]，并认为这是由剪应力引起的。基于第一性原理的理论计算也支持剪应力显著影响硅冲击相变压力的结论，但是，轻气炮实验中却未观察到剪应力影响相变压力的证据。因此，为深入理解硅高压相变的应变率效应，仍需开展进一步的实验研究。

5.   结　论

得益于先进的可控动态加载平台和高时间分辨原位相结构表征技术，硅在不同加载应变率下的高压相变行为得到了系统的实验研究。随着加压速率的增大，硅的高压相变行为（如相界、亚稳相等）变得愈加复杂。无论是相界、相变路径，还是塑性变形机制，都呈现出显著的加载应变率相关性，但是并没有形成定量或定性的应变率依赖关系，需要进一步的实验研究。

目前，高压相变的应变率效应实验研究以及理论计算仍处于起步阶段，许多科学问题有待深入研究，如相界的应变率依赖关系、亚稳相的形成条件、相变机制的应变率依赖关系等，这些问题是地球与行星科学、材料科学、凝聚态物理等关注的核心问题，在航空航天、功能材料制备和工程等领域具有广阔的应用前景。

感谢北京高压科学研究中心林传龙研究员和中国科学院高能物理研究所杨栋亮副研究员对快速加压装置和同步辐射时间分辨X射线衍射技术的指导和支持。