Structure and Properties of Novel Superhard C5N:A First-Principles Study
doi: 10.11858/gywlxb.20170606
-
Abstract: By using the developed particle swarm optimization algorithm on the crystal structural prediction, we proposed 6 novel carbon nitride phases with a 5:1 stoichiometry.Their structures, stability, mechanical and electronic properties were investigated by first-principle calculations with the density functional theory.Our calculations indicate that P62m-C5N is energetically favorable in the 6 structures.Both elastic constants and phonon-dispersion calculations show that these structures remain mechanically and dynamically stable at 0 GPa.Electronic calculations indicate that I41-C5N is metallic while the other 5 are semiconductive.The Vickers hardness shows that all the 6 structures are superhard materials except for I41-C5N.Formation enthalpy calculations suggest that these 6 structures can be synthesized at attainable high pressures (19-83 GPa).
-
Key words:
- carbon nitride /
- first-principle calculations /
- superhard /
- high shear modulus
-
Superhard materials (Vickers hardness Hv≥40 GPa) are of fundamental interest and practical importance due to their outstanding mechanical and thermal properties, such as excellent hardness, high melting point, and wear resistance.Diamond and cubic-BN (c-BN) are two types of typical superhard materials that have been widely used in industry because of the aforementioned superior properties.However, in the past few decades, the search for new superhard materials has continued.In this context, carbon nitride has become a primary candidate for low-compressibility or superhard materials due to its relatively short bond length and low bond ionicity[1].Since the pioneering work of Liu and Cohen[2-3] that predicted hexagonal β-C3N4 with extraordinary hardness, considerable efforts have been devoted theoretically[4-14] and experimentally[15-24] to searching new stoichiometric carbon nitride materials with novel properties.On the theoretical side, several approaches have been applied to predict and design new superhard carbon nitrides with different stoichiometries, such as Pnnm-CN, P42/m-CN, α-C3N2, and bct-CN2.On the experimental side, melamine, cyanamide, and other related triazine-based compounds can be used as precursors to synthesize α-, β-, and mainly g-C3N4 through different mechanochemical techniques in the form of thin films and nanocrystals.However, these structures have been unverified because of the limited quantity and heterogeneity of the samples, thereby resulting in extensive debates.Other structure phases of carbon nitride may exist besides C3N4.Recently, Stavrou et al.[25] synthesized Pnnm-CN using single-crystal graphite and high-pressure gas-loaded N2 in a standard laser heated (LH) diamond anvil cell (DAC) configuration.This research achievement verifies the aforementioned assumption.Therefore, with the theoretical calculations guiding the research, other carbon nitrides with different stoichiometric measurements should be designed and calculated in the future.
1. Method
The crystal structure search is based on the global minimization of free energy surfaces merging ab initio total energy calculations through the particle swarm optimization (PSO) technique as implemented in the CALYPSO code[26].First-principle calculations were performed using the CASTEP[27] code based on the density functional theory[28-29].The exchange and correlation effects were described by the generalized gradient approximation-Perdew-Burke-Ernzerh (GGA-PBE) exchange-correlation functional[30].Ultrasoft pseudopotentials were expanded using a plane-wave basis set with a cutoff energy of 500 eV, and a k-point spacing (2π×0.4 nm-1) was assigned to generate Monkhorst-Pack k-point grids for Brillouin zone sampling[31].Structural optimization using the BFGS minimization method was performed until the energy change of each atom was less than 5×10-6 eV, the forces on atoms were less than 0.1 eV·nm-1, and all the stress components were less than 0.02 GPa[32].The phonon modes of the equilibrium crystal structure obtained after structural relaxation were calculated using the finite displacement theory[33].For the calculated phonon dispersion in reciprocal space, the high symmetry point coordinates were G (0, 0, 0), A (0, 0, 0.5), H (-0.33, 0.67, 0.50), K (-0.33, 0.67, 0), M (0, 0.5, 0), and L (0, 0.5, 0.5).The polycrystalline bulk modulus, shear modulus, Young's modulus, and Poisson's ratio were estimated using the Voigt-Reuss-Hill approximation[34].
2. Results and Discussions
2.1 Crystal Structure
Through the CALYPSO code, crystal structure searches were performed with a cell size of up to 48 atoms (40 C atoms and 8 N atoms) within a pressure range of 0-70 GPa.Analysis of the predicted structures gives us a shortlist of candidate structures with space groups P62m (2 formula units per cell), I41 (4 formula units per cell), Pbcn (8 formula units per cell), P63cm (8 formula units per cell), P212121 (8 formula units per cell) and Pna21 (8 formula units per cell), respectively, as shown in Fig. 1.In these novel structures, I41-C5N and P63cm-C5N are sp2-sp3 hybridized while the other 4 are all sp3 hybridized phases.The N atoms and the 3 bonded C atoms are almost coplanar in the P62m-C5N, I41-C5N, Pbcn-C5N and P63cm-C5N structures, while they form a tetrahedron in the Pna21-C5N.Furthermore, in the P212121-C5N structure, these 2 kinds of N atoms both exist.The optimized lattice parameters, atom positions, space groups, densities and cell volumes of the 6 types of C5N are obtained and listed in Table 1.
Figure 1. Predicted structure graphs of C5N (The spheres in color black and blue represent C and N atoms, respectively.)Table 1. Structure parameters of the newly predicted C5NPhase Crystal system Space group Atoms positions Lattice parameters/
nmDensity/
(g·cm-3)Cell volume/
nm3P62m-C5N Hexagonal P62m C:6i (0.6686, 0, 0.6851)
C:4h (0.3333, 0.6667, 0.1846)
N:2e (0, 0, 0.2520)a=0.42610
c=0.449043.48366 0.0706056 I41-C5N Tetragonal I41 C:8b (1.3922, 0.3047, 0.2213)
C:8b (0.8888, 0.3025, 0.5651)
C:4a (0.5000, -0.5000, 0.6649)
N:4a (0.5000, -0.5000, 0.3717)a=0.56554
c=0.461993.32925 0.147761 Pbcn-C5N Orthorhombic Pbcn C:8d (0.8394, 1.1859, 0.6653)
C:8d (0.9443, 0.5105, 1.3780)
C:8d (0.7272, 1.4193, 1.3967)
C:8d (1.4165, 1.4451, 0.5433)
C:4c (0.5000, 1.3491, 0.7500)
C:4c (0.5000, 0.8125, 0.7500)
N:8d (1.3159, 0.7079, 1.4033)a=0.69219
b=0.75985
c=0.552513.38559 0.290603 P63cm-C5N Hexagonal P63cm C:12d (0.1549, 0.4103, 0.8667)
C:12d (0.4099, 0.1542, 0.6931)
C:6c (0.7822, 0, 0.6486)
C:4b (0.3333, 0.6667, 0.1247)
C:4b (0.3333, 0.6667, 0.9413)
C:2a (0, 0, 0.4143)
N:6c (0.7847, 0, 0.9101)
N:2a (0, 0, 0.1519)a=0.61546
c=0.943323.17945 0.309444 P212121-C5N Orthorhombic P212121 C:4a (-0.4660, 0.3648, 0.0251)
C:4a (-0.0356, 0.4140, 0.7846)
C:4a (0.4082, 0.7553, 0.4943)
C:4a (-0.3026, 0.2316, 0.4965)
C:4a (-0.3414, 0.7023, 0.9899)
C:4a (0.4321, 0.3975, 0.5198)
C:4a (-0.4450, 0.6928, 0.4812)
C:4a (0.1246, 0.4389, 0.5229)
C:4a (0.3131, 0.9248, 0.4703)
C:4a (-0.0722, 0.9907, 0.4778)
N:4a (-0.2536, 0.8681, 0.9885)
N:4a (-0.2860, 0.6001, 0.0557)a=0.80449
b=1.40889
c=0.256593.3830 0.290827 Pna21-C5N Orthorhombic Pna21 C:4a (0.6262, 0.2909, 0.7966)
C:4a (0.6669, 0.4999, 0.8488)
C:4a (0.4457, 0.1191, 0.1296)
C:4a (0.3513, 0.0085, 0.1102)
C:4a (0.4412, 0.8115, 0.8592)
C:4a (0.1030, 0.1609, 0.0666)
C:4a (0.9757, 0.2005, 0.3318)
C:4a (0.2617, 0.1527, 0.5419)
C:4a (0.7486, 0.6235, 0.4587)
C:4a (0.3119, 0.0399, 0.6076)
N:4a (0.3225, 0.4796, 0.8604)
N:4a (0.7712, 0.2000, 0.7768)a=0.40299
b=1.40205
c=0.516403.3720 0.291776 | Show Table
DownLoad:
CSV
2.2 Stability Analysis
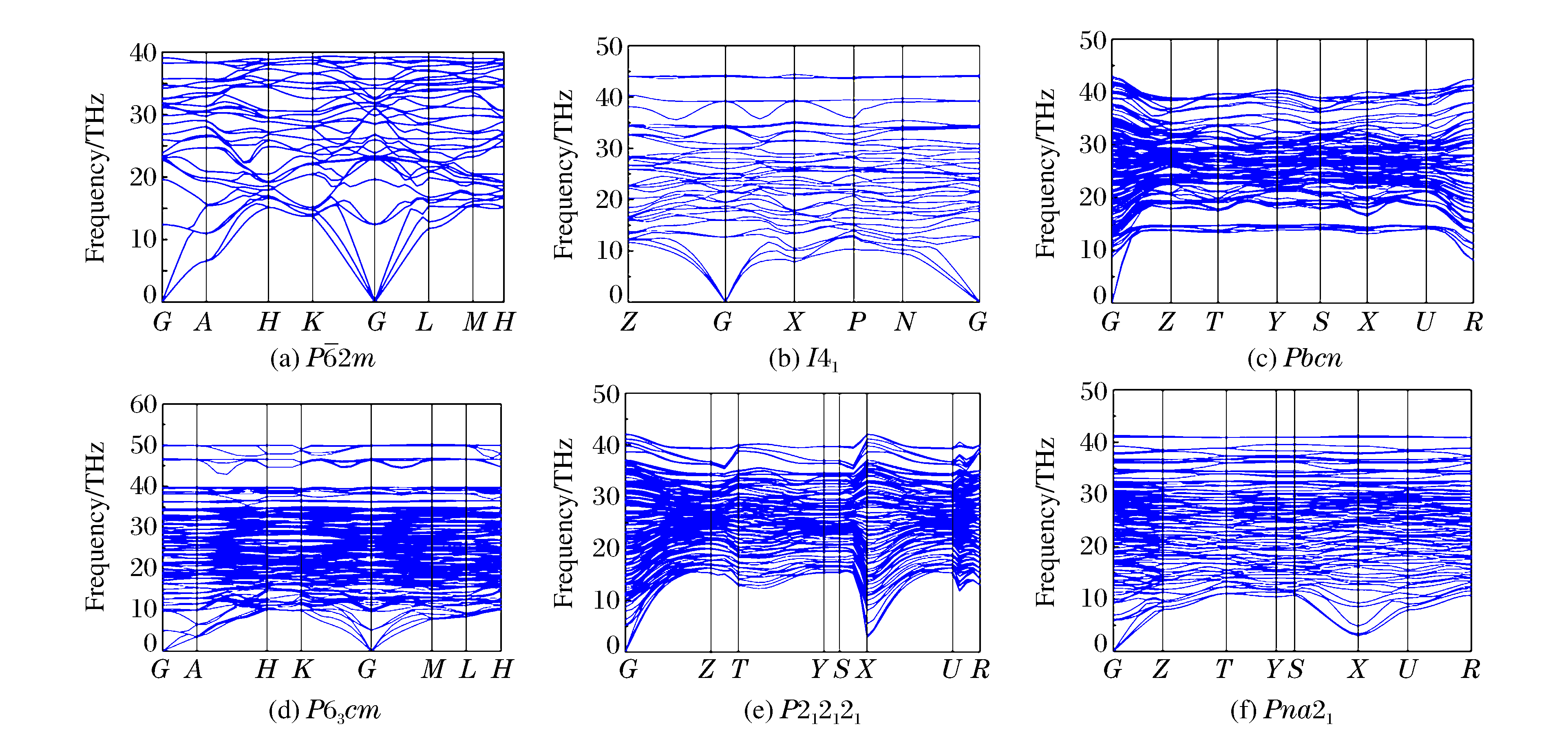
To explore the thermodynamic stability for further experimental synthesis, the formation enthalpy of the 6 novel C5N structures with respect to the separate phases is quantified by ΔHf=EC5N-5EC-(1/2)EN2, where EC5N is the ground state enthalpy of the new C5N phase, EC is the energy of C atom obtained from graphite, and EN2 is the total energy of the stable molecular α phase of nitrogen reported earlier[35].The calculated formation enthalpy of these C5N structures as functions of pressure is shown in Fig. 2. From Fig. 2, the formation enthalpies suggest that these C5N phase is metastable at 0 GPa because of its positive value, which is similar to that of diamond and c-BN.Meanwhile, as the pressure rises from 19 GPa to 83 GPa, all these 6 phases gradually become thermodynamically stable.Moreover, the use of high temperature is a classical way to improve both the diffusion processes and the reactivity of precursors for the material synthesis of bulk forms or thin films.That is, if we use graphite and nitrogen as precursors for condensed carbon nitrides at high pressure, then these C5N phases may be synthesized at readily attainable pressures and temperatures.Furthermore, our phonon calculations have verified that these 6 C5N is dynamically stable as evidenced by the absence of any imaginary frequency in the whole Brillouin zone at ambient pressure (see Fig. 3).
Figure 2. Formation enthalpy of the newly predicted C5N phases relative to graphite and nitrogenas a function of pressure
Figure 3. Phonon dispersion spectrum at 0 GPaTo evaluate the mechanical stability of a crystal structure, the elastic constants of a crystal should satisfy the generalized elastic stability criteria.For a hexagonal crystal, 5 independent elastic constants, namely, C11, C33, C44, C12, and C13, should obey the following generalized Born mechanical stability criteria[35]:C44 > 0, C11 > |C12|, and (C11+2C12)C33 > 2C132.For a tetragonal crystal, there are 6 independent elastic constants, namely, C11, C33, C44, C66, C12, and C13, and the corresponding mechanical stability criterion is given by:Cij > 0 (i=j=1-6), C11 -C12 > 0, C11 +C33 -2C13 > 0, 2(C11+C12)+C33+4C13 > 0.For an orthorhombic crystal, there are 9 independent elastic constants, namely, C11, C22, C33, C44, C55, C66, C12, C13 and C23, and the corresponding mechanical stability criterion is as follow:Cij > 0 (i=j=1-6), C11+C22+C33+ 2(C12+C13+C23) > 0, C11+C22-2C12 > 0, C11+C33-2C13 > 0, C22+C33-2C23 > 0.The calculated elastic constant values of C5N phases by the strain-stress method are listed in Table 2.According to the above criteria, the calculation results indicate that these novel structures are all mechanically stable under ambient pressure.
Table 2. Calculated independent elastic constants Cij of the newly predicted C5NPhase C11/
GPaC22/
GPaC33/
GPaC44/
GPaC55/
GPaC66/
GPaC12/
GPaC13/
GPaC15/
GPaC23/
GPaC25/
GPaP62m-C5N 1194 930 252 113 18 I41-C5N 649 931 244 247 244 75 Pbcn-C5N 762 663 822 382 360 364 190 83 165 P63cm-C5N 873 490 192 170 35 P212121-C5N 902 847 893 299 341 347 120 93 40 902 847 Pna21-C5N 891 659 736 289 372 324 86 153 149 891 659 | Show TableDownLoad:
CSV
2.3 Properties
Based on the Voigt-Reuss-Hill approximation, bulk modulus (B), shear modulus (G), Young's modulus (E) and Poisson's ratio (σ) can be obtained from the calculated elastic constants.The calculated B, G, E and σ of the newly predicted C5N are listed in Table 3.Bulk modulus is a measure of the resistance against volume change imposed by the applied pressure, while shear modulus represents the resistance to the shear deformation against external forces, which indicates the resistance to the change in the bond angle.The hardness is deduced from the size of the indentation after deformation.A superhard material typically requires a high bulk modulus to support the volume decrease created by the applied pressure and a high shear modulus so that the material will not deform in a direction different from that of the applied load.From Table 3, it is obvious that both B and G of P62m-C5N are the largest among the 6 new structures, indicating it can withstand stronger compression and higher shear stress than the other structures.
Table 3. Calculated bulk modulus B, shear modulus G, Young's modulus E and Vickers hardness Hv ofthe newly predicted C5N (Also shown are G/B ratio and Poisson's ratio σ)Phase B/GPa G/GPa E/GPa σ G/B Hv/GPa P62m-C5N 397 394 888 0.127 0.992 62.5 I41-C5N 335 238 577 0.213 0.711 30.0 Pbcn-C5N 347 338 765 0.133 0.973 55.0 P63cm-C5N 286 267 611 0.144 0.934 44.5 P212121-C5N 349 354 794 0.121 1.014 59.6 Pna21-C5N 338 321 731 0.139 0.949 51.6 | Show TableDownLoad:
CSV
The relative directionality of covalent bonds of materials also has an important effect on their hardness and mechanical properties, which can be determined by the G/B ratio[36].Table 3 shows that the G/B ratio of P212121-C5N (1.014) is higher than that of the other 5 C5N phases, thereby suggesting the stronger directional bonding feature of P212121-C5N.The relative orientation of material bonding also has an important effect on their hardness.Young's modulus (E) is defined as the ratio between stress and strain and is used to provide a measure of stiffness of the solid, i.e., the larger the value of E, the stiffer the material is.Poisson's ratio (σ) quantifies the stability of the crystal against shear.Except for I41-C5N, the fairly large value of E and small Poisson's ratio indicate that the other 5 C5N phases are rather stiff and relatively stable against shear.Thus, the preceding results reveal that these new C5N phases are potential candidates for superhard materials and this will be confirmed by our following calculations of the Vickers hardness.
The electronic structure determines the fundamental physical and chemical properties of materials.The calculated electronic band structure of the new predicted C5N phases at 0 GPa are presented in Fig. 4.From Fig. 4, we can find that I41-C5N are metallic, Pbcn-C5N and Pna21-C5N are direct-bandgap semiconductors while P62m-C5N, P63cm-C5N and P212121-C5N are indirect-bandgap semiconductors.All these 5 semiconductors are narrow bandgap semiconductors and their bandgaps are 1.131, 0.971, 2.312, 0.796 and 1.088 eV, respectively.The narrow bandgap feature suggests that they may be used as photocatalyst.In addition, the direct bandgap feature indicates Pbcn-C5N and Pna21-C5N are promising materials for the solar cell or photography industry.
Figure 4. Electronic band structures of newly predicted C5N at 0 GPaIn order to figure out the theoretical Vickers hardness of the new C5N structures, we employed the macroscopic hardness model proposed by Chen et al.[37] and revised by Tian et al.[38].The formula is Hv(GPa)=0.92k1.137G0.708, where k is the Pugh modulus ratio and equal to G/B.The results are shown in Table 3.We can find that these new compounds are superhard materials except for I41-C5N and the hardness of P62m-C5N and P212121-C5N are even close to that of c-BN.
3. Conclusions
In summary, using the developed PSO technique on the crystal structure prediction, we predicted 6 types of C5N structures, and systematically investigated their geometry structures, stability, electronic and mechanical properties by first-principle calculations based on the density functional theory.All these new phases are metastable at ambient pressure but become energetically more stable than graphite and N2 under high pressures (19-83 GPa).In addition, they are proved to be mechanically and dynamically stable at ambient pressure by computing their elastic constants and phonon dispersions.Furthermore, except for I41-C5N, the other 5 phases are superhard semiconductors as well.The present work has great implications for designing and researching novel superhard materials.
-
Figure 1. Predicted structure graphs of C5N (The spheres in color black and blue represent C and N atoms, respectively.)
Figure 2. Formation enthalpy of the newly predicted C5N phases relative to graphite and nitrogenas a function of pressure
Table 1. Structure parameters of the newly predicted C5N
Phase Crystal system Space group Atoms positions Lattice parameters/
nmDensity/
(g·cm-3)Cell volume/
nm3P62m-C5N Hexagonal P62m C:6i (0.6686, 0, 0.6851)
C:4h (0.3333, 0.6667, 0.1846)
N:2e (0, 0, 0.2520)a=0.42610
c=0.449043.48366 0.0706056 I41-C5N Tetragonal I41 C:8b (1.3922, 0.3047, 0.2213)
C:8b (0.8888, 0.3025, 0.5651)
C:4a (0.5000, -0.5000, 0.6649)
N:4a (0.5000, -0.5000, 0.3717)a=0.56554
c=0.461993.32925 0.147761 Pbcn-C5N Orthorhombic Pbcn C:8d (0.8394, 1.1859, 0.6653)
C:8d (0.9443, 0.5105, 1.3780)
C:8d (0.7272, 1.4193, 1.3967)
C:8d (1.4165, 1.4451, 0.5433)
C:4c (0.5000, 1.3491, 0.7500)
C:4c (0.5000, 0.8125, 0.7500)
N:8d (1.3159, 0.7079, 1.4033)a=0.69219
b=0.75985
c=0.552513.38559 0.290603 P63cm-C5N Hexagonal P63cm C:12d (0.1549, 0.4103, 0.8667)
C:12d (0.4099, 0.1542, 0.6931)
C:6c (0.7822, 0, 0.6486)
C:4b (0.3333, 0.6667, 0.1247)
C:4b (0.3333, 0.6667, 0.9413)
C:2a (0, 0, 0.4143)
N:6c (0.7847, 0, 0.9101)
N:2a (0, 0, 0.1519)a=0.61546
c=0.943323.17945 0.309444 P212121-C5N Orthorhombic P212121 C:4a (-0.4660, 0.3648, 0.0251)
C:4a (-0.0356, 0.4140, 0.7846)
C:4a (0.4082, 0.7553, 0.4943)
C:4a (-0.3026, 0.2316, 0.4965)
C:4a (-0.3414, 0.7023, 0.9899)
C:4a (0.4321, 0.3975, 0.5198)
C:4a (-0.4450, 0.6928, 0.4812)
C:4a (0.1246, 0.4389, 0.5229)
C:4a (0.3131, 0.9248, 0.4703)
C:4a (-0.0722, 0.9907, 0.4778)
N:4a (-0.2536, 0.8681, 0.9885)
N:4a (-0.2860, 0.6001, 0.0557)a=0.80449
b=1.40889
c=0.256593.3830 0.290827 Pna21-C5N Orthorhombic Pna21 C:4a (0.6262, 0.2909, 0.7966)
C:4a (0.6669, 0.4999, 0.8488)
C:4a (0.4457, 0.1191, 0.1296)
C:4a (0.3513, 0.0085, 0.1102)
C:4a (0.4412, 0.8115, 0.8592)
C:4a (0.1030, 0.1609, 0.0666)
C:4a (0.9757, 0.2005, 0.3318)
C:4a (0.2617, 0.1527, 0.5419)
C:4a (0.7486, 0.6235, 0.4587)
C:4a (0.3119, 0.0399, 0.6076)
N:4a (0.3225, 0.4796, 0.8604)
N:4a (0.7712, 0.2000, 0.7768)a=0.40299
b=1.40205
c=0.516403.3720 0.291776 
下载: 导出CSV
Table 2. Calculated independent elastic constants Cij of the newly predicted C5N
Phase C11/
GPaC22/
GPaC33/
GPaC44/
GPaC55/
GPaC66/
GPaC12/
GPaC13/
GPaC15/
GPaC23/
GPaC25/
GPaP62m-C5N 1194 930 252 113 18 I41-C5N 649 931 244 247 244 75 Pbcn-C5N 762 663 822 382 360 364 190 83 165 P63cm-C5N 873 490 192 170 35 P212121-C5N 902 847 893 299 341 347 120 93 40 902 847 Pna21-C5N 891 659 736 289 372 324 86 153 149 891 659
下载: 导出CSV
Table 3. Calculated bulk modulus B, shear modulus G, Young's modulus E and Vickers hardness Hv ofthe newly predicted C5N (Also shown are G/B ratio and Poisson's ratio σ)
Phase B/GPa G/GPa E/GPa σ G/B Hv/GPa P62m-C5N 397 394 888 0.127 0.992 62.5 I41-C5N 335 238 577 0.213 0.711 30.0 Pbcn-C5N 347 338 765 0.133 0.973 55.0 P63cm-C5N 286 267 611 0.144 0.934 44.5 P212121-C5N 349 354 794 0.121 1.014 59.6 Pna21-C5N 338 321 731 0.139 0.949 51.6
下载: 导出CSV
-
[1] LÉGER J-M, HAINES J.The search for superhard materials[J]. Endeavour, 1997, 21(3):121-124. doi: 10.1016/S0160-9327(97)80221-9 [2] LIU A Y, COHEN M L.Prediction of new low compressibility solids[J]. Science, 1989, 245(4920):841-842. doi: 10.1126/science.245.4920.841 [3] LIU A Y, COHEN M L.Structural properties and electronic structure of low-compressibility materials:β-Si3N4 and hypothetical β-C3N4[J]. Physical Review B, 1990, 41(15):10727-10734. doi: 10.1103/PhysRevB.41.10727 [4] CÔTÉAND M, COHEN M L.Carbon nitride compounds with 1:1 stoichiometry[J]. Physical Review B, 1997, 55(9):5684. doi: 10.1103/PhysRevB.55.5684 [5] HALES J, BARNARD A S.Thermodynamic stability and electronic structure of small carbon nitride nanotubes[J]. Journal of Physics:Condensed Matter, 2009, 21(14):144203. doi: 10.1088/0953-8984/21/14/144203 [6] KIM E, CHEN C, KÖHLER T, et al.Tetragonal crystalline carbon nitrides:theoretical predictions[J]. Physical Review Letters, 2001, 86(4):652-655. doi: 10.1103/PhysRevLett.86.652 [7] KIM E, CHEN C.Stability of tetragonal crystalline carbon nitrides:the nitrogen content dependence[J]. Physics Letters A, 2001, 282(6):415-420. doi: 10.1016/S0375-9601(01)00200-6 [8] HART J N, CLAEYSSENS F, ALLAN N L, et al.Carbon nitride:ab initio investigation of carbon-rich phases[J]. Physical Review B, 2009, 80(17):174111. doi: 10.1103/PhysRevB.80.174111 [9] SANDRÉ É, PICKARD C J, COLLIEX C.What are the possible structures for CNx ompounds?The example of C3N[J]. Chemical Physics Letters, 2000, 325(1/2/3):53-60. http://www.sciencedirect.com/science/article/pii/S000926140000539X [10] TIAN F, WANG J, HE Z, et al.Superhard semiconducting C3N2 compounds predicted via first-principles calculations[J]. Physical Review B, 2008, 78(23):235431. doi: 10.1103/PhysRevB.78.235431 [11] WANG X.Polymorphic phases of sp3-hybridized superhard CN[J]. The Journal of Chemical Physics, 2012, 137(18):184506. doi: 10.1063/1.4765324 [12] ZHANG M, WEI Q, YAN H, et al.A novel superhard tetragonal carbon mononitride[J]. The Journal of Physical Chemistry C, 2014, 118(6):3202-3208. doi: 10.1021/jp409152t [13] LI Q, LIU H, ZHOU D, et al.A novel low compressible and superhard carbon nitride:body-centered tetragonal CN2[J]. Physical Chemistry Chemical Physics, 2012, 14(37):13081-13087. doi: 10.1039/c2cp41694h [14] XU Y, GAO S P.Band gap of C3N4 in the GW approximation[J]. International Journal of Hydrogen Energy, 2012, 37(15):11072-11080. doi: 10.1016/j.ijhydene.2012.04.138 [15] GUO L P, CHEN Y, WANG E G, et al.Identification of a new tetragonal C-N phase[J]. Journal of Crystal Growth, 1997, 178(4):639-644. doi: 10.1016/S0022-0248(96)01067-6 [16] SEKINE T, KANDA H, BANDO Y, et al.A graphitic carbon nitride[J]. Journal of Materials Science Letters, 1990, 9(12):1376-1378. doi: 10.1007/BF00721588 [17] STEVENS A J, KOGA T, AGEE C B, et al.Stability of carbon nitride materials at high pressure and temperature[J]. Journal of the American Chemical Society, 1996, 118(44):10900-10901. doi: 10.1021/ja9625182 [18] STEVENS A J, AGEE C B, LIEBER C M. High-pressure chemistry of carbon nitride materials[J/OL]. MRS Online Proceedings Library, 1997, 499: 309(2011-02-01)[2017-06-29]. https://doi.org/10.1557/PROC-499-309. [19] CHEN Y A, GUO L, WANG E G.Alpha beta experimental evidence for alpha-and beta-phases of pure crystalline C3N4 in films deposited on nickel substrates[J]. Philosophical Magazine Letters, 1997, 75(3):155-162. doi: 10.1080/095008397179714 [20] NIU C, LU Y Z, LIEBER C M.Experimental realization of the covalent solid carbon nitride[J]. Science, 1993, 261(5119):334-337. doi: 10.1126/science.261.5119.334 [21] YU K M, COHEN M L, HALLER E E, et al.Observation of crystalline C3N4[J]. Physical Review B, 1994, 49(7):5034-5037. doi: 10.1103/PhysRevB.49.5034 [22] YIN L W, LI M S, LIU Y X, et al.Synthesis of beta carbon nitride nanosized crystal through mechanochemical reaction[J]. Journal of Physics:Condensed Matter, 2003, 15(2):309-314. doi: 10.1088/0953-8984/15/2/330 [23] GUO Q, XIE Y, WANG X, et al.Characterization of well-crystallized graphitic carbon nitride nanocrystallites via a benzene-thermal route at low temperatures[J]. Chemical Physics Letters, 2003, 380(1/2):84-87. https://www.sciencedirect.com/science/article/pii/S0009261403015525 [24] JÜRGENS B, IRRAN E, SENKER J, et al.Melem (2, 5, 8-triamino-tri-s-triazine), an important intermediate during condensation of melamine rings to graphitic carbon nitride:synthesis, structure determination by X-ray powder diffractometry, solid-state NMR, and theoretical studies[J]. Journal of the American Chemical Society, 2003, 125(34):10288-10300. doi: 10.1021/ja0357689 [25] STAVROU E, LOBANOV S, DONG H, et al.Synthesis of ultra-incompressible sp3-hybridized carbon nitride with 1:1 stoichiometry[J]. Chemistry of Materials, 2016, 28(19):6925-6933. doi: 10.1021/acs.chemmater.6b02593 [26] WANG Y, LV J, ZHU L, et al.Crystal structure prediction via particle-swarm optimization[J]. Physical Review B, 2010, 82(9):094116. doi: 10.1103/PhysRevB.82.094116 [27] SEGALL M D, PHILIP J D L, PROBERT M J, et al.First-principles simulation:ideas, illustrations and the CASTEP code[J]. Journal of Physics:Condensed Matter, 2002, 14(11):2717-2744. doi: 10.1088/0953-8984/14/11/301 [28] KOHN W, SHAM L J.Self-consistent equations including exchange and correlation effects[J]. Physical Review A, 1965, 140(4):1133-1138. http://tu-freiberg.de/sites/default/files/media/institut-fuer-theoretische-physik-10451/Lehre/Dichtefunktionaltheorie/a9rf1a3.pdf [29] HOHENBERG P, KOHN W.Inhomogeneous electron gas[J]. Physical Review B, 1964, 136(3):864-871. http://www.researchgate.net/publication/235563075_Inhomogeneous_Electron_Gas [30] JONES R O, GUNNARSSON O.The density functional formalism, its applications and prospects[J]. Reviews of Modern Physics, 1989, 61(3):689-746. doi: 10.1103/RevModPhys.61.689 [31] MONKHORST H J, PACK J D.Special points for Brillouin-zone integrations[J]. Physical Review B, 1976, 13(12):5188-5192. doi: 10.1103/PhysRevB.13.5188 [32] FISCHER T H, ALMLOF J.General methods for geometry and wave function optimization[J]. The Journal of Physical Chemistry, 1992, 96(24):9768-9774. doi: 10.1021/j100203a036 [33] CLARK S J, SEGALL M D, PICKARD C J, et al.First principles methods using CASTEP[J]. Zeitschrift für Kristallographie-Crystalline Materials, 2005, 220(5/6):567-570. http://ci.nii.ac.jp/naid/10026643729 [34] HILL R.The elastic behaviour of a crystalline aggregate[J]. Proceedings of the Physical Society:Section A, 1952, 65(5):349. doi: 10.1088/0370-1298/65/5/307 [35] VENABLES J A, ENGLISH C A.Electron diffraction and the structure of α-N2[J]. Acta Crystallographica Section B:Structural Science, Crystal Engineering and Materials, 1974, 30:929-935. doi: 10.1107/S0567740874004067 [36] WU Z J, ZHAO E J, XIANG H P, et al.Crystal structures and elastic properties of superhard IrN2 and IrN3 from first principles[J]. Physical Review B, 2007, 76(5):054115. doi: 10.1103/PhysRevB.76.054115 [37] CHEN X Q, NIU H, LI D, et al.Modeling hardness of polycrystalline materials and bulk metallic glasses[J]. Intermetallics, 2011, 19(9):1275-1281. doi: 10.1016/j.intermet.2011.03.026 [38] TIAN Y, XU B, ZHAO Z.Microscopic theory of hardness and design of novel superhard crystals[J]. International Journal of Refractory Metals and Hard Materials, 2012, 33:93-106. doi: 10.1016/j.ijrmhm.2012.02.021 -
DownLoad:
下载:
点击查看大图
计量
- 文章访问数: 8685
- HTML全文浏览量: 2863
- PDF下载量: 145
