应用于高压科学研究的国产铰链式六面顶压机技术发展历程

彭放; 贺端威

doi:10.11858/gywlxb.20170600

应用于高压科学研究的国产铰链式六面顶压机技术发展历程

doi: 10.11858/gywlxb.20170600

彭放,
贺端威^*, ,

四川大学原子与分子物理研究所，四川成都 610065

详细信息

作者简介:
彭放(1960-), 男, 博士, 教授, 博士生导师, 主要从事高压科学与凝聚态物理研究. E-mail:pengfang@scu.edu.cn

通讯作者:
贺端威(1969-), 男, 博士, 教授, 博士生导师, 主要从事高压物理、超硬材料、大腔体静高压技术研究.E-mail:duanweihe@scu.edu.cn

中图分类号: O521.3
计量
- 文章访问数: 8447
- HTML全文浏览量: 2936
- PDF下载量: 743
出版历程
- 收稿日期: 2017-06-26
- 修回日期: 2017-09-26

Development of Domestic Hinge-Type Cubic Presses Based on High Pressure Scientific Research

PENG Fang,
HE Duanwei^{*
, ,}

Institute of Atomic and Molecular Physics, Sichuan University, Chengdu 610065, China

摘要

摘要: 国产铰链式六面顶压机是我国独立发展起来的大腔体高压装置，经过50多年的不断发展，在工业高压合成和高压科学研究领域取得了丰硕成果，在国际上占据一席之地。本文仅以四川大学高压科学与技术实验室在国产铰链式六面顶压机应用于高压科学研究的技术研发为镜，展示国产铰链式六面顶压机大腔体高压装置在应用于高压科学研究领域的发展历程和技术特点，以利于该装置在今后的研发过程中继续保持和发挥其独特性能，为我国工业高压合成和高压科学研究发挥更大的作用。
- 六面顶压机 /
- 高压科学与技术 /
- 大腔体压机 /
- 超硬材料
Abstract: The China-made hinge-type large volume cubic press is a high-pressure device independently developed in China.After 50 years of continuous development, it has achieved fruitful results in industrial synthesis and high-pressure scientific research and occupied a special place in the world.In this paper, we take the topic of China-made hinge-type large volume cubic press used in high-pressure scientific research at the High Pressure Science and Technology Laboratory of Sichuan University, and demonstrate its development and technical characteristics.The technical performance of the device will be continuously improved in the development process in the future, so that it will play a greater role in China's industrial high-pressure synthesis and high-pressure scientific research.
- cubic press /
- high pressure science and technology /
- large volume press /
- superhard material

HTML全文

The corrugated interface between different fluids grow when accelerated from a low-density fluid to a high-density fluid, which is called Rayleigh-Taylor (RT) instability^[1-2].This phenomenon may also occur in metals, but usually under a high pressure and at a high strain-rate, but differs most distinctly from the RT instability in fluids in its strength effect of the metal, which can stabilize the perturbation growth^[3-4] and make the metallic RT instability more complex and difficult.Here, it is also affected by the loading state and the properties of the metallic materials.The metallic RT instability at high pressure and strain-rate can be observed in inertial confinement fusion^[5], supernova explosion^[6], asteroid collision^[7], the motion of earth's inner core and plate tectonics^[8], and so on.Therefore, the metallic RT instability is currently a major concern for researchers and receives a great deal of academic attention.

In theoretical studies about the metallic interface instability, dispersion relations of the perturbation growth are derived mainly based on the energy^[9-11] or force equilibrium^[12-13].However, the previous linear analysis cannot predict the perturbation growth accurately just by applying the perfect plastic constitutive relation and constant pressure loading state.Based on the energy balance, a perturbation growth equation using Steinberg-Guinan (SG) and the Johnson-Cook constitutive models, as well as a variable pressure loading process consistent with experiments, has been derived that precisely predicts the growth of metallic RT instabilities driven by detonation and laser plasma.However, the linear analysis still has its limitations and does not take full account of the loading procedure.

Experimental studies of the metallic RT instability started in the 1970s.The pioneering experimental research^[14] was the perturbation growth of a flat aluminum plate accelerated by the expansion of detonation products, which was observed using a high-energy X-ray facility.What was achieved then inspired researchers, and the similar equipment was utilized in later research^[15-17].In the USA and Russia particularly, numerous numerical simulations and experimental investigations for the metallic RT instability have been carried out, but have mainly concentrated on the perturbation growth and such influencing factors as the initial amplitude, the wavelength and material properties.Igonin and Ignatova et al.^[18-19] experimentally and numerically studied the dynamic behaviors of copper (Cu) and tantalum (Ta) subjected to both shock and shockless loading by employing a perturbation growth method.They observed that the formation of the bi-periodic twin structures resulted in an initial loss of the shear strength of Cu, but failed to observe localization in Ta.Olson et al.^[16] experimentally studied the effects of the grain size and material processing on the RT perturbation growth of Cu.They found that both the single-crystal orientation and the strain hardening due to the material processing can affect the perturbation growth, but the polycrystalline grain size cannot.For the plane detonation, the loading pressure is generally about 30 GPa.To enhance the loading pressure, Henry de Frahan et al.^[17] studied the beryllium RT instability using an iron flyer plate to impact the second high explosive (HE) to raise the pressure to 50 GPa in their experiments, and combined numerical simulations to calibrate the feasibility of different constitutive models.When the sample is driven by electromagnetism^[20-21] or laser^{[4, 22]}, the loading pressure can be further increased.Very extreme conditions of pressures over 1 000 GPa and strain rates of 10⁸ s^-1 have been achieved at the National Ignition Facility, USA, where the RT instability experiment in vanadium was carried out, and constitutive models in solid phase were tested by comparing simulations with experiments measuring the perturbation growth^[23] under the extreme conditions mentioned.

In the metallic interface instability, the perturbation growth is related to and arrested by the material strength.Moreover, some investigations have demonstrated that the material strength increases under these extreme conditions.Results from the metallic RT experiments and computations by Barnes et al.^[14] show that the yield strength of 1100-0 aluminum is over 10 times larger than the standard parameter, and the yield strength of 304 stainless steel also increases by more than three times.Using the SG constitutive strength model, calculations of plasma-driven quasi-isentropic RT experiments of Al-6061-T6 using the Omega laser at a peak drive pressure of 20 GPa indicate that its yield strength is a factor of about 3.6 times over the ambient value^[22].In Park et al.'s^[4] plasma-driven quasi-isentropic polycrystalline vanadium RT experiments using the Omega laser with a peak drive pressure of 100 GPa, the measured RT growth was substantially lower than predictions using the existing constitutive models (SG and Preston-Tonks-Wallace) that work well at low pressures and long timescales.Using the SG model, the simulations agree with the RT experimental data when the initial strength is raised by a factor of 2.3.Therefore, the SG and Preston-Tonks-Wallace models underestimate the strength of vanadium under very high pressures and strain rates.Belof et al.^[24] first measured the dynamic strength of iron undergoing solid-solid phase transition by using RT instability.In conjunction with detailed hydrodynamic simulations, the analysis results revealed significant strength enhancement of the dynamically generated ε-Fe and reverted α′-Fe, comparable in magnitude to the strength of austenitic stainless steels.Therefore, the metallic RT instability was suggested and used as a tool for evaluating the material strength of solids at high pressures and high strain rates^{[3, 25]}, and then for modifying or developing new constitutive models for these conditions^[26-27].

In view of the dominant role of the material strength in metallic interface instabilities, and the limitations of existing constitutive models at high pressures and high strain rates, we aimed to investigate the material strength and its effects on metallic interface instabilities.In this paper, we also conducted an RT instability experiment in explosion-driven aluminum, and measured the perturbation growth using X-ray radiography.In combination with elastic-plastic hydrodynamic simulations, we investigated the dynamic behavior of metallic RT instabilities and the role of the material strength in these.

1. Experimental Setup

Following that of Barnes et al., ^[14]our experiment used the setup as shown in Fig. 1(a), where we have a sketch of the experimental setup consisting of a detonator, a booster, plane wave lens, JO-9159 HE (100 mm in diameter and 50 mm in thickness), an aluminum sample, and a vacuum.Fig. 1(b) shows the experimental sample of aluminum with a diameter of 65 mm and a thickness of 1.5 mm in the central region.An initial sinusoidal perturbation was machined on the side of the aluminum sample facing the HE.The perturbation amplitude and wavelength were 0.25 mm and 6 mm, respectively.The HE products crossed the void of 3.5 mm between the sample and HE and accumulated on the perturbation interface of the sample, providing a smooth rise to peak pressure and a quasi-isentropic drive.Moreover, the void between the sample and HE can ensure that the temperature of the sample at high pressures remain below the melting point^[22].

Figure 1. Sketch of the experimental setup and sample

DownLoad: Full-Size Img PowerPoint

In the experiment, X-ray radiography was used to record the evolution of the perturbation interface from the JO-9159 explosive detonation at zero time.A Doppler pin system was used to measure the history of the free surface velocity, which can be integrated to obtain the corresponding free surface displacement.Fig. 1(c) shows the distribution of measurement points of the free surface velocity, where both points 1 and 2 correspond to the wave trough positions with one wavelength interval, and point 3 corresponds to the wave crest position with 1.5 wavelength intervals from point 2.

2. Numerical Methods

Based on our indoor hydrodynamic code of compressible multi-viscous flow and turbulence (MVFT)^[28-30], we developed a detonation and shock dynamics code with high fidelity by considering the explosive detonation and the elastic-plastic behavior of the material.This code can be used to study the physical problem of multi-materials, large deformation, and strong shock.The governing equations in conserved form are as follows

$\left\{ \begin{array}{l} \frac{\partial }{{\partial t}}\int_V {\rho {\rm{d}}V} =-\oint_S {\rho {u_i}{n_i}{\rm{d}}S} \\ \frac{\partial }{{\partial t}}\int_V {\rho {u_j}{\rm{d}}V} =-\oint_S {P{n_j}{\rm{d}}S}-\oint_S {\rho {u_i}{u_j}{n_i}{\rm{d}}S} + \oint_S {{s_{ij}}{n_i}{\rm{d}}S} \\ \frac{\partial }{{\partial t}}\int_V {\rho E{\rm{d}}V} = - \oint_S {{u_i}P{n_i}{\rm{d}}S} - \oint_S {\rho {u_i}E{n_i}{\rm{d}}S} + \oint_S {{s_{ij}}{u_j}{n_i}{\rm{d}}S} \end{array} \right.$

(1)

where i and j represent the x, y, and z directions; V is the control volume, S the surface of control volume, n the unit vector of the external normal direction, ρ, u_k (where k=i, j), p, and E are the density, velocity, pressure, and total energy of per unit mass; and s_ij the deviation stress tensor.

The physical problem as described by Eq.(1) was decomposed into three one-dimensional problems.For each of them, the physical quantities in a cell were interpolated and reconstructed using a piecewise parabolic method (PPM).The one-dimensional problem was then resolved using a two-step Euler algorithm:First the physical quantities were solved by the Lagrange matching, and then remapped back to the stationary Euler meshes.The effect of material strength, explosive detonation, and artificial viscosity were implemented in the Lagrange step.The multi-material interface was captured by applying a volume-of-fluid (VOF) method.

2.1 Equation of State

In our numerical simulations, the equation of state (EOS) for the explosive and aluminum are the Jones-Wilkins-Lee (JWL) and Mie-Grüneisen equations of state, respectively.The Jones-Wilkins-Lee equation of state is

$p\left( {\rho, T} \right) = A\left( {1-\frac{\omega }{{{R_1}v}}} \right){{\rm{e}}^{-{R_1}v}} + B\left( {1-\frac{\omega }{{{R_2}v}}} \right){{\rm{e}}^{ - {R_2}v}} + \frac{{\omega \bar E}}{v}$

(2)

where v=ρ₀/ρ is specific volume; A, B, R₁, R₂, and ω are constants; and E is the internal energy per unit volume.Table 1 lists the JWL EOS parameters of the JO-9159 explosive.The Mie-Grüneisen equation of state is

Table 1. Equation of state parameters of JO-9159 explosive

ρ/(g·cm^-3)	p_CJ/GPa	D_CJ/(km·s^-1)	A/GPa	B/GPa	R₁	R₂	ω
1.86	36	8.862	934.8	12.7	4.6	1.1	0.37

| Show Table

DownLoad: CSV

$p = \frac{{{\rho _0}{c^2}\mu \left[{1 + \left( {1-{\gamma _0}/2} \right)\mu-a{\mu ^2}/2} \right]}}{{{{\left[{1-\left( {{S_1}-1} \right)\mu-\frac{{{S_2}{\mu ^2}}}{{\mu + 1}} - \frac{{{S_3}{\mu ^3}}}{{{{\left( {\mu + 1} \right)}^2}}}} \right]}^2}}} + \left( {{\gamma _0} + a\mu } \right)\bar E$

(3)

where μ=ρ/ρ₀-1 is the relative compression, ρ₀ the initial density, c the sound velocity at zero pressure, γ₀ the Grüneisen coefficient, and a, S₁, S₂, and S₃ are constants (in Table 2).

Table 2. Mie-Grüneisen equation of state parameters of aluminum

ρ/(g·cm^-3)	c/(km·s^-1)	γ₀	a	S₁	S₂	S₃
2.703	5.22	1.97	0.47	1.37	0	0

| Show Table

DownLoad: CSV

2.2 Constitutive Model

In our simulations, the elastic-plastic behavior of aluminum at high pressures and high strain rates was described using the SG constitutive model.The SG model introduces pressure, temperature, and strain-rate terms into the elastic-plastic constitutive equation, while the coupling effect of pressure and strain rate on flow stress was characterized by the separating variables.Additionally, as the flow stress in the SG model relies on pressure, there is a coupling relationship between the material constitutive equation and the equation of state, which indicates the feature of pressure hardening of metal under high pressure.The dynamic yield strength Y_SG and the shear modulus G determined by the SG model are expressed as

${Y_{{\rm{SG}}}} = {Y_0}{\left[{1 + \beta \left( {{\varepsilon _p} + {\varepsilon _i}} \right)} \right]^n}\left[{1 + Ap{\eta ^{-1/3}}-B\left( {T-300} \right)} \right]$

(4)

$G = {G_0}\left[{1 + Ap{\eta ^{-1/3}}-B\left( {T-300} \right)} \right]$

(5)

where Y₀ and G₀ are the initial yield strength and the shear modulus, respectively; β and n are the material strain hardening coefficient and the hardening index, respectively; A is the pressure hardening coefficient; η=ρ/ρ₀ is the material compression ratio; and B is the temperature softening coefficient (in Table 3).

Table 3. Steinberg-Guinan constitutive model parameters of aluminum

Y₀/GPa	Y_max/GPa	G₀/GPa	β	n	A/GPa^-1	B/(10^-3K^-1)
0.29	0.68	27.6	125	0.1	0.0652	0.616

| Show Table

DownLoad: CSV

3. Results and Discussions

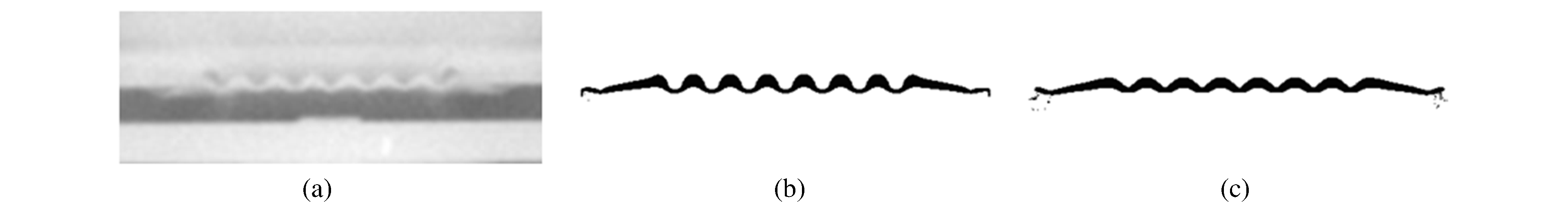
In our experiment, X-ray radiography recorded an image of the perturbed interface at 7.78 μs, as shown in Fig. 2(a), from which we obtained the amplitude of 0.77 mm simultaneously by image processing.In the simulations, the mesh size was 15.6 μm, and Tables 2 and 3 list the parameters of the Mie-Grüneisen EOS and the SG constitutive model of aluminum, respectively.

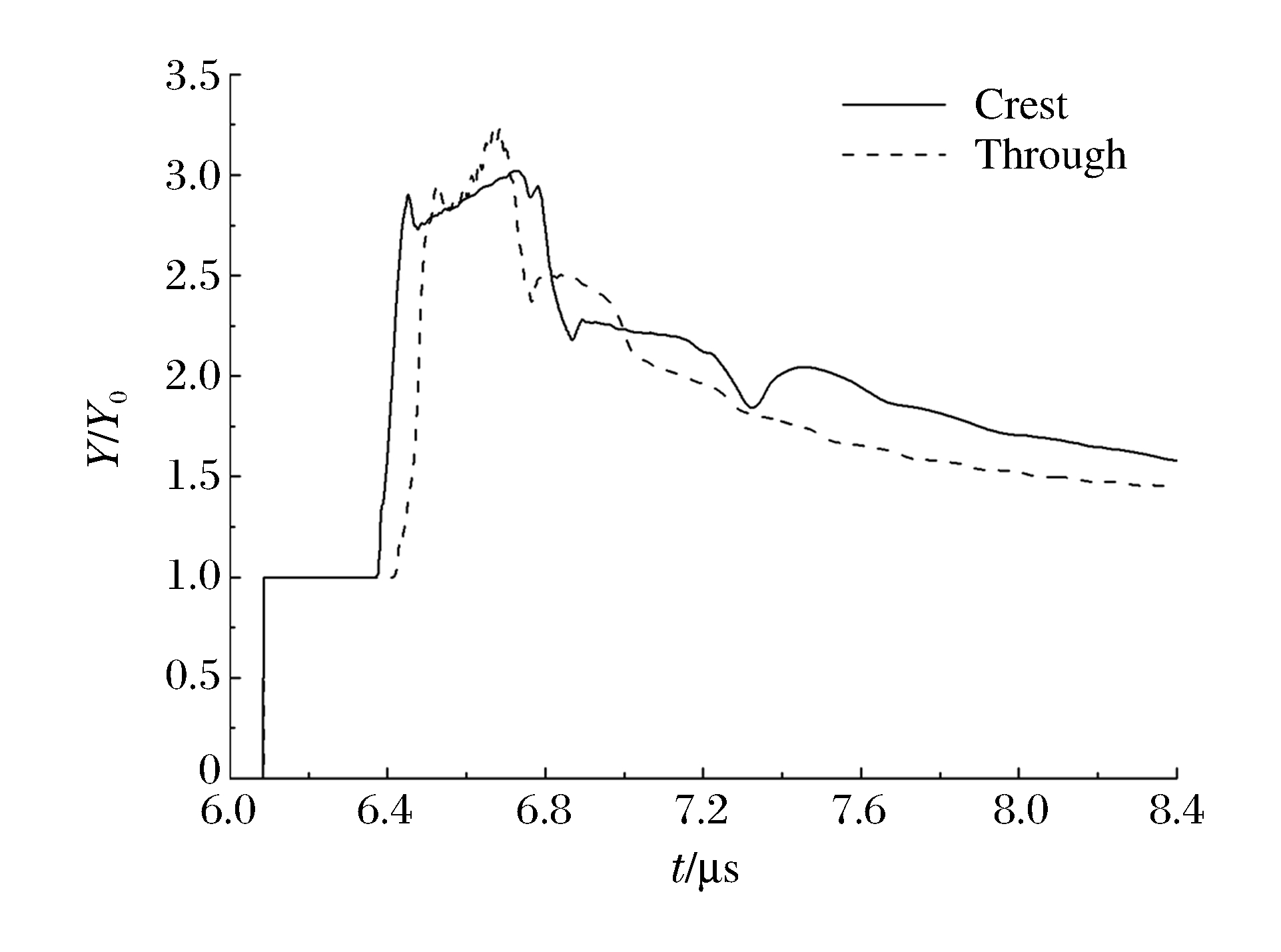
Figure 2. Comparisons of the perturbed interface between experiment and numerical simulations ((a) Experimental image, (b) Simulated image at normal strengths Y₀ and G₀, (c) Simulated image at 10 times the normal strengths Y₀ and G₀)

DownLoad: Full-Size Img PowerPoint

Fig. 3 shows the pressure histories of the crest (solid line) and the trough (dashed line) on the loading surface, which increase continuously and smoothly in a short time and form an approximate quasi-isentropic drive.Afterwards, the expansion of the detonation products decelerates gradually, and the loading pressure on the interface rises slowly.However, the pressure at the trough ascends faster than that at the crest, and the peak pressure at the trough is also relatively larger, because the detonation products converges at the trough and diverges at the crest.The average peak pressure is about 25 GPa, and the strain rate reaches 10⁶ s^-1.The loading pressure then reduces gradually, which is attributed to the decrease of the expansion pressure of the detonation products and the unloading effect of the rarefaction wave reflecting from the free surface.

Figure 3. Pressure histories of crest and trough at the loading surface

DownLoad: Full-Size Img PowerPoint

Fig. 4 shows several contours of local pressure (a), density (b), and temperature (c) at 6 different times after the arrival of the detonation products at the loading surface, from left to right and top to bottom, at 6.36, 6.5, 6.7, 6.9, 7.1 and 7.3 μs.They exhibit an evolution process of the perturbed interface and the interaction of the wave and the interface.The blue part of the temperature contour is the aluminum sample, and the sample temperature remains below 500 K and far below the melting point of 1 200 K, which indicates that the sample is in the elastic-plastic state all the time.

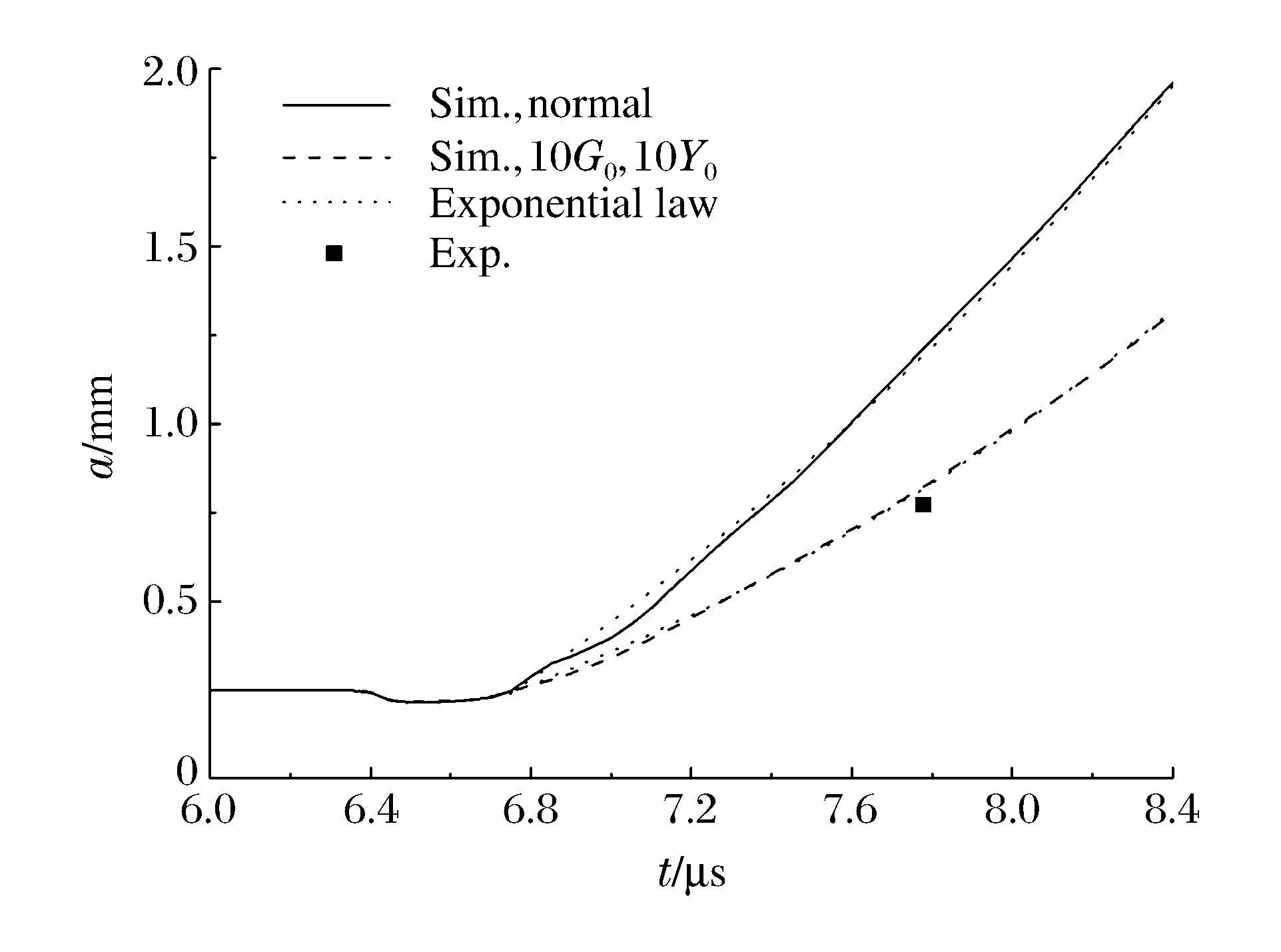
Figure 4. Contours of local pressure (a), density (b), and temperature (c) at 6.36, 6.5, 6.7, 6.9, 7.1, and 7.3 μs from left to right and top to bottom after the arrival of detonation products at the loading surface

DownLoad: Full-Size Img PowerPoint

Fig. 2(b) shows an image of the sample at 7.78 μs when the initial yield strength Y₀ and the shear modulus G₀ are normal values.Fig. 5 shows a comparison of the perturbation amplitudes between the experiment and numerical simulations, where the square symbol corresponds to the experimental result, and the solid black line corresponds to the numerical results when Y₀ and G₀ are normal.The simulated amplitude is much larger than that in the experiment when using normal values of Y₀ and G₀.This is because the aluminum strengthens under such conditions, and the SG constitutive model underestimates its strength, which can suppress the perturbation growth.

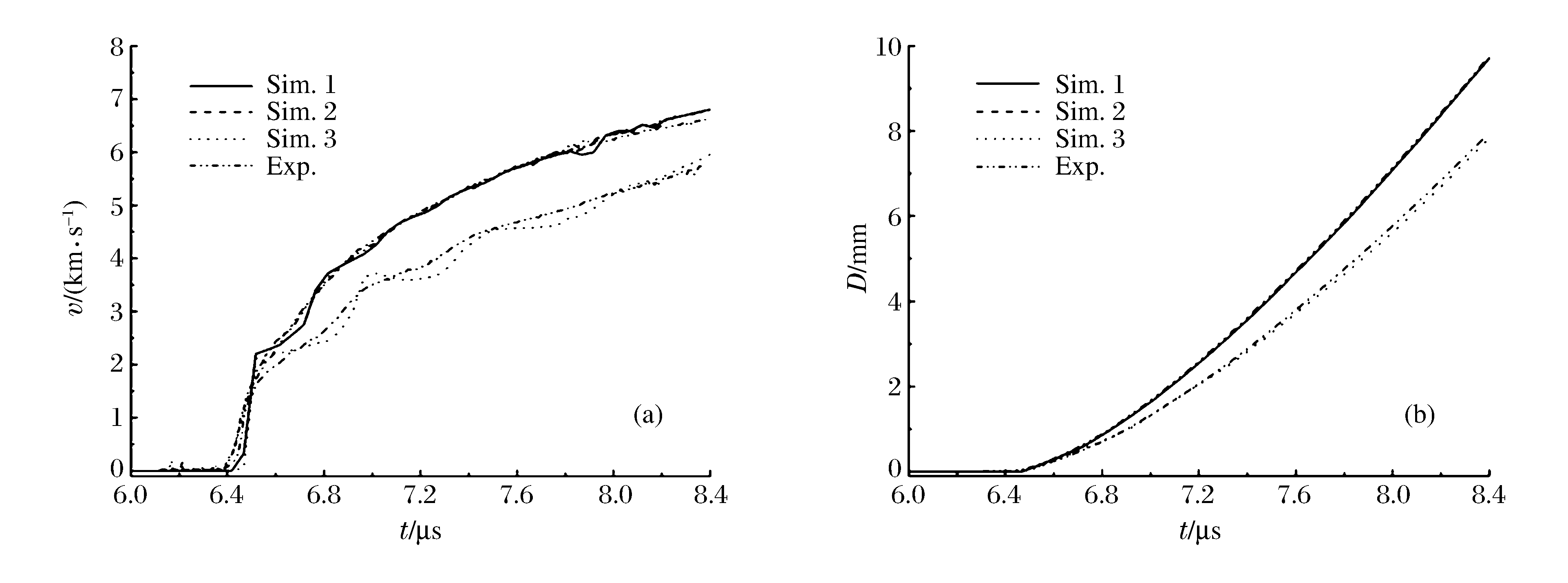
Figure 5. Growth histories of the perturbation amplitude

DownLoad: Full-Size Img PowerPoint

Fig. 6 shows the time histories of the free surface velocity (a) and displacement (b) at 3 measurement points (dot-dot-dashed lines:experiment; solid, dashed, and dotted lines:simulations), which agree well with each other.Therefore, the calculations of detonation of the explosive and the thermodynamic state of the sample are exact.

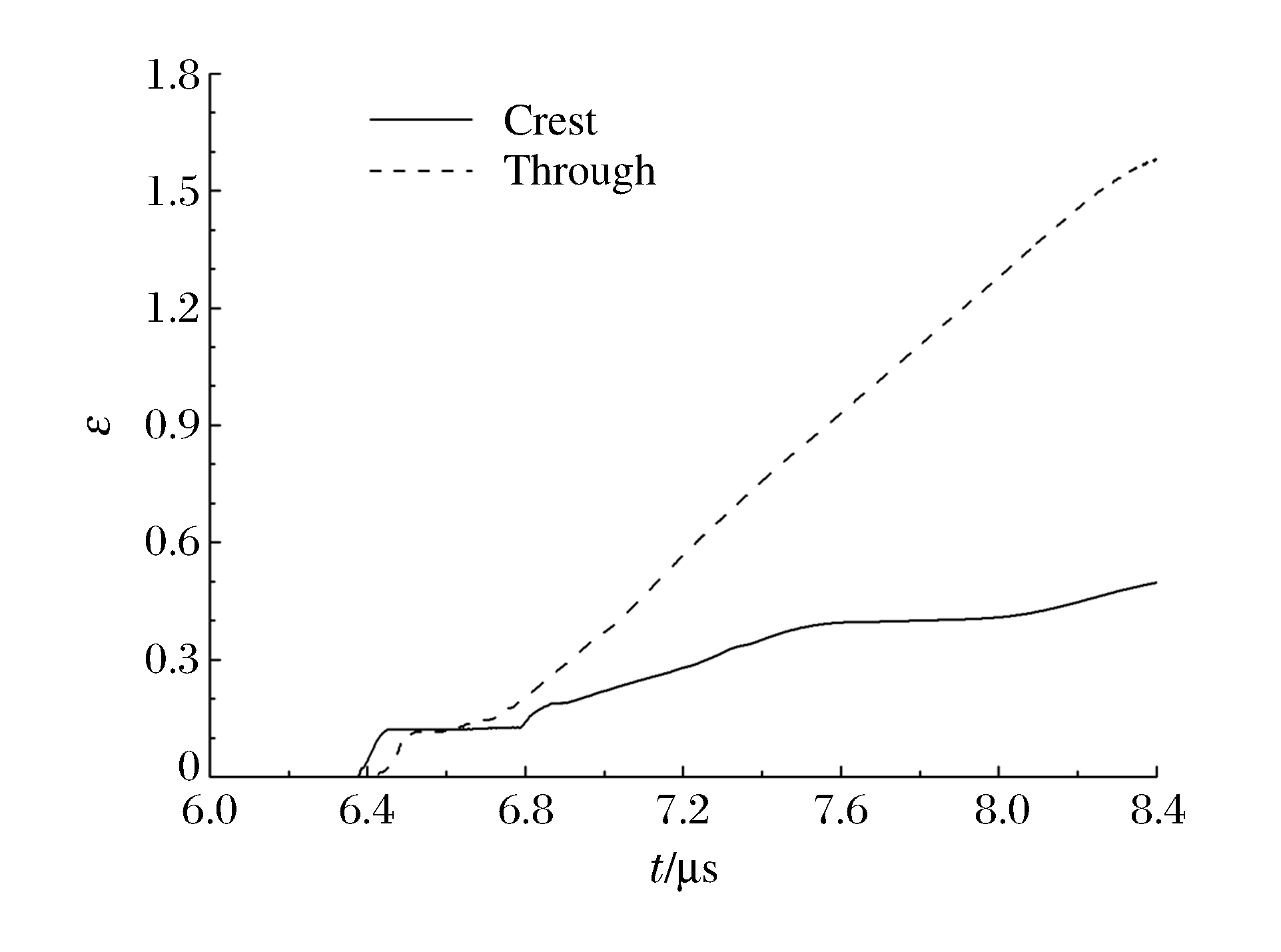
Figure 6. Time histories of the free surface velocity (a) and displacement (b)

DownLoad: Full-Size Img PowerPoint

Fig. 7 shows the calculated time histories of the strain at the crest (solid line) and trough (dashed line) of the loading surface.The deformation at the trough is much larger than that at the crest because the trough of the sample is in a stronger tensile stress state, which is the main mechanism for the deformation of the perturbation interface.Fig. 8 shows the time histories of the dynamic yield strength at the crest (solid line) and trough (dashed line) of the loading surface, calculated using the SG constitutive model, similar to the profile of the loading pressure, which demonstrate that the material strength increased as did the loading pressure under a certain condition.

Figure 7. Time histories of strain at the crest and trough of the loading surface

DownLoad: Full-Size Img PowerPoint

Figure 8. Time histories of yield strength at the crest and trough of the loading surface

DownLoad: Full-Size Img PowerPoint

Moreover, when the loading pressure reaches a peak, the dynamic yield strength was up to 3 times that of the initial value.In fact, the normal SG model is generally calibrated by conventional Hopkinson and Taylor impacts experiment with a lower strain rate.Under the current loading conditions (loading pressure of 25 GPa and strain rate of 10⁶ s^-1), the strength is not great enough to suppress the perturbation growth.However, when the initial yield strength Y₀ and the shear modulus G₀ increase to 10 times that of the normal values, good agreement between the experiment and simulation is achieved, as shown in Fig. 2(c) for the perturbation interface and in Fig. 5 for the perturbation amplitude with the dashed line.Therefore, the material strength intensively stabilizes the perturbation growth.The dotted lines in Fig. 5 are fitted lines from the simulated results, indicating that the perturbation amplitude grows exponentially over time.

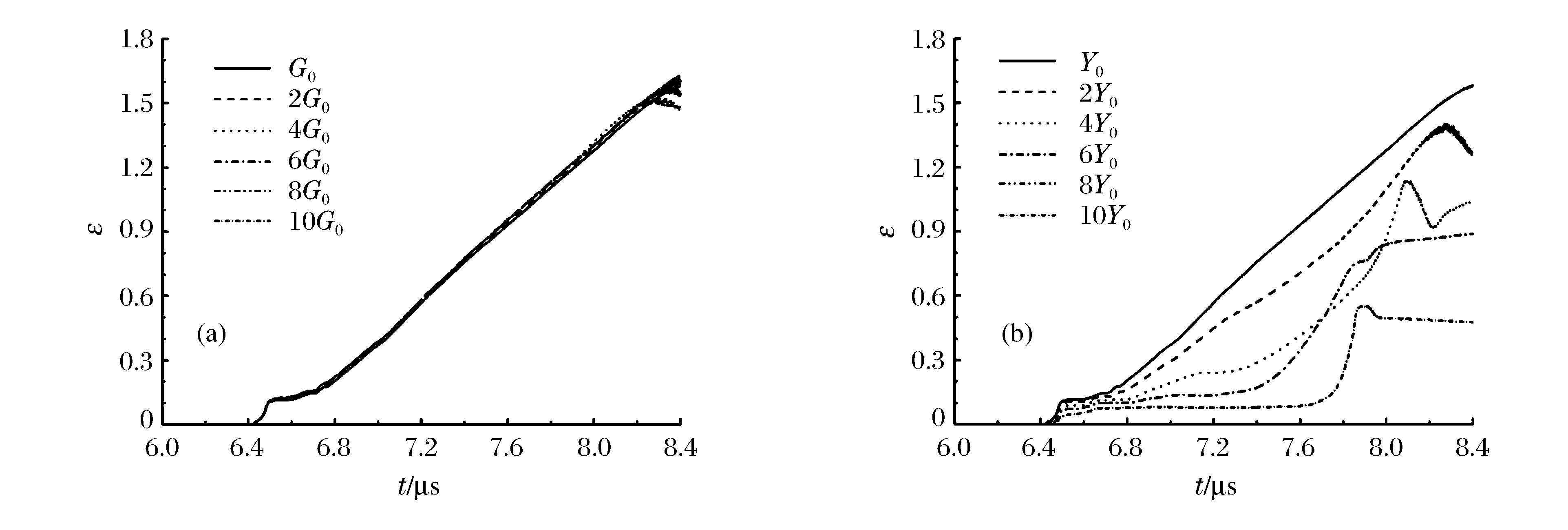
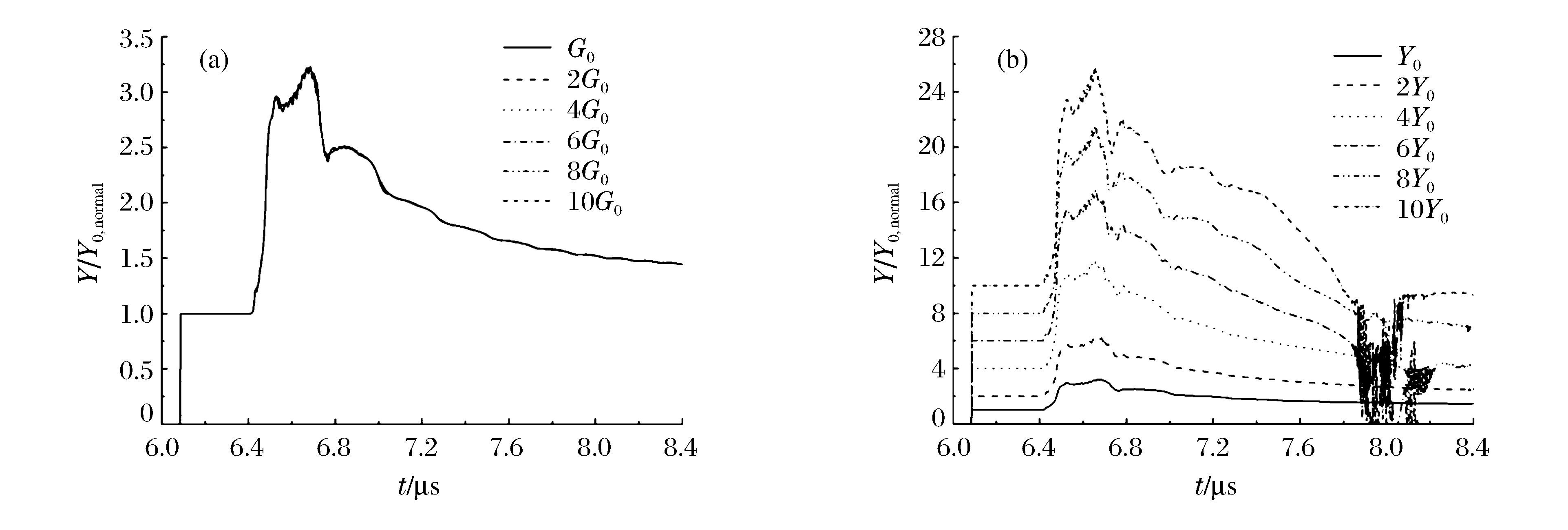
We studied the effect of the initial yield strength and the initial shear modulus of the material on the evolution and growth of the perturbed interface.Figs. 9(a), 10(a), and 11(a) show the calculated growth histories of perturbation amplitude, strain histories, and dynamic yield strength histories at the trough of the loading surface, respectively, when the initial yield strength is fixed at the normal value and as the initial shear modulus increases gradually.The growth of the perturbation amplitude exhibits no change even when the initial shear modulus increases to 10 times that of the normal value, which means that the initial shear modulus has no influence on the material deformation and does not affect the dynamic yield strength.

Figure 9. Growth histories of the perturbation amplitude for different values of initial shear modulus (a) and yield strength (b)

DownLoad: Full-Size Img PowerPoint

Figure 10. Time histories of strain at the trough of the loading surface for different values of initial shear modulus (a) and yield strength (b)

DownLoad: Full-Size Img PowerPoint

Figure 11. Time histories of yield strength at the trough of the loading surface for different values of initial shear modulus (a) and yield strength (b)

DownLoad: Full-Size Img PowerPoint

Figs. 9(b), 10(b), and 11(b) show the numerical results when the initial shear modulus is fixed and the initial yield strength gradually increases to 10 times that of the normal value.These indicate that, with the increase of the initial yield strength, the dynamic yield strength also increases, the material deformation is retarded, and the perturbation growth is suppressed markedly.Therefore, the initial shear modulus of the material exerts no effect on the growth of the metallic RT instability within a certain range, while the initial yield strength has an obvious effect on it.

4. Conclusions

We have established an experimental setup and developed a numerical simulation method to investigate the RT instability in metallic materials driven by explosion.We also studied the RT instability in aluminum, and drew the following conclusions:

(1) The perturbation amplitude grows following an exponential law over time.

(2) When using the normal physical property parameters of aluminum, simulated evolution of the perturbed interface agrees with experiment only qualitatively, and there is a big quantitative difference between them because the aluminum strengthens under high pressures and at high strain rates, and the SG constitutive model underestimates its strength as being not great enough to suppress the perturbation growth.

(3) When the initial yield strength and the initial shear modulus increase to 10 times their normal values, the numerical and experimental results are in good agreement both qualitatively and quantitatively.The underlying physical mechanism is the stabilization effect of material strength on the perturbation growth.Moreover, the initial shear modulus has no influence on the perturbation growth within a certain range whereas the initial yield strength does influence it strongly.Therefore, the material strength dominates the evolution of the metallic RT instability.

图 1 芶清泉(前排中间)、洪时明(后排右一)和若槻雅男(前排右一)

Figure 1. Gou Qingquan (seated at the center), Hong Shiming (standing, first from the right), and Masao Wakatsuki (seated, first from the right) and their research team

下载: 全尺寸图片幻灯片

图 2 实现上下独立加压的国产六面顶压机(a)及其顶锤压力变化曲线(b)

Figure 2. Domestic-made cubic presses implemented the function of independent loading in the vertical direction (a) and oil pressure-time history (b)

下载: 全尺寸图片幻灯片

图 3 国外2-6-8型增压装置^[13-14]

Figure 3. Foreign-made pressurized device of 2-6-8 type^[13-14]

下载: 全尺寸图片幻灯片

图 4 国产六面顶压机6-8型增压装置

Figure 4. Domestic-made pressurized device of 6-8 type cubic presses

下载: 全尺寸图片幻灯片

图 5 工业高温高压合成区域发展示意

Figure 5. Pressure-temperature diagram of industrial synthesis

下载: 全尺寸图片幻灯片

参考文献(18)

[1]	LIEBERMANN R C.Multi-anvil, high pressure apparatus:a half-century of development and progress[J]. High Pressure Research, 2011, 31(4):493-532. doi: 10.1080/08957959.2011.618698
[2]	KUNIMOTO T, IRIFUNE T. Pressure generation to 125 GPa using a 6-8-2 type multianvil apparatus with nano-polycrystalline diamond anvils[C]//Journal of Physics: Conference Series. IOP Publishing, 2010, 215(1): 012190.
[3]	KUNIMOTO T, IRIFUNE T, SUMIYA H.Pressure generation in a 6-8-2 type multi-anvil system:a performance test for third-stage anvils with various diamonds[J]. High Pressure Research, 2008, 28(3):237-244. doi: 10.1080/08957950802246530
[4]	SUMIYA H, IRIFUNE T.Indentation hardness of nano-polycrystalline diamond prepared from graphite by direct conversion[J]. Diamond and Related Materials, 2004, 13(10):1771-1776. doi: 10.1016/j.diamond.2004.03.002
[5]	SUMIYA H, HARANO K, IRIFUNE T.Ultrahard diamond indenter prepared from nanopolycrystalline diamond[J]. Review of Scientific Instruments, 2008, 79(5):056102. doi: 10.1063/1.2918985
[6]	SUMIYA H, IRIFUNE T.Hardness and deformation microstructures of nano-polycrystalline diamonds synthesized from various carbons under high pressure and high temperature[J]. Journal of Materials Research, 2007, 22(8):2345-2351. doi: 10.1557/jmr.2007.0295
[7]	LE GUILLOU C, BRUNET F, IRIFUNE T, et al.Nanodiamond nucleation below 2 273 K at 15 GPa from carbons with different structural organizations[J]. Carbon, 2007, 45(3):636-648. doi: 10.1016/j.carbon.2006.10.005
[8]	IRIFUNE T, KURIO A, SAKAMOTO S, et al.Formation of pure polycrystalline diamond by direct conversion of graphite at high pressure and high temperature[J]. Physics of the Earth and Planetary Interiors, 2004, 143:593-600. https://www.sciencedirect.com/science/article/pii/S0031920104000780
[9]	DUBROVINSKY L, DUBROVINSKAIA N, BYKOVA E, et al.The most incompressible metal osmium at static pressures above 750 gigapascals[J]. Nature, 2015, 525(7568):226-229. doi: 10.1038/nature14681
[10]	王福龙, 贺端威, 房雷鸣, 等.基于铰链式六面顶压机的二级6-8型大腔体静高压装置[J].物理学报, 2008, 57(9):5429-5434. doi: 10.7498/aps.57.5429 WANG F L, HE D W, FANG L M, et al.Design and assembly of split-sphere high pressure apparatus based on the hinge-type cubic-anvil press[J]. Acta Physica Sinica, 2008, 57(9):5429-5434. doi: 10.7498/aps.57.5429
[11]	方啸虎.中国超硬材料与制品50周年精选论文集[M].杭州:浙江大学出版社, 2014:23-31.
[12]	王海阔. 基于国产六面顶压机增压装置的压力产生极限扩展与应用[D]. 成都: 四川大学, 2013. WANG H K. Devlopment and application of pressure generation techniques based on hinge-type cubic press[D]. Chengdu: Sichuan University, 2013.
[13]	WALKER D, CARPENTER M A, HITCH C M.Some simplifications to multianvil devices for high pressure experiments[J]. The American Mineralogist, 1990, 75(9/10):1020-1028. https://pubs.geoscienceworld.org/msa/ammin/article-abstract/75/9-10/1020/42423/some-simplifications-to-multianvil-devices-for
[14]	OHTANI E, OKADA Y, KAGAWA N, et al. Development of a new guide-block system and high pressure and temperature generation[C]//Abstract of the 28th High Pressure Conference of Japan. Kobe, 1987: 222-223.
[15]	XU C, HE D W, WANG H K, et al.Nano-polycrystalline diamond formation under ultra-high pressure[J]. International Journal of Refractory Metals and Hard Materials, 2013, 36:232-237. doi: 10.1016/j.ijrmhm.2012.09.004
[16]	王海阔, 贺端威, 许超, 等.复合型多晶金刚石末级压砧的制备并标定六面顶压机6-8型压腔压力至35 GPa[J].物理学报, 2013, 62(18):180703. doi: 10.7498/aps.62.180703 WANG H K, HE D W, XU C, et al.Calibration of pressure to 35 GPa for the cubic press using the diamond-cemented carbide compound anvil[J]. Acta Physica Sinica, 2013, 62(18):180703. doi: 10.7498/aps.62.180703
[17]	LIU G D, KOU Z L, YAN X Z, et al.Submicron cubic boron nitride as hard as diamond[J]. Applied Physics Letters, 2015, 106(12):121901. doi: 10.1063/1.4915253
[18]	WANG P, HE D W, WANG L P, et al.Diamond-cBN alloy:a universal cutting material[J]. Applied Physics Letters, 2015, 107(10):101901. doi: 10.1063/1.4929728