Prediction of Ballistic Limit of Composite GFRP Sandwich Panels under Hypervelocity Impact
doi: 10.11858/gywlxb.20170529
-
Abstract: In the present study, analytical solutions were presented for the prediction of the penetration and perforation of composite glass fiber-reinforced plastic (GFRP) sandwich panels struck normally by flat-nosed cylindrical projectiles over a wide range of impacting velocities, projectile mass and core thickness.The analysis model involved a three-stage perforation process including perforation of the front steel skin, the GFRP core, and the back steel skin.The formulation were based on assumptions that the deformations of steel skins are localized and the projectile is considered as a rigid body in the perforation of GFRP composite laminate.The energy absorption of the front and back steel skins and the GFRP core were estimated with the upsetting effect of the projectile and the adiabatic shear effect of the steel skins taken into consideration.In addition, based on the energy balance, the ballistic limit of the sandwich panel were obtained and compared with the available experimental results.The results show that the analytical predictions are in good agreement with the available experimental data.
-
Key words:
- ballistic impact /
- sandwich panel /
- glass fiber reinforced /
- ballistic limit
摘要: 研究了玻璃纤维复合三明治板在圆柱形平头弹体打击下的预测弹道极限的理论预测方法。建立了玻璃纤维复合三明治板的三阶段侵彻模型,包括侵彻面板阶段、侵彻复合材料夹芯层阶段和侵彻内板阶段。基于高速弹体侵彻下靶板的局部变形假设建立了理论关系,将弹体侵彻复合材料夹心层时视为刚体处理,面板和背板的侵彻阶段考虑了弹体的墩粗效应和靶板的绝热剪切效应。基于能量平衡原理,推导了复合材料三明治板的弹道极限,并将理论计算结果与实验结果进行对比和分析,研究了不同侵彻速度、弹体质量和夹心层厚度对弹道极限的影响。结果表明,理论计算结果与实验结果具有较好的一致性。 -
随着微电子技术的飞速发展,特别是5G技术的出现以及电子器件微型化要求其功率密度越来越高,对电子封装材料的散热要求越来越严格,其导热性能和热匹配直接决定着电子元器件的稳定性和可靠性[1-2]。目前电子封装材料已经经历第1代合金(10~20 W/(m·K))、第2代W(Mo)-Cu合金(200 W/(m·K))、第3代陶瓷/金属SiCP/Al(120~230 W/(m·K))发展到现今的第4代Al(Cu)/Diamond(400~900 W/(m·K))。目前,高导热、低热膨胀、轻量化是新一代电子封装材料的发展趋势。金刚石具有较高的导热系数(1 000~2 200 W/(m·K)),同时金刚石已实现工业化生产,价格低廉,是制备金属基导热复合材料最理想的高效填充材料。与铜、银、钨等金属基体相比,铝具有优良的导热系数(237 W/(m·K)),同时兼具低密度、低成本等优点[3-6]。因此,高导热铝/金刚石复合材料是极具发展潜力的高导热金属基电子封装材料。
近年来,利用真空热压法(VHP)、放电等离子体烧结法(SPS)和气压熔渗法(GPI)等制备方式,通过长时间连续烧结、金属基体合金化、金刚石表面金属化等手段相继制备出高性能金刚石/铝复合材料,其热导率达到140~760 W/(m·K)[7-11]。这些制备方式的共同特点是受设备升降温速率影响,整个样品制备周期较长,至少需要1~2 h。与上述制备方式相比,高温高压(HTHP)方式在制备高导热金属/金刚石复合材料上具有较高的效能。高温高压制备方式通常用来合成金刚石、立方氮化硼等超硬材料,近年来国内外开始利用高温高压方式制备高导热复合材料[12-20]。俄罗斯Ekimove等[18]在1 200 ℃、8 GPa超高压条件下使用体积分数为95%的200 μm金刚石颗粒制备了热导率高达900 W/(m·K)的金刚石/铜复合材料,认为高压实现了金刚石与金刚石界面的桥接,形成了部分连续导热通道,从而大幅度提升了整体复合材料的热导率。然而,8 GPa的超高制备压力目前无法实现工业化生产,国内六面顶压机能够达到的制备压力一般在5~6 GPa。日本Yoshida等[19]在1 150 ℃、4.5 GPa高压熔渗工艺条件下利用粒度为90~110 μm、体积分数为70%的金刚石颗粒作为增强体,成功制备出具有超高导热性能的金刚石/铜复合材料,其热导率达到742 W/(m·K),他们也认为高压导致材料内部实现了部分金刚石颗粒之间的连通,即金刚石/铜界面被金刚石/金刚石界面所取代,从而大幅度降低了界面热阻。相关高温高压制备金属基金刚石复合导热材料的文献报道大部分是以铜基体为主[16-21]。同时,有文献报道采用VHP、SPS、GPI等方式制备的镀钛金刚石/铝复合材料能够促进金刚石与基体的润湿性,减小空隙热阻,热导率得到极大提升[5-7, 10, 20, 22-23]。然而,利用高温高压方式制备以镀钛金刚石为填充材料的金刚石/铝复合材料却鲜有报道。
本研究使用经放电等离子烧结处理的200 μm镀钛金刚石颗粒为填充材料,使用纯铝为金属基体材料,在3 GPa、700 ℃条件下烧结10 min,考察金刚石/铝复合材料的微观组织形貌、导热性能和热膨胀性能。
1. 实验方法
1.1 镀钛金刚石的制备
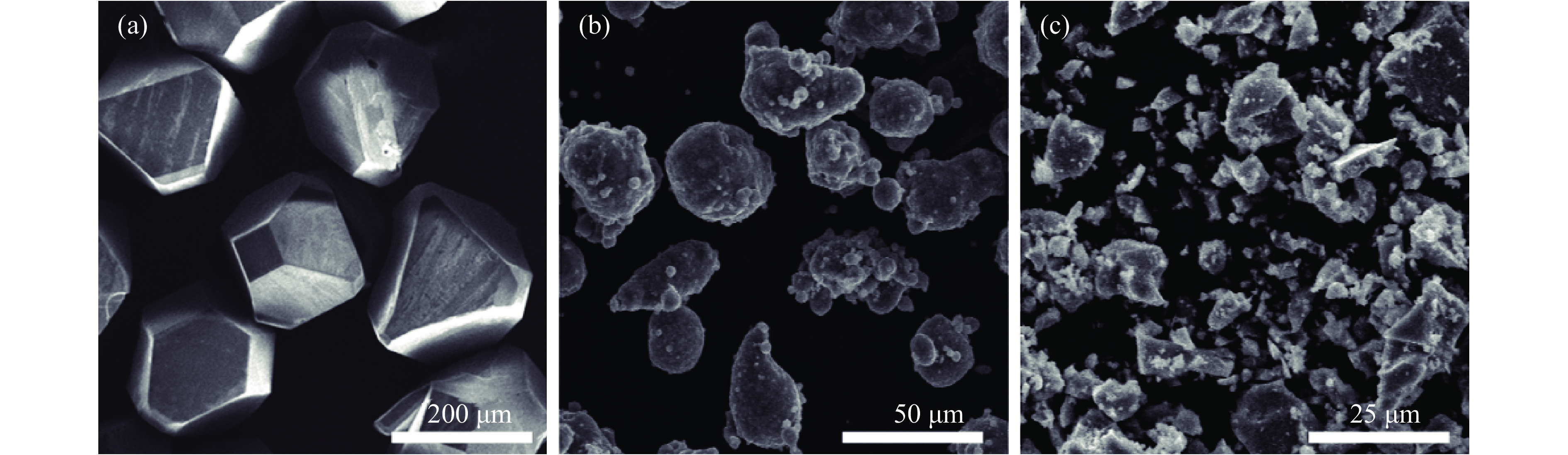
实验所用的原材料金刚石、铝粉和钛粉形貌如图1所示。金刚石颗粒粒度为200 μm,购于河南黄河旋风有限公司,利用傅里叶红外光谱测试其氮含量范围在0.015%~0.020%,根据相关文献计算其热导率范围为1 500~2 000 W/(m·K)[18, 24]。使用粒径10 μm、纯度99.99%的钛粉作为镀覆材料,使用粒径40 μm、纯度99.99%的铝粉作为金属基体材料。
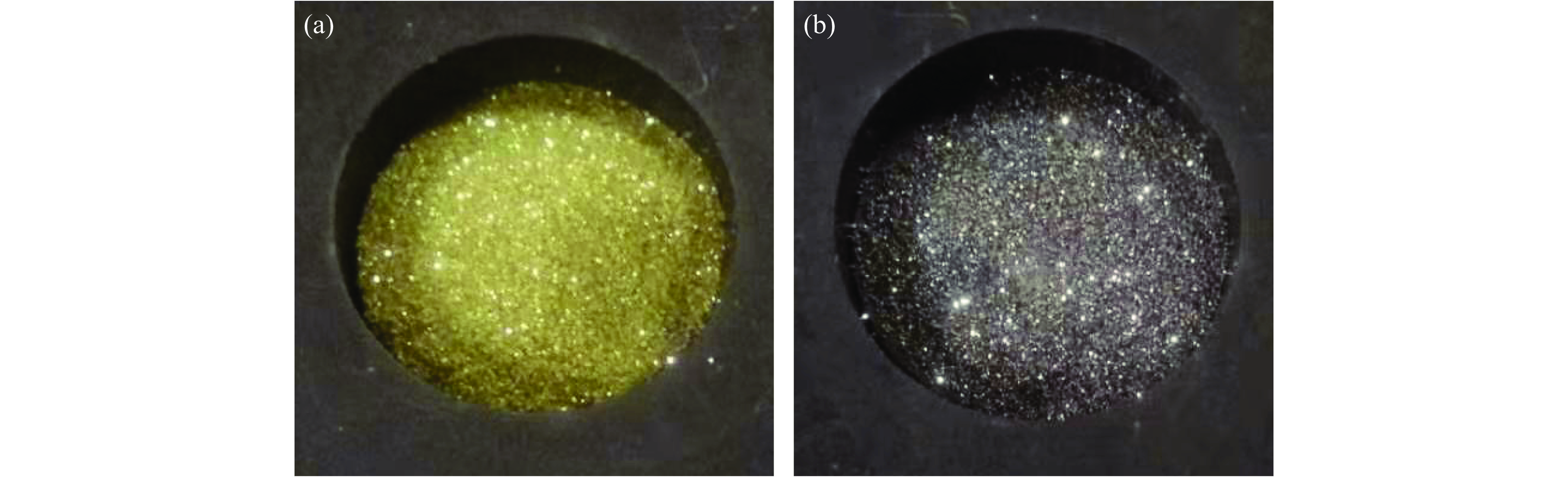
金刚石和钛粉按照质量比9∶1在玛瑙研钵内混合2 h,混合过程中加入乙二醇使金刚石和钛粉混合均匀。将混合料放入直径20 mm的石墨模具内,通过油压机冷压成型,然后将石墨模具放入放电等离子体烧结设备(日本思立LABOX-325R型)进行烧结处理。抽真空至真空度低于10 Pa后加压至50 MPa,然后以100 ℃/min的加热速度加热至800 ℃,保温30 min后停止加热自然降温。冷却脱模取出样品,样品经破碎、超声、洗涤、干燥,最后获得镀层厚度在150~200 nm左右的镀钛金刚石颗粒[15]。图2所示为金刚石/钛粉末放电等离子体烧结处理前后的光学照片。可以看出,经放电等离子体烧结处理的金刚石/钛混合粉末由黄色变为灰黑色,说明钛粉末颗粒在放电等离子体烧结处理过程中粘覆到金刚石晶面上,导致光线不能透过金刚石晶面,进而显现为灰黑色。
1.2 金刚石/铝复合材料的制备和样品处理
铝粉与制备的镀钛金刚石颗粒按照体积比1∶1放入玛瑙研钵中混合2 h,混合过程中加入乙二醇。均匀混合后的颗粒使用金属模具冷压成
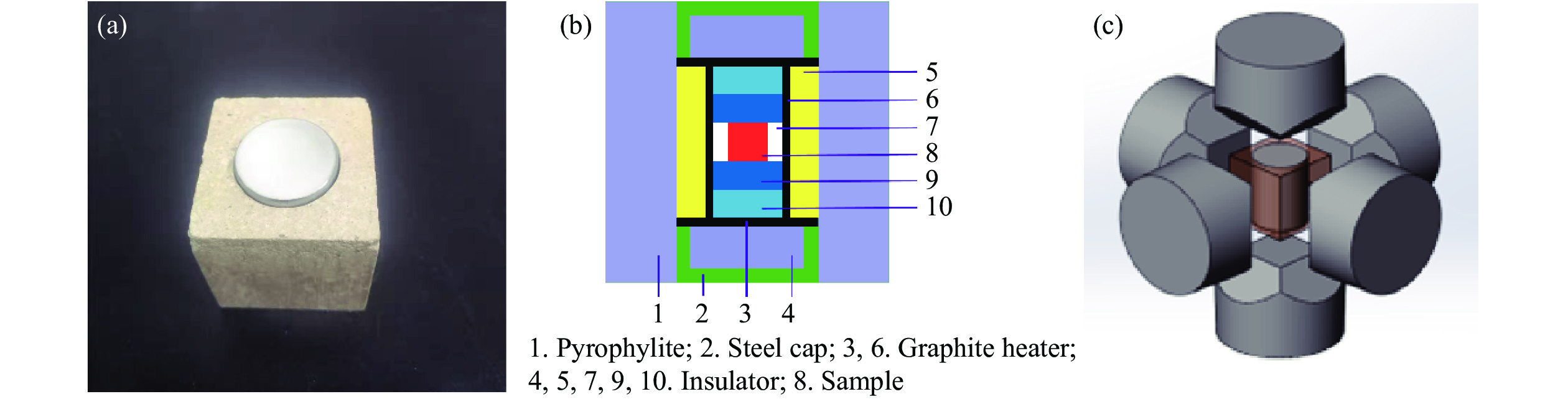
∅ 10.0 mm × 3.5 mm的圆柱,然后将样品放入高压组装腔体内,将高压组装块放置在烘箱内,于120 ℃干燥2 h,去除吸附水和乙二醇。实验组装如图3所示。实验所用的高压设备是国产铰链式六面顶压机(BT6 × 800),通过电加热石墨管获取实验所需的温度,将经过干燥处理的高压组装块放入高压设备腔体内,先升压至3 GPa,然后以200 ℃/min的升温速率升温至700 ℃并保温10 min,而后停止加热、卸载压力,取出高压组装块和样品。使用不经镀钛处理的金刚石颗粒做对比,样品经表面打磨、清洗以去除表面粘附的杂质,并于真空干燥处理。在实验过程中,温度根据Pt-30%Rh/Pt-6%Rh热电偶进行标定,压力根据标压物Bi、Ba的高压相变点建立的油压和腔体压力关系进行标定。
1.3 样品表征
使用激光导热仪(德国耐驰LFA467)测试金刚石/铝样品的热扩散系数。根据公式
λ=ρcpα (λ 为热导率,α 为热扩散系数,ρ 为样品密度,cp为样品定压比热容)进行计算[24-25]。通过阿基米德排水法测量样品的密度。使用光学显微镜和X射线衍射仪(日本理学DMAX-RB)对镀钛金刚石颗粒和物性进行分析。使用扫描电子显微镜(日本电子IT200)分析金刚石/铝复合材料的微观组织形貌。通过热膨胀仪(德国耐驰DIL402C)测量样品的热膨胀系数。2. 分析和讨论
2.1 镀钛金刚石物性分析和形貌表征
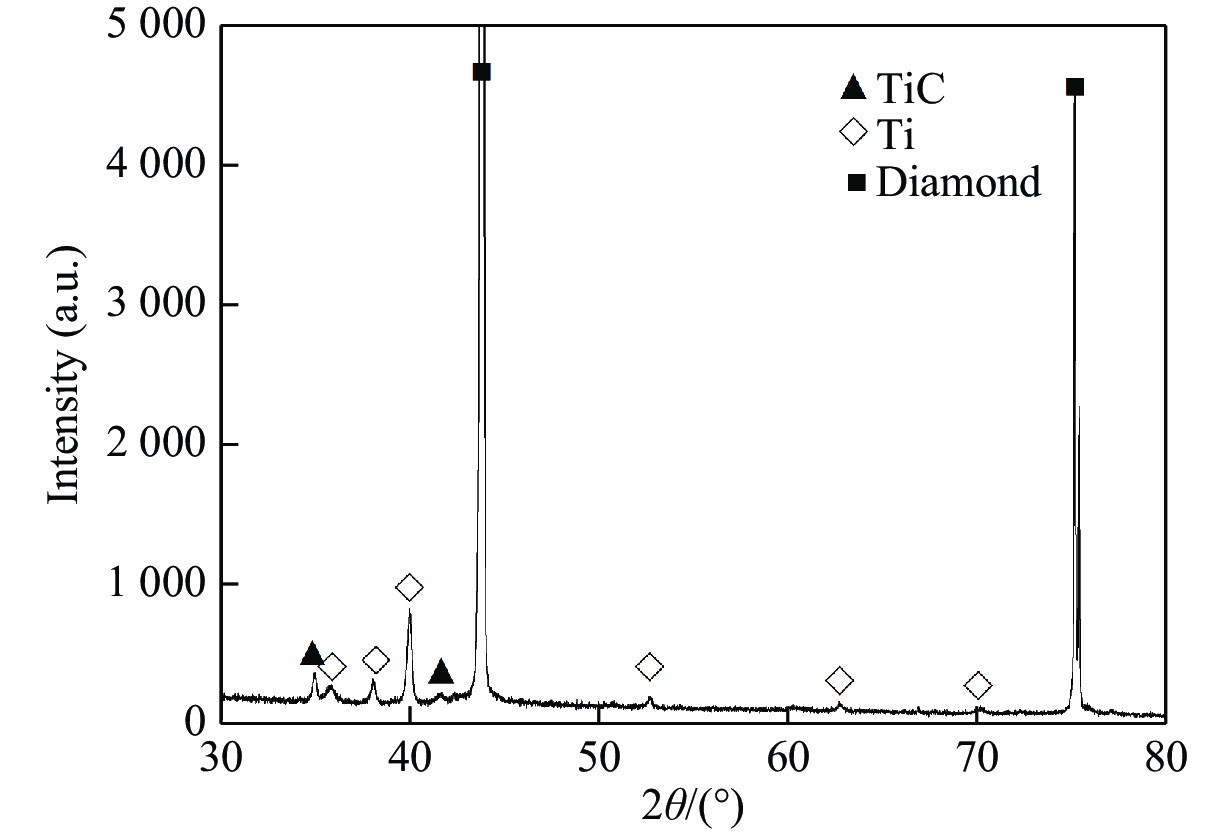
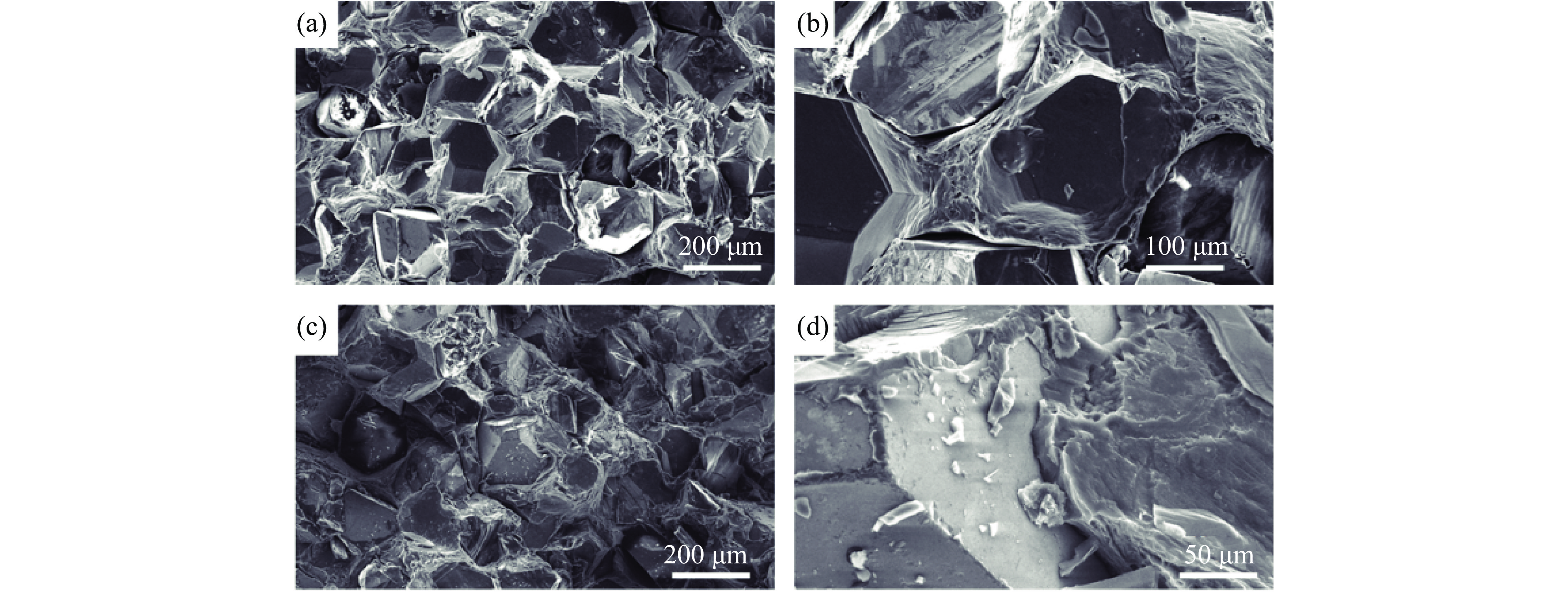
图4是镀钛金刚石颗粒的XRD图谱。可以看出,除金刚石特征峰外,还存在单质钛和碳化钛的特征峰,说明经过放电等离子体烧结处理金刚石和钛混合粉末能够在金刚石晶面形成镀层,同时镀层的主要成分为单质钛和碳化钛[15]。镀钛层能够有效抑制易潮解相碳化铝的形成,提升其使用寿命[5-7, 10]。图5(a)和图5(b)分别为金刚石镀钛前、后的普通光学照片,金刚石从金黄色变为灰黑色,从图5(b)的反光性来看,镀层相对均匀;从图5(c)镀钛颗粒的扫描电镜图像可以发现,镀层较为平整,表面存在一定数量、形状不规格的凹坑;进一步在图5(d)中可以看出,在部分晶面交界处存在部分漏镀区域,说明放电等离子烧结镀覆方式虽然能够实现金刚石表面镀钛,但会存在凹坑和漏镀的缺陷。然而,放电等离子体烧结镀覆方式的技术要求相对较低、操作简单,可以扩展到其他金属基体。
2.2 金刚石/铝复合材料的微观组织形貌
表1是制备的金刚石/铝复合材料密度和致密度的测量结果。在3 GPa、700 ℃条件下,未镀钛处理的金刚石/铝复合材料密度达到了3.06 g·cm−3,是理论密度的98.4%,该数值高于常规制备方式。而在相同的制备条件下,镀钛金刚石样品密度可上升到理论密度3.11 g·cm−3水平,样品达到全密度[3, 8]。高温高压制备时样品所受压力远远超过常规制备方式的压力范围(30~100 MPa),高制备压力能够有效促进铝基体与金刚石颗粒结合并减少空隙。当使用镀钛金刚石颗粒作为填充材料后,由于钛镀层与铝基体具有较好的润湿性,能够更好地促进铝基体与金刚石颗粒的充分结合,进一步降低空隙热阻,提高复合材料的导热率。
表 1 金刚石/铝复合材料的密度和致密度Table 1. Density and relative density of diamond/Al compositesDiamond type Density of composites/(g·cm−3) Relative density of composites/% Raw 3.06 98.40 Ti-coated 3.11 100.00 图6为金刚石/铝复合材料断裂面扫描电镜图像。从图6(a)可以发现,未镀钛处理的金刚石和铝基体界面处存在一定数量的空隙,从而产生较高的空隙热阻,阻碍热量传递。从图6(b)放大图中也可以发现,金刚石晶面上基本没有发现铝基体残留,说明金刚石和铝基体的非润湿特性导致两相界面结合较差。从图6(c)中可以看出,金刚石颗粒表面存在一定量的铝基体残留,图6(d)放大图可以清楚看到金刚石晶面处有大量的铝基体包覆,说明镀钛层能够改善金刚石和铝基体的润湿性,提升两相的界面结合,减少空隙热阻对热传导的阻碍,有利于提升复合材料整体的传热性能。此外,图6中未发现金刚石破碎,说明金刚石体积分数在50%以内时,其颗粒之间的硬接触较少,不会导致金刚石破碎[16, 20]。
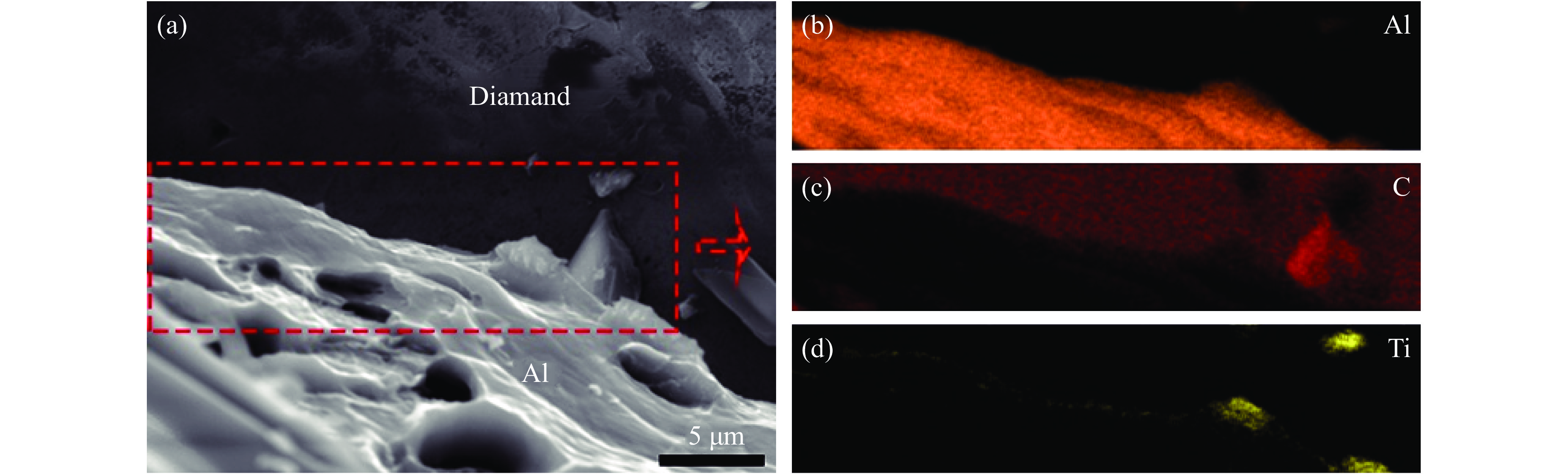
为了进一步分析镀钛层对复合材料界面结合的增强效果,对金刚石颗粒与铝基体界面结合区域中铝、钛、碳元素分布进行扫描,如图7所示。图7中钛元素主要集中在金刚石和金刚石与铝基体的界面结合处,说明钛镀层在金刚石和铝基体结合部分起到过渡的作用,能够有效改善金刚石和铝基体的润湿性,在增强界面结合方面具有关键作用。
2.3 金刚石/铝复合材料的导热性能
图8为使用镀钛与未镀钛金刚石制备的金刚石/铝复合材料的热扩散系数和换算后的热导率。对比发现,未镀钛处理制备的金刚石/铝复合材料的热导率为144 W/(m·K),低于纯铝的热导率230 W/(m·K),主要由于热量从铝基体传导到金刚石内,由电子运动传导转换为声子振动传导,具有较强的界面热阻,可以达到2.2 × 10−8 m2·K/W[26]。当使用经过镀钛处理的金刚石时,其热导率显著提高到529 W/(m·K),充分说明钛镀层是提升热导率的关键因素。我们认为钛镀层能够增强铝基体与金刚石的润湿性,有效改善铝基体与金刚石颗粒的界面结合,降低界面热阻,进而提高复合材料的热导率[27-30]。
为了更好地理解钛涂层对金刚石/铝复合材料热导率的影响,采用Maxwell模型对复合材料的理论热导率进行计算[31]
λcom=λm2Vd(λdλm−1)+(λdλm+2)Vd(1−λmλd)+(λdλm+2) (1) 式中:
λ com为复合材料的热导率;λ m为铝基体的热导率,设定为230 W/(m·K);λ d为金刚石颗粒的热导率,设定为1 500 W/(m·K);Vd为金刚石颗粒的体积分数(50%)。根据参考数值,计算得到体积分数为50%的金刚石填充金刚石/铝复合材料的理论热导率λ com为561 W/(m·K)。本实验结果为理论热导率的94%,远超过常规制备方式[3, 8-9]。2.4 金刚石/铝复合材料的热膨胀性能
图9所示为未镀钛与镀钛金刚石制备金刚石/铝复合材料的热膨胀性能测试结果。从图9(a)中可以看到,普通金刚石制备的复合材料在不同测试温度下的相对伸长率(dL/L)远高于镀钛处理金刚石制备的复合材料。普通金刚石制备的复合材料热膨胀系数(CTE)为2.0 × 10−5 K−1,使用镀钛金刚石制备的复合材料其热膨胀系数下降至1.3 × 10−5 K−1,如图9(b)所示。分析认为,由于未镀钛金刚石与铝基体界面结合较弱,铝基体在复合材料的热膨胀中占主导地位;而镀钛金刚石增强的金刚石/铝复合材料中,由于钛镀层能够有效促进铝基体与金刚石的界面结合,可以抑制高温带来的弹性和塑性变形[10, 32],表明钛镀层对于提高热导率、降低热膨胀系数起主要作用[15, 23]。高压制备方式虽然能够促进界面结合,但由于受到金刚石和铝基体没有润湿性的影响,其界面结合易受外界环境因素的影响[33]。因此,高温高压与金刚石表面镀钛处理两者共同作用能够显著提升金刚石/铝复合材料制备效能。
3. 结 论
采用高温高压粉末冶金方式,使用纯铝粉末和镀钛金刚石颗粒成功制备出热导率为529 W/(m·K)的高导热金刚石/铝复合材料,热导率达到Maxwell理论模型的94%。研究表明:高温高压制备方式和镀钛处理能够有效提高基体和金刚石的界面结合,钛镀层有效降低了界面热阻,显著提升了复合材料的热导率,降低了热膨胀系数。本研究为高温高压制备新型金属基导热复合材料的发展提供了重要的实验支撑。
感谢郑州大学物理学院(微电子学院)贾晓鹏教授课题组的全体成员。
-
Table 1. Mechanical and geometrical properties of steel skins
hf/mm hb/mm ρf/(kg·m-3) σu/MPa n γi 6.2 10.6 7 850 779 0.586 1.4 Table 2. Comparison of ballistic limit computed by theoretical model with experimental results
Mass/g Core thickness/mm Exp. value/(m·s-1) Calc. value/(m·s-1) Error/% 40 40 753.8 831.9 10.7 30 60 1 422.9 1 402.9 ─ 40 60 1 162.7 1 132.4 2.6 50 60 974.1 958.1 1.6 50 75 879.0 1 144.7 ─ Table 3. Energy absorption ratio of composite GFRP sandwich panels under impact of flat-nosed cylindrical projectile with ballistic limit velocity
Mass/g Core thickness/mm (Efro/Eabs)/% (Ecor/Eabs)/% (Ebac/Eabs)/% 40 40 2.9 90.7 6.4 30 60 1.6 95.0 3.4 40 60 1.4 95.7 2.9 50 60 1.8 94.4 3.8 50 75 1.2 96.1 2.7 -
[1] JOHNSON W S, MASTERS J E, WILSON D W, et al.Comparison of the low and high velocity impact response of kevlar fiber-reinforced epoxy composites[J]. Journal of Composites Technology & Research, 1999, 21(4):224-229. [2] VAIDYA U K, ULVEN C, PILLAY S, et al.Impact damage of partially foam-filled co-injected honeycomb core sandwich composites[J]. Journal of Composite Materials, 2003, 37(7):611-626. doi: 10.1177/002199803029724 [3] KEPLER J.Impact penetration of sandwich panels at different velocities-an experimental parameter study:part Ⅰ-parameters and results[J]. Journal of Sandwich Structures & Materials, 2004, 6(4):357-374. doi: 10.1177/1099636204038217 [4] 曹海要, 战再吉.铜/金刚石复合材料电磁轨道烧蚀特性的实验研究[J].高压物理学报, 2016, 30(4):317-322. doi: 10.11858/gywlxb.2016.04.008CAO H Y, ZHAN Z J.Experimental study of Cu/diamond composite electromagnetic rail ablation characteristics[J]. Chinese Journal of High Pressure Physics, 2016, 30(4):317-322. doi: 10.11858/gywlxb.2016.04.008 [5] 王志刚, 徐亮, 李绪海, 等.碳化硅-金刚石超硬复合材料的弹性性质[J].高压物理学报, 2015, 29(4):263-267. doi: 10.11858/gywlxb.2015.04.004WANG Z G, XU L, LI X H, et al.Elastic property of SiC-diamond composite under hydrostatic pressure[J]. Chinese Journal of High Pressure Physics, 2015, 29(4):263-267. doi: 10.11858/gywlxb.2015.04.004 [6] LI G, JONES N.Development of rubberized syntactic foam[J]. Composites Part A:Applied Science and Manufacturing, 2007, 38(6):1483-1492. doi: 10.1016/j.compositesa.2007.01.009 [7] JOVER N, SHAFIQ B, VAIDYA U.Ballistic impact analysis of balsa core sandwich composites[J]. Composites Part B:Engneering, 2014, 67:160-169. doi: 10.1016/j.compositesb.2014.07.002 [8] NIA A A, RAZAVI S B, MAJZOOBI G H.Ballistic limit determination of aluminum honeycombs—experimental study[J]. Materials Science and Engineering A, 2008, 488(1/2):273-280. https://www.sciencedirect.com/science/article/pii/S0921509307018308 [9] WEN H M.Predicting the penetration and perforation of FRP laminates struck normally by projectiles with different nose shapes[J]. Composite Structures, 2000, 49(3):321-329. doi: 10.1016/S0263-8223(00)00064-7 [10] WEN H M.Penetration and perforation of thick FRP laminates[J]. Composites Science & Technology, 2001, 61(8):1163-1172. https://www.sciencedirect.com/science/article/pii/S0266353801000203 [11] GU B.Analytical modeling for the ballistic perforation of planar plain-woven fabric target by projectile[J]. Composites Part B:Engineering, 2003, 34(4):361-371. doi: 10.1016/S1359-8368(02)00137-3 [12] NAIK N K, DOSHI A V.Ballistic impact behaviour of thick composites:parametric studies[J]. Composite Structures, 2008, 82(3):447-464. doi: 10.1016/j.compstruct.2007.01.025 [13] NAIK N K, SHRIRAO P, REDDY B C K.Ballistic impact behaviour of woven fabric composites:formulation[J]. International Journal of Impact Engineering, 2006, 32(9):1521-1552. doi: 10.1016/j.ijimpeng.2005.01.004 [14] GAMA B A, GILLESPIE J W Jr.Punch shear based penetration model of ballistic impact of thick-section composites[J]. Composite Structures, 2008, 86(4):356-369. doi: 10.1016/j.compstruct.2007.11.001 [15] CHAN S, FAWAZ Z, BEHDINAN K, et al.Ballistic limit prediction using a numerical model with progressive damage capability[J]. Composite Structures, 2007, 77(4):466-474. doi: 10.1016/j.compstruct.2005.08.022 [16] FELI S, YAS M H, ASGARI M R.An analytical model for perforation of ceramic/multi-layered planar woven fabric targets by blunt projectiles[J]. Composite Structures, 2011, 93(2):548-556. doi: 10.1016/j.compstruct.2010.08.025 [17] SILVA M A G, CISMASIU C, CHIOREAN C G.Numerical simulation of ballistic impact on composite laminates[J]. International Journal of Impact Engineering, 2005, 31(3):289-306. doi: 10.1016/j.ijimpeng.2004.01.011 [18] GOLDSMITH W, WANG G T, LI K, et al.Perforation of cellular sandwich plates[J]. International Journal of Impact Engineering, 1997, 19(5/6):361-379. https://www.sciencedirect.com/science/article/pii/S0734743X97000031 [19] SKVORTSOV V, KEPLER J, BOZHEVOLNAYA E.Energy partition for ballistic penetration of sandwich panels[J]. International Journal of Impact Engineering, 2003, 28(7):697-716. doi: 10.1016/S0734-743X(02)00153-7 [20] ZHOU D W, STRONGE W J.Ballistic limit for oblique impact of thin sandwich panels and spaced plates[J]. International Journal of Impact Engineering, 2008, 35(11):1339-1354. doi: 10.1016/j.ijimpeng.2007.08.004 [21] RYAN S, SCHAEFER F, DESTEFANIS R, et al.A ballistic limit equation for hypervelocity impacts on composite honeycomb sandwich panel satellite structures[J]. Advances in Space Research, 2008, 41(7):1152-1166. doi: 10.1016/j.asr.2007.02.032 [22] BUITRAGO B L, SANTIUSTE C, SANCHEZ-SAEZ S, et al.Modelling of composite sandwich structures with honeycomb core subjected to high-velocity impact[J]. Composite Structures, 2010, 92(9):2090-2096. doi: 10.1016/j.compstruct.2009.10.013 [23] GARCIA-CASTILLO S K, BUITRAGO B L, BARBERO E.Behavior of sandwich structures and spaced plates subjected to high-velocity impacts[J]. Polymer Composites, 2011, 32(2):290-296. doi: 10.1002/pc.v32.2 [24] FELI S, POUR M H N.An analytical model for composite sandwich panels with honeycomb core subjected to high-velocity impact[J]. Composites Part B, 2012, 43(5):2439-2447. doi: 10.1016/j.compositesb.2011.11.028 [25] BAI Y L, JOHNSON W.Plugging:physical understanding and energy absorption[J]. Materials Science & Technology, 2013, 9(1):182-190. https://www.researchgate.net/publication/272248744_Plugging_physical_understanding_and_energy_absorption [26] LANGSETH M, LARSEN P K.The behaviour of square steel plates subjected to a circular blunt ended load[J]. International Journal of Impact Engineering, 1992, 12(4):617-638. doi: 10.1016/0734-743X(92)90271-T [27] BORVIK T, LANGSETH M, HOPPERSTAD O S, et al.Ballistic penetration of steel plates[J]. International Journal of Impact Engineering, 1999, 22(9/10):855-886. https://www.sciencedirect.com/science/article/pii/S0734743X99000111 -








 下载:
下载:



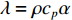
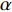
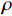





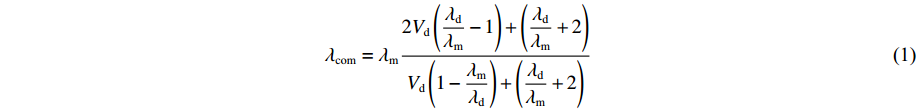
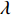
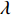
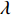
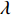


 下载:
下载: