密度非均匀流场中冲击加载双模态界面失稳现象的数值模拟

肖佳欣; 柏劲松; 王涛

doi:10.11858/gywlxb.20170501

密度非均匀流场中冲击加载双模态界面失稳现象的数值模拟

doi: 10.11858/gywlxb.20170501

中国工程物理研究院流体物理研究所，四川绵阳 621999

基金项目:

国家自然科学基金 11532012

国家自然科学基金 11372294

科学挑战专题 JCKY2016212A501

挑战计划 TZ2016001

详细信息

作者简介:
肖佳欣(1992—), 女, 硕士研究生, 主要从事流体力学研究. E-mail: crystalshaw1125@126.com

通讯作者:
柏劲松(1968—), 男, 研究员, 博士生导师, 主要从事计算力学研究. E-mail: bjsong@foxmail.com

中图分类号: O354.5
计量
- 文章访问数: 7649
- HTML全文浏览量: 2909
- PDF下载量: 267
出版历程
- 收稿日期: 2017-01-04
- 修回日期: 2017-03-13

Numerical Study of Shock Wave Impacting on the Double-Mode Interface in Nonuniform Flows

Institute of Fluids Physics, China Academy of Engineering Physics, Mianyang 621999, China

摘要

摘要: 利用可压缩多介质黏性流动和湍流大涡模拟的二维计算程序MVFT-2D，针对初始非均匀流场密度为高斯分布、马赫数Ma=1.27激波作用下的双模态界面失稳现象，进行了数值模拟研究。数值模拟结果表明，处于非均匀流场中的双模态振幅耦合效应较弱，而且低密度区的初始大振幅界面扰动增长最快，高密度区的初始小振幅界面扰动增长最慢。通过进一步分析可知，在一定初始振幅范围内，非均匀流场低密度区的振幅增长率较高，混合区域更宽，湍动能较大，受初始振幅影响较大，导致该区域界面不稳定演化较快。其变化规律与均匀流场呈现相反趋势，说明非均匀流场界面不稳定性的发展规律与均匀流场存在较大差异。
- 密度非均匀流场 /
- Richtmyer-Meshkov不稳定性 /
- 初始扰动 /
- 数值模拟
Abstract: The double-mode Richtmyer-Meshkov (RM) instability, when the incident shock (Ma=1.27) impacting on several groups of initial double-mode cosine interface formed by different amplitudes in initially nonuniform flows whose density is Gaussian distribution, was numerically investigated using the large-eddy simulation code MVFT (multi-viscous-flow and turbulence).The numerical results show that the coupling effects between different amplitudes in the nonuniform flows are weak and the evolution of the interface with a large initial amplitude in the low density nonuniform area grows fastest, while that with a small initial amplitude in the high density nonuniform area grows slowly.Further analyses reveal that within a certain initial amplitude range, the amplitude growth rate and energy in the low density zone of nonuniform flows is larger than those in the high density zone, thus the influence of the initial amplitude on the low density zone is more obvious, which eventually leads to a faster evolution process of the RM instability.Moreover, the changing phenomena of uniform flows are opposite to nonuniform flows.Thus, it can be concluded that the evolution mechanisms of the RM instability between the nonuniform and uniform flows are distinctive.
- nonuniform flows /
- Richtmyer-Meshkov instability /
- initial perturbation /
- numerical simulation

HTML全文

激波加载初始扰动下密度间断的界面会产生Richtmyer-Meshkov(RM)不稳定性现象，其产生机理是界面处密度、压力梯度不共线引起的斜压涡效应。对激波与交界面相互作用的研究不仅具有重要的学术意义，而且具有重要的工程应用价值。超燃冲压发动机中的燃料混合以及发动机的结构设计^[1]，天体物理中超新星塌缩和爆炸、射流、超新星反弹强冲击波与外壳及周围非均匀星际云的相互作用^[2]以及惯性约束聚变^[3-6]等都需要考虑界面不稳定性。

近年来，关于界面不稳定性的研究已经成为国际学术和工程界热点研究课题之一，在理论、数值模拟和实验方面均已取得较大的进展，并发现初始条件对界面不稳定性的演化有重要的影响。为使超燃发动机中燃料和氧化剂快速有效混合，Yang等^[1]对气柱界面的不稳定性进行了数值模拟，最终确定密度比等7种因素对圆柱RM界面不稳定性发展的影响较大。Kumar等^[7]对三气柱RM界面不稳定性进行了实验研究，发现气柱之间下游的扩散材料越多，漩涡结构的耦合联结就越强烈。Orlicz等^[8]通过实验研究了无膜SF₆气帘在马赫数Ma=1.2和1.5激波冲击下界面不稳定性的演化过程，发现随着马赫数的增加，尖钉、气泡尺寸越大，后期湍流混合现象越明显。

然而，之前学者主要关注均匀流场的情况，对非均匀流场的研究较少。流场的非均匀性是影响界面不稳定性发展的重要因素，对可变密度流体力学界面不稳定性的研究是美国Los Alamos国家实验室MaRIE计划^[9]研究要点之一。刘金宏等^[10]开展了平面激波冲击非均匀流场界面的激波管实验，并利用阴影技术获得了各扰动区域的线性、非线性发展图像，发现低密度区域界面扰动增长较快。Bai等^[11]结合实验数据，构造初始非均匀流场，通过数值模拟研究发现，满足高斯分布的初始流场密度能准确地描述实验流场，并且经过进一步研究^[12]发现：加载前的线性和弱非线性阶段，流场的非均匀性对不稳定性的演化具有相当大的影响；而加载后的强非线性阶段，非均匀性效应急剧衰减。在之前工作^[11-13]基础上，为了深入研究初始扰动因素对非均匀流场界面不稳定性发展的影响机理，本工作利用MVFT(Multi-Viscous Flow and Turbulence)程序，通过数值模拟研究激波Ma=1.27、初始非均匀流场密度为高斯分布情况下多组初始振幅组合的双模态界面不稳定性的演化，并与均匀流场数值模拟结果进行对比，分析初始扰动及流场密度非均匀性对界面不稳定性演化的影响。

1. 计算方法

数值模拟使用适用于可压缩多介质黏性流场的大涡模拟程序MVFT，该程序可精确地模拟多介质流场中激波冲击密度间断界面的演化过程。MVFT的基本控制方程组是基于考虑黏性和热传导的可压缩多介质NS方程，通过Favre滤波后，略去非线性项，表示为如下张量形式

$\left\{ \begin{array}{l} \frac{{\partial \bar \rho }}{{\partial t}} + \frac{{\partial \bar \rho {{\tilde u}_j}}}{{\partial {x_j}}} = 0\\ \frac{{\partial \bar \rho {{\tilde u}_j}}}{{\partial t}} + \frac{{\partial \bar \rho {{\tilde u}_j}{{\tilde u}_i}}}{{\partial {x_j}}} + \frac{{\partial \bar \rho }}{{\partial {x_i}}} = \frac{{\partial \left( {{\sigma _{ij}} + {\tau _{ij}}} \right)}}{{\partial {x_j}}}\\ \frac{{\partial \bar \rho \bar E}}{{\partial t}} + \frac{{\partial \left( {\bar \rho {{\tilde u}_j}\bar E + \bar \rho {{\tilde u}_j}} \right)}}{{\partial {x_j}}} = - \frac{{\partial \left( {{q_j} + {Q_j}} \right)}}{{\partial {x_j}}} + \frac{{\partial \left[{{{\tilde u}_i}\left( {{\sigma _{ij}} + {\tau _{ij}}} \right)} \right]}}{{\partial {x_j}}}\\ \frac{{\partial {{\tilde Y}^{\left( s \right)}}}}{{\partial t}} + {{\tilde u}_j}\frac{{\partial {{\tilde Y}^{\left( s \right)}}}}{{\partial {x_j}}} = \frac{\partial }{{\partial {x_j}}}\left( {D\frac{{\partial {{\tilde Y}^{\left( s \right)}}}}{{\partial {x_j}}}} \right), \;\;\;\;s = 1, 2, 3, \cdots, N - 1 \end{array} \right.$

(1)

式中：i、j表示x、y、z方向，当i和j相同时，表示对指标进行求和；、 ${\tilde u}$ 、分别为可解尺度流体密度、流体速度、流体压强；为单位质量的总能量；N为流体的种类数； ${{{\tilde Y}^{\left( s \right)}}}$ 为第s种流体的体积分数；D为扩散系数，满足D=ν/S_c，其中ν为流体运动黏性系数，S_c为施密特数。

黏性应力张量为

${\sigma _{ij}} = {\mu _1}\left( {\frac{{\partial {{\tilde u}_i}}}{{\partial {x_j}}} + \frac{{\partial {{\tilde u}_j}}}{{\partial {x_i}}} - \frac{2}{3}{\delta _{ij}}\frac{{\partial {{\tilde u}_k}}}{{\partial {x_k}}}} \right)$

(2)

亚格子尺度(SGS)应力张量为

${\tau _{ij}} = \rho \left( {{{\tilde u}_i}{{\tilde u}_j} - \widetilde {{u_i}{u_j}}} \right)$

(3)

另外，q_j和Q_j分别为可解尺度和不可解尺度的单位时间空间的能量流，满足

$\left\{ \begin{array}{l} {q_j} = - \frac{{{\lambda _{\rm{l}}}\partial T}}{{\partial {x_j}}}, \;\;\;\;\;{\lambda _{\rm{l}}} = \frac{{{\mu _{\rm{l}}}{c_p}}}{{P{r_{\rm{l}}}}}\\ {Q_j} = - \frac{{{\lambda _{\rm{t}}}\partial T}}{{\partial {x_j}}}, \;\;\;\;\;{\lambda _{\rm{t}}} = \frac{{{\mu _{\rm{t}}}{c_p}}}{{P{r_{\rm{t}}}}} \end{array} \right.$

(4)

式中：μ为流体黏性系数，c_p为定压比热容，Pr_l和Pr_t分别为可解尺度和不可解尺度的普朗特数。

利用算子分裂技术将该物理问题分解为无黏流量计算、黏性流量计算及热流量计算3个子过程。其中无黏流量可以通过两步Lagrange-mapping型的PPM(Piecewise-Parabolic-Mapping)方法进行计算，分为以下4个步骤：(1)物理量分段抛物插值；(2)近似求解Riemann问题；(3) Lagrange方程的推进求解；(4)物理量重新映射到固定的欧拉网格。在此基础上，使用二阶空间中心差分法和两步Runge-Kutta时间推进法求解黏性流量及热流量，具体数值方法见文献[12]。

2. 计算结果与分析

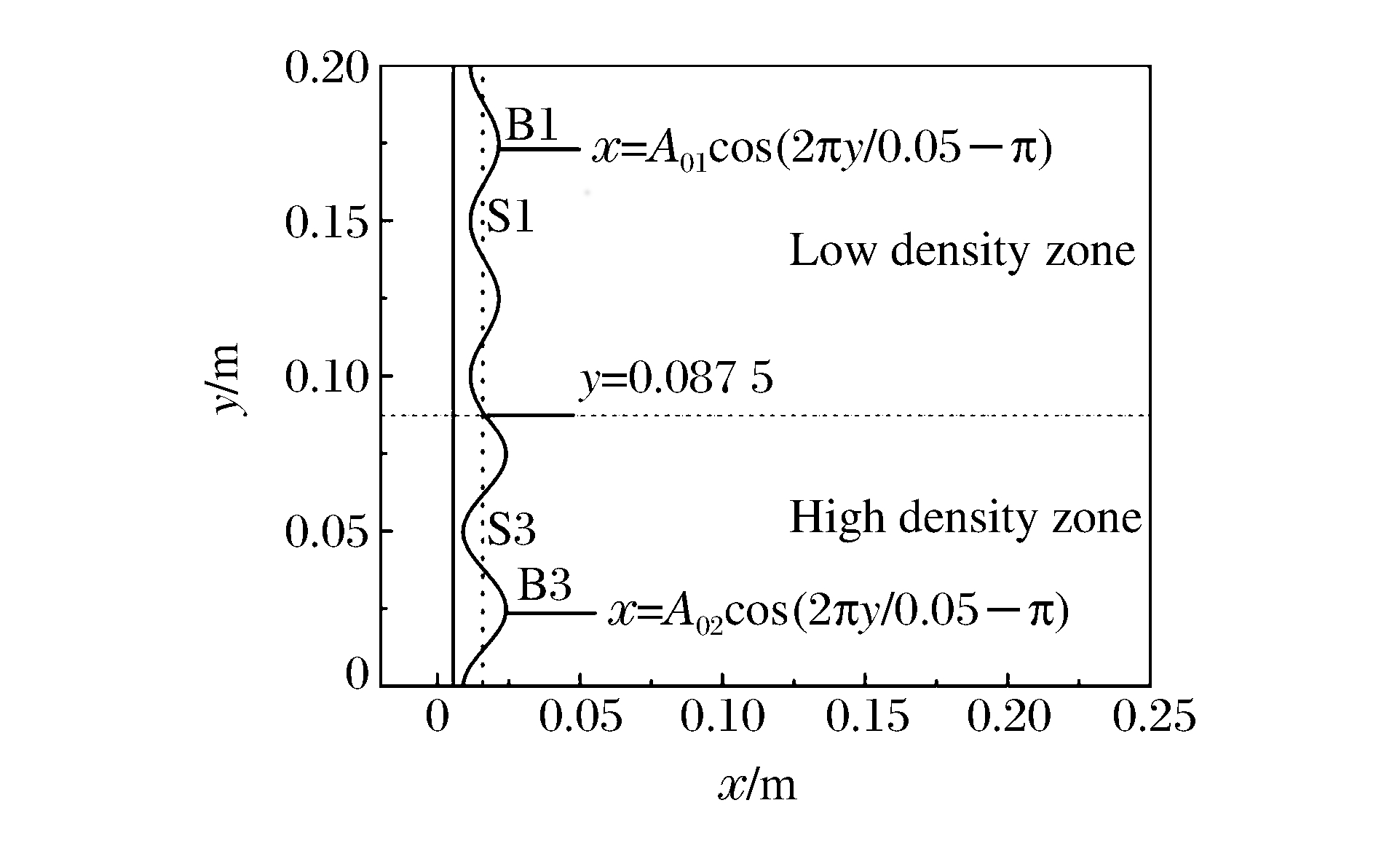
在前期工作^[10]基础上，选择20 ℃、1个标准大气压下的空气和SF₆作为界面两侧的气体，气体参数见表 1，其中γ为比热比。初始计算模型如图 1所示，界面为双模态的余弦扰动界面，波长均为0.05 m，扰动平衡位置x=0.016 m，B1和S1对应界面初始振幅为A₀₁时波峰和波谷的位置；B3和S3对应界面初始振幅为A₀₂时相应波峰和波谷的位置，虚线表示界面平衡位置。入射激波马赫数为1.27，初始激波位置在界面左侧，界面右侧为SF₆密度非均匀流场，其沿y轴方向的密度满足高斯分布，即

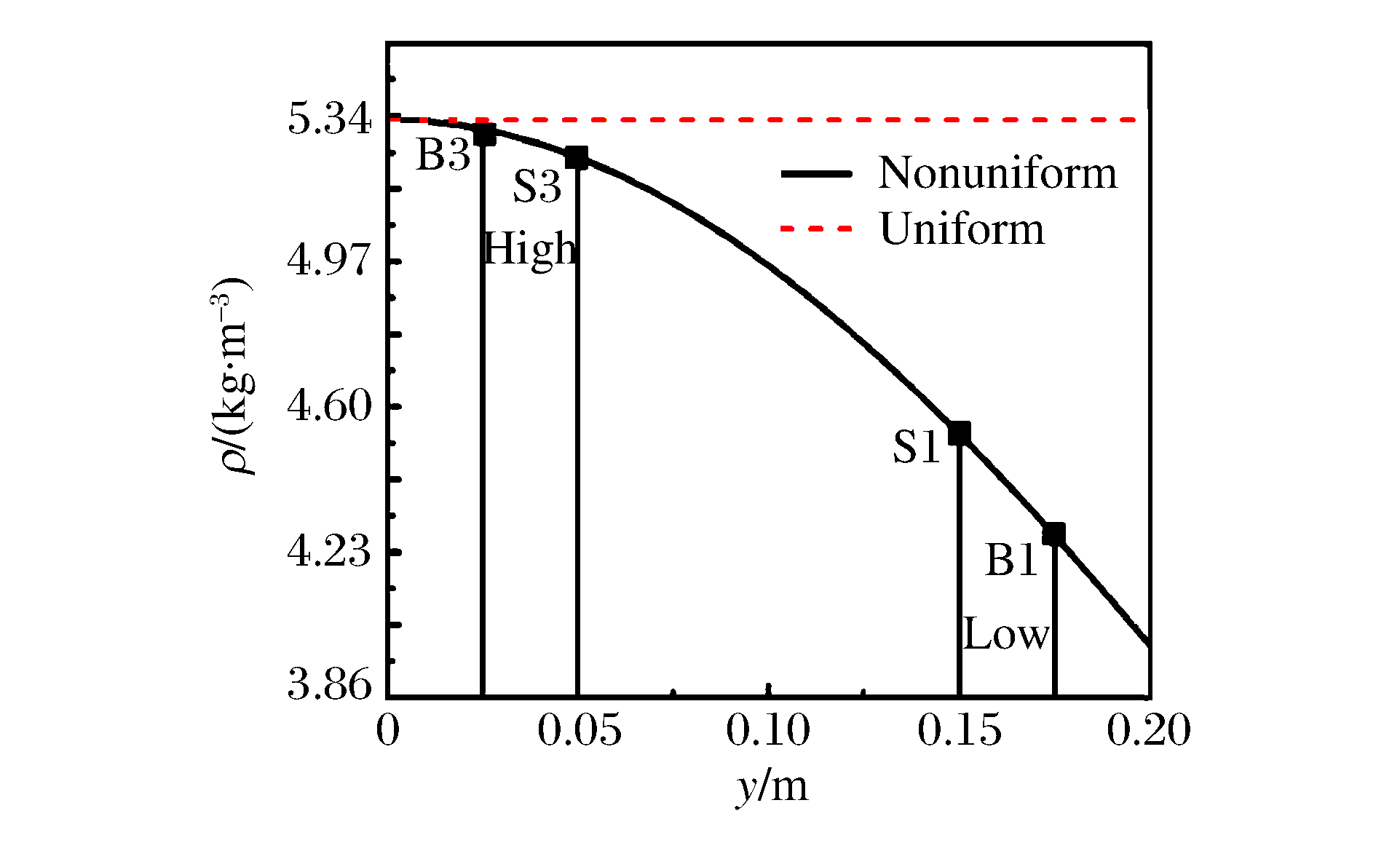
$\rho \left( y \right) = {\rho _{{\rm{S}}{{\rm{F}}_6}}}\exp \left[{-{{\left( {y-{y_{\rm{c}}}} \right)}^2}/{\delta ^2}} \right]$

(5)

表 1 气体参数

Table 1. Initial paramenters of air and SF₆

Gas	Density/(kg·m^-3)	γ	η/(10^-6 m²·s^-1)	Pr_l	D/(cm²·s^-1)
Air	1.29	1.40	15.5	0.71	0.204
SF₆	5.34	1.09	18.2	0.90	0.097

下载: 导出CSV

| 显示表格

图 1 计算模型

Figure 1. Computational model

下载: 全尺寸图片幻灯片

式中：δ=0.327 9 m，y_c=0。为了更加直观地获得密度分布，图 2给出了均匀和非均匀流场密度沿y轴方向的变化情况。

图 2 均匀和非均匀流场的y方向密度

Figure 2. Density profiles of uniform and nonuniform flows

下载: 全尺寸图片幻灯片

双模态界面的振幅如表 2所示，共设置7组初始扰动振幅组合。计算区域为[-0.02 m, 0.25 m]×[0, 0.20 m]，模拟过程中网格划分为540×400，右边界为流动边界。为了模拟界面演化至非线性阶段，取模拟时间为2 ms。界面初始振幅可以表示为

$x = \left\{ \begin{array}{l} {A_{01}}\cos \left( {2{\rm{ \mathsf{ π} }}y/0.05 - {\rm{ \mathsf{ π} }}} \right)\;\;\;\;\;\;0.0875\;{\rm{m}} < y < 0.20\;{\rm{m}}\\ {A_{02}}\cos \left( {2{\rm{ \mathsf{ π} }}y/0.05 - {\rm{ \mathsf{ π} }}} \right)\;\;\;\;\;\;0 < y \le 0.0875\;{\rm{m}} \end{array} \right.$

(6)

表 2 双模态界面的初始振幅

Table 2. Initial amplitudes of double-mode cosine interface

No.	A₀₁/mm	A₀₂/mm
1	5.0	7.5
2	7.5	5.0
3	2.5	7.5
4	7.5	2.5
5	7.5	7.5
6	7.5	10.0
7	10.0	7.5

下载: 导出CSV

| 显示表格

式中：A₀₁和A₀₂为双模态的初始振幅。为分析流场密度梯度对激波和界面的影响，首先对初始界面无扰动时的非均匀流场进行数值模拟，得到其密度分布如图 3所示。由图 3可知，由于非均匀流场y方向在1 ms时存在密度梯度，导致界面和激波的倾斜，上方激波传播速度较快。

图 3 t=1 ms时非均匀流场无扰动界面的密度云图

Figure 3. Density images of nonuniform flow without perturbation on interface at t=1 ms

下载: 全尺寸图片幻灯片

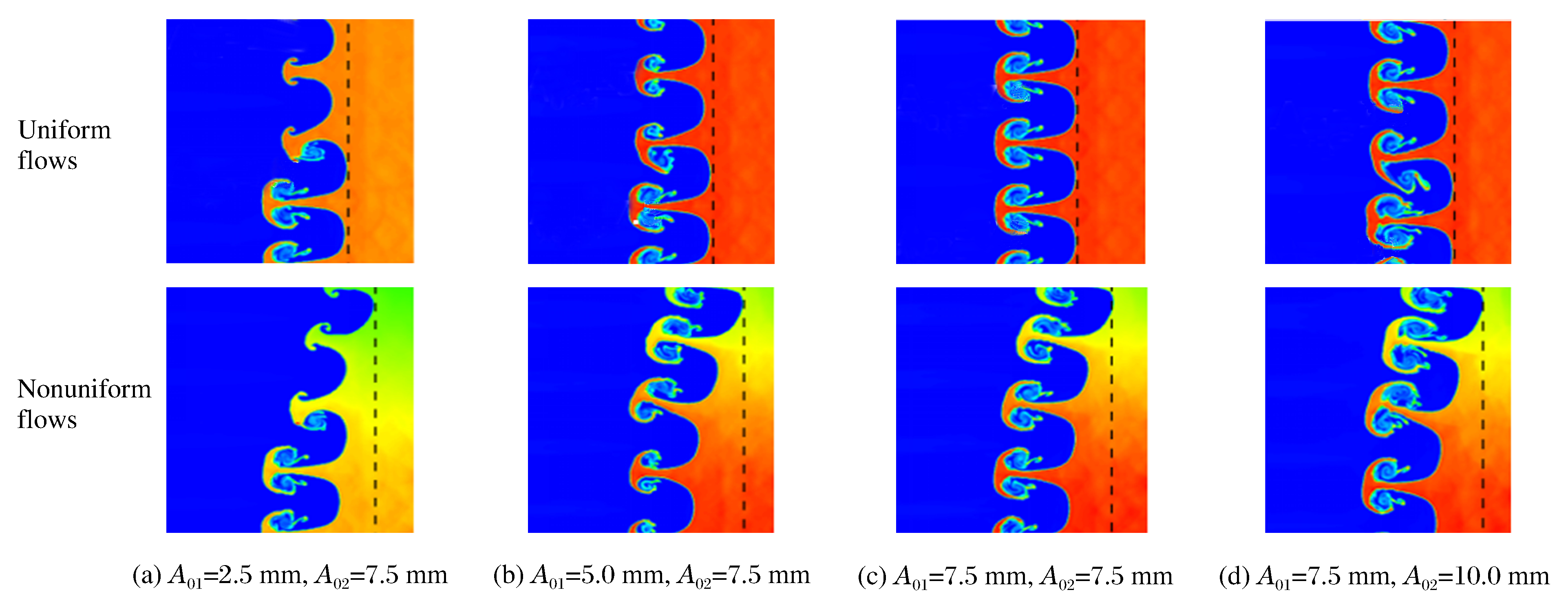
图 4给出了不同初始振幅组合下均匀和非均匀流场的密度分布。由图 4可知，均匀流场中，初始大振幅的界面演化快于初始小振幅的界面演化，但是对于非均匀流场，其演化规律出现了相反的趋势，位于非均匀流场低密度区的小振幅界面扰动赶上并超越了非均匀流场高密度区的大振幅界面，该现象说明初始流场的非均匀性对RM不稳定性的演化发展有重要的影响。

图 4 t=1 ms时不同初始振幅组合下的流场密度云图

Figure 4. Density images of uniform and nonuniform flows under different groups of initial amplitudes at t=1 ms

下载: 全尺寸图片幻灯片

2.1 振幅分析

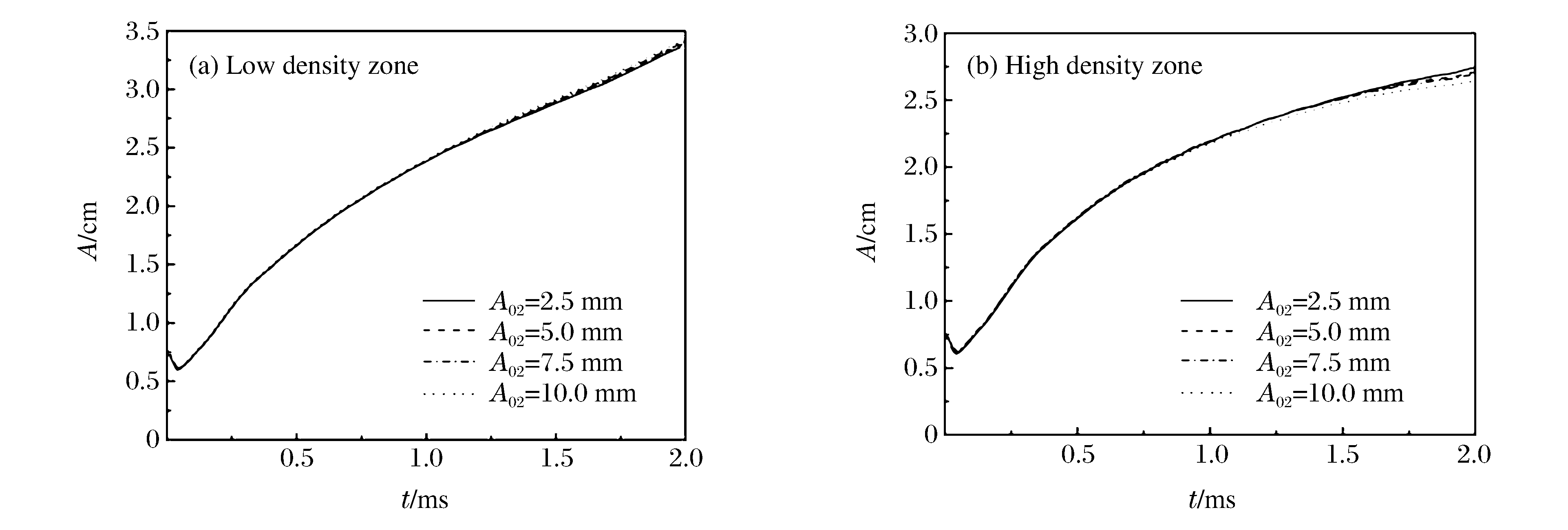
为了讨论双模态之间的耦合效应，令A₀₁=7.5 mm，将其与不同初始振幅A₀₂进行组合，计算得到高、低密度非均匀流场的振幅(A)增长情况，如图 5所示。可以看出，在非均匀流场中，界面发展后期振幅只有很小的差异，因此可以认为在所考虑的时间范围内，双模态耦合效应很弱，基本可以忽略。

图 5 在A₀₂不同情况下非均匀流场的振幅增长曲线

Figure 5. Perturbation amplitudes of nonuniform flows with 4 different amplitudes of A₀₂

下载: 全尺寸图片幻灯片

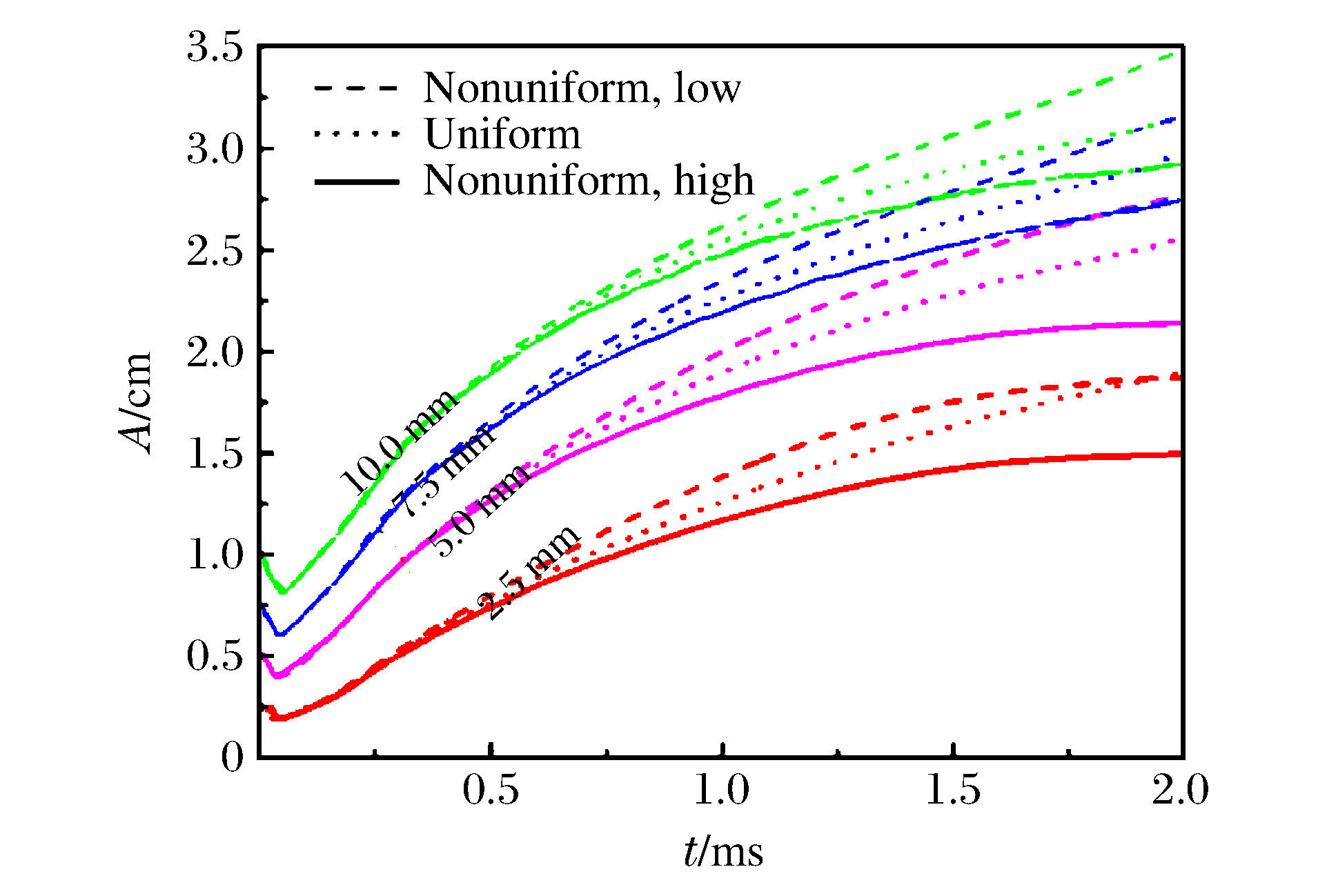
基于以上结论，对3种流场中、4种初始振幅情况下的振幅增长情况进行对比，如图 6所示。由图 6可知：均匀及非均匀流场高、低密度区中，随着初始振幅A₀(当A₀₁和A₀₂相等时，令A₀₁=A₀₂=A₀)由2.5 mm增加到10.0 mm，相应界面处的振幅增长也逐渐增加。相同初始振幅、不同密度区域条件下的振幅增长与流场的非均匀性息息相关：非均匀流场低密度区振幅较大，界面演化发展较快；高密度区振幅较小，界面演化发展较慢。以上结果与均匀流场中界面不稳定性的演化有很大区别，在均匀流场、初始振幅一定的情况下，随着密度的增大，Atwood数增加，界面扰动发展更快。

图 6 均匀流场与非均匀流场高、低密度区的振幅变化

Figure 6. Perturbation amplitudes of uniform flow and high and low density zone of nonuniform flow with different A₀

下载: 全尺寸图片幻灯片

2.2 振幅增长率分析

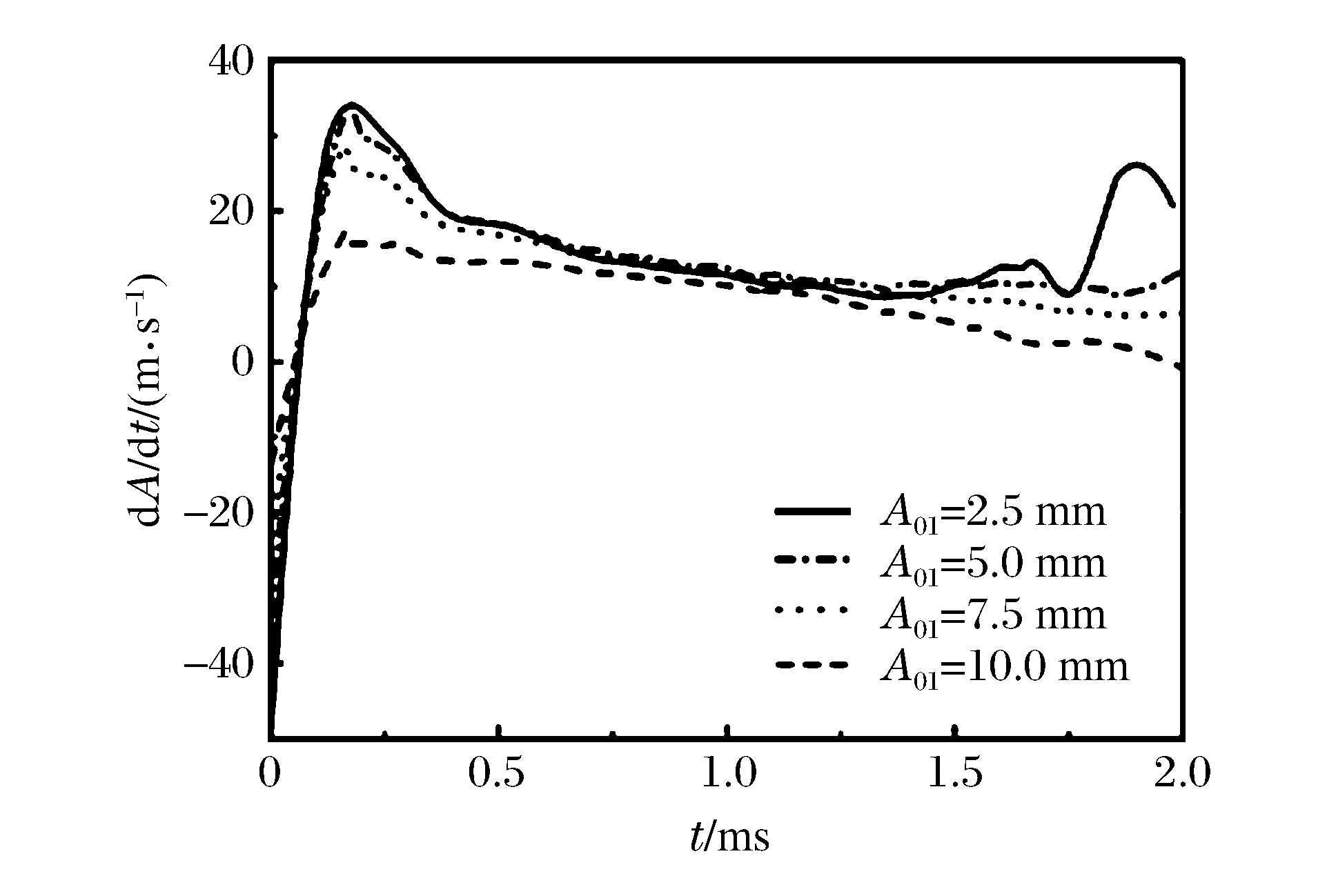
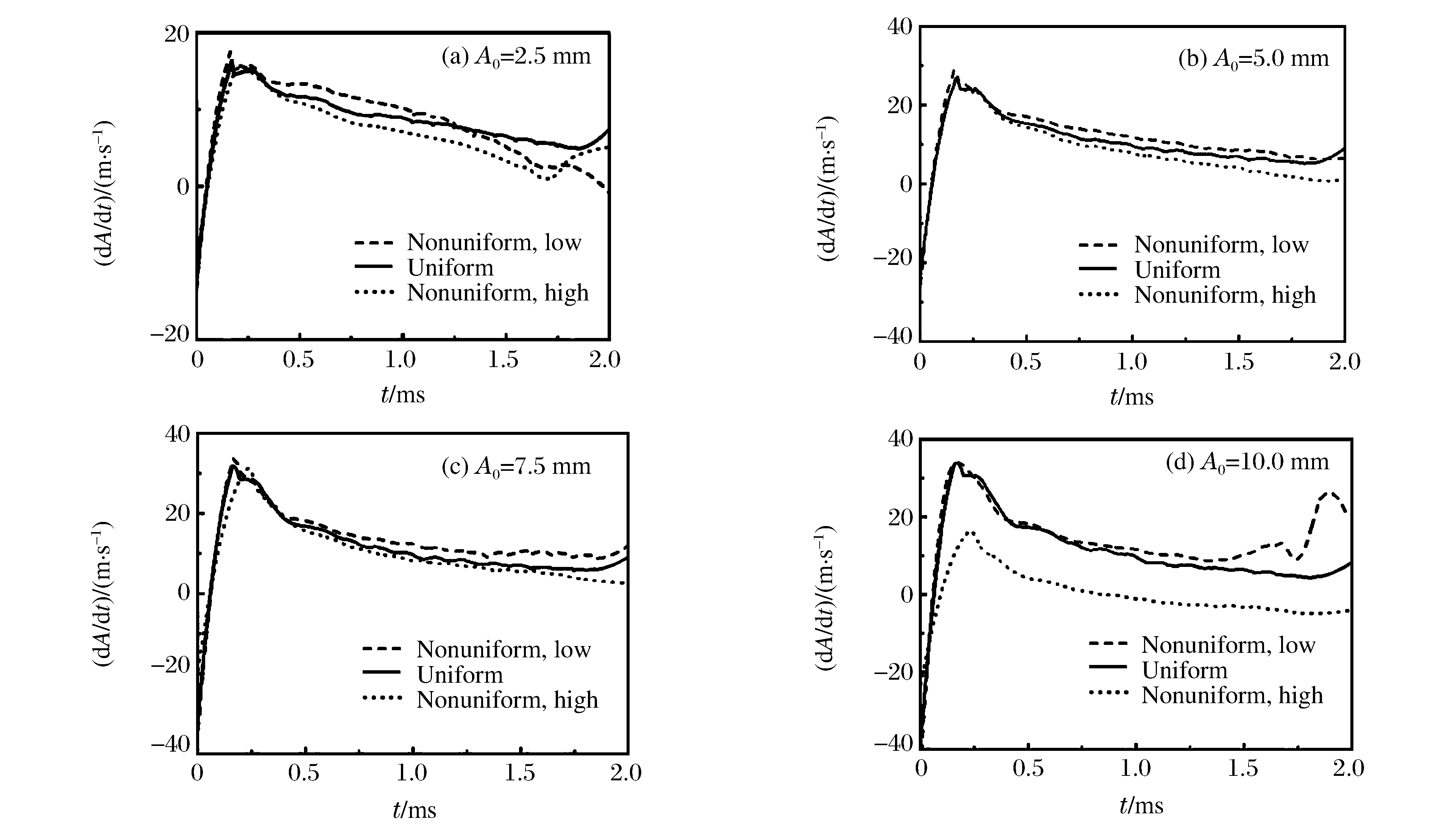
振幅增长率可以表征激波加载后界面不稳定性的增长速度。图 7为4组不同初始振幅(A₀₁)条件下非均匀流场低密度区振幅增长率随时间的变化情况。由图 7可知：不同初始振幅条件下的振幅增长率变化情况基本一致；初始时，界面振幅增长率陡增，后期振幅增长率缓慢下降。对于初始大振幅界面，其相应的振幅增长更快，振幅增长率也更大。图 8给出了非均匀流场高、低密度区和均匀流场的振幅增长率。由图 8(a)~图 8(d)可知，对于同一初始振幅而言，初始非均匀流场低密度区的振幅增长率大于其高密度区的数值，而均匀流场基本处于这两种密度流场之间，与之前所得振幅增长规律相吻合。

图 7 不同初始扰动振幅下非均匀流场界面振幅增长率

Figure 7. Growth rate over time of nonuniform flows with 4 different amplitudes of A₀₁

下载: 全尺寸图片幻灯片

图 8 非均匀流场高、低密度区及均匀流场中界面振幅增长率

Figure 8. Growth rate over time in high-density and low-density nonuniform flows and uniform flows

下载: 全尺寸图片幻灯片

从以上分析可知，振幅增长率的变化规律与振幅增长规律相吻合，初始非均匀流场低密度区的振幅增长率大于高密度区及均匀流场的振幅增长率，说明低密度区域的密度非均匀性可能引起了更加剧烈的界面振幅增长，与均匀流场呈现相反的规律。

2.3 湍动能分析

湍动能K可以表征混合区域的湍流混合强度，为得到x方向的湍动能，首先对y方向进行平均，即

$\bar a\left( {x, t} \right) = \frac{1}{N}\sum\limits_{j = 0}^{N - 1} {a\left( {x, j\Delta y, t} \right)}$

(7)

然后，计算其质量平均值，即

$a''\left( {x, y, t} \right) = a\left( {x, y, t} \right) - \tilde a\left( {x, t} \right), \;\;\;\;\tilde a\left( {x, t} \right) = \overline {\rho a\left( {x, t} \right)} /\bar \rho \left( {x, t} \right)$

(8)

由此可得湍动能 $K = \widetilde {u''{{u''}_i}}/2$ ，其中u_i"为速度u的湍流脉动量。

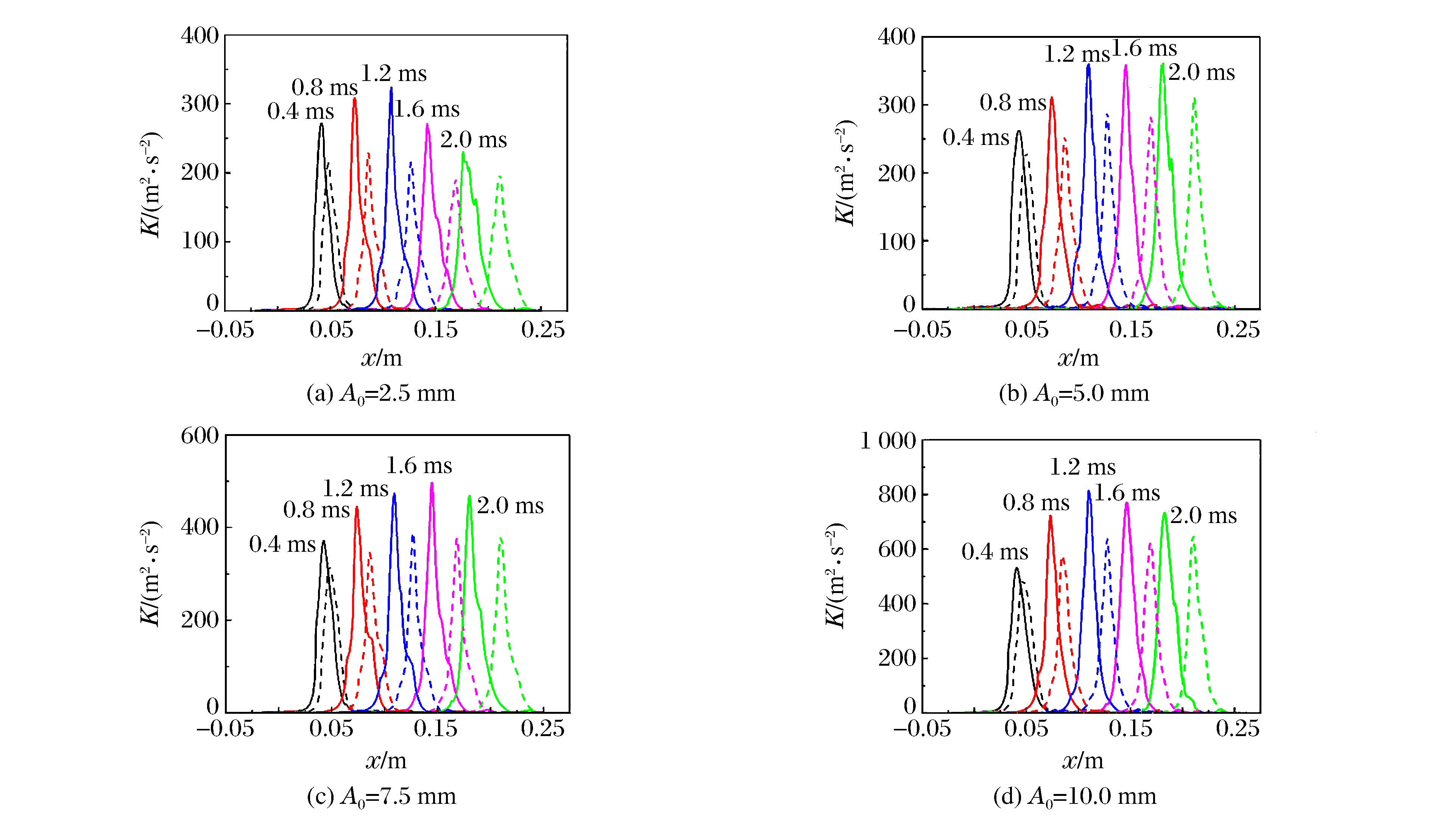
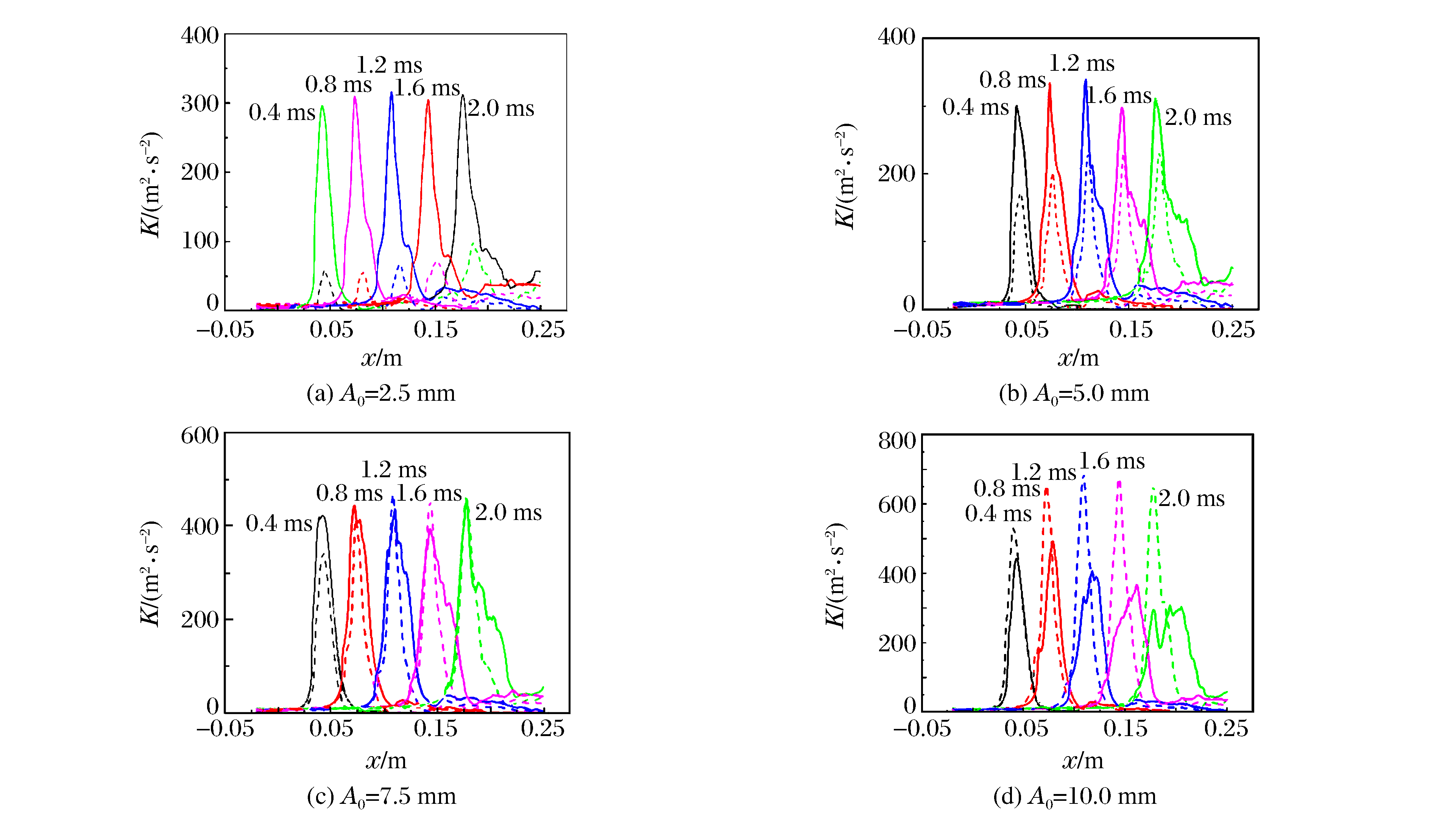
为了更清晰地对比非均匀流场和均匀流场的差异，增加了对低密度(ρ=4 kg/m³)均匀流场的数值模拟，图 9和图 10分别给出了均匀流场和非均匀流场高、低密度区湍动能随时间的变化情况。由图 9和图 10均可看出，湍动能最主要集中在湍流混合区域，混合区域随着激波传播而轴向移动。

图 9 初始均匀流场中不同初始振幅情况下的湍动能分布(实线：高密度；虚线：低密度)

Figure 9. Turbulent kinetic-energy profiles in high-density (solid line) and low-density (dashed line) uniform flows at various times under different initial amplitudes

下载: 全尺寸图片幻灯片

图 10 初始非均匀流场中不同初始振幅情况下的湍动能分布(实线：高密度；虚线：低密度)

Figure 10. Turbulent kinetic-energy profiles in high-density (dashed line) and low-density (solid line) nonuniform flows at various times under different initial amplitudes

下载: 全尺寸图片幻灯片

由图 9可知，均匀流场中，高密度区的湍动能大于低密度区的湍动能，说明高密度区的界面湍流混合程度更高，但是对于初始振幅一定、不同密度的均匀流场情况下, 其湍动能随密度变化的差值较为稳定。对于非均匀流场(见图 10)，考虑到本研究模拟时初始振幅较大，因此其湍动能的分布区域相比于于均匀流场更宽。当初始振幅在2.5~7.5 mm范围内时，低密度区域内的湍动能大于高密度区域内湍动能，并且随着初始振幅的减小，高、低密度区域内的湍动能差异愈加明显。这与之前的振幅、振幅增长率的变化趋势吻合，位于非均匀流场低密度区的界面不稳定性演化更厉害，导致更大的湍动能。但是对于初始振幅为10 mm时，湍动能变化情况(见图 10(d))不满足以上变化规律，可能是因为初始大振幅导致了湍动能统计区域较宽，湍动能分散情况较明显。

总体而言，在数值模拟时间内，均匀流场和非均匀流场高、低密度区的湍动能变化趋势具有相反的特征。均匀流场高密度区的湍动能大于低密度区的湍动能；在一定初始振幅范围内，非均匀流场的低密度区湍动能大于高密度区的湍动能，其混合范围较宽。湍动能的变化规律与之前振幅和振幅增长率的变化特征吻合较好，从能量角度解释了低密度区大振幅界面扰动增长最快、高密度区小振幅界面扰动增长最慢的现象。

2.4 斜压涡量分析

斜压涡量的沉积是界面不稳定性发展的重要机理，分析不同情况下斜压涡量的沉积可用于研究界面不稳定性的演化。斜压涡量可以表示为

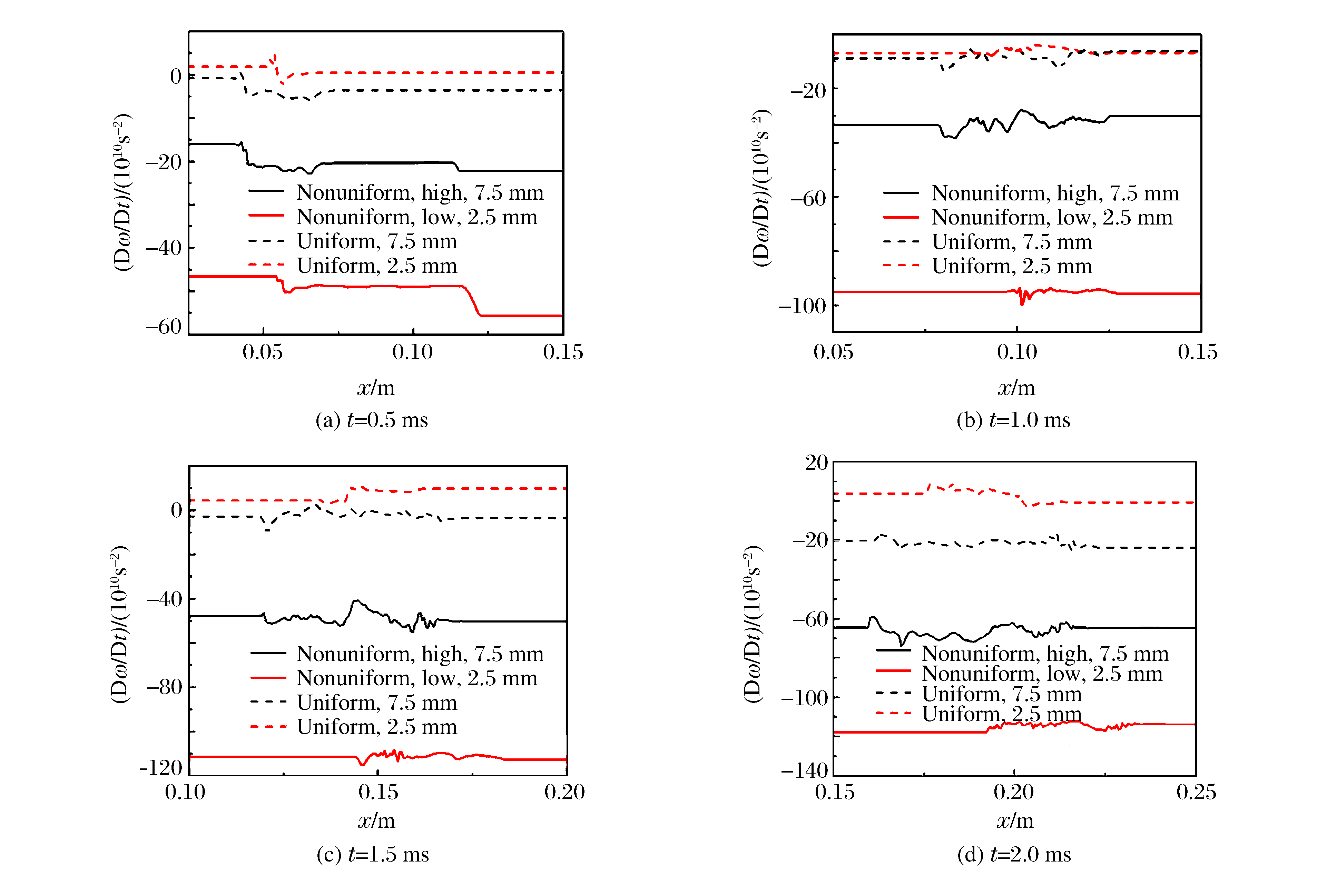
$\frac{{{\rm{D}}\mathit{\boldsymbol{\omega }}}}{{{\rm{D}}t}} = \left( {\mathit{\boldsymbol{\omega }} \cdot \nabla } \right)\mathit{\boldsymbol{U}} - \mathit{\boldsymbol{\omega }}\left( {\nabla \cdot \mathit{\boldsymbol{U}}} \right) + \frac{1}{{{\rho ^2}}}\left( {\nabla \rho \times \nabla p} \right) + \nu {\nabla ^2}\mathit{\boldsymbol{\omega }}$

(9)

忽略(9)式中的黏性项和小量，保留斜压涡产生项(▽ρ×▽p)/ρ²，通过数值计算得出相应情况下的斜压涡量。选取t=0.5、1.0、1.5、2.0 ms下，初始界面振幅组合为A₀₁=2.5 mm，A₀₂=7.5 mm中均匀和非均匀流场的斜压涡量进行对比，如图 11所示。

图 11 当A₀₁=2.5 mm，A₀₂=7.5 mm时初始均匀和非均匀流场中尖钉处的斜压涡量分布

Figure 11. Baroclinic vorticity in uniform and nonuniform flows with A₀₁=2.5 mm, A₀₂=7.5 mm at various times

下载: 全尺寸图片幻灯片

由图 11可知，4个时间点斜压涡量呈现相似的变化趋势，可总结为以下几点：(1)高斯分布下的非均匀流场，极大地促使了负斜压涡量的产生；(2)非均匀流场斜压涡量远大于均匀流场的斜压涡量；(3)在非均匀流场中，低密度区小振幅的斜压涡量大于高密度区大振幅的斜压涡量；与均匀流场呈现的趋势相反，均匀流场中斜压涡量与振幅有关，初始界面振幅越大，其斜压涡量越高。

总之，非均匀流场中，密度梯度和压力梯度的改变在一定程度和范围内促进了斜压涡量的沉积和界面的演化发展，并且低密度区斜压涡沉积量大于高密度区。上述结果可用于解释之前振幅、振幅增长率及湍动能的变化规律。

3. 结论

为研究密度非均匀流场中冲击加载双模态界面失稳现象的规律，利用MVFT-2D程序数值模拟了密度分布为高斯分布的初始非均匀流场中，Ma=1.27激波作用下双模态余弦扰动的界面不稳定性演化。一方面，在密度一定条件下，初始振幅对均匀及非均匀流场中界面不稳定性演化的影响基本一致，即初始振幅越大，界面扰动增长越快。另一方面，流场非均匀性能够引起界面各方向的密度变化和速度改变，从而导致流场中不同区域振幅增长率和湍动能的差异。在密度梯度和压力梯度共同作用下，相比于非均匀流场高密度区，低密度区的斜压涡沉积量增加，使得界面振幅较大，振幅增长率更高，混合区域范围较宽，湍动能数值较大。

由此可知，在初始振幅和流场非均匀性协同作用下，非均匀流场低密度区大振幅扰动增长较快，高密度区小振幅扰动增长较慢。在此研究基础上，后期可利用此机理，研究更接近实际流场的RM界面不稳定性的演化，构造初始非均匀流场中的平行扰动增长双模态界面等。

图 1 计算模型

Figure 1. Computational model

下载: 全尺寸图片幻灯片

图 2 均匀和非均匀流场的y方向密度

Figure 2. Density profiles of uniform and nonuniform flows

下载: 全尺寸图片幻灯片

图 3 t=1 ms时非均匀流场无扰动界面的密度云图

Figure 3. Density images of nonuniform flow without perturbation on interface at t=1 ms

下载: 全尺寸图片幻灯片

图 4 t=1 ms时不同初始振幅组合下的流场密度云图

Figure 4. Density images of uniform and nonuniform flows under different groups of initial amplitudes at t=1 ms

下载: 全尺寸图片幻灯片

图 5 在A₀₂不同情况下非均匀流场的振幅增长曲线

Figure 5. Perturbation amplitudes of nonuniform flows with 4 different amplitudes of A₀₂

下载: 全尺寸图片幻灯片

图 6 均匀流场与非均匀流场高、低密度区的振幅变化

Figure 6. Perturbation amplitudes of uniform flow and high and low density zone of nonuniform flow with different A₀

下载: 全尺寸图片幻灯片

图 7 不同初始扰动振幅下非均匀流场界面振幅增长率

Figure 7. Growth rate over time of nonuniform flows with 4 different amplitudes of A₀₁

下载: 全尺寸图片幻灯片

图 8 非均匀流场高、低密度区及均匀流场中界面振幅增长率

Figure 8. Growth rate over time in high-density and low-density nonuniform flows and uniform flows

下载: 全尺寸图片幻灯片

图 9 初始均匀流场中不同初始振幅情况下的湍动能分布(实线：高密度；虚线：低密度)

Figure 9. Turbulent kinetic-energy profiles in high-density (solid line) and low-density (dashed line) uniform flows at various times under different initial amplitudes

下载: 全尺寸图片幻灯片

图 10 初始非均匀流场中不同初始振幅情况下的湍动能分布(实线：高密度；虚线：低密度)

Figure 10. Turbulent kinetic-energy profiles in high-density (dashed line) and low-density (solid line) nonuniform flows at various times under different initial amplitudes

下载: 全尺寸图片幻灯片

图 11 当A₀₁=2.5 mm，A₀₂=7.5 mm时初始均匀和非均匀流场中尖钉处的斜压涡量分布

Figure 11. Baroclinic vorticity in uniform and nonuniform flows with A₀₁=2.5 mm, A₀₂=7.5 mm at various times

下载: 全尺寸图片幻灯片

表 1 气体参数

Table 1. Initial paramenters of air and SF₆

Gas	Density/(kg·m^-3)	γ	η/(10^-6 m²·s^-1)	Pr_l	D/(cm²·s^-1)
Air	1.29	1.40	15.5	0.71	0.204
SF₆	5.34	1.09	18.2	0.90	0.097

下载: 导出CSV

表 2 双模态界面的初始振幅

Table 2. Initial amplitudes of double-mode cosine interface

No.	A₀₁/mm	A₀₂/mm
1	5.0	7.5
2	7.5	5.0
3	2.5	7.5
4	7.5	2.5
5	7.5	7.5
6	7.5	10.0
7	10.0	7.5

下载: 导出CSV

参考文献(13)

[1]	YANG J, KUBOTA T, ZUKOSKI E E.Applications of shock-induced mixing to supersonic combustion[J].AIAA Journal, 1993, 31(5):854-862. doi: 10.2514/3.11696
[2]	ARNETT D.The role of mixing in astrophysics[J].Physics, 1999, 127(2):213-217. https://128.84.21.199/abs/astro-ph/9909031v1
[3]	AMENDT P, COLVIN J D, TIPTON R E, et al.Indirect-drive noncryogenic double-shell ignition targets for the national ignition facility:design and analysis[J].Physics of Plasmas, 2002, 9(5):2221-2233. doi: 10.1063/1.1459451
[4]	LINDL J, LANDEN O, EDWARDS J, et al.Review of the National Ignition Campaign 2009-2012[J].Physics of Plasmas, 2014, 21(2):020501. doi: 10.1063/1.4865400
[5]	SMALYUK V A, CASEY D T, CLARK D S, et al.First measurements of hydrodynamic instability growth in indirectly driven implosions at ignition-relevant conditions on the National Ignition Facility[J].Physical Review Letters, 2014, 112(18):185003. doi: 10.1103/PhysRevLett.112.185003
[6]	SMALYUK V A, TIPTON R E, PINO J E, et al.Measurements of an ablator-gas atomic mix in indirectly driven implosions at the National Ignition Facility[J].Physical Review Letters, 2014, 112(2):025002. doi: 10.1103/PhysRevLett.112.025002
[7]	KUMAR S, VOROBIEFF P, ORLICZ G, et al.Complex flow morphologies in shock-accelerated gaseous flows[J].Physica D:Nonlinear Phenomena, 2007, 235(1):21-28. https://www.sciencedirect.com/science/article/pii/S016727890700262X
[8]	ORLICZ G C, BALAKUMAR B J, TOMKINS C D, et al.A Mach number study of the Richtmyer-Meshkov instability in a varicose, heavy-gas curtain[J].Physics of Fluids, 2009, 21(6):064102. doi: 10.1063/1.3147929
[9]	ANDREWS M J. Workshop: research needs for material mixing at extremes[R]. Los Alamos National Laboratory, 2011.
[10]	刘金宏, 谭多望, 柏劲松, 等.激波管实验研究非均匀流场RM不稳定性[J].实验力学, 2012, 27(2):160-164. http://d.wanfangdata.com.cn/Periodical_sylx201202005.aspx LIU J H, TAN D W, BAI J S, et al.Experimental study of Richtmyer-Meshkov instability in nonuniform flow by shock tube[J].Journal of Experimental Mechanics, 2012, 27(2):160-164. http://d.wanfangdata.com.cn/Periodical_sylx201202005.aspx
[11]	BAI J S, LIU J H, WANG T, et al.Investigation of the Richtmyer-Meshkov instability with double perturbation interface in nonuniform flows[J].Physical Review E, 2010, 81(5):056302. doi: 10.1103/PhysRevE.81.056302
[12]	WANG T, BAI J S, LI P, et al.The numerical study of shock-induced hydrodynamic instability and mixing[J].Chinese Physics B, 2009, 18(3):1127-1135. doi: 10.1088/1674-1056/18/3/048
[13]	XIAO J X, BAI J S, WANG T.Numerical study of initial perturbation effects on Richtmyer-Meshkov instability in nonuniform flows[J].Physical Review E, 2016, 94(1):013112. doi: 10.1103/PhysRevE.94.013112