高压二氧化碳对桃果胶甲基酯酶活性的钝化效果与动力学研究

周林燕; 廖小军; 曹霞敏; 刘凤霞; 毕秀芳; 易建勇; 李淑荣

doi:10.11858/gywlxb.2014.06.017

高压二氧化碳对桃果胶甲基酯酶活性的钝化效果与动力学研究

doi: 10.11858/gywlxb.2014.06.017

周林燕^{1, 2,},
廖小军^1,*, ,,
曹霞敏¹,
刘凤霞¹,
毕秀芳¹,
易建勇²,
李淑荣²

1.
中国农业大学食品科学与营养工程学院，北京 100083
2.
中国农业科学院农产品加工研究所，北京 100193

基金项目: 国家自然科学基金(31171770)；国家863计划项目(2011AA100801)

详细信息

作者简介:
周林燕(1984—), 女，博士，助理研究员，主要从事农产品加工与贮藏研究.E-mail:zhoulinyan916@hotmail.com

通讯作者:
廖小军(1966—), 男，博士，教授，主要从事农产品加工与贮藏研究、食品非热加工研究.E-mail:liaoxjun@hotmail.com

中图分类号: O521.9;TS205
计量
- 文章访问数: 5942
- HTML全文浏览量: 1952
- PDF下载量: 297
出版历程
- 收稿日期: 2012-08-21
- 修回日期: 2012-10-08

Inactivation Kinetics of Pectin Methylesterase from Peach under High Pressure Carbon Dioxide

1.
College of Food Science and Nutritional Engineering, China Agricultural University, Beijing 100083, China
2.
Institute of Agro-Products Processing Science and Technology, Chinese Academy of Agricultural Science, Beijing 100193, China

摘要

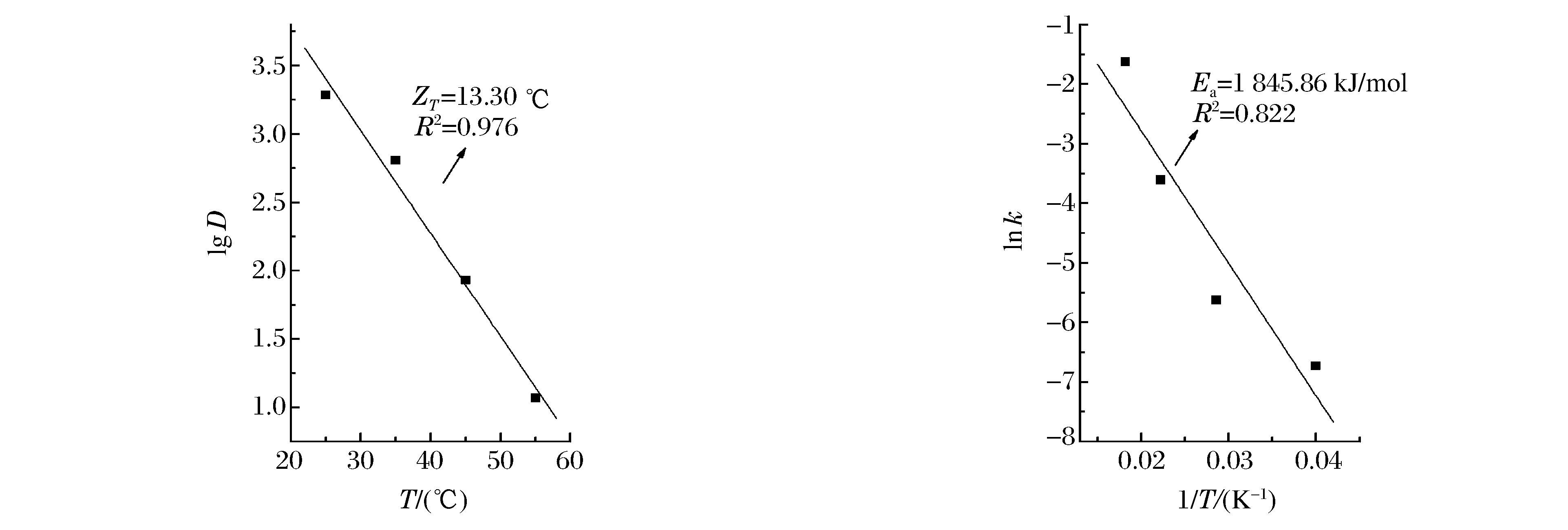
摘要: 采用高压二氧化碳技术(High Pressure Carbon Dioxide, HPCD)处理桃果胶甲基酯酶(Pectin Methylesterase, PME)粗酶液，分析了HPCD对粗酶液中PME的钝化效果及动力学，进一步比较了粗酶液和桃汁两种体系中PME对HPCD的敏感性。HPCD对粗酶液中PME具有较好的钝化效果，处理温度和压力的共同作用导致了PME活性降低，钝化动力学遵从一级动力学模型。随着处理压力和温度的提高，钝化速率逐渐增大，而指数递减时间则逐渐减小；在最优的处理条件(22 MPa、55 ℃)下，HPCD钝酶的钝化速率和指数递减时间分别为0.408 8 min^-1和5.63 min。温度为55 ℃时HPCD钝酶的压力敏感指数为16.40 MPa，活化体积为-383.00 cm³/mol；压强为15 MPa时HPCD钝酶的温度敏感指数为13.30 ℃，活化能为1 845.86 kJ/mol。比较HPCD对桃汁和粗酶液中PME的钝化效果，发现HPCD处理16 min后(15 MPa、55 ℃)，桃汁中PME残存酶活在80%左右，而粗酶液中PME活性已完全钝化，表明桃汁体系中存在保护PME活性的因素，有关机制需要进一步研究。
- 高压二氧化碳技术 /
- 果胶甲基酯酶 /
- 钝化动力学
Abstract: The inactivation of pectin methylesterase (PME) from peach in buffer and juice by high pressure carbon dioxide (HPCD) was investigated.PME in buffer is effectively inactivated by HPCD, their residual activity decreases with the increase of pressure and temperature.The residual activity of the PME exhibited a fast decrease after a prolonged treatment time; their inactivation kinetics is adequately modeled by the first order kinetics model.With the increase of pressure and temperature, the kinetic rate constant increases and the decimal reduction time decreases for PME in buffer inactivated by HPCD.The Kinetic rate and the decimal reduction time are 0.408 8 min^-1 and 5.63 min respectively at the optimal condition (22 MPa, 55 ℃).The activation volume and the pressure sensitivity exponent of inactivation PME by HPCD at 55 ℃ are -383.00 cm³/mol and 16.40 MPa respectively.In contrst, the activation energy and the pressure sensitivity exponent at 15 MPa are 1 845.86 kJ/mol and 13.30 ℃ respectively.The residual activity of PME in peach juice is significantly greater than that in buffer after the same HPCD treatment, indicating that the original PME in the juice is less inactivated by HPCD.
- high pressure carbon dioxide /
- pectin methylesterase /
- inactivation kinetics

HTML全文

功能梯度材料(Functionally Graded Materials, FGM)的概念是1984年在航空飞机计划中首次提出的^[1]，FGM的特性在于它的组成和结构随着体积的变化而变化，从而导致材料相应性质发生改变。因其材料特性呈幂律分布^[2-3]，FGM被广泛应用于工程领域，如航空航天、机械工程、生物医学等。圆柱壳在联合荷载作用下的屈曲分析备受学术界关注^[4-5]。目前，对FGM板壳的研究较为深入^[6-8]。Beni等^[9]利用改进的偶应力理论，对FGM圆柱壳在不同边界条件下的动力屈曲进行了分析；Kargarnovin等^[10]研究了轴向荷载作用下FGM圆柱壳的动力屈曲；Sofiyev等^[11]研究了横向压力下功能梯度正交各向异性圆柱壳的动力屈曲，推导出基于一阶剪切变形理论的功能梯度正交各向异性圆柱壳的稳定性和相容性方程；Khazaeinejad等^[12]研究了弹性模量在厚度方向上连续变化的FGM圆柱壳在复合外压和轴向压缩载荷作用下的动力屈曲；Khalili等^[13]研究了横向冲击载荷作用下FGM圆柱壳的动力屈曲；Alashti等^[14]对变厚度FGM圆柱壳外压和轴向压缩的动力屈曲问题进行了分析。

基于以上研究，本研究讨论了FGM圆柱壳在轴向荷载作用下的动力屈曲。根据Donnell壳体理论和经典板壳理论，利用Hamilton变分原理得到FGM圆柱壳的动力屈曲控制方程；采用分离变量法求得动力屈曲临界荷载表达式；通过MATLAB软件计算动力屈曲临界荷载，讨论由不同材料(陶瓷和钛、陶瓷和铁、陶瓷和铜)组成的FGM圆柱壳的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)等对临界荷载的影响。

1. FGM圆柱壳的屈曲控制方程

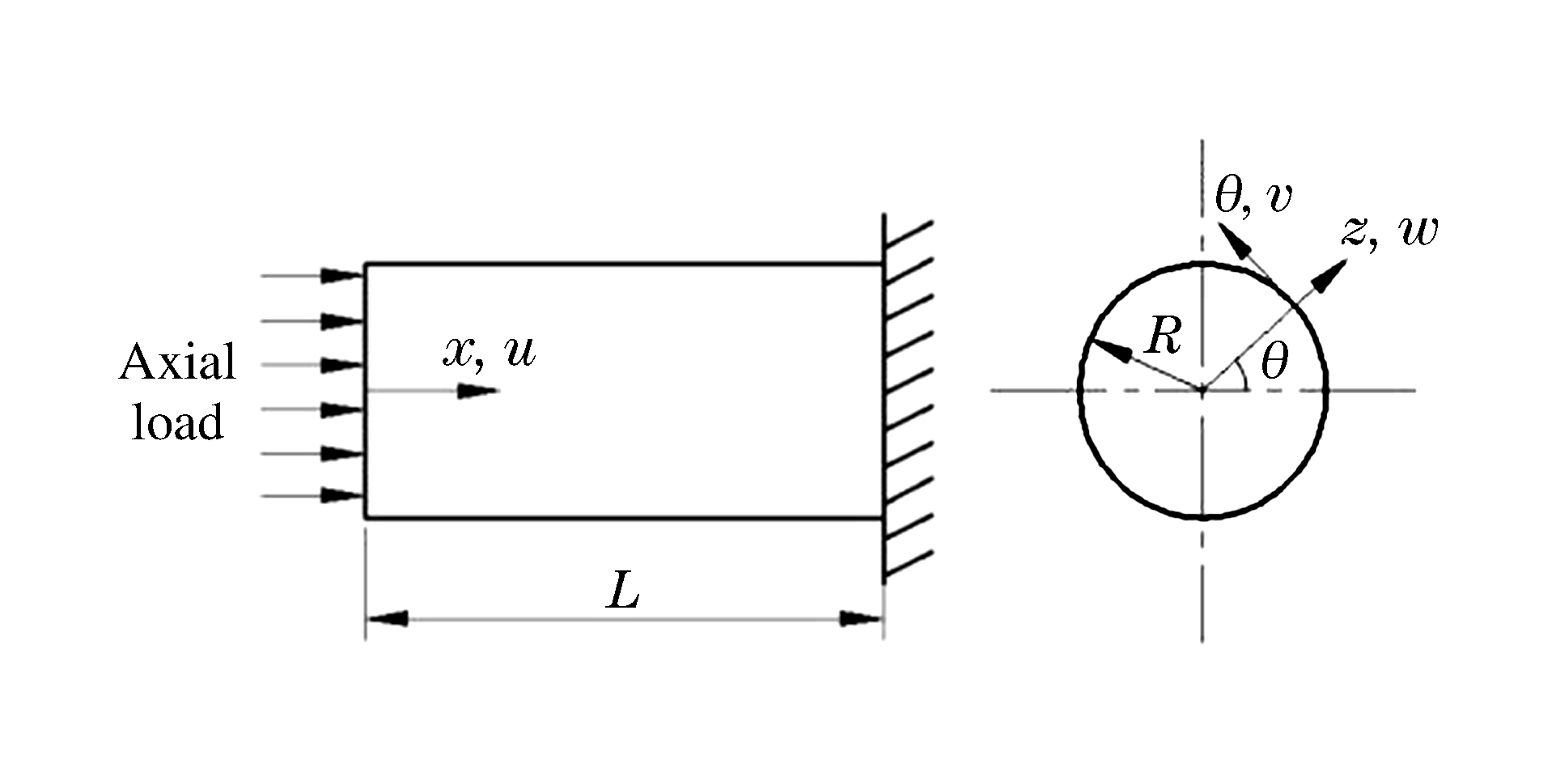
如图 1所示，FGM圆柱壳长度为l，半径为R，总厚度为h，选取柱坐标系(x, θ, z)，其相应位移为(u, v, w)。FGM的材料属性(弹性模量E、密度ρ、泊松比μ等)呈幂律分布^[2-3]，表示为

$P\left( z \right) = \left( {{P_1} - {P_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {P_2}$

(1)

图 1 圆柱壳坐标系统

Figure 1. Cylindrical shell coordinates

下载: 全尺寸图片幻灯片

式中：P为物性参数，下标“1”和“2”分别代表组分1和组分2；k为梯度指数，k∈(0, ∞)。圆柱壳内任意点的物性参数为

$\left\{ \begin{array}{l} E\left( z \right) = \left( {{E_1} - {E_2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {E_2}\\ \rho \left( z \right) = \left( {{\rho _1} - {\rho _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\rho _2}\\ \mu \left( z \right) = \left( {{\mu _1} - {\mu _2}} \right){\left( {\frac{{2z + h}}{{2h}}} \right)^k} + {\mu _2} \end{array} \right.$

(2)

根据Donnell壳体理论，圆柱壳的小挠度几何方程为

$\begin{array}{*{20}{c}} {\left\{ \begin{array}{l} {\varepsilon _x} = \varepsilon _x^0 + z{K_x}\\ {\varepsilon _\theta } = \varepsilon _\theta ^0 + z{K_\theta }\\ {\gamma _{x\theta }} = \gamma _{x\theta }^0 + z{K_{x\theta }} \end{array} \right.,}&{\left\{ \begin{array}{l} u = {u_0} - z\frac{{\partial w}}{{\partial x}}\\ v = {v_0} - z\frac{{\partial w}}{{R\partial \theta }}\\ w = {w_0} \end{array} \right.,}&{\left\{ \begin{array}{l} \varepsilon _x^0 = \frac{{\partial {u_0}}}{{\partial x}}\\ \varepsilon _\theta ^0 = \frac{{\partial {v_0}}}{{R\partial \theta }} - \frac{{{w_0}}}{R}\\ \gamma _{x\theta }^0 = \frac{{\partial {u_0}}}{{R\partial \theta }} - \frac{{\partial {v_0}}}{{\partial x}} \end{array} \right.,}&{\left\{ \begin{array}{l} {K_x} = - \frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}}\\ {K_\theta }{\rm{ = }} - \frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}}\\ {K_{x\theta }}{\rm{ = }} - \frac{{2{\partial ^2}{w_0}}}{{R\partial x\partial \theta }} \end{array} \right.} \end{array}$

(3)

式中：ε为正应变，γ为切应变，上、下标“0”表示壳体中面，K为壳体曲率。

根据经典板壳理论，FGM圆柱壳的内力N与内力矩M可表示为

$\left( {\begin{array}{*{20}{c}} {{N_x}}\\ {{N_\theta }}\\ {{N_{x\theta }}}\\ {{M_x}}\\ {{M_\theta }}\\ {{M_{x\theta }}} \end{array}} \right) = \left( {\begin{array}{*{20}{c}} {{A_{11}}}&{{A_{12}}}&{{A_{16}}}&{{B_{11}}}&{{B_{12}}}&{{B_{16}}}\\ {{A_{21}}}&{{A_{22}}}&{{A_{26}}}&{{B_{21}}}&{{B_{22}}}&{{B_{26}}}\\ {{A_{16}}}&{{A_{26}}}&{{A_{66}}}&{{B_{16}}}&{{B_{26}}}&{{B_{66}}}\\ {{B_{11}}}&{{B_{12}}}&{{B_{16}}}&{{D_{11}}}&{{D_{12}}}&{{D_{16}}}\\ {{B_{21}}}&{{B_{22}}}&{{B_{26}}}&{{D_{21}}}&{{D_{22}}}&{{D_{26}}}\\ {{B_{16}}}&{{B_{26}}}&{{B_{66}}}&{{D_{16}}}&{{D_{26}}}&{{D_{66}}} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {\varepsilon _x^0}\\ {\varepsilon _\theta ^0}\\ {\gamma _{x\theta }^0}\\ {{K_x}}\\ {{K_\theta }}\\ {{K_{x\theta }}} \end{array}} \right)$

(4)

式中：A_ij、B_ij、D_ij(i, j=1, 2, 6)分别为FGM圆柱壳的拉伸刚度、耦合刚度和弯曲刚度系数矩阵分量。 ${A_{11}} = {A_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{12}} = {A_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}} {\rm{d}}z$ , ${A_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}} {\rm{d}}z$ , ${B_{11}} = {B_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;}{\rm{d}}z$ , ${B_{12}} = {B_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}z\;} {\rm{d}}z$ , ${B_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}z\;} {\rm{d}}z$ , ${D_{11}} = {D_{22}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{12}} = {D_{21}} = \int_{ - h/2}^{h/2} {\frac{{\mu \left( z \right)E\left( z \right)}}{{1 - {\mu ^2}\left( z \right)}}{z^2}\;} {\rm{d}}z$ , ${D_{66}} = \int_{ - h/2}^{h/2} {\frac{{E\left( z \right)}}{{2\left[ {1 + \mu \left( z \right)} \right]}}{z^2}\;} {\rm{d}}z$ 。FGM圆柱壳的力学性能为各向同性^[15]，那么：A₁₆=A₂₆=B₁₆=B₂₆=D₁₆=D₂₆=0。

对于圆柱壳，系统的应变能(不考虑剪力)为

$U = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\left( {{N_x}\varepsilon _x^0 + {N_\theta }\varepsilon _\theta ^0 + {N_{x\theta }}\gamma _{x\theta }^0 + {M_x}{K_x} + {M_\theta }{K_\theta } + {M_{x\theta }}{K_{x\theta }}} \right)} R{\rm{d}}x{\rm{d}}\theta }$

(5)

动能为

$T = \frac{1}{2}\int_{ - h/2}^{h/2} {\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {\rho \left( z \right)\left[ {{{\left( {\frac{{\partial u}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial v}}{{\partial t}}} \right)}^2} + {{\left( {\frac{{\partial w}}{{\partial t}}} \right)}^2}} \right]R{\rm{d}}x{\rm{d}}\theta {\rm{d}}z} } }$

(6)

外力功为

$W = \frac{1}{2}\int_0^{2{\rm{ \mathsf{ π} }}} {\int_0^l {N\left( t \right){{\left( {\frac{{\partial {w_0}}}{{\partial x}}} \right)}^2}} R{\rm{d}}x{\rm{d}}\theta }$

(7)

Hamilton变分原理为

${\rm{ \mathsf{ δ} }}\int_{{t_2}}^{{t_1}} {\left( {T - U + W} \right){\rm{d}}t} = 0$

(8)

将(3)式~(7)式代入(8)式中，由Donnell壳体理论可知，圆柱壳内力沿环向均匀分布，忽略中面位移^[16]，由u₀、v₀、w₀的变分系数为零，整理得到FGM圆柱壳的动力屈曲控制方程为

$\begin{array}{l} 4{I_0}\frac{{{\partial ^2}{w_0}}}{{\partial t}} - {I_2}\left( {\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {\theta ^2}\partial {t^2}}} + \frac{{{\partial ^4}{w_0}}}{{\partial {x^2}\partial {t^2}}}} \right) = - 4{A_{22}}\frac{{{w_0}}}{{{R^2}}} - 4\frac{{{B_{12}}}}{{{R^2}}}\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} - 4\frac{{{B_{22}}}}{R}\frac{{{\partial ^2}{w_0}}}{{{R^2}\partial {\theta ^2}}} - {D_{11}}\frac{{{\partial ^4}{w_0}}}{{\partial {x^4}}} - \\ \;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;\;2\left( {{D_{12}} + 2{D_{66}}} \right)\frac{{{\partial ^4}{w_0}}}{{{R^2}\partial {x^2}\partial {\theta ^2}}} - {D_{22}}\frac{{{\partial ^4}{w_0}}}{{{R^4}\partial {\theta ^4}}} + N\left( t \right)\frac{{{\partial ^2}{w_0}}}{{\partial {x^2}}} \end{array}$

(9)

2. 控制方程的求解

设径向位移表示为^[17]

$w = Y\left( x \right)T\left( t \right){{\rm{e}}^{{\rm{i}}m\theta }}$

(10)

将(10)式代入(9)式中，分离变量得

$\left\{ \begin{array}{l} {Y^{\left( 4 \right)}} = {\alpha ^2}Y'' + {\beta ^2}Y = 0\\ \ddot T - \lambda T = 0 \end{array} \right.$

(11)

其中

${\alpha ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{B_{12}}}}{{{R^2}}} + \frac{2}{{{R^2}}}\left( {{D_{12}} + 2{D_{66}}} \right){m^2} - N\left( t \right) - {I_2}\lambda } \right]$

(12)

${\beta ^2} = \frac{1}{{{D_{11}}}}\left[ {4\frac{{{A_{22}}}}{{{R^2}}} + 4\frac{{{B_{22}}}}{{{R^3}}} + \frac{{{D_{22}}}}{{{R^4}}}{m^4} + 4{I_0}\lambda - \frac{{{I_2}}}{{{R^2}}}{m^2}\lambda } \right]$

(13)

当α⁴＞4β²＞0且λ＞0时，圆柱壳屈曲^[18-20]，其动力屈曲解为

$Y\left( x \right) = {C_1}\sin \left( {{k_1}x} \right) + {C_2}\cos \left( {{k_1}x} \right) + {C_3}\sin \left( {{k_2}x} \right) + {C_4}\cos \left( {{k_2}x} \right)$

(14)

式中：C₁~C₄为系数， ${k_1} = \sqrt {\frac{{{\alpha ^2} - \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}} , {k_2} = \sqrt {\frac{{{\alpha ^2} + \sqrt {{\alpha ^4} - 4{\beta ^2}} }}{2}}$ 。

(14)式满足下列两种边界条件：

(1) 对于一端夹支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y'\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(15)

(2) 对于一端简支另一端固支的圆柱壳，其边界条件为

$\left\{ \begin{array}{l} Y\left( 0 \right) = Y''\left( 0 \right) = 0\\ Y\left( l \right) = Y'\left( l \right) = 0 \end{array} \right.$

(16)

将(14)式代入(15)式中，整理得到如下齐次线性方程组

$\left( {\begin{array}{*{20}{c}} 0&1&0&1\\ {{k_1}}&0&{{k_2}}&0\\ {\sin \left( {{k_1}l} \right)}&{\cos \left( {{k_1}l} \right)}&{\sin \left( {{k_2}l} \right)}&{\cos \left( {{k_2}l} \right)}\\ {{k_1}\cos \left( {{k_1}l} \right)}&{ - {k_1}\sin \left( {{k_1}l} \right)}&{{k_2}\cos \left( {{k_2}l} \right)}&{ - {k_2}\sin \left( {{k_2}l} \right)} \end{array}} \right)\left( {\begin{array}{*{20}{c}} {{C_1}}\\ {{C_2}}\\ {{C_3}}\\ {{C_4}} \end{array}} \right) = 0$

(17)

若要(17)式有非平凡解，其系数行列式必为零，于是

$2{k_1}{k_2} - 2{k_1}{k_2}\cos \left( {{k_1}l} \right)\cos \left( {{k_2}l} \right) - \left( {k_1^2 + k_2^2} \right)\sin \left( {{k_1}l} \right)\sin \left( {{k_2}l} \right) = 0$

(18)

由k₁和k₂可得

$\left\{ \begin{array}{l} k_1^2 + k_2^2 = \left( {n_1^2 + n_2^2} \right){{\rm{ \mathsf{ π} }}^2}/{l^2} = {\alpha ^2}\\ k_1^2k_2^2 = n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}/{l^4} = {\beta ^2} \end{array} \right.$

(19)

将(19)式代入(12)式和(13)式中，得一端夹支另一端固支时FGM圆柱壳动力屈曲临界荷载N_cr，即

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} - \frac{{{B_{12}}}}{{{R^2}}} + \frac{{2\left( {{D_{12}} + 2{D_{66}}} \right){m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} + 4{B_{22}}{m^2}{R^2}{l^4} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(20)

式中：n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+2。

同理可得一端简支另一端固支时FGM圆柱壳动力屈曲临界荷载

(21)

此时，n₁=n=1, 2, 3, …；m=1, 2, 3, …；n₂=n+1。

将FGM退化成金属材料，得到金属材料圆柱壳动力屈曲临界荷载

${N_{{\rm{cr}}}} = \frac{{{D_{11}}{{\rm{ \mathsf{ π} }}^2}\left( {n_1^2 + n_2^2} \right)}}{{{l^2}}} + \frac{{2{D_{12}}{m^2}}}{{{R^2}}} + \frac{{{h^2}\left( {{D_{11}}n_1^2n_2^2{{\rm{ \mathsf{ π} }}^4}{R^4} - {A_{22}}{l^4}{R^2} - {D_{22}}{l^4}{m^4}} \right)}}{{{l^4}{R^2}\left( {{h^2}{m^2} - 12{R^2}} \right)}}$

(22)

(22)式与文献[16]中的表达式相同。

根据(10)式，取一端夹支另一端固支时圆柱壳动力屈曲解的表达式^[16]

$w = T\left( t \right)\left[ {\sin \left( {\frac{{{n_1}{\rm{ \mathsf{ π} }}x}}{l}} \right) - \frac{{{n_1}}}{{{n_2}}}\sin \left( {\frac{{{n_2}{\rm{ \mathsf{ π} }}x}}{l}} \right)} \right]\sin \left( {m\theta } \right)$

(23)

将(23)式代入控制方程(9)式中，计算并化简整理得到临界荷载表达式

${N_{{\rm{cr}}}} = \frac{{\left( {n_1^2 + n_2^2} \right){D_{11}}{{\rm{ \mathsf{ π} }}^2}}}{{{l^2}}} + \frac{{2{m^2}\left( {{D_{12}} + 2{D_{66}}} \right)}}{{{R^2}}}$

(24)

(24)式与不考虑转动惯量时用分离变量得到的结果相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+2。同理可得当边界条件为一端简支另一端固支时的临界荷载表达式，与(24)式相同，此时n₁=n=1, 2, 3, …; m=1, 2, 3, …; n₂=n+1。

3. 算例分析

采用MATLAB软件编程，对FGM圆柱壳动力屈曲临界荷载进行计算。讨论由不同材料(陶瓷-钛、陶瓷-铁、陶瓷-铜)组成的FGM圆柱壳(见图 2)的径厚比(R/h)、梯度指数(k)、环向模态数(m)、轴向模态数(n)对临界荷载N_cr的影响。基本材料参数如表 1所示。

图 2 材料沿壁厚分布

Figure 2. Distribution of material along wall thickness

下载: 全尺寸图片幻灯片

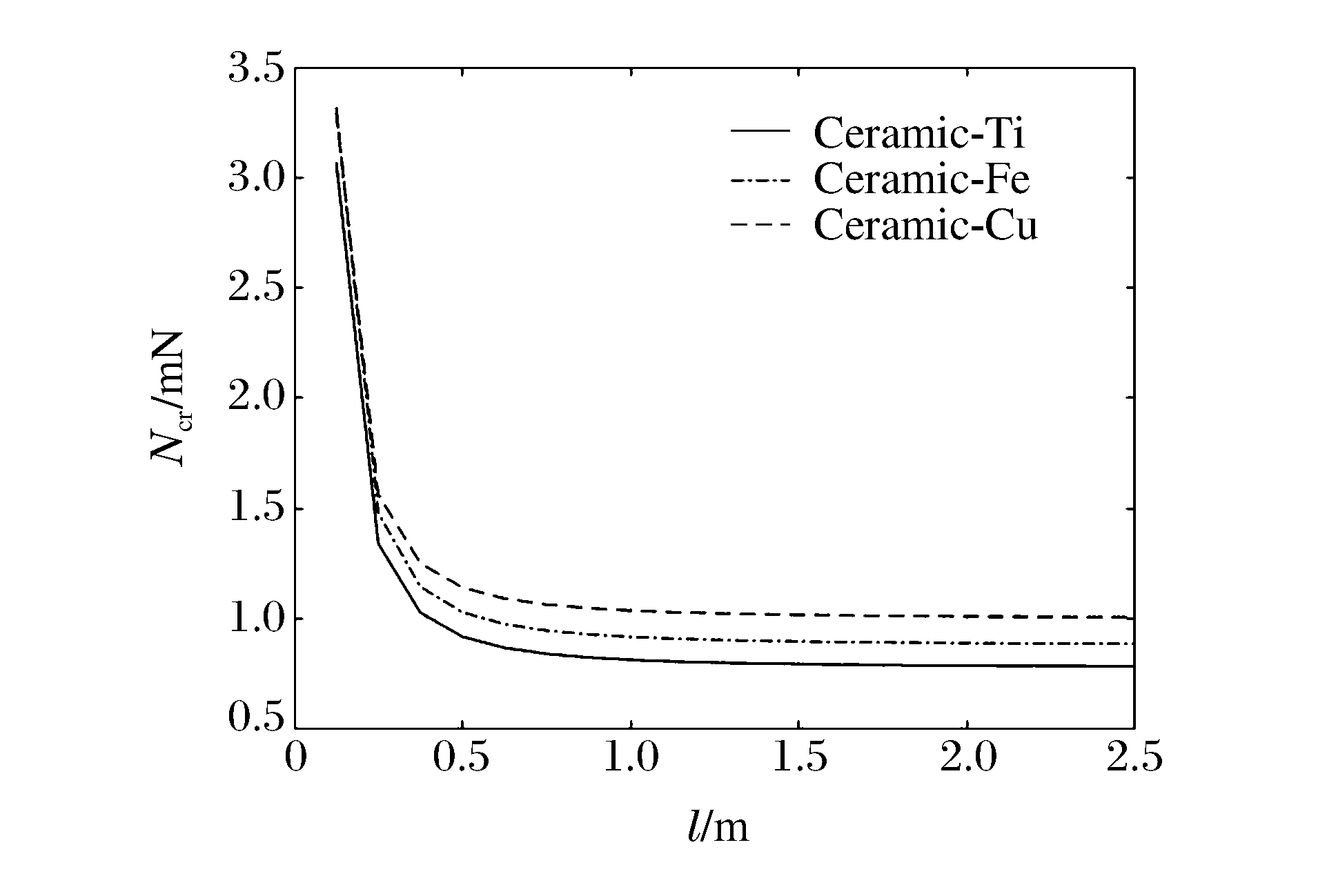
表 1 材料参数

Table 1. Material parameters

Material	E/GPa	ρ/(g·cm^-3)	μ
Ceramic	385	3.96	0.230
Ti	109	4.54	0.410
Fe	155	7.86	0.291
Cu	119	8.96	0.326

下载: 导出CSV

| 显示表格

图 3表示n=1、m=2、k=1、R/h=20时，不同材料组成下N_cr与临界长度l(本研究中临界长度即为圆柱壳长度)的关系曲线。从图 3可以看出：N_cr随l的增加而减小；当l＜0.5 m时，N_cr随l的增加而迅速减小；当l＞0.5 m时，N_cr随l的增大缓慢减小，且逐渐趋于常数；同一l下，陶瓷-铜的N_cr最大，陶瓷-铁次之，陶瓷-钛的N_cr最小。以下均以陶瓷-钛为例进行讨论。

图 3 不同材料组成下临界荷载与临界长度的关系

Figure 3. Critical load vs.critical length under different material composition conditions

下载: 全尺寸图片幻灯片

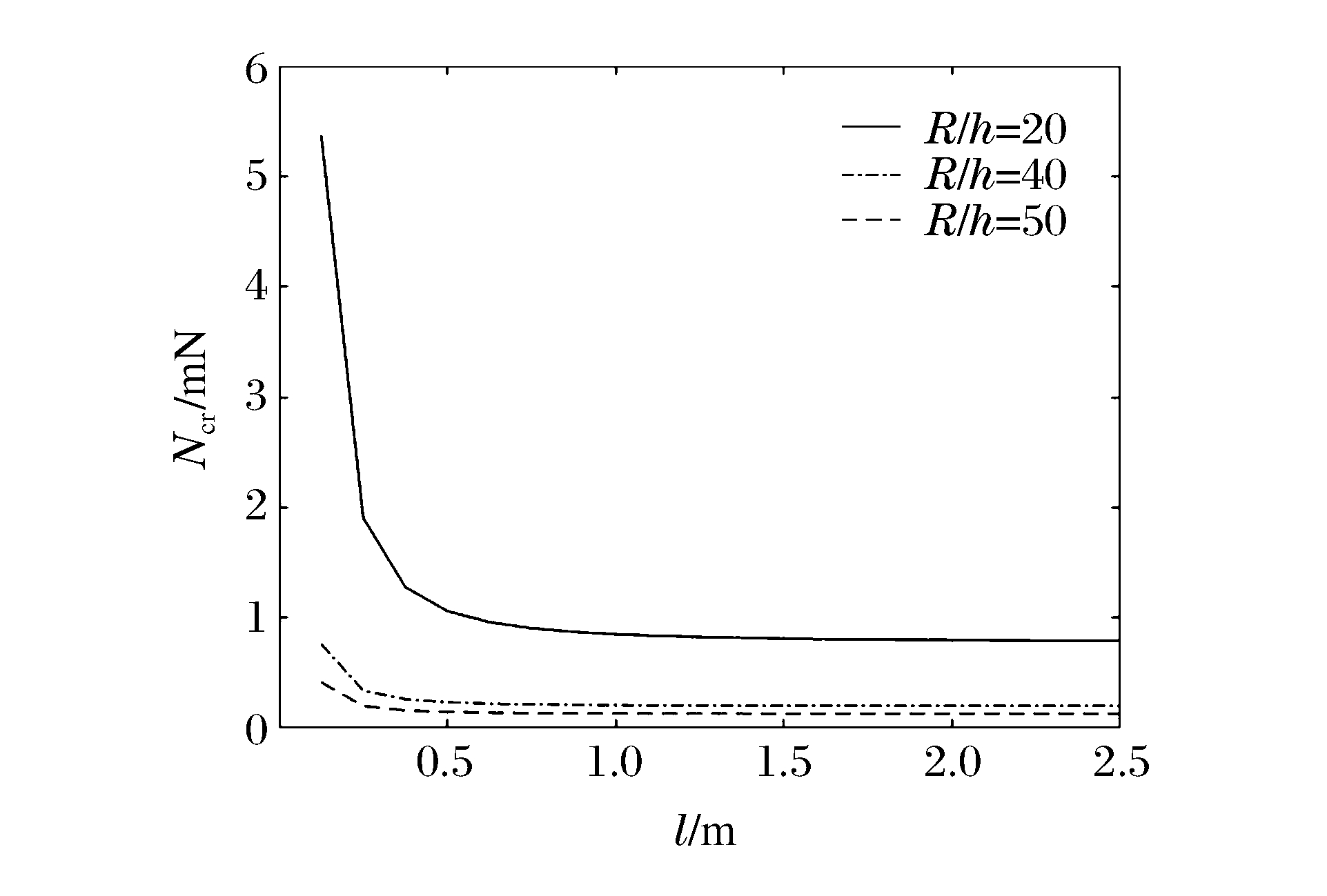
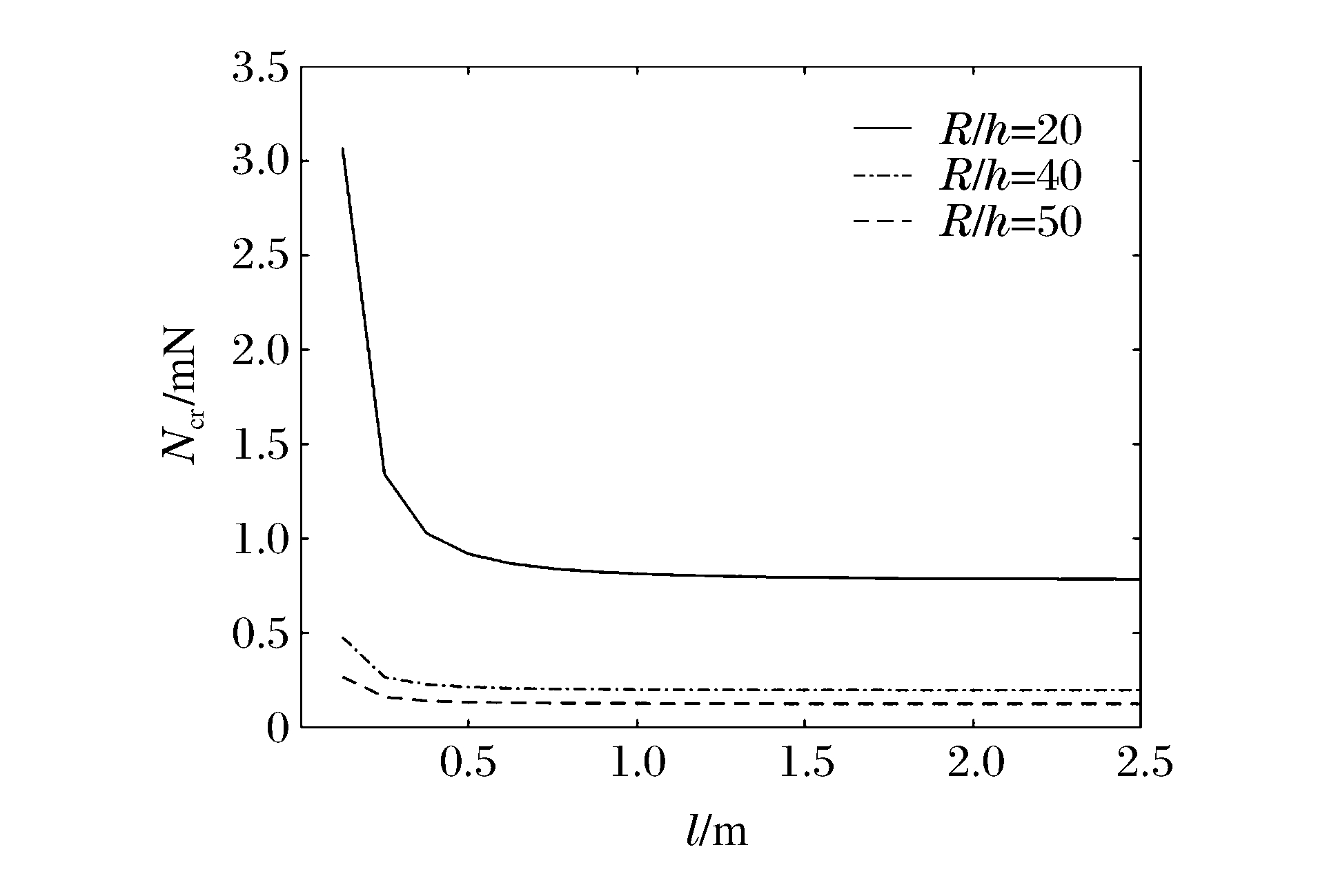
图 4和图 5分别表示冲击端为夹支和简支时n=1、m=2、k=1时不同R/h下N_cr与l的关系曲线。可以看出：当l增加时，N_cr减小，且逐渐趋于常数；在同一l下圆柱壳的N_cr随着R/h的增大而减小；当R/h和l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，说明约束条件对N_cr有较大影响。

图 4 冲击端为夹支时不同径厚比下临界荷载与临界长度的关系

Figure 4. Critical load vs.critical length under clamped edge and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

图 5 冲击端为简支时不同径厚比下临界荷载与临界长度的关系

Figure 5. Critical load vs.critical length under simple support and different diameter-thickness ratios conditions

下载: 全尺寸图片幻灯片

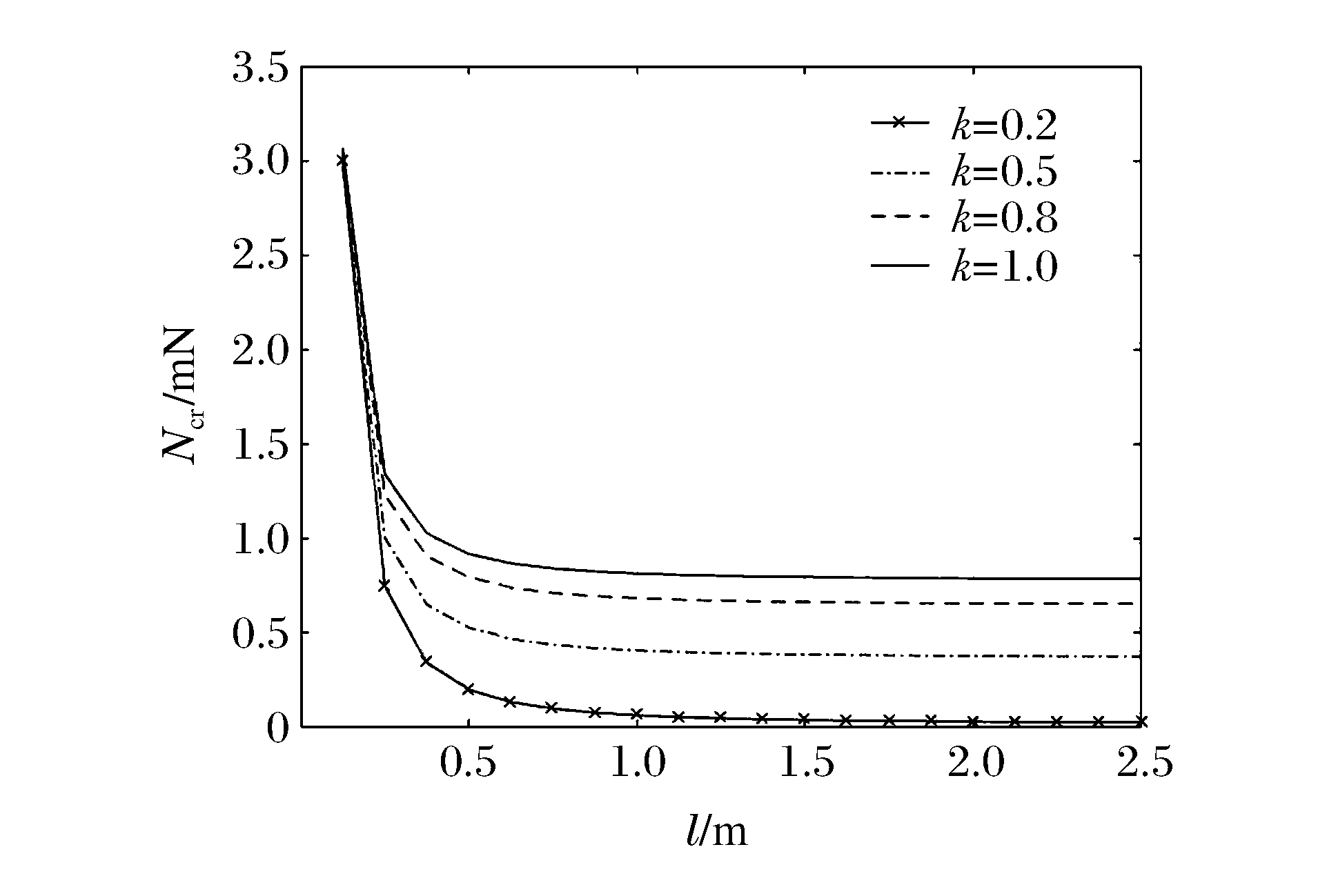
图 6和图 7分别表示冲击端为夹支和简支条件下n=1、m=2、R/h=20时不同k下N_cr与l的关系曲线。可见：FGM圆柱壳的N_cr随着l的增加而减小；在同一l下，FGM圆柱壳的N_cr随着k的增加而增加；当l＜0.5 m时，N_cr随l的增加迅速减小，当l＞0.5 m时，N_cr随l的增加缓慢减小并逐渐趋于常数；当k=1且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，再次说明约束条件对N_cr的影响较大。

图 6 冲击端为夹支时不同梯度指数下临界荷载与临界长度的关系

Figure 6. Critical load vs.critical length under clamped edge and different gradient indexes conditions

下载: 全尺寸图片幻灯片

图 7 冲击端为简支时不同梯度指数下临界荷载与临界长度的关系

Figure 7. Critical load vs.critical length under simple support and different gradient indexes conditions

下载: 全尺寸图片幻灯片

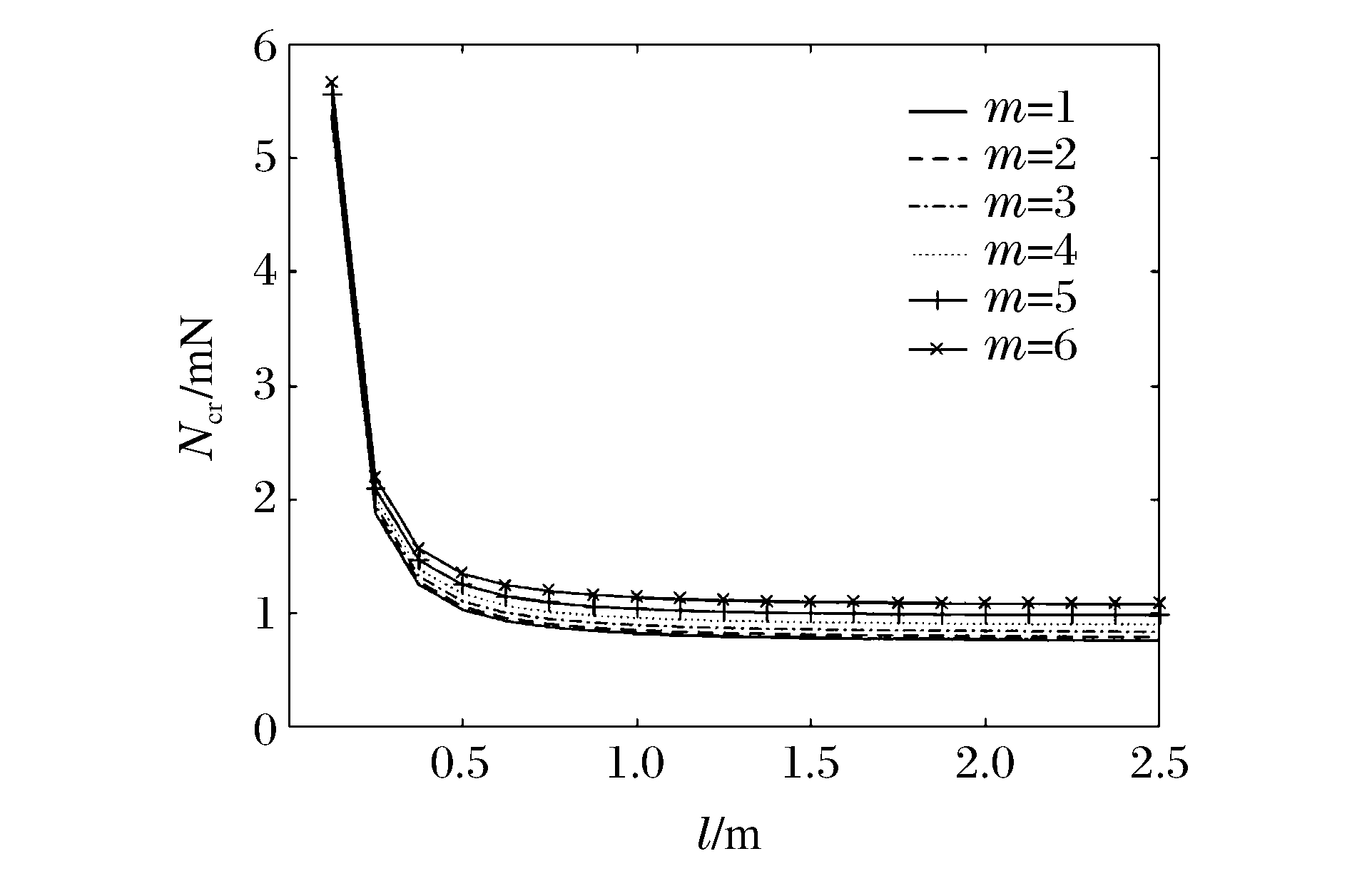
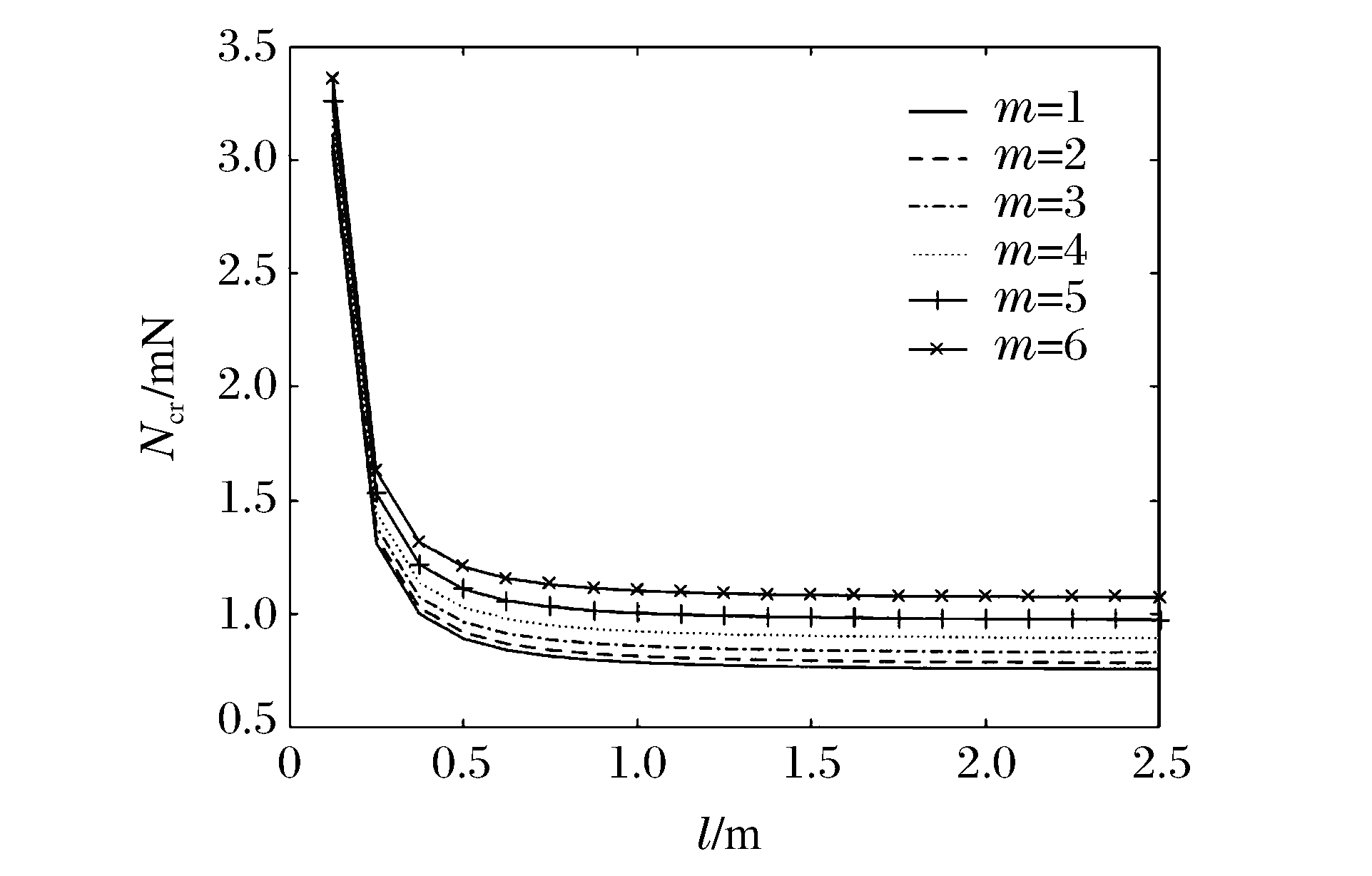
图 8和图 9分别表示冲击端为夹支和简支，n=1、k=1、R/h=20时不同m下N_cr与l的关系曲线。可以看出：当l在一定范围内时，N_cr随l的增加而迅速减小，超出这一范围后N_cr随l的增加而缓慢减小且逐渐趋于常数；同一l下，随着m的增大，FGM圆柱壳的N_cr增大，表明N_cr越大，高阶模态越易被激发。当m=6且l一定时，冲击端为夹支时的N_cr明显比冲击端为简支时的N_cr大，表明约束条件对N_cr有较大影响。

图 8 冲击端为夹支时不同环向模态数下临界荷载与临界长度的关系

Figure 8. Critical load vs.critical length under clamped edge and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

图 9 冲击端为简支时不同环向模态数下临界荷载与临界长度的关系

Figure 9. Critical load vs.critical length under simple support and different circumferential modal number conditions

下载: 全尺寸图片幻灯片

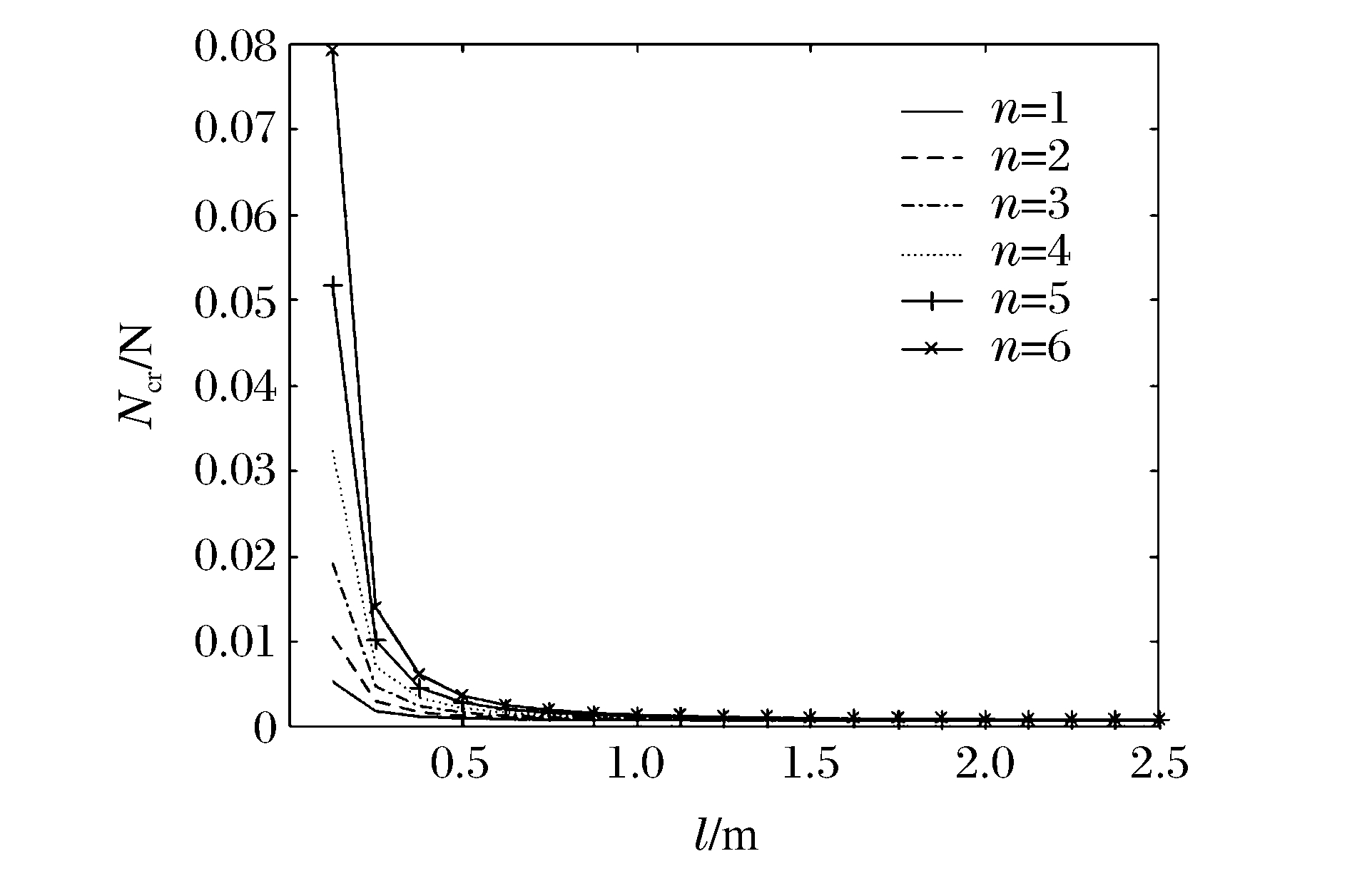
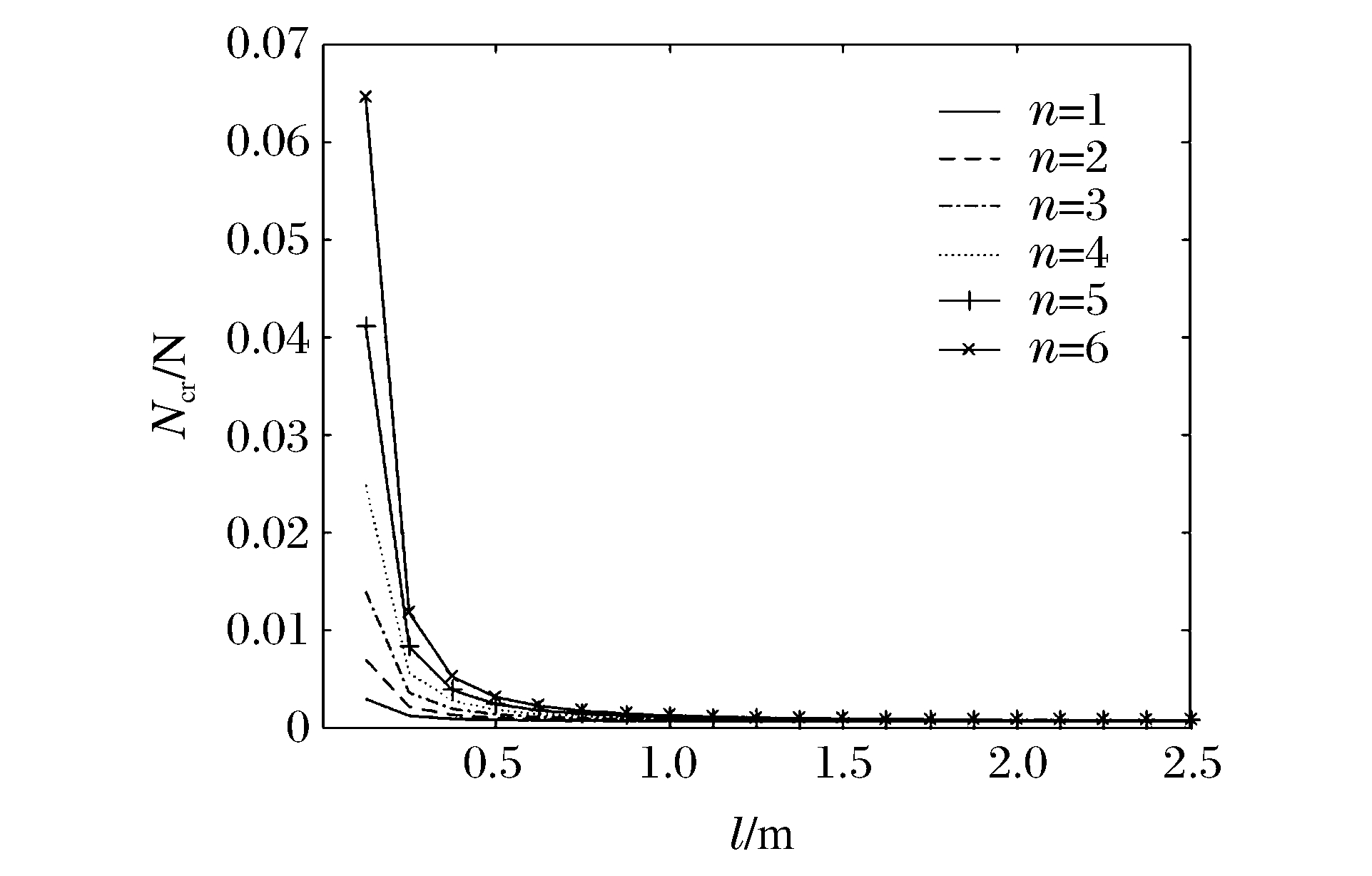
图 10和图 11分别表示冲击端为夹支和简支，m=1、k=1、R/h=20时不同n下N_cr与l的关系曲线。图 10和图 11显示：N_cr随着l的增加而减小；不同n条件下，N_cr随l的增加逐渐趋于同一值；当l＜1 m时，在同一l下N_cr随n的增加而增加，说明N_cr越大，高阶模态越容易被激发。

图 10 冲击端为夹支时不同轴向模态数下临界荷载与临界长度的关系

Figure 10. Critical load vs.critical length under clamped edge and different axial modal number conditions

下载: 全尺寸图片幻灯片

图 11 冲击端为简支时不同轴向模态数下临界荷载与临界长度的关系

Figure 11. Critical load vs.critical length under simple support and different axial modal number conditions

下载: 全尺寸图片幻灯片

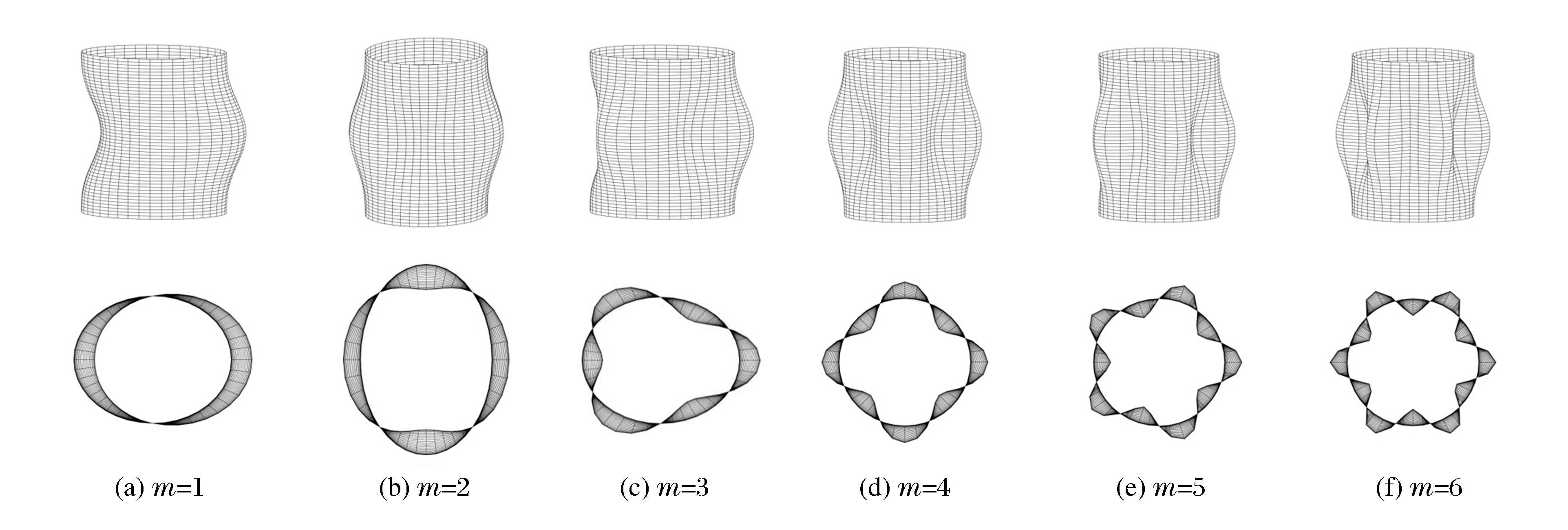
图 12为不同环向模态数m下FGM圆柱壳的动力屈曲模态。可以看出：随着m的增大，圆柱壳的模态变得越来越复杂，俯视图由单一形变为多瓣形；当m=6时，俯视图为12瓣形。

图 12 不同环向屈曲模态图(n=1;m=1, 2, 3, 4, 5, 6)

Figure 12. Different circumferential buckling modes (n=1;m=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

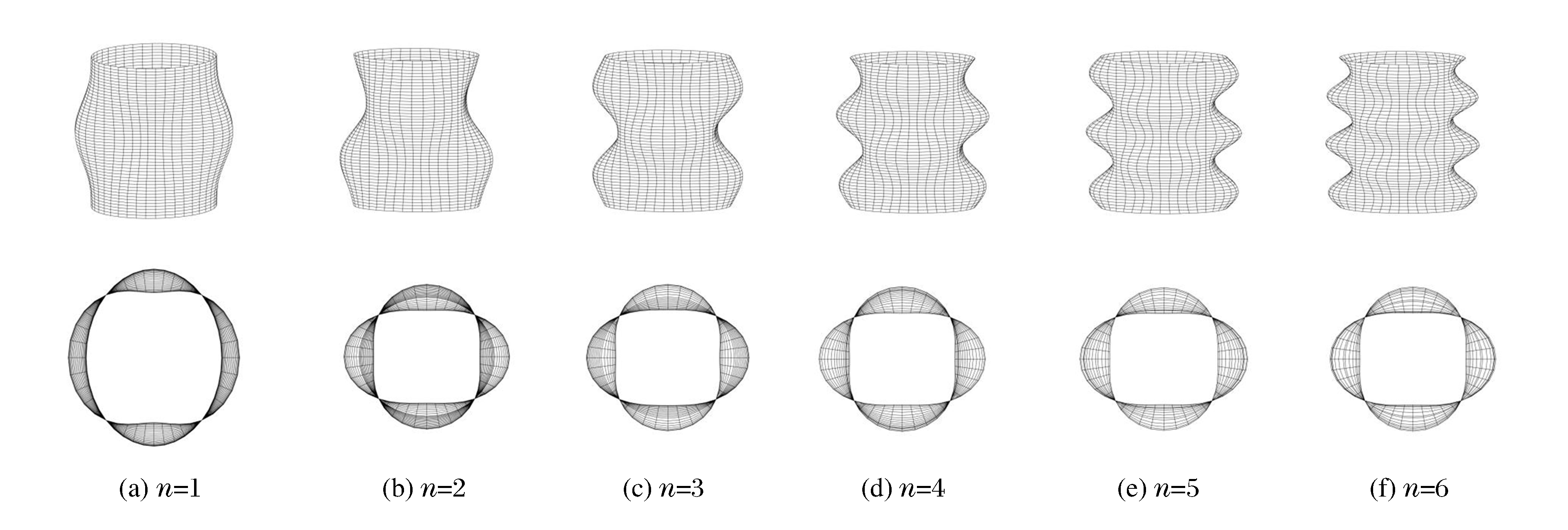
图 13为不同轴向模态数n下FGM圆柱壳的动力屈曲模态图。由图 13可知：随着n的增加，模态图变得越来越复杂。由FGM圆柱壳的俯视图可知，各阶模态数下动力屈曲模态图为轴对称。

图 13 不同轴向屈曲模态图(m=2;n=1, 2, 3, 4, 5, 6)

Figure 13. Different axial buckling modes (m=2;n=1, 2, 3, 4, 5, 6)

下载: 全尺寸图片幻灯片

4. 结论

(1) 根据Donnell壳体理论和经典板壳理论，由Hamilton变分原理得到轴向荷载作用下FGM圆柱壳的动力屈曲控制方程。

(2) 由圆柱壳周向连续性设出径向位移的周向形式，并用分离变量法得到不同约束条件下FGM圆柱壳动力屈曲临界荷载的表达式和屈曲解式。

(3) 利用MATLAB对临界荷载进行计算，得到：在轴向模态数(n)、环向模态数(m)、梯度指数(k)、径厚比(R/h)一定的情况下，同种材料组成的圆柱壳的临界荷载随着临界长度的增加而减小；在n、m、k、l一定的情况下，临界荷载随着径厚比的增大而减小；在n、m、R/h、l一定的情况下，临界荷载随着梯度指数k的增加而增加；不同约束条件下，冲击端为夹支的临界荷载大于冲击端为简支的临界荷载，表明约束条件对临界荷载有较大影响；圆柱壳的临界荷载随模态数的增加而增大，表明临界荷载越大，越容易激发高阶模态；圆柱壳的动力屈曲模态随模态数的增加变得更为复杂。

图 1 果胶甲基脂酶作用原理^[5]

Figure 1. Reaction of pectincatalyzed by pectin methylesterase^[5]

下载: 全尺寸图片幻灯片

图 2 HPCD处理装置

Figure 2. High pressure carbon dioxide processing equipment

1.CO₂ cylinder; 2.CO₂ filter; 3.Pressure gauge; 4.Cooling unit; 5.Plunger pump; 6.Pressure transducer; 7.Biohazard clean safety facility for aseptic operation; 8.High pressure vessel; 9.Thermocouples; 10.Thermostatic bath; 11.Vacuum pump; 12.Displaying panel

下载: 全尺寸图片幻灯片

图 3 不同压强下HPCD处理(55 ℃)桃PME的钝化动力学

Figure 3. Inactivation kinetics of peach PME by HPCD at 55 ℃ modelled using the first-order kinetic model

下载: 全尺寸图片幻灯片

图 4 HPCD(55 ℃)钝化桃PME的Z_p和V_a值理

Figure 4. Z_p and V_a for pressure-dependence inactivation of peach PME by HPCD at 55 ℃

下载: 全尺寸图片幻灯片

图 5 不同温度下HPCD处理(15 MPa)桃PME的钝化动力学

Figure 5. Inactivation kinetics of peach PME by HPCD at 15 MPa modelled using the first-order kinetic model

下载: 全尺寸图片幻灯片

图 6 HPCD处理(15 MPa)钝化桃PME的Z_T和E_a值

Figure 6. Z_T and E_a for temparature-dependence inactivation of peach PME by HPCD at 15 MPa

下载: 全尺寸图片幻灯片

图 7 HPCD处理(55 ℃，15 MPa)对桃汁和PME粗酶液的钝化效果(重复次数:n=3；不同字母a, b, c, d, e表示有显著性差异)

Figure 7. Comparison of the A_R of peach PME in juice and buffer by HPCD (55 ℃, 15 MPa) (Times of repetition:n=3;Different letters represent that there are significant diffrences)

下载: 全尺寸图片幻灯片

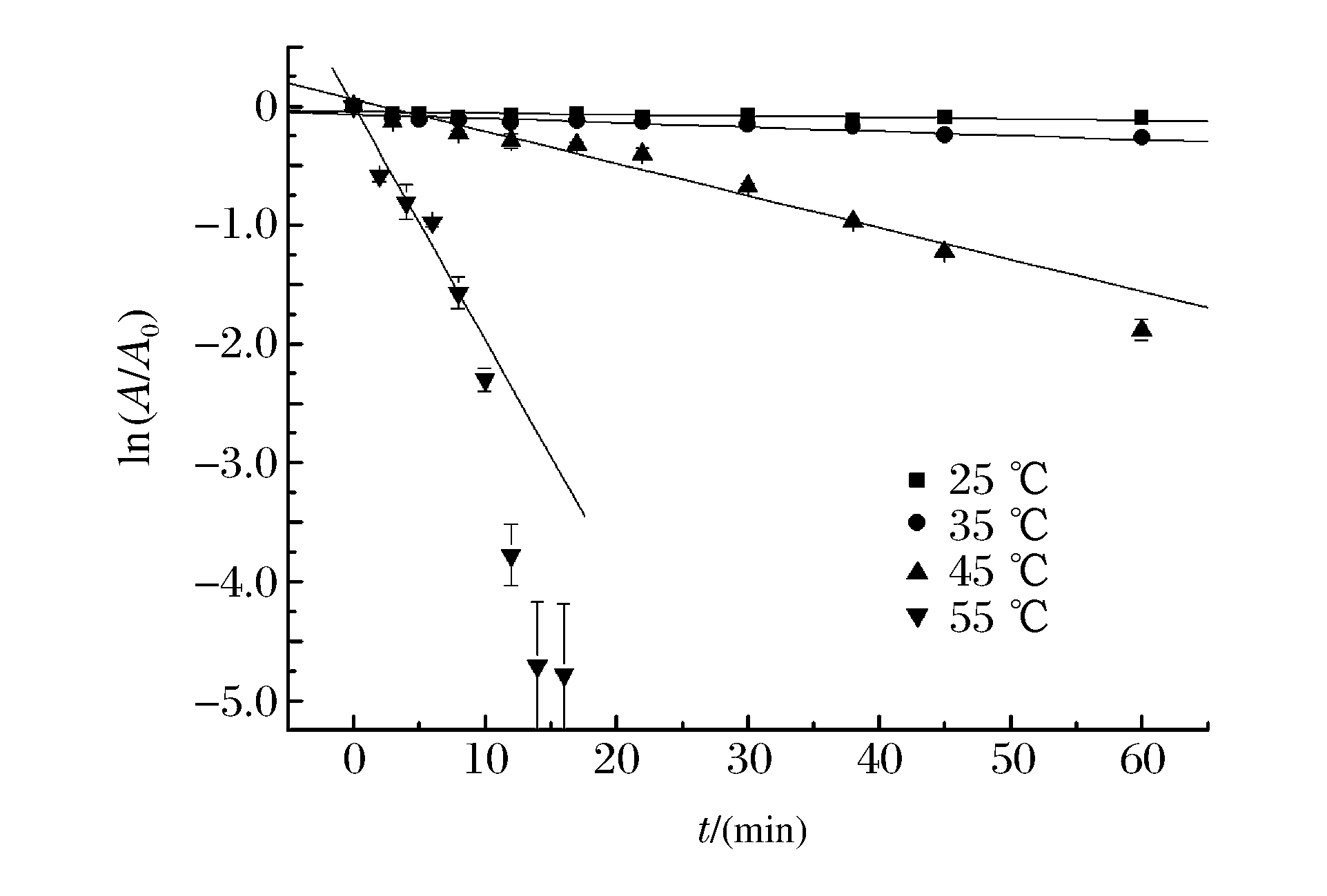
表 1 不同压强HPCD钝化桃PME的动力学参数(55 ℃)

Table 1. Estimation of inactivation kinetic parameters of peach PME by HPCD at 55 ℃ using the first-order kinetic model

Pressure/(MPa)	Kinetic parameters
Pressure/(MPa)	k/(min^-1)	D/(min)	R²(P < 0.05)
5	0.036 5±0.000 8	63.08	0.76
8	0.066 4±0.001 5	34.68	0.79
12	0.168 3±0.004 0	13.68	0.94
15	0.196 5±0.022 2	11.72	0.91
22	0.408 8±0.044 1	5.63	0.86
Note：R² represents the linear regression coefficient, P represents the significance level.

下载: 导出CSV

表 2 不同温度HPCD钝化桃PME的动力学参数(15 MPa)

Table 2. Estimation of inactivation kinetic parameters of peach PME by HPCD at 15 MPa using the first-order kinetic model

Temperature/(℃)	Kinetic parameters
Temperature/(℃)	k/(min^-1)	D/(min)	R²(P < 0.05)
25	0.001 2±0.000 5	1 918.82	0.360
35	0.003 6±0.000 8	639.61	0.667
45	0.027 0±0.001 5	85.28	0.970
55	0.196 5±0.022 2	11.72	0.910
Note：R² represents the linear regression coefficient, P represents the significance level.

下载: 导出CSV

参考文献(31)

[1]	周林燕, 廖红梅, 张文佳, 等.食品高压技术研究进展和应用现状[J].中国食品学报, 2009, 9(4): 165-169. http://d.wanfangdata.com.cn/Periodical_zgspxb200904027.aspx Zhou L Y, Liao H M, Zhang W J, et al. The research progress and application status of high pressure technology in food science[J]. Journal of Chinese Institute of Food Science and Technology, 2009, 9(4): 165-169. (in Chinese) http://d.wanfangdata.com.cn/Periodical_zgspxb200904027.aspx
[2]	Knorr D. Novel approaches in food-processing technology: New technologies for preserving foods and modifying function[J]. Curr Opin Biotech, 1992, 10(5): 485-491. http://www.sciencedirect.com/science/article/pii/S0958166999000154
[3]	Lado B H, Yousef A E. Alternative food-preservation technologies: Efficacy and mechanisms[J]. Microbes Infect, 2002, 4(4): 433-440. doi: 10.1016/S1286-4579(02)01557-5
[4]	Damar D, Blaban M O. Review of dense phase CO₂ technology: Microbiol and enzyme inactivation, and effects on food quality[J]. J Food Sci, 2006, 71(1): 1-11.
[5]	Francis K E, Lam S Y, Copenhaver G P. Separation of arabidopsis pollen tetrads is regulated by QUARTET1, a pectin methylesterase gene[J]. Plant Physiol, 2006, 142(3): 1004-1013. doi: 10.1104/pp.106.085274
[6]	Ly-Nguyen B, van Loey A M, Smout C, et al. Effect of mild-heat and high-pressure processing on banana pectin methylesterase: A kinetic study[J]. J Agric Food Chem, 2003, 51(27): 7974-7979. doi: 10.1021/jf034658i
[7]	Guiavarc'h Y, Segovia O, Hendrickx M, et al. Purification, characterization, thermal and high-pressure inactivation of a pectin methylesterase from white grapefruit(Citrus paradisi)[J]. Innov Food Sci Emerg, 2005, 6(4): 363-371. doi: 10.1016/j.ifset.2005.06.003
[8]	Duvetter T, Sila D N, van Buggenhout S V, et al. Pectins in processed fruit and vegetables: Part I-stability and catalytic activity of pectinases[J]. Compr Rev Food Sci Food Saf, 2009, 8(2): 75-85. doi: 10.1111/j.1541-4337.2009.00070.x
[9]	Zhi X, Zhang Y, Hu X, et al. Inactivation of apple pectin methylesterase induced by dense phase carbon dioxide[J]. J Agric Food Chem, 2008, 56(13): 5394-5400. doi: 10.1021/jf800260c
[10]	Niu S, Xu Z, Fang Y, et al. Comparative study on cloudy apple juice qualities from apple slices treated by high pressure carbon dioxide and mild heat[J]. Innov Food Sci Emerg, 2010, 11(1): 91-97. http://www.cabdirect.org/abstracts/20103061936.html
[11]	Zhou L, Zhang Y, Hu X, et al. Comparison of the inactivation kinetics of pectin methylesterases from carrot and peach by high-pressure carbon dioxide[J]. Food Chem, 2009, 115(2): 449-455. doi: 10.1016/j.foodchem.2008.12.028
[12]	Liu X, Gao Y X, Peng X T, et al. Inactivation of peroxidase and polyphenol oxidase in red beet(Beta vulgaris L.)extract with high pressure carbon dioxide[J]. Innov Food Sci Emerg, 2008, 9(1): 24-31. doi: 10.1016/j.ifset.2007.04.010
[13]	Zhou L Y, Zhang Y, Leng X J, et al. Acceleration of precipitation formation in peach juice induced by high-pressure carbon dioxide[J]. J Agric Food Chem, 2010, 58(17): 9605-9610. doi: 10.1021/jf101430j
[14]	Sampedro F, Rodrigo D, Hendrickx M. Inactivation kinetics of pectin methyl esterase under combined thermal-high pressure treatment in an orange juice-milk beverage[J]. J Food Eng, 2008, 86(1): 133-139. doi: 10.1016/j.jfoodeng.2007.09.019
[15]	Eagerman B A, Rouse A H. Heat inactivation temperature-time relationships for pectinesterase inactivation in citrus juices[J]. J Food Sci, 1976, 41(6): 1396-1397. doi: 10.1111/j.1365-2621.1976.tb01180.x
[16]	Balaban M O, Arreola A G, Marshall M, et al. Inactivation of pectinesterase in orange juice by supercritical carbon dioxide[J]. Journal Food Sci, 1991, 56(3): 743-746. doi: 10.1111/j.1365-2621.1991.tb05372.x
[17]	Weemaes C, Ludikhuyze L, van den Broeck I, et al. High pressure inactivation of polyphenoloxidases[J]. J Food Sci, 1998, 63(5): 873-877. doi: 10.1111/j.1365-2621.1998.tb17917.x
[18]	Javeri H, Wicker L. Partial purification and characterization of peach pectinesterase[J]. J Food Biochem, 1991, 15(4): 241-252. doi: 10.1111/j.1745-4514.1991.tb00159.x
[19]	Ly-Nguyen B, van Loey A M, Fachin D, et al. Partial purification, characterization, and thermal and high-pressure inactivation of pectin methylesterase from carrots(Daucu carrota L.)[J]. J Agric Food Chem, 2002, 50(19): 5437-5444. doi: 10.1021/jf011666v
[20]	Ly-Nguyen B, van Loey A M, Fachin D, et al. Purification, characterization, thermal, and high-pressure inactivation of pectin methylesterase from bananas(cv Cavendish)[J]. Biotechnol Bioeng, 2002, 78(6): 683-691. http://www.ncbi.nlm.nih.gov/pubmed/11992533
[21]	Alonso J, Rodrguez M T, Canet W. Purification and characterization of four pectinesterases from sweet cherry(Prunus avium L.)[J]. J Agric Food Chem, 1996, 44(11): 3416-3422. doi: 10.1021/jf960204s
[22]	Alonso J, Howell N, Canet W. Purification and characterization of two pectinmethylesterase from persimmon(Diospyros kaki)[J]. J Sci Food Agric, 1997, 75(3): 352-358. doi: 10.1002/(SICI)1097-0010(199711)75:3<352::AID-JSFA885>3.0.CO;2-G
[23]	Nunes C S, Castro S M, Saraiva J A, et al. Thermal and high-pressure stability of purified pectin methylesterase from plums(Prunus Domestica)[J]. J Food Biochem, 2006, 30(2): 138-154. doi: 10.1111/j.1745-4514.2006.00057.x
[24]	Viar-Vera M A, Salazar-Montoya J A, Calva-Calva G, et al. Extraction, thermal stability and kinetic behavior of pectinmethylesterase from hawthorn(Crataegus pubescens)fruit[J]. LWT-Food Sci Technol, 2007, 40(2): 278-284. doi: 10.1016/j.lwt.2005.10.005
[25]	Zhang Y, Wang Y Y, Zhou L Y, et al. A comparative study of inactivation of peach polyphenol oxidase and carrot polyphenol oxidase induced by high-pressure carbon dioxide[J]. Int J Food Sci Tech, 2010, 45(11): 2297-2305. doi: 10.1111/j.1365-2621.2010.02403.x
[26]	刘野, 张超, 许勇, 等.高压二氧化碳对西瓜汁中过氧化物酶钝化动力学的研究[J].食品工业科技, 2011, 34(11): 160-163. http://www.cqvip.com/QK/92916X/20117/38429900.html Liu Y, Zhang C, Xu Y, et al. Research of the inactivation kinetics of peroxidase in watermelon juice by high pressure carbon dioxide[J]. Science and Technology of Food Industry, 2011, 34(11): 160-163. (in Chinese) http://www.cqvip.com/QK/92916X/20117/38429900.html
[27]	Katsaros G, Apseridis I, Taoukis P. Modelling of high hydrostatic pressure inactivation of pectinmethylesterase from persimmon fruit[C]//IUFOST 13th World Congress of Food Science & Technology. Nantes, France, 2006.
[28]	Balogh T, Smout C, Ly-Nguyen B, et al. Thermal and high-pressure inaavtivation kinetics of carrot pectinmethylesterase: From model system to real foods[J]. Innov Food Sci Emerg, 2004, 5(4): 429-436. doi: 10.1016/j.ifset.2004.06.002
[29]	Gui F, Chen F, Wu J, et al. Inactivation and structural change of horseradish peroxidase treated with supercritical carbon dioxide[J]. Food Chem, 2006, 97(3): 480-489. doi: 10.1016/j.foodchem.2005.05.028
[30]	Gui F, Wu J, Chen F, et al. Inactivation of polyphenol oxidases in cloudy apple juice exposed to supercritical carbon dioxide[J]. Food Chem, 2007, 100(4): 1678-1685. doi: 10.1016/j.foodchem.2005.12.048
[31]	Liao X J, Zhang Y, Bei J, et al. Alterations of molecular properties of lipoxygenase induced by Dense Phase Carbon Dioxide[J]. Innov Food Sci Emerg, 2009, 10(1): 47-53. doi: 10.1016/j.ifset.2008.06.007