一种适用宽速域冲击的明胶鸟弹数值建模方法

彭鸿博; 侯润峰; 李旭阳; 王计真; 白春玉; 石霄鹏

doi:10.11858/gywlxb.20240726

一种适用宽速域冲击的明胶鸟弹数值建模方法

doi: 10.11858/gywlxb.20240726

1.
中国民航大学航空工程学院, 天津　300300
2.
中国民航大学中欧航空工程师学院, 天津　300300
3.
中国飞机强度研究所, 陕西西安　710000
4.
中国民航大学科技创新研究院, 天津　300300

基金项目: 中央高校基本科研业务费专项资金（31220200069）

详细信息

作者简介:
彭鸿博（1972－），男，硕士，副教授，主要从事航空发动机数值分析、发动机维修及可靠性研究.E-mail：hbpeng@cauc.edu.cn

通讯作者:
石霄鹏（1987－），男，博士，讲师，主要从事民机结构冲击防护与民机客舱安全研究.E-mail：xpshi@cauc.edu.cn

中图分类号: O347; O521.9
计量
- 文章访问数: 34
- HTML全文浏览量: 13
- PDF下载量: 7
出版历程
- 收稿日期: 2024-02-06
- 修回日期: 2024-03-29
- 网络出版日期: 2024-07-15
- 刊出日期: 2024-09-29

A Numerical Modeling Method of Gelatin Bird Projectile Suitable for Wide-Speed-Range Impact

1.
College of Aeronautical Engineering, Civil Aviation University of China, Tianjin 300300, China
2.
Sino-European Institute of Aviation Engineering, Civil Aviation University of China, Tianjin 300300, China
3.
Aircraft Strength Research Institute of China, Xi’an 710000, Shaanxi, China
4.
Science and Technology Innovation Research Institute, Civil Aviation University of China, Tianjin, 300300, China

摘要

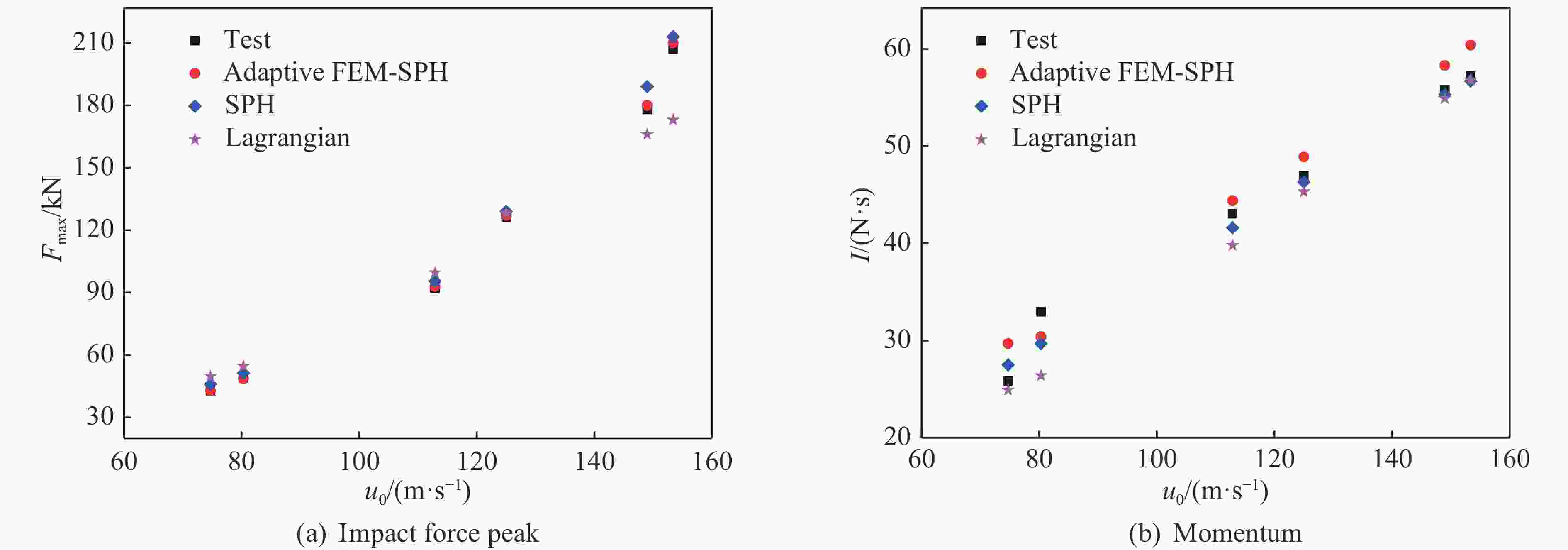
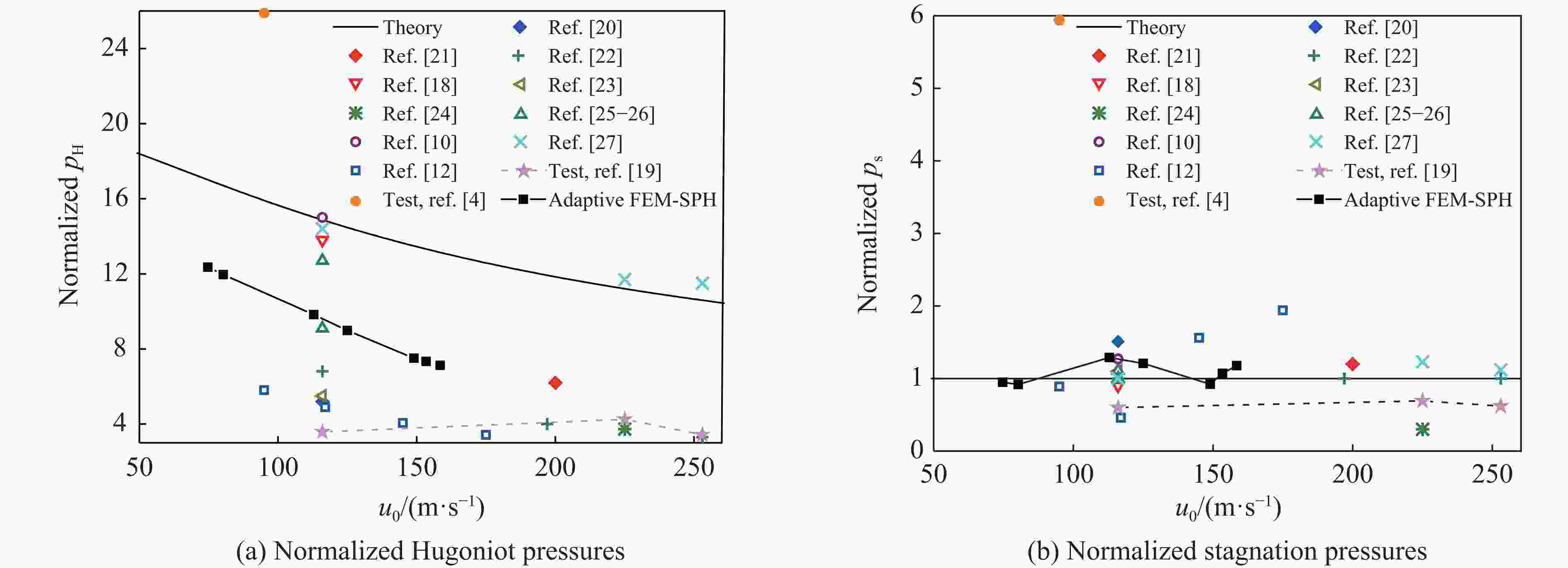
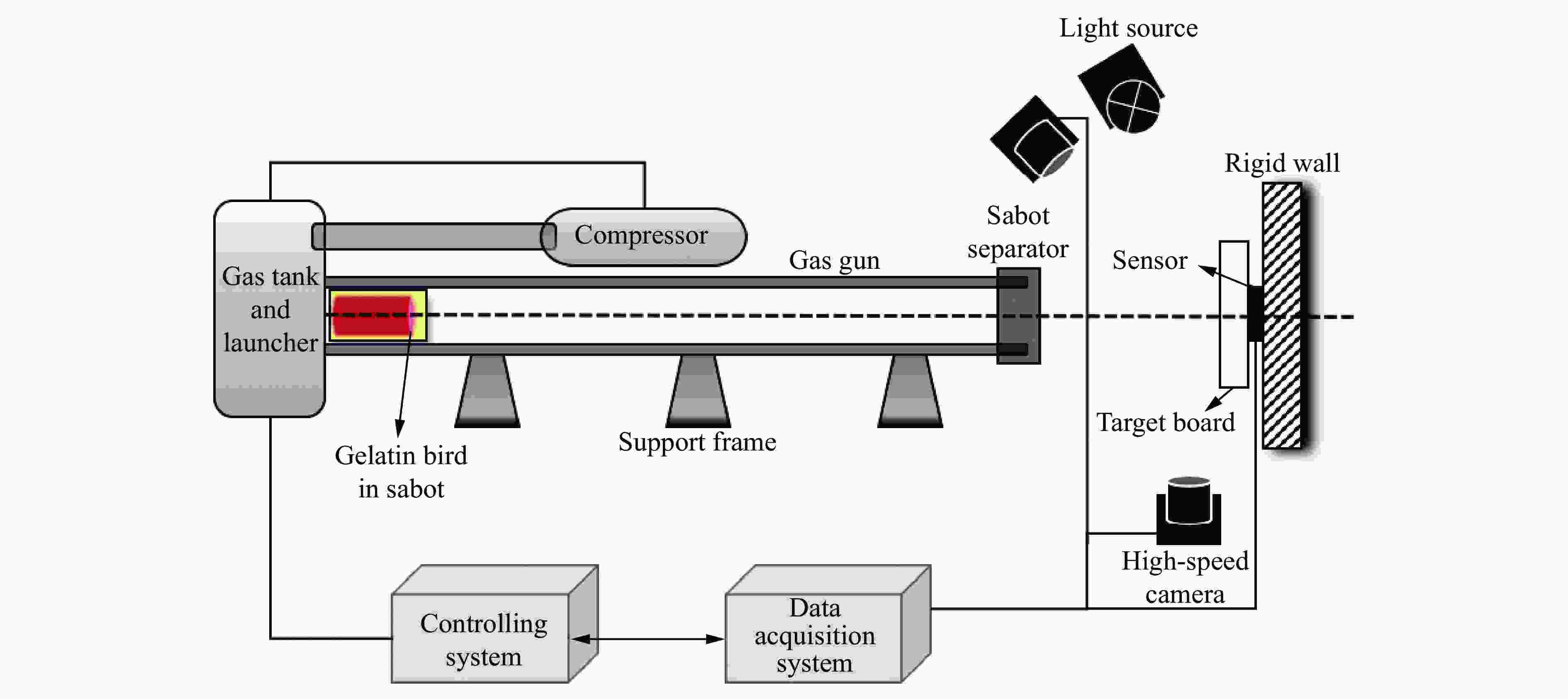
摘要: 明胶鸟弹在不同撞击速度下表现出不同的响应特性。为解决传统明胶鸟弹本构表征方法在不同速度范围内不能通用的问题，开展了330 g明胶鸟弹以70～190 m/s速度、60°或90°入射刚性铝合金平板试验，记录了冲击力数据及撞击形貌。结果表明，随着撞击速度的提高，鸟弹碎裂得更充分，碎块体积减小。利用LS-DYNA建立了自适应FEM-SPH（finite element method-smoothed particle hydrodynamics）鸟体模型。依据试验结果反演得到一组鸟体本构参数：切线模量为1.33 MPa，剪切模量为115.95 MPa，Murnaghan状态方程参数γ为10.49，k₀为69.77 MPa，体积模量为246.4 MPa，失效塑性应变为1.15，初始屈服应力为0.21 MPa。仿真结果与试验结果具有很好的一致性，冲击力峰值的相对误差在2%以内，冲量的相对误差在10%以内。自适应FEM-SPH鸟体模型具有比SPH模型和拉格朗日模型更高的精度。由自适应模型得到的Hugoniot压强与理论结果具有相同的变化趋势，滞止压强与理论值较接近。
- 鸟撞 /
- 明胶鸟弹 /
- 自适应 /
- 有限元法 /
- 光滑粒子流体动力学 /
- 本构参数识别
Abstract: Previous studies revealed that gelatin birds show different mechanical behaviors at different impact velocities. In order to solve the problem that the traditional constitutive methods of gelatin bird cannot be universal in different velocity ranges, the tests of 330 g gelatin birds impacting rigid aluminum alloy plate at 60° and 90° incident angles, covering a velocity range of 70−190 m/s were carried out to record the impact force data and impact morphology. With the increase of velocity, the birds were broken more fully and smaller fragments were observed. The adaptive FEM-SPH (finite element method-smoothed particle hydrodynamics) model of bird was established in LS-DYNA, and a set of constitutive parameters were inverted according to the test results: tangent modulus equals to 1.33 MPa, shear modulus equals to 115.95 MPa, the parameters of Murnaghan equation of state γ equals to 10.49, k₀ equals to 69.77 MPa, bulk modulus equals to 246.4 MPa, failure plastic strain is 1.15, yield stress is 0.21 MPa. The simulation results were in good agreement with the test results, and had higher accuracy compared to the SPH models and the Lagrangian models. The Hugoniot pressure of the adaptive model had the same change trend as the theoretical value, and the stagnation pressure was close to the theoretical value.
- bird impact /
- gelatin bird /
- adaptive /
- finite element method /
- smoothed particle hydrodynamics /
- constitutive parameters identification

HTML全文

图 1 鸟撞试验装置示意图

Figure 1. Schematic diagram of bird impact test apparatus

下载: 全尺寸图片幻灯片

图 2 靶板的外形及尺寸

Figure 2. Shape and size of target plate

下载: 全尺寸图片幻灯片

图 3 90°撞击工况（工况12）的冲击力对比

Figure 3. Comparison of the impact forces in Case 12 with the impact angle of 90°

下载: 全尺寸图片幻灯片

图 4 60°撞击工况（工况1）的冲击力对比

Figure 4. Comparison of the impact forces in Case 1 with the impact angle of 60°

下载: 全尺寸图片幻灯片

图 5 鸟弹1^#的高速摄影照片

Figure 5. High-speed camera photos of projectile 1^#

下载: 全尺寸图片幻灯片

图 6 鸟弹18^#的高速摄像照片

Figure 6. High-speed camera photos of projectile 18^#

下载: 全尺寸图片幻灯片

图 7 不同冲击速度下的高速摄像图像对比（θ=90°）

Figure 7. Comparison of high-speed camera photos at different impact velocities (θ=90°)

下载: 全尺寸图片幻灯片

图 8 不同工况下试验与数值模拟得到的冲击力对比

Figure 8. Comparison of the test and numerical impact forces in different cases

下载: 全尺寸图片幻灯片

图 9 90°工况下数值模拟得到的峰值载荷及冲量与试验结果的对比

Figure 9. Comparison of the numerical and test impact force peak and momentum under 90° impact

下载: 全尺寸图片幻灯片

图 10 60°工况下数值模拟得到的峰值载荷及冲量与试验结果的对比

Figure 10. Comparison of the numerical and test impact force peak and momentum under 60° impact

下载: 全尺寸图片幻灯片

图 11 74.7 m/s撞击速度下数值模拟和试验得到的撞击过程对比

Figure 11. Comparison of the numerical and test impact processes at the impact velocity of 74.7 m/s

下载: 全尺寸图片幻灯片

图 12 112.9 m/s撞击速度下数值模拟与试验得到的撞击过程对比

Figure 12. Comparison of the numerical and test impact processes at the impact velocity of 112.9 m/s

下载: 全尺寸图片幻灯片

图 13 158.5 m/s撞击速度下数值模拟与试验得到的撞击过程对比

Figure 13. Comparison of the numerical and test impact processes at the impact velocity of 158.5 m/s

下载: 全尺寸图片幻灯片

图 14 具有不同孔隙率的圆柱明胶鸟弹的理论Hugoniot压强随撞击速度的变化

Figure 14. Hugoniot pressure vs. impact velocity for cylindrical gelatin bird projectiles with various porosities

下载: 全尺寸图片幻灯片

图 15 归一化Hugoniot压强和归一化滞止压强的数值计算结果与试验结果及理论值的对比

Figure 15. Comparison of normalized Hugoniot pressures and normalized stagnation pressures between the theoretical values, test values and numerical results

下载: 全尺寸图片幻灯片

表 1 不同工况下鸟撞试验结果

Table 1. Test results of bird impact under various impact conditions

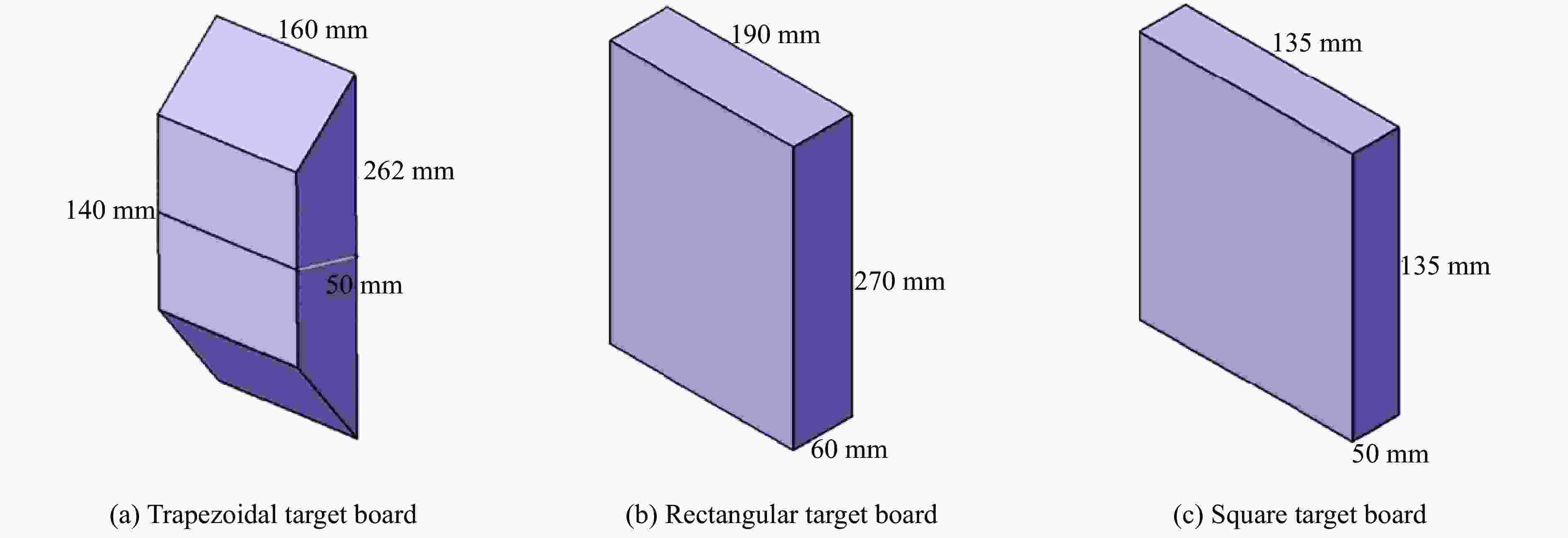
Case	u₀/(m·s⁻¹)	θ/(°)	Plate shape	Bird projectile No. (m, actual impact velocity)
1	110	60	Rectangular	18^# (334 g, 110.1 m/s), 20^# (329.5 g, 107.0 m/s)
2	110	60	Rectangular	29^# (326 g, 114.7 m/s)
3	120	60	Rectangular	21^# (327 g, 124.6 m/s), 22^# (328 g, 124.1 m/s)
4	150	60	Rectangular	23^# (331 g, 150.1 m/s), 24^# (330 g, 153.7 m/s)
5	160	60	Rectangular	25^# (331 g, 166.6 m/s)
6	170	60	Rectangular	26^# (328 g, 175.9 m/s)
7	180	60	Rectangular	28^# (331 g, 185.3 m/s)
8	190	60	Rectangular	27^# (332 g, 191.8 m/s)
9	70	60	Square	39^# (328 g, 75.2 m/s), 40^# (328 g, 73.6 m/s)
10	90	60	Square	37^# (328 g, 94.6 m/s)
11	100	60	Square	38^# (327 g, 99.6 m/s)
12	110	90	Trapezoidal	1^# (336 g, 112.9 m/s), 2^# (328 g, 113.1 m/s)
13	120	90	Trapezoidal	5^# (333 g, 125.0 m/s), 12^# (334 g, 121.8 m/s)
14	150	90	Trapezoidal	6^# (332 g, 149.0 m/s), 7^# (328 g, 148.8 m/s)
15	150	90	Trapezoidal	8^# (332 g, 153.4 m/s), 9^# (333 g, 148.8 m/s)
16	160	90	Trapezoidal	10^# (325 g, 165.3 m/s), 11^# (329 g, 158.5 m/s)
17	80	90	Square	34^# (330 g, 84.9 m/s), 35^# (329 g, 80.3 m/s)
18	70	90	Square	36^# (330.5 g, 74.7 m/s)

下载: 导出CSV

表 2 铝合金的材料参数

Table 2. Material parameters of aluminum alloy

Density/(kg·m⁻³)	Young’s modulus/GPa	Poisson’s ratio	Yield stress/MPa	Tangent modulus/GPa
2796	71	0.33	450	5

下载: 导出CSV

表 3 SPH鸟体材料参数

Table 3. Material parameters of SPH bird model

Density/(kg·m⁻³)	Cut-off pressure/MPa	Dynamic viscosity/(Pa·s)
950	−1	0.001

下载: 导出CSV

表 4 自适应FEM-SPH模型的本构参数优化结果

Table 4. Optimization results of constitutive parameters for adaptive FEM-SPH model

Value	E_t/MPa	G/MPa	γ	K/MPa	k₀/MPa	ε_f	σ_s/MPa
Range	0.01−2.00	1−300	1−20	1−300	1−300	0.01−1.20	0.01−1.00
Optimal value	1.33	115.95	10.49	246.4	69.77	1.15	0.21

下载: 导出CSV

表 5 不同学者通过数值模拟得到的归一化Hugoniot压强和滞止压强^{[10, 12, 18, 20–27]}

Table 5. Numerical results of normalized Hugoniot and stagnation pressures calculated by different researchers^{[10, 12, 18, 20–27]}

Ref.	u₀/(m·s⁻¹)	Normalized p_H	Normalized p_s	m/kg	Density/(kg·m⁻³)	Bird model geometry
[20]	116	5.2	1.5	1.8	938	Hemispherical-ended
[21]	200	6.2	1.2	0.6	930	Cylinder
[22]	116	6.8	1.0	1.8	934	Hemispherical-ended
[22]	197	4.0	1.0	1.8	934	Hemispherical-ended
[22]	253	3.3	1.0	1.8	934	Hemispherical-ended
[18]	116	13.8	0.9	1.0	950	Hemispherical-ended
[23]	116	5.5	1.1	1.8	950	Hemispherical-ended
[24]	225	3.7	0.3	1.8	934	Cylinder
[25]	116	12.7	1.1	1.8	938	Hemispherical-ended
[26]	116	9.1	1.0	1.7	950	Hemispherical-ended
[10]	116	15.0	1.3	0.2	1019	Cylinder
[27]	116	14.4	1.0	0.8	938	Hemispherical-ended
[27]	225	11.7	1.2	0.8	938	Hemispherical-ended
[27]	253	11.5	1.1	0.8	938	Hemispherical-ended
[12]	95	5.8	0.9	1.3	968	Cylinder
[12]	117	4.9	0.5	1.3	968	Cylinder
[12]	145	4.1	1.6	1.3	968	Cylinder
[12]	175	3.4	1.9	1.3	968	Cylinder

下载: 导出CSV

参考文献(27)

[1]	GUIDA M, MARULO F, BELKHELFA F Z, et al. A review of the bird impact process and validation of the SPH impact model for aircraft structures [J]. Progress in Aerospace Sciences, 2022, 129: 100787. doi: 10.1016/j.paerosci.2021.100787
[2]	张迎春, 陆晓华, 张柱国. 运输类飞机鸟撞适航要求解析及审定实践 [M]. 北京: 科学出版社, 2023: 39–40. ZHANG Y C, LU X H, ZHANG Z G. Transport category airplane bird-strike airworthiness regulations interpretation and certification practice [M]. Beijing: Science Press, 2023: 39–40.
[3]	刘小川, 王计真, 白春玉. 人工鸟研究进展及在飞机结构抗鸟撞中的应用 [J]. 振动与冲击, 2021, 40(12): 80–89. doi: 10.13465/j.cnki.jvs.2021.12.011 LIU X C, WANG J Z, BAI C Y. Overview on artificial bird and application on the structural bird-strike [J]. Journal of Vibration and Shock, 2021, 40(12): 80–89. doi: 10.13465/j.cnki.jvs.2021.12.011
[4]	LAVOIE M A, GAKWAYA A, ENSAN M N, et al. Bird’s substitute tests results and evaluation of available numerical methods [J]. International Journal of Impact Engineering, 2009, 36(10/11): 1276–1287. doi: 10.1016/j.ijimpeng.2009.03.009
[5]	王富生, 李立州, 王新军, 等. 鸟体材料参数的一种反演方法 [J]. 航空学报, 2007, 28(2): 344–347. doi: 10.3321/j.issn:1000-6893.2007.02.018 WANG F S, LI L Z, WANG X J, et al. A method to identify bird’s material parameters [J]. Acta Aeronautica et Astronautica Sinica, 2007, 28(2): 344–347. doi: 10.3321/j.issn:1000-6893.2007.02.018
[6]	LIU J, LI Y L, GAO X S. Bird strike on a flat plate: experiments and numerical simulations [J]. International Journal of Impact Engineering, 2014, 70: 21–37. doi: 10.1016/j.ijimpeng.2014.03.006
[7]	王计真, 刘小川. 鸟撞平板试验与鸟体本构参数识别方法 [J]. 航空学报, 2017, 38(Suppl 1): 721550. doi: 10.7527/S1000-6893.2017.721550 WANG J Z, LIU X C. Test of bird striking on panel and identification method for bird constitutive parameters [J]. Acta Aeronautica et Astronautica Sinica, 2017, 38(Suppl 1): 721550. doi: 10.7527/S1000-6893.2017.721550
[8]	ZHANG Y L, ZHOU Y D. Evaluating constitutive models of smoothed particle hydrodynamics for bird-strike simulation [J]. International Journal of Crashworthiness, 2023: 1–10. doi: 10.1080/13588265.2023.2258650
[9]	YU P, YU S L, HE S, et al. Inversion for constitutive model parameters of bird in case of bird striking [J]. Shock and Vibration, 2022: 2456777. doi: 10.1155/2022/2456777
[10]	ZAKIR S M, LI Y L. Dynamic response of the leading edge wing under soft body impact [J]. International Journal of Crashworthiness, 2012, 17(4): 357–376. doi: 10.1080/13588265.2012.661239
[11]	冯振宇, 霍雨佳, 裴惠, 等. 明胶鸟弹撞击力传感器试验及数值建模方法研究 [J]. 振动与冲击, 2019, 38(12): 206–212. doi: 10.13465/j.cnki.jvs.2019.12.029 FENG Z Y, HUO Y J, PEI H, et al. An experiment and numerical modeling method of gelatin bird striking on force sensors [J]. Journal of Vibration and Shock, 2019, 38(12): 206–212. doi: 10.13465/j.cnki.jvs.2019.12.029
[12]	ASLAM M A, RAYHAN S B, KE Z G, et al. Ballistic gelatin Lagrange Mooney-Rivlin material model as a substitute of bird in finite element bird strike case studies [J]. Latin American Journal of Solids and Structures, 2020, 17(6): e298. doi: 10.1590/1679-78256215
[13]	PERNAS-SÁNCHEZ J, ARTERO-GUERRERO J, VARAS D, et al. Artificial bird strike on Hopkinson tube device: experimental and numerical analysis [J]. International Journal of Impact Engineering, 2020, 138: 103477. doi: 10.1016/j.ijimpeng.2019.103477
[14]	刘小川, 郭军, 孙侠生, 等. 用于鸟撞试验的仿真鸟弹研究 [J]. 实验力学, 2012, 27(5): 623–629. LIU X C, GUO J, SUN X S, et al. Investigation on the artificial bird projectile used in bird strike test [J]. Journal of Experimental Mechanics, 2012, 27(5): 623–629.
[15]	YANG X F, LIU M B, PENG S L. Smoothed particle hydrodynamics modeling of viscous liquid drop without tensile instability [J]. Computers & Fluids, 2014, 92: 199–208. doi: 10.1016/j.compfluid.2014.01.002
[16]	刘军, 李玉龙, 郭伟国, 等. 鸟撞45#钢平板动响应试验研究 [J]. 振动与冲击, 2013, 32(4): 15–20. doi: 10.3969/j.issn.1000-3835.2013.04.004 LIU J, LI Y L, GUO W G, et al. Tests for bird striking on a plate made of 45# steel [J]. Journal of Vibration and Shock, 2013, 32(4): 15–20. doi: 10.3969/j.issn.1000-3835.2013.04.004
[17]	HE Q G, CHEN X W, CHEN J F. Finite element-smoothed particle hydrodynamics adaptive method in simulating debris cloud [J]. Acta Astronautica, 2020, 175: 99–117. doi: 10.1016/j.actaastro.2020.05.056
[18]	LAVOIE M A, GAKWAYA A, ENSAN M N, et al. Validation of available approaches for numerical bird strike modeling tools [J]. International Review of Mechanical Engineering, 2007, 1(4): 380–389.
[19]	WILBECK J S. Impact behavior of low strength projectiles [D]. College Station: Texas A&M University, 1977.
[20]	JOHNSON A F, HOLZAPFEL M. Modelling soft body impact on composite structures [J]. Composite Structures, 2003, 61(1/2): 103–113. doi: 10.1016/S0263-8223(03)00033-3
[21]	AIROLDI A, CACCHIONE B. Modelling of impact forces and pressures in Lagrangian bird strike analyses [J]. International Journal of Impact Engineering, 2006, 32(10): 1651–1677. doi: 10.1016/j.ijimpeng.2005.04.011
[22]	JENQ S T, HSIAO F B, LIN I C, et al. Simulation of a rigid plate hit by a cylindrical hemi-spherical tip-ended soft impactor [J]. Computational Materials Science, 2007, 39(3): 518–526. doi: 10.1016/j.commatsci.2006.08.008
[23]	THO C H, SMITH M R. Accurate bird strike simulation methodology for BA609 tiltrotor [J]. Journal of the American Helicopter Society, 2011, 56(1): 12007. doi: 10.4050/JAHS.56.012007
[24]	MEGUID S A, MAO R H, NG T Y. FE analysis of geometry effects of an artificial bird striking an aeroengine fan blade [J]. International Journal of Impact Engineering, 2008, 35(6): 487–498. doi: 10.1016/j.ijimpeng.2007.04.008
[25]	SMOJVER I, IVANČEVIĆ D. Numerical simulation of bird strike damage prediction in airplane flap structure [J]. Composite Structures, 2010, 92(9): 2016–2026. doi: 10.1016/j.compstruct.2009.12.006
[26]	SMOJVER I, IVANČEVIĆ D. Advanced modelling of bird strike on high lift devices using hybrid Eulerian-Lagrangian formulation [J]. Aerospace Science and Technology, 2012, 23(1): 224–232. doi: 10.1016/j.ast.2011.07.010
[27]	HEDAYATI R, SADIGHI M, MOHAMMADI-AGHDAM M. On the difference of pressure readings from the numerical, experimental and theoretical results in different bird strike studies [J]. Aerospace Science and Technology, 2014, 32(1): 260–266. doi: 10.1016/j.ast.2013.10.008